University Physics with Modern Physics (14th Edition)
14th Edition
ISBN: 9780321973610
Author: Hugh D. Young, Roger A. Freedman
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 16.6, Problem 16.6TYU
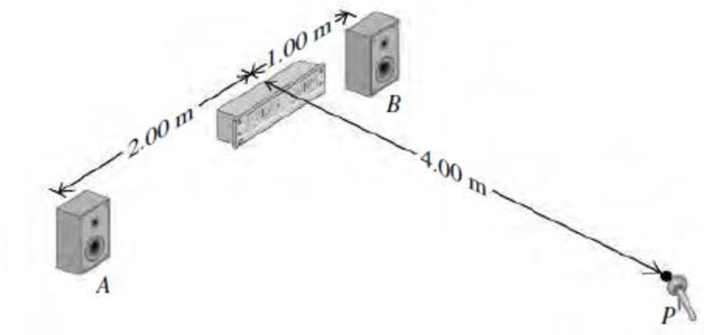
Suppose that speaker A in Fig. 16.23 emits a sinusoidal sound wave of frequency 500 Hz and speaker B emits a sinusoidal sound wave of frequency 1000 Hz. What sort of interference will there be between these two waves? (i) Constructive interference at various points, including point P, and destructive interference at various other points; (ii) destructive interference at various points, including point P, and constructive interference at various points; (iii) neither (i) nor (ii).
16.23 What sort of interference occurs at P?
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Checkpoint 4
The figure shows four orientations of an electric di-
pole in an external electric field. Rank the orienta-
tions according to (a) the magnitude of the torque
on the dipole and (b) the potential energy of the di-
pole, greatest first.
(1)
(2)
E
(4)
What is integrated science.
What is fractional distillation
What is simple distillation
19:39 ·
C
Chegg
1 69%
✓
The compound beam is fixed at Ę and supported by rollers at A and B. There are pins at C and D. Take
F=1700 lb. (Figure 1)
Figure
800 lb
||-5-
F
600 lb
بتا
D
E
C
BO
10 ft 5 ft 4 ft-—— 6 ft — 5 ft-
Solved Part A The compound
beam is fixed at E and...
Hình ảnh có thể có bản quyền. Tìm hiểu thêm
Problem
A-12
% Chia sẻ
kip
800 lb
Truy cập )
D Lưu
of
C
600 lb
|-sa+ 10ft 5ft 4ft6ft
D
E
5 ft-
Trying
Cheaa
Những kết quả này có
hữu ích không?
There are pins at C and D To F-1200 Egue!)
Chegg
Solved The compound b...
Có Không ☑
|||
Chegg
10
וח
Chapter 16 Solutions
University Physics with Modern Physics (14th Edition)
Ch. 16.1 - You use an electronic signal generator to produce...Ch. 16.2 - Mercury is 13.6 times denser than water. Based on...Ch. 16.3 - Prob. 16.3TYUCh. 16.4 - If you connect a hose to one end of a metal pipe...Ch. 16.5 - A stopped organ pipe of length L has a fundamental...Ch. 16.6 - Suppose that speaker A in Fig. 16.23 emits a...Ch. 16.7 - One tuning fork vibrates at 440 Hz, while a second...Ch. 16.8 - You are at an outdoor concert with a wind blowing...Ch. 16.9 - What would you hear if you were directly behind...Ch. 16 - Prob. 16.1DQ
Ch. 16 - The hero of a western movie listens for an...Ch. 16 - Would you expect the pitch (or frequency) of an...Ch. 16 - In most modern wind instruments the pitch is...Ch. 16 - Symphonic musicians always warm up their wind...Ch. 16 - In a popular and amusing science demonstration, a...Ch. 16 - Prob. 16.7DQCh. 16 - (a) Does a sound level of 0 dB mean that there is...Ch. 16 - Which has a more direct influence on the loudness...Ch. 16 - If the pressure amplitude of a sound wave is...Ch. 16 - Does the sound intensity level obey the...Ch. 16 - A small fraction of the energy in a sound wave is...Ch. 16 - A small metal band is slipped onto one of the...Ch. 16 - An organist in a cathedral plays a loud chord and...Ch. 16 - Prob. 16.15DQCh. 16 - Two vibrating tuning forks have identical...Ch. 16 - A large church has part of the organ in the front...Ch. 16 - A sound source and a listener are both at rest on...Ch. 16 - Can you think of circumstances in which a Doppler...Ch. 16 - Prob. 16.20DQCh. 16 - If you wait at a railroad crossing as a train...Ch. 16 - In case 1, a source of sound approaches a...Ch. 16 - Does an aircraft make a sonic boom only at the...Ch. 16 - If you are riding in a supersonic aircraft, what...Ch. 16 - Prob. 16.25DQCh. 16 - Example 16.1 (Section 16.1) showed that for sound...Ch. 16 - Prob. 16.2ECh. 16 - Consider a sound wave in air that has displacement...Ch. 16 - A loud factory machine produces sound having a...Ch. 16 - BIO Ultrasound and Infrasound. (a) Whale...Ch. 16 - (a) In a liquid with density 1300 kg/m3,...Ch. 16 - A submerged scuba diver hears the sound of a boat...Ch. 16 - Prob. 16.8ECh. 16 - An oscillator vibrating at 1250 Hz produces a...Ch. 16 - CALC (a) Show that the fractional change in the...Ch. 16 - A 60.0-m-long brass rod is struck at one end. A...Ch. 16 - Prob. 16.12ECh. 16 - BIO Energy Delivered to the Ear. Sound is detected...Ch. 16 - (a) By what factor must the sound intensity be...Ch. 16 - Eavesdropping! You are trying to overhear a juicy...Ch. 16 - BIO Human Hearing. A fan at a rock concert is 30 m...Ch. 16 - A sound wave in air at 20C has a frequency of 320...Ch. 16 - You live on a busy street, but as a music lover,...Ch. 16 - BIO For a person with normal hearing, the faintest...Ch. 16 - The intensity due to a number of independent sound...Ch. 16 - CP A babys mouth is 30 cm from her fathers ear and...Ch. 16 - The Sacramento City Council adopted a law to...Ch. 16 - CP At point A, 3.0 m from a small source of sound...Ch. 16 - (a) If two sounds differ by 5.00 dB, find the...Ch. 16 - Standing sound waves are produced in a pipe that...Ch. 16 - The fundamental frequency of a pipe that is open...Ch. 16 - Prob. 16.27ECh. 16 - BIO The Vocal Tract. Many opera singers (and some...Ch. 16 - The longest pipe found in most medium-size pipe...Ch. 16 - Singing in the Shower. A pipe closed at both ends...Ch. 16 - You blow across the open mouth of an empty test...Ch. 16 - Prob. 16.32ECh. 16 - A 75.0-cm-long wire of mass 5.625 g is tied at...Ch. 16 - Small speakers A and B are driven in phase at 725...Ch. 16 - Prob. 16.35ECh. 16 - Two loudspeakers, A and B (see Fig. E16.35), are...Ch. 16 - Two loudspeakers, A and B, are driven by the same...Ch. 16 - Two loudspeakers, A and B, are driven by the same...Ch. 16 - Two small stereo speakers are driven in step by...Ch. 16 - Two guitarists attempt to play the same note of...Ch. 16 - Prob. 16.41ECh. 16 - Adjusting Airplane Motors. The motors that drive...Ch. 16 - Two organ pipes, open at one end but closed at the...Ch. 16 - In Example 16.18 (Section 16.8), suppose the...Ch. 16 - On the planet Arrakis a male ornithoid is flying...Ch. 16 - A railroad train is traveling at 25.0 m/s in still...Ch. 16 - Two train whistles, A and B, each have a frequency...Ch. 16 - Moving Source vs. Moving Listener. (a) A sound...Ch. 16 - A swimming duck puddles the water with its feet...Ch. 16 - A railroad train is traveling at 30.0 m/s in still...Ch. 16 - A car alarm is emitting sound waves of frequency...Ch. 16 - While sitting in your car by the side of a country...Ch. 16 - Prob. 16.53ECh. 16 - The siren of a fire engine that is driving...Ch. 16 - A stationary police car emits a sound of frequency...Ch. 16 - How fast (as a percentage of light speed) would a...Ch. 16 - A jet plane flies overhead at Mach 1.70 and at a...Ch. 16 - The shock-wave cone created by a space shuttle at...Ch. 16 - A soprano and a bass are singing a duet. While the...Ch. 16 - CP The sound from a trumpet radiates uniformly in...Ch. 16 - Prob. 16.61PCh. 16 - CP A uniform 165-N bar is supported horizontally...Ch. 16 - An organ pipe has two successive harmonics with...Ch. 16 - Prob. 16.64PCh. 16 - Prob. 16.65PCh. 16 - A bat flies toward a wall, emitting a steady sound...Ch. 16 - The sound source of a ships sonar system operates...Ch. 16 - BIO Ultrasound in Medicine. A 2.00-MHZ sound wave...Ch. 16 - BIO Horseshoe bats (genus Rhinolophus) emit sounds...Ch. 16 - CP A police siren of frequency fsiren is attached...Ch. 16 - CP A turntable 1.50 m in diameter rotates at 75...Ch. 16 - DATA A long, closed cylindrical tank contains a...Ch. 16 - Prob. 16.73PCh. 16 - DATA Supernova! (a) Equation (16.30) can be...Ch. 16 - CALC Figure P16.75 shows the pressure fluctuation...Ch. 16 - CP Longitudinal Waves on a Spring. A long spring...Ch. 16 - BIO ULTRASOUND IMAGING. A typical ultrasound...Ch. 16 - BIO ULTRASOUND IMAGING. A typical ultrasound...Ch. 16 - BIO ULTRASOUND IMAGING. A typical ultrasound...Ch. 16 - BIO ULTRASOUND IMAGING. A typical ultrasound...Ch. 16 - BIO ULTRASOUND IMAGING. A typical ultrasound...
Additional Science Textbook Solutions
Find more solutions based on key concepts
WHAT IF? Suppose a new fishery is discovered, and you are put in charge of developing it sustainably. What ecol...
Campbell Biology in Focus (2nd Edition)
1.1 Write a one-sentence definition for each of the following:
a. chemistry
b. chemical
Chemistry: An Introduction to General, Organic, and Biological Chemistry (13th Edition)
The pHactivity profile for glucose-6-phosphate isomerase indicates the participation of a group with a pKa = 6....
Organic Chemistry (8th Edition)
Heat lamps are commonly used to maintain foods at about 50C for as long as 12 hours in cafeteria serving lines....
Microbiology: An Introduction
Where is transitional epithelium found and what is its importance at those sites?
Anatomy & Physiology (6th Edition)
27. Consider the reaction.
Express the rate of the reaction in terms of the change in concentration of each of...
Chemistry: Structure and Properties (2nd Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- air is pushed steadily though a forced air pipe at a steady speed of 4.0 m/s. the pipe measures 56 cm by 22 cm. how fast will air move though a narrower portion of the pipe that is also rectangular and measures 32 cm by 22 cmarrow_forwardNo chatgpt pls will upvotearrow_forward13.87 ... Interplanetary Navigation. The most efficient way to send a spacecraft from the earth to another planet is by using a Hohmann transfer orbit (Fig. P13.87). If the orbits of the departure and destination planets are circular, the Hohmann transfer orbit is an elliptical orbit whose perihelion and aphelion are tangent to the orbits of the two planets. The rockets are fired briefly at the depar- ture planet to put the spacecraft into the transfer orbit; the spacecraft then coasts until it reaches the destination planet. The rockets are then fired again to put the spacecraft into the same orbit about the sun as the destination planet. (a) For a flight from earth to Mars, in what direction must the rockets be fired at the earth and at Mars: in the direction of motion, or opposite the direction of motion? What about for a flight from Mars to the earth? (b) How long does a one- way trip from the the earth to Mars take, between the firings of the rockets? (c) To reach Mars from the…arrow_forward
- No chatgpt pls will upvotearrow_forwarda cubic foot of argon at 20 degrees celsius is isentropically compressed from 1 atm to 425 KPa. What is the new temperature and density?arrow_forwardCalculate the variance of the calculated accelerations. The free fall height was 1753 mm. The measured release and catch times were: 222.22 800.00 61.11 641.67 0.00 588.89 11.11 588.89 8.33 588.89 11.11 588.89 5.56 586.11 2.78 583.33 Give in the answer window the calculated repeated experiment variance in m/s2.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Principles of Physics: A Calculus-Based Text
Physics
ISBN:9781133104261
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
University Physics Volume 1
Physics
ISBN:9781938168277
Author:William Moebs, Samuel J. Ling, Jeff Sanny
Publisher:OpenStax - Rice University
Physics for Scientists and Engineers: Foundations...
Physics
ISBN:9781133939146
Author:Katz, Debora M.
Publisher:Cengage Learning
What Are Sound Wave Properties? | Physics in Motion; Author: GPB Education;https://www.youtube.com/watch?v=GW6_U553sK8;License: Standard YouTube License, CC-BY