
For the circuit in Fig. 15.57, (a) determine the transfer function H(s) = Vout/Vin in terms of circuit parameters R1, R2, R3, L1, and L2; (b) determine the magnitude and phase of the transfer function at ω = 0, 3 × 103 rad/s, and as ω → ∞ for the case where circuit values are R1 = 2 kΩ, R2 = 2 kΩ, R3 = 20 kΩ, L1 = 2 H, and L2 = 2 H.
FIGURE 15.57
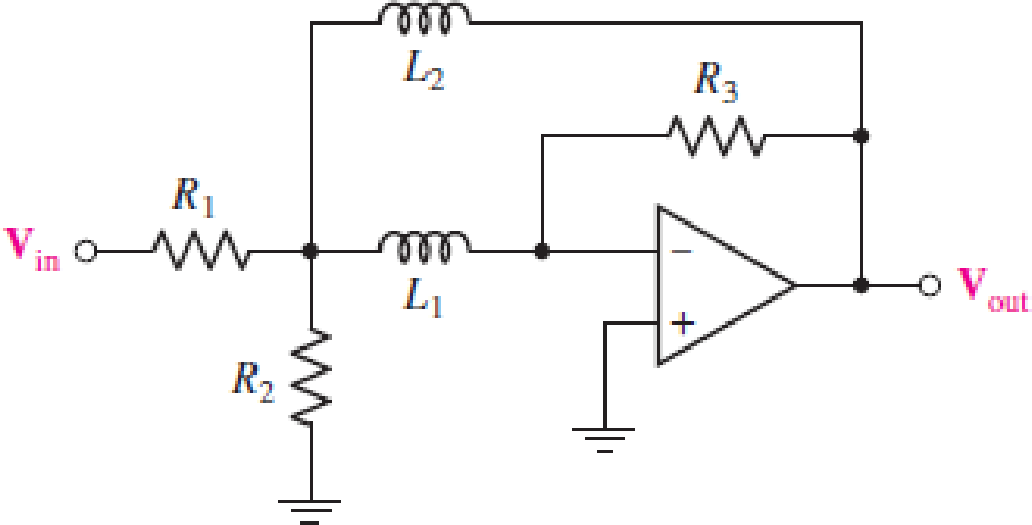
(a)
The transfer function
Answer to Problem 7E
The transfer function
Explanation of Solution
Given data:
The required diagram is shown in Figure 1.
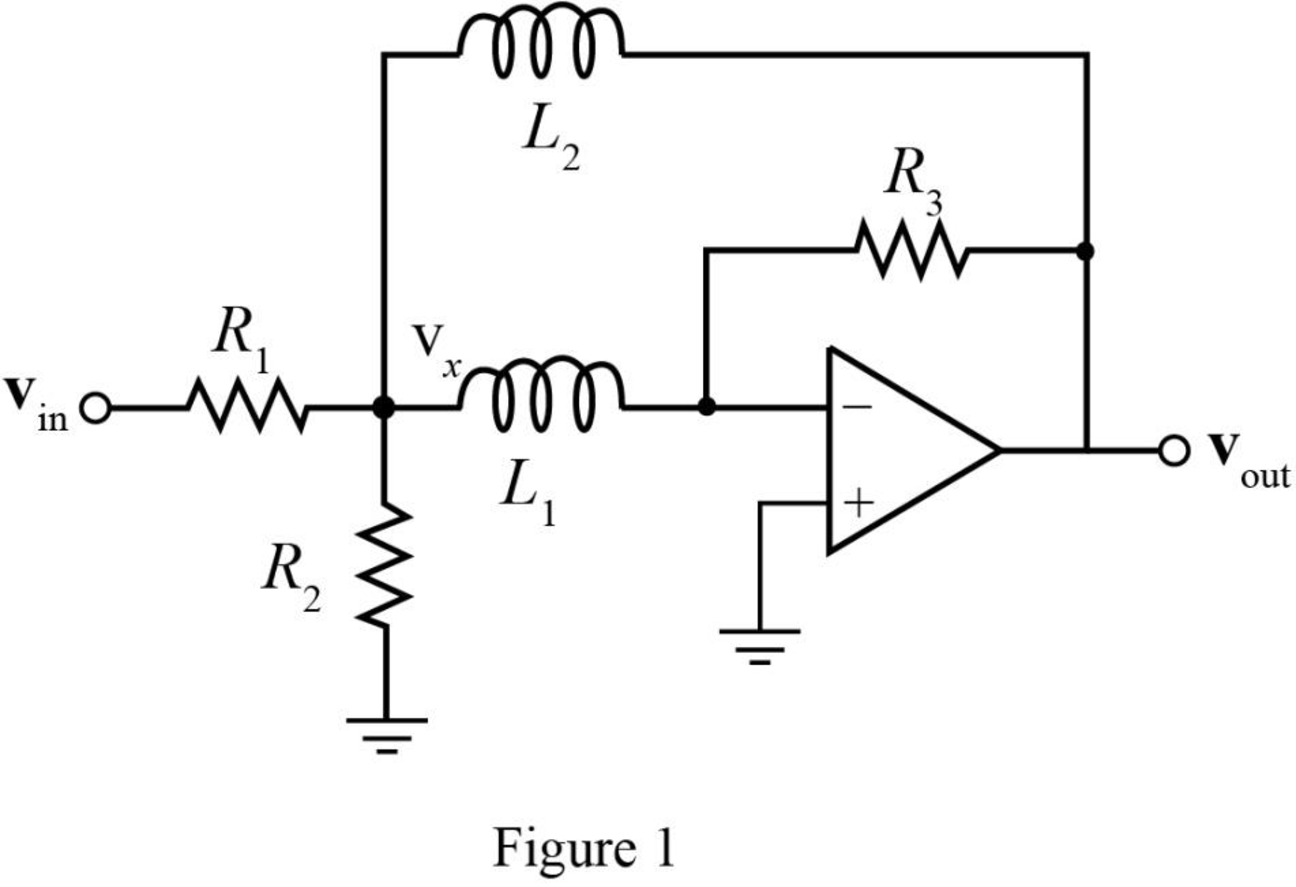
Calculation:
Assign the node intersecting the two resistors
The KCL equation at node
The KCL equation at node
Here,
Simplifying the equation (2) as,
The transfer function
Substitute
Substitute
Conclusion:
Therefore, the transfer function
(b)
The magnitude of transfer function
Answer to Problem 7E
The magnitude of transfer function
Explanation of Solution
Given data:
The resistance
The resistance
The resistance
The inductance
The inductance
The angular frequency
The angular frequency
The angular frequency
Calculation:
The conversion of
The conversion of
The conversion of
The magnitude of transfer function
The phase of transfer function
Substitute
Substitute
The magnitude of transfer function
The phase of transfer function
Substitute
Substitute
The magnitude of transfer function
The phase of transfer function
Substitute
Substitute
Conclusion:
Therefore, the magnitude of transfer function
Want to see more full solutions like this?
Chapter 15 Solutions
Loose Leaf for Engineering Circuit Analysis Format: Loose-leaf
Additional Engineering Textbook Solutions
Database Concepts (8th Edition)
Mechanics of Materials (10th Edition)
Starting Out with Programming Logic and Design (5th Edition) (What's New in Computer Science)
SURVEY OF OPERATING SYSTEMS
Elementary Surveying: An Introduction To Geomatics (15th Edition)
Java: An Introduction to Problem Solving and Programming (8th Edition)
- (i) Find the inverse z-transform of the system H(z) = for the following regions of convergence. Write in the final answer for each case in the allocated rectangular box below (a) |z| 3 (c) 1arrow_forwardQ3: Material A and Material B are collected in a tank as shown where the system consists of three Push-Button, three Level Sensors, two Inlet valve, one Outlet valve, Heater, Temperature Sensor, Agitator Motor, and Alarm Light. Material A and Material B are to be mixed and heated until it reaches 90°C temperature, and it will be drain using outlet valve also high-level Alarm Light will come ON when the tank is full and stay on even if the tank level drops until the operator press Reset Push-Button. Implement automation of this system in PLC using Ladder Diagram programming language (Note: The tank is fed with Material A before B and the temperature sensor can withstand 200°C and it gives voltage from 0 to 10 volts) (25 Marks) Valve A Agitator Motor Valve B Level B Heater E Level A Low Level Sta Start Push-Button Stop Push-Button 36. ویر نکند Temperature sensor Outlet Valve Reset Push-Button Alarm Lightarrow_forward.Explain how a gated J-K latch operates differently from an edge-triggered J-K flip-flop. . For the gated T Latch circuit, answer the following: a) Draw the gate-level diagram of a gated T latch using basic logic gates and SR latch b) Write the characteristic equation. c) Draw the state diagram.arrow_forwardA Digital Filter is described by the following. difference equation: Y(n)=0.5x(n) 0.5(n-2) - Find the transfer function ..arrow_forwardQ4) answer just two from three the following terms: A) Design ADC using the successive method if the Vmax=(3) volt, Vmin=(-2) volt, demonstrate the designing system for vin-1.2 volt.arrow_forward(a) For a voltage phasor V(jω) and a current phasor I(jω), give an expression for the complex power.(b)Give three examples of how real (average) power might be dissipated.(c)A time-domain voltage is defined by the expression v(t)= 5 cos(πt/3) V. When this is applied across an impedance Z = 4∠60° Ω, determine:(i)The instantaneous power.(ii)The average power.arrow_forwardConsider the LTI system with the input x(t) = e^28(t) and the impulse response h(t) = e−²tu(t). a) Determine the Laplace transform of x(t) and h(t). (10 marks) b) Using convolutional property, determine the Laplace transform and the ROC for the output response y(t).arrow_forward解出R1和R2arrow_forwardAccording to the book the answers are m= 30 and n = 5 and number of switch blocks is 220arrow_forwardfind reactive power demand , capacitor bank provides and overcompenstationarrow_forward(A) Consider a communication system where the number of successful transsions out of 10 trials follows a binomial distribution. The success probability for each triat is 0,95, Let X be the random variable representing the number of successful transmissions. -Sketch the cumulative distribution function (CDF) of the distribution. 2- Find Skewness coefficients and check if the distribution is symmetrical or skewed to the right or left. 3- Find kurtosis coefficients, Check if the distribution is mesokurtic, leptokurtic or platykurtic. 4- Find the probability of getting at most eigh. successful transmissions. 5- Find the probability P(20 with a mean 2-1 calculate the probability that the noise is greater than 3 units.arrow_forwardQ4: (A) Find the mean of a random variable X if S f(x)= 2x 0 2 for 0arrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_iosRecommended textbooks for you
 Introductory Circuit Analysis (13th Edition)Electrical EngineeringISBN:9780133923605Author:Robert L. BoylestadPublisher:PEARSON
Introductory Circuit Analysis (13th Edition)Electrical EngineeringISBN:9780133923605Author:Robert L. BoylestadPublisher:PEARSON Delmar's Standard Textbook Of ElectricityElectrical EngineeringISBN:9781337900348Author:Stephen L. HermanPublisher:Cengage Learning
Delmar's Standard Textbook Of ElectricityElectrical EngineeringISBN:9781337900348Author:Stephen L. HermanPublisher:Cengage Learning Programmable Logic ControllersElectrical EngineeringISBN:9780073373843Author:Frank D. PetruzellaPublisher:McGraw-Hill Education
Programmable Logic ControllersElectrical EngineeringISBN:9780073373843Author:Frank D. PetruzellaPublisher:McGraw-Hill Education Fundamentals of Electric CircuitsElectrical EngineeringISBN:9780078028229Author:Charles K Alexander, Matthew SadikuPublisher:McGraw-Hill Education
Fundamentals of Electric CircuitsElectrical EngineeringISBN:9780078028229Author:Charles K Alexander, Matthew SadikuPublisher:McGraw-Hill Education Electric Circuits. (11th Edition)Electrical EngineeringISBN:9780134746968Author:James W. Nilsson, Susan RiedelPublisher:PEARSON
Electric Circuits. (11th Edition)Electrical EngineeringISBN:9780134746968Author:James W. Nilsson, Susan RiedelPublisher:PEARSON Engineering ElectromagneticsElectrical EngineeringISBN:9780078028151Author:Hayt, William H. (william Hart), Jr, BUCK, John A.Publisher:Mcgraw-hill Education,
Engineering ElectromagneticsElectrical EngineeringISBN:9780078028151Author:Hayt, William H. (william Hart), Jr, BUCK, John A.Publisher:Mcgraw-hill Education,
 Introductory Circuit Analysis (13th Edition)Electrical EngineeringISBN:9780133923605Author:Robert L. BoylestadPublisher:PEARSON
Introductory Circuit Analysis (13th Edition)Electrical EngineeringISBN:9780133923605Author:Robert L. BoylestadPublisher:PEARSON Delmar's Standard Textbook Of ElectricityElectrical EngineeringISBN:9781337900348Author:Stephen L. HermanPublisher:Cengage Learning
Delmar's Standard Textbook Of ElectricityElectrical EngineeringISBN:9781337900348Author:Stephen L. HermanPublisher:Cengage Learning Programmable Logic ControllersElectrical EngineeringISBN:9780073373843Author:Frank D. PetruzellaPublisher:McGraw-Hill Education
Programmable Logic ControllersElectrical EngineeringISBN:9780073373843Author:Frank D. PetruzellaPublisher:McGraw-Hill Education Fundamentals of Electric CircuitsElectrical EngineeringISBN:9780078028229Author:Charles K Alexander, Matthew SadikuPublisher:McGraw-Hill Education
Fundamentals of Electric CircuitsElectrical EngineeringISBN:9780078028229Author:Charles K Alexander, Matthew SadikuPublisher:McGraw-Hill Education Electric Circuits. (11th Edition)Electrical EngineeringISBN:9780134746968Author:James W. Nilsson, Susan RiedelPublisher:PEARSON
Electric Circuits. (11th Edition)Electrical EngineeringISBN:9780134746968Author:James W. Nilsson, Susan RiedelPublisher:PEARSON Engineering ElectromagneticsElectrical EngineeringISBN:9780078028151Author:Hayt, William H. (william Hart), Jr, BUCK, John A.Publisher:Mcgraw-hill Education,
Engineering ElectromagneticsElectrical EngineeringISBN:9780078028151Author:Hayt, William H. (william Hart), Jr, BUCK, John A.Publisher:Mcgraw-hill Education,