Chapter 15, Problem 15.4P

To determine

Find the factors of safety with respect to overturning, sliding, and bearing capacity failure.

Answer to Problem 15.4P

The factor of safety with respect to overturning is $\underset{\_}{4.37}$.

The factor of safety with respect to sliding is $\underset{\_}{1.58}$.

The factor of safety with respect to bearing capacity failure is $\underset{\_}{2.81}$.

Explanation of Solution

Given information:

The cohesion $\left({c^{\prime }}_1\right)$ of backfill soil is 0.

The unit weight $\left({\gamma }_1\right)$ of the backfill soil is $18.0{\text{kN/m}}^3$.

The friction angle $\left({{\varphi }^{\prime }}_1\right)$ of the backfill soil is $34°$.

The unit weight $\left({\gamma }_c\right)$ of the concrete is $24.0{\text{kN/m}}^3$.

The cohesion $\left({c^{\prime }}_2\right)$ of soil in front of the heel and under the base is $10{\text{kN/m}}^2$.

The unit weight of soil $\left({\gamma }_2\right)$ in front of the heel and under the base is $19.0{\text{kN/m}}^3$.

The friction angle $\left({{\varphi }^{\prime }}_2\right)$ of the soil in front of the heel and under the base is $24°$.

The backfill angle $\left(\alpha \right)$ is $10°$.

Calculation:

Check stability with respect to overturning.

Consider point C as the left end of the toe base as named as C.

Divide the retaining wall into section as in Figure 1.

Sketch the section of the retaining wall as shown in Figure 1.

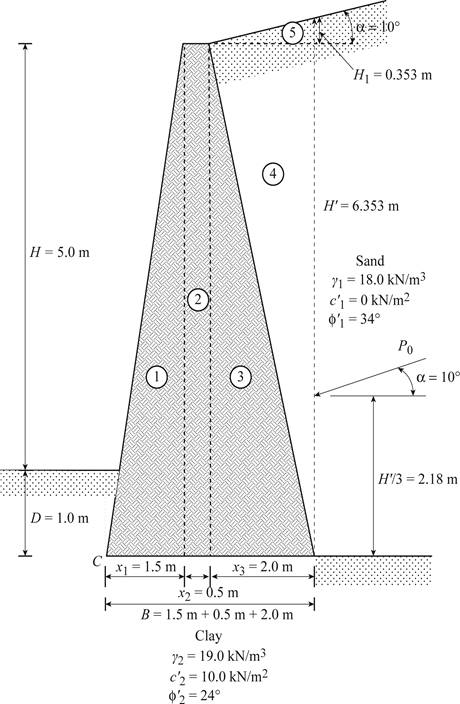
Here, $P_0$ is the resultant thrust on the retaining wall.

Refer Table 14.2, “Values of $K_a$” in the textbook.

Take the value of active earth pressure coefficient $\left(K_a\right)$ with corresponding inclination of backfill $\left(\alpha \right)$ of $10°$ and effective soil friction angle $\left({\varphi }^{\prime }\right)$ of $34°$ is 0.2944.

Refer Figure 1.

Find the height of the inclined portion of backfill $\left(H_1\right)$, using the tangent rule:

$\begin{array}{l}\tan \alpha =\frac{H_1}{x_3}\\ H_1=x_3\tan \alpha \end{array}$

Substitute 2 m for $x_3$ and $10°$ for $\alpha$.

$\begin{array}{c}{H}_1=2\times \tan 10°\\ =0.353\text{m}\end{array}$

Find the total height of the inclined backfill $\left(H^{\prime }\right)$.

$H^{\prime }=H+D+H_1$

Here, H is the height of retaining wall and D is the depth to the bottom of the base slab.

Substitute 5.0 m for H, 1.0 m for D, and 0.353 m $H_1$.

$\begin{array}{c}{H}^{\prime }=5+1.0+0.353\\ =6.353\text{m}\end{array}$

Find the active earth pressure $\left(P_a\right)$ on the retaining wall due to the backfill using the equation:

$P_a=\frac{1}{2}{\gamma }_1{\left(H^{\prime }\right)}^2{K}_a$

Substitute $18{\text{kN/m}}^{\text{3}}$ for ${\gamma }_1$, 6.353 m for $H^{\prime }$, and 0.2994 for $K_a$.

$\begin{array}{c}{P}_a=\frac{1}{2}\times 18\times {6.353}^2\times 0.2994\\ =108.76\text{kN}/\text{m}\end{array}$

Find the vertical component of the active earth pressure $\left(P_v\right)$ using the equation:

$P_v=P_a\sin \alpha$

Substitute $108.76\text{kN}/\text{m}$ for $P_a$ and $10°$ for $\alpha$.

$\begin{array}{c}{P}_v=108.76\times \sin 10°\\ =18.89\text{kN}/\text{m}\end{array}$

Find the horizontal component of the active earth pressure $\left(P_h\right)$ using the equation:

$P_h=P_a\cos \alpha$

Substitute $108.76\text{kN}/\text{m}$ for $P_a$ and $10°$ for $\alpha$.

$\begin{array}{c}{P}_h=108.76\times \cos 10°\\ =107.11\text{kN}/\text{m}\end{array}$

Find the weight of section 1 $\left(W_1\right)$.

$\begin{array}{c}{W}_1=A_1{\gamma }_c\\ =\left(\frac{1}{2}{x}_{1}H\right){\gamma }_c\end{array}$

Here, $A_1$ is the area of the section 1.

Substitute 1.5 m for $x_1$, 6 m for H, and $24{\text{kN/m}}^{\text{3}}$ for ${\gamma }_c$.

$\begin{array}{c}{W}_1=\frac{1}{2}\times 1.5\times 6\times 24\\ =108\text{kN}/\text{m}\end{array}$

Find the moment arm or lever arm $\left[{\left(MA\right)}_1\right]$ of section 1 from point C.

${\left(MA\right)}_1=\frac{2}{3}{x}_1$

Substitute 1.5 m for $x_1$.

$\begin{array}{c}{\left(MA\right)}_1=\frac{2}{3}\times 1.5\text{m}\\ =1.0\text{m}\end{array}$

Find the moment about point C $\left(M_{C,1}\right)$ due to the self-weight of section 1:

$M_{C,1}=W_1\times {\left(MA\right)}_1$

Substitute $108\text{kN}/\text{m}$ for $W_1$ and 1.0 m for ${\left(MA\right)}_1$.

$\begin{array}{c}{M}_{C,1}=108\times 1.0\\ =108\text{kN}\end{array}$

Find the weight of section 2 $\left(W_2\right)$.

$\begin{array}{c}{W}_2=A_2{\gamma }_c\\ =\left(x_{2}H\right){\gamma }_c\end{array}$

Here, $A_2$ is the area of the section 2.

Substitute 0.5 m for $x_2$, 6 m for H, and $24{\text{kN/m}}^{\text{3}}$ for ${\gamma }_c$.

$\begin{array}{c}{W}_2=0.5\times 6\times 24\\ =72\text{kN}/\text{m}\end{array}$

Find the moment arm or lever arm $\left[{\left(MA\right)}_2\right]$ of section 2 from point C.

${\left(MA\right)}_2=x_1+\frac{x_2}{2}$

Substitute 1.5 m for $x_1$ and 0.5 m for $x_2$.

$\begin{array}{c}{\left(MA\right)}_2=1.5\text{m}+\frac{0.5\text{m}}{2}\\ =1.75\text{m}\end{array}$

Find the moment about point C $\left(M_{C,2}\right)$ due to the self-weight of section 2.

$M_{C,2}=W_2\times {\left(MA\right)}_2$

Substitute $72\text{kN}/\text{m}$ for $W_2$ and 1.75 m for ${\left(MA\right)}_2$.

$\begin{array}{c}{M}_{C,2}=72\times 1.75\\ =126\text{kN}\end{array}$

Find the weight of section 3 $\left(W_3\right)$.

$\begin{array}{c}{W}_3=A_3{\gamma }_c\\ =\left(\frac{1}{2}{x}_{3}H\right){\gamma }_c\end{array}$

Here, $A_3$ is the area of the section 3.

Substitute 2.0 m for $x_3$, 6 m for H, and $24{\text{kN/m}}^{\text{3}}$ for ${\gamma }_c$.

$\begin{array}{c}{W}_3=\frac{1}{2}\times 2.0\times 6\times 24\\ =144\text{kN}/\text{m}\end{array}$

Find the moment arm or lever arm $\left[{\left(MA\right)}_3\right]$ of section 3 from point C.

${\left(MA\right)}_3=x_1+x_2+\frac{x_3}{3}$

Substitute 0.5 m for $x_2$, 1.5 m for $x_1$, and 2.0 m for $x_3$.

$\begin{array}{c}{\left(MA\right)}_3=1.5+\text{0}\text{.5}+\frac{2.0}{3}\\ =2.67\text{m}\end{array}$

Find the moment about point C $\left(M_{C,3}\right)$ due to the self-weight of section 3.

$M_{C,3}=W_3\times {\left(MA\right)}_3$

Substitute $144\text{kN}/\text{m}$ for $W_3$ and 2.67 m for ${\left(MA\right)}_3$.

$\begin{array}{c}{M}_{C,3}=144\text{kN}/\text{m}\times 2.67\text{m}\\ =384.5\text{kN}\end{array}$

Find the weight of section 4 $\left(W_4\right)$.

$\begin{array}{c}{W}_4=A_4{\gamma }_1\\ =\left(\frac{1}{2}{x}_{3}H\right){\gamma }_1\end{array}$

Here, $A_4$ is the area of the section 4.

Substitute 2.0 m for $x_3$, 6 m for H, and $18{\text{kN/m}}^{\text{3}}$ for ${\gamma }_1$.

$\begin{array}{c}{W}_4=\frac{1}{2}\times 2.0\times 6\times 18\\ =108\text{kN}/\text{m}\end{array}$

Find the moment arm or lever arm $\left[{\left(MA\right)}_4\right]$ of section 4 from point C.

${\left(MA\right)}_4=x_1+x_2+\frac{2}{3}{x}_3$

Substitute 2.0 m for $x_3$, 0.5 m for $x_2$, and 1.5 m for $x_1$.

$\begin{array}{c}{\left(MA\right)}_4=1.5+0.5+\frac{2}{3}\times 2.0\\ =3.33\text{m}\end{array}$

Find the moment about point C $\left(M_{C,4}\right)$ due to the self-weight of section 4.

$M_{C,4}=W_4\times {\left(MA\right)}_4$

Substitute 108 kN/m for $W_4$ and 3.33 m for ${\left(MA\right)}_4$.

$\begin{array}{c}{M}_{C,4}=108\times 3.33\\ =359.6\text{kN}\end{array}$

Find the weight of section 5 $\left(W_5\right)$.

$\begin{array}{c}{W}_5=A_5{\gamma }_1\\ =\left(\frac{1}{2}{x}_3{H}_1\right){\gamma }_1\end{array}$

Substitute 2 m for $x_3$, 0.353 m for H, and $18{\text{kN/m}}^{\text{3}}$ for ${\gamma }_1$.

$\begin{array}{c}{W}_5=\frac{1}{2}\times 2.0\times 0.353\times 18\\ =6.35\text{kN}/\text{m}\end{array}$

Find the moment arm or lever arm $\left[{\left(MA\right)}_5\right]$ of section 4 from point C.

$\left[{\left(MA\right)}_5\right]=x_1+x_2+\frac{2}{3}{x}_3$

Substitute 2.0 m for $x_3$, 0.5 m for $x_2$, and 1.5 m for $x_1$.

$\begin{array}{c}{\left(MA\right)}_4=1.5+0.5+\frac{2}{3}\times 2.0\\ =3.33\text{m}\end{array}$

Find the moment about point C $\left(M_{C,5}\right)$ due to the self-weight of section 5.

$M_{C,5}=W_5\times {\left(MA\right)}_5$

Substitute $6.35\text{kN}/\text{m}$ for $W_5$ and 3.33 m for ${\left(MA\right)}_4$.

$\begin{array}{c}{M}_{C,5}=6.35\times 3.33\\ =21.15\text{kN}\end{array}$

Find the moment arm or lever arm $\left[{\left(MA\right)}_{P_v}\right]$ of vertical component of active earth pressure from point C.

${\left(MA\right)}_{P_v}=x_1+x_2+x_3$

Substitute 0.5 m for $x_2$, 1.5 m for $x_1$, and 2.0 m for $x_3$.

$\begin{array}{c}{\left(MA\right)}_{P_v}=1.5+0.5+2.0\\ =4.0\text{m}\end{array}$

Find the moment about point C $\left(M_{C,P_v}\right)$ due to the vertical component of active earth pressure.

$M_{C,P_v}=P_v\times {\left(MA\right)}_{P_v}$

Substitute $18.89\text{kN}/\text{m}$ for $P_v$ and 4.0 m for ${\left(MA\right)}_{P_v}$.

$\begin{array}{c}{M}_{C,P_v}=18.89\times 4\\ =75.56\text{kN}\end{array}$

Find the total moment about the point C $\left({\displaystyle \sum M_C}\right)$.

${\displaystyle \sum M_C}=M_{C,1}+M_{C,2}+M_{C,3}+M_{C,4}+M_{C,5}+M_{C,P_v}$

Substitute $108\text{kN-m}/\text{m}$ for $M_{C,1}$, $126\text{kN-m}/\text{m}$ for $M_{C,2}$, $384.5\text{kN-m}/\text{m}$ for $M_{C,3}$, $359.6\text{kN-m}/\text{m}$ for $M_{C,4}$, $21.15\text{kN-m}/\text{m}$ for $M_{C,5}$, and $75.56\text{kN-m}/\text{m}$ for $M_{C,P_v}$.

$\begin{array}{c}{\displaystyle \sum M_C}=\left(108+126+384.5+359.6+21.15+75.56\right)\\ =1,074.8\text{kN-m}/\text{m}\end{array}$

Find the total vertical load $\left({\displaystyle \sum V}\right)$ due to the retaining wall and the inclined backfill.

${\displaystyle \sum V}=W_1+W_2+W_3+W_4+W_5+P_v$

Substitute $108\text{kN}/\text{m}$ for $W_1$, $72\text{kN}/\text{m}$ for $W_2$, $144\text{kN}/\text{m}$ for $W_3$, $108\text{kN}/\text{m}$ for $W_4$, $6.35\text{kN}/\text{m}$ for $W_5$, and $18.89\text{kN}/\text{m}$ for $P_v$.

$\begin{array}{c}{\displaystyle \sum V}=\left(108+72+144+108+6.35+18.89\right)\\ =475.24\text{kN}/\text{m}\end{array}$

Summarize the values of weight, moment arm from C, and moment about C as shown in Table 1.

Section	weight	moment arm from C	moment about C
1	108	1	108
2	72	1.75	126
3	144	2.67	384.5
4	108	3.33	359.6
5	6.35	3.33	21.15
	$p_v=18.89$	4	75.56
	${\displaystyle \sum V}=475.24$		${\displaystyle \sum M_R=1,074.8}$

Find the overturning moment $\left(M_O\right)$ due the horizontal component of the active earth pressure using the equation:

$M_O=P_h\frac{H^{\prime }}{3}$

Substitute $107.11\text{kN}/\text{m}$ for $P_h$ and 6.353 m for $H^{\prime }$.

$\begin{array}{c}{M}_O=107.11\times \frac{6.353}{3}\\ =226.82\text{kN-m}/\text{m}\end{array}$

Find the factor of safety $\left[F{S}_{\left(\text{overturning}\right)}\right]$ about the toe (point C) of the retaining wall using the equation:

$F{S}_{\left(\text{overturning}\right)}=\frac{{\displaystyle \sum M_R}}{M_O}$

Substitute $1,074.8\text{kN-m}/\text{m}$ for ${\displaystyle \sum M_R}$ and $226.82\text{kN-m}/\text{m}$ for $M_O$.

$\begin{array}{c}F{S}_{\left(\text{overturning}\right)}=\frac{1,074.8}{226.82}\\ =4.74\end{array}$

Therefore, the factor of safety with respect to overturning is $\underset{\_}{4.74}$.

Check the stability with respect to sliding.

Find the coefficient of passive earth pressure $\left(K_p\right)$ using the equation:

$K_p={\tan }^2\left(45+\frac{{{\varphi }^{\prime }}_2}{2}\right)$

Substitute $24°$ for ${{\varphi }^{\prime }}_2$.

$\begin{array}{c}{K}_p={\tan }^2\left(45+\frac{24°}{2}\right)\\ ={\tan }^2\left(57°\right)\\ =2.37\end{array}$

Find the passive earth pressure $\left(P_p\right)$ using the equation:

$P_p=\frac{1}{2}{\gamma }_2{D}^2{K}_p$

Here, ${\gamma }_2$ is the unit weigh of soil in front of the heel and under the base.

Substitute 1 m for D, $19.0{\text{kN/m}}^{\text{3}}$ for ${\gamma }_2$, and 2.37 for $K_p$.

$\begin{array}{c}{P}_p=\frac{1}{2}\times 19.0\times {\left(1\right)}^2\times 2.37\\ =22.51\text{kN}/\text{m}\end{array}$

Find the angle of friction $\left({\delta }^{\prime }\right)$ between the soil and the base slab using the equation:

${\delta }^{\prime }=\frac{2}{3}{{\varphi }^{\prime }}_2$

Substitute $24°$ for ${{\varphi }^{\prime }}_2$.

$\begin{array}{c}{\delta }^{\prime }=\frac{2}{3}\times 24°\\ =16°\end{array}$

Find the factor of safety against sliding $\left[F{S}_{\left(\text{sliding}\right)}\right]$ using the equation:

$F{S}_{\left(\text{sliding}\right)}=\frac{\left({\displaystyle \sum V}\right)\tan {\delta }^{\prime }+P_p}{P_h}$

Substitute $475.24\text{kN}/\text{m}$ for ${\displaystyle \sum V}$, $16°$ for ${\delta }^{\prime }$, $22.51\text{kN}/\text{m}$ for $P_p$, and $107.11\text{kN}/\text{m}$ for $P_h$.

$\begin{array}{c}F{S}_{\left(\text{sliding}\right)}=\frac{475.24\times \tan \left(16°\right)+22.51}{107.11}\\ =1.48\end{array}$

Therefore, the factor of safety with respect to sliding is $\underset{\_}{1.48}$.

Check the stability with bearing capacity failure.

Find the eccentricity (e) using the equation:

$e=\frac{B}{2}-\frac{{\displaystyle \sum M_R}-{\displaystyle \sum M_O}}{{\displaystyle \sum V}}$

Substitute 4 m for B, $1,074.8\text{kN-m}/\text{m}$ for ${\displaystyle \sum M_R}$, $226.82\text{kN-m}/\text{m}$ for ${\displaystyle \sum M_O}$, and $475.24\text{kN}/\text{m}$ for ${\displaystyle \sum V}$.

$\begin{array}{c}e=\frac{4}{2}-\frac{1,074.8-226.82}{475.24}\\ =2-1.78\\ =0.22\text{m}\end{array}$

Check for eccentricity.

$e<\frac{B}{6}$

Substitute 0.22 m for e and 4 m for B.

$\begin{array}{c}0.22\text{m}<\frac{4}{6}\\ 0.22\text{m}<0.67\text{m}\end{array}$

The eccentricity is within the limit. Therefore, there is no tensile stress produced at the end of the steel section.

Find the maximum pressure $\left(q_{\text{toe}}\right)$ occurring at the end of the toe base using the equation:

$\begin{array}{c}{q}_{\text{max}}=q_{\text{toe}}\\ q_{\text{toe}}=\frac{{\displaystyle \sum V}}{B}\left(1+\frac{6e}{B}\right)\end{array}$

Substitute $475.24\text{kN}/\text{m}$ for ${\displaystyle \sum V}$, 4 m for B, and 0.22 m for e.

$\begin{array}{c}{q}_{\text{toe}}=\frac{475.24}{4}\times \left(1+\frac{6\times 0.22}{4}\right)\\ =158\text{kN}/{\text{m}}^{\text{2}}\end{array}$

Find the effective breadth $\left(B^{\prime }\right)$ using the equation:

$B^{\prime }=B-2e$

Substitute 4 m for B and 0.22 m for e.

$\begin{array}{c}{B}^{\prime }=4-\left(2\times 0.22\right)\\ =3.56\text{m}\end{array}$

Refer Table 16.2, “Bearing Capacity Factors” in the textbook.

Take the value of bearing capacity factor, $N_q$ with corresponding angle of friction $\left({\varphi }^{\prime }\right)$ of $24°$ is 9.60.

Take the value of bearing capacity factor, $N_c$ with corresponding angle of friction $\left({\varphi }^{\prime }\right)$ of $24°$ is 19.32.

Take the value of bearing capacity factor, $N_{\gamma }$ with corresponding angle of friction $\left({\varphi }^{\prime }\right)$ of $24°$ is 9.44.

Find the depth factor $F_{cd}$ using the equation:

$F_{cd}=1+0.4\left(\frac{D}{B}\right)$

Substitute 1 m for D and 4 m for B.

$\begin{array}{c}{F}_{cd}=1+0.4\times \left(\frac{\text{1}\text{.0}\text{m}}{\text{4}\text{m}}\right)\\ =1.10\end{array}$

Find the load (q) due the soil in front of heel using the equation:

$q={\gamma }_{2}D$

Substitute $19{\text{kN/m}}^{\text{3}}$ for ${\gamma }_2$ and 1.0 m for D.

$\begin{array}{c}q=19\times 1.0\\ =19\text{kN}/{\text{m}}^{\text{2}}\end{array}$

Find the inclination angle of vertical load $\left(\psi \right)$ using the equation:

$\begin{array}{c}\psi ={\tan }^{-1}\left(\frac{P_a\cos \alpha }{{\displaystyle \sum V}}\right)\\ ={\tan }^{-1}\left(\frac{P_h}{{\displaystyle \sum V}}\right)\end{array}$

Substitute $107.11\text{kN}/\text{m}$ for $P_a$ and $475.24\text{kN}/\text{m}$ for ${\displaystyle \sum V}$.

$\begin{array}{c}\psi ={\tan }^{-1}\left(\frac{107.11}{475.24}\right)\\ ={\tan }^{-1}\left(0.238\right)\\ =12.7°\end{array}$

Find the inclination factor $F_{qi}$ and $F_{ci}$ using the equation:

$\begin{array}{c}{F}_{qi}=F_{ci}\\ ={\left(1-\frac{\psi }{90}\right)}^2\end{array}$

Substitute $12.7°$ for $\psi °$.

$\begin{array}{c}{F}_{qi}={\left(1-\frac{12.7°}{90}\right)}^2\\ =0.74\end{array}$

Find the depth factor $\left(F_{qd}\right)$ using the equation:

$F_{qd}=1+2\tan {{\varphi }^{\prime }}_2{\left(1-\sin {{\varphi }^{\prime }}_2\right)}^2\frac{D_f}{B}$

Here, $D_f$ is depth of flange.

Substitute $24°$ for ${{\varphi }^{\prime }}_2$, 1.0 m for $D_f$, and 4 m for B.

$\begin{array}{c}{F}_{qd}=1+2\tan \left(24°\right)\times {\left(1-\sin 24°\right)}^2\times \frac{1.0}{4}\\ =1+\left(0.890\times 0.352\times 0.25\right)\\ =1.08\end{array}$

The depth factor $F_{\gamma d}$ is 1.0 for ${\varphi }^{\prime }>0$.

Find the inclination factor $F_{\gamma i}$ using the equation:

$F_{\gamma i}={\left(1-\frac{\psi }{{{\varphi }^{\prime }}_2}\right)}^2$

Substitute $24°$ for ${{\varphi }^{\prime }}_2$ and $12.7°$ for $\psi$.

$\begin{array}{c}{F}_{\gamma i}={\left(1-\frac{12.7°}{24°}\right)}^2\\ =0.22\end{array}$

Find the ultimate bearing capacity of the shallow foundation $\left(q_u\right)$ using the equation:

$q_u=c^{\prime }{N}_c{F}_{cd}{F}_{ci}+q{N}_q{F}_{qd}{F}_{qi}+\frac{1}{2}{\gamma }_2{B}^{\prime }{N}_{\gamma }{F}_{\gamma d}{F}_{\gamma i}$

Substitute $10{\text{kN/m}}^{\text{2}}$ for $c^{\prime }$, 19.32 for $N_c$, 1.1 for $F_{cd}$, 0.74 for $F_{ci}$, $19{\text{kN/m}}^{\text{2}}$ for q, 9.60 for $N_q$, 1.08 for $F_{qd}$, 0.74 for $F_{qi}$, $19{\text{kN/m}}^{\text{3}}$ for ${\gamma }_2$, 3.56 m for $B^{\prime }$, 9.44 for $N_{\gamma }$, 1 for $F_{\gamma d}$, and 0.22 for $F_{\gamma i}$.

$\begin{array}{c}{q}_u=\left[\begin{array}{l}\left(10\times 19.32\times 1.1\times 0.74\right)+\left(19\times 9.60\times 1.08\times 0.74\right)\\ +\left(\frac{1}{2}\times 19\times 3.56\times 9.44\times 1\times 0.22\right)\end{array}\right]\\ =157.26+145.77+70.23\\ =373.26\text{kN}/{\text{m}}^{\text{2}}\end{array}$

Find the factor of safety against bearing capacity failure $\left[F{S}_{\left(\text{bearing}\right)}\right]$ using the equation:

$F{S}_{\left(\text{bearing}\right)}=\frac{q_u}{q_{\text{max}}}$

Substitute $373.26{\text{kN/m}}^{\text{2}}$ for $q_u$ and $158{\text{kN/m}}^{\text{2}}$ for $q_{\text{toe}}$.

$\begin{array}{c}F{S}_{\left(\text{bearing}\right)}=\frac{373.26}{158}\\ =2.36\end{array}$

Therefore, the factor of safety with respect to bearing capacity failure is $\underset{\_}{2.36}$.