Chapter 1.5, Problem 1.28E

Preschool The ages (in months) at which 50 children were first enrolled in a preschool are listed below.

38   40   30   35   39   40   48   36   31   36

47   35   34   43   41   36   41   43   48   40

32   34   41   30   46   35   40   30   46   37

55   39   33   32   32   45   42   41   36   50

42   50   37   39   33   45   38   46   36   31

a. Construct a stem and leaf display for the data.

b. Construct a relative frequency histogram for these data. Start the lower boundary of the first class at 30 and use a class width of 5 months.

c. Compare the graphs in parts a and b. Are there any significant differences that would cause you to choose one as the better method for displaying the data?

d. What proportion of the children were 35 months (2 years. 11 months) or older, but less than 45 months (3 years. 9 months) of age when first enrolled in preschool?

e. If one child were selected at random from this group of children, what is the probability that the child was less than 50 months old (4 years. 2 months) when first enrolled in preschool?

To determine

To construct a stem and leaf display for the data given in the question.

Answer to Problem 1.28E

The stem and leaf display is below as:-

Stem	Leaf
3	0 0 0 1 1 2 2 2 3 3 4 4 5 5 5 6 6 6 6 6 7 7 8 8 9 9 9
4	0 0 0 0 1 1 1 1 2 2 3 3 5 5 6 6 6 7 8
5	0 0 5
6
7	8

Explanation of Solution

The set of data is given in the question.

Each observation in the data is dividedbetween the ones and the tens place. The number to the left is the stem and the number to the right is the leaf.

To determine

To construct a relative frequency histogram for the data given in the question.

Answer to Problem 1.28E

The relative frequency histogram is as follows:-

  

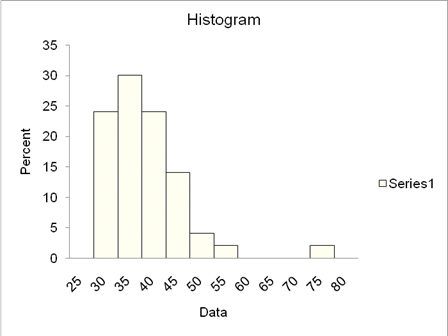
Explanation of Solution

The observations are given in the question.

By considering the observations, Relative frequency distribution is constructed with the lower boundary of the first class at 30 and the class width of 5 months.

To determine

To compare the graphs in (a) and (b) and also to find if there is any significant differences that would cause us to choose one as the better method for displaying the data.

Answer to Problem 1.28E

The graphs in (a) and (b) are both skewed to the right but in (a) the data is displayed in horizontal view and in (b) the data is displayed in the vertical view.

Yes, there is significant difference in the view and also the vertical view is more convenient way to display and understand the data i.e. in (b) than in (a).

Explanation of Solution

The graph in (a) is stem and leaf in which each observation in the data is divided between the ones and the tens place. The number to the left is the stem and the number to the right is the leaf. This is displayed in horizontal view.

And the graph in (b) is arelative frequency histogramfor a quantitative data set is a bar graph in which the height of the bar shows “how often” (measured as a proportion or relative frequency) measurements fall in a particular class or subinterval. This is displayed in vertical view.

Both the graphs are skewed to the right by looking at the graph in both the parts.

And also the data can be better understandable and easy to view in vertical form. Thus, the graph in part (b) is better method for displaying the data.

To determine

To calculate the proportion of the children who are $35$ months or older, but less than $45$ months of age when first enrolled in preschool.

Answer to Problem 1.28E

The proportion of the children who are $35$ months or older, but less than $45$ months of age when first enrolled in preschool is $27$ .

Explanation of Solution

By looking at the relative frequency data from the histogram, we get that:-

	Data						cumulative
lower		upper	midpoint	width	frequency	percent	frequency	percent
25	<	30	28	5	0	0.0	0	0.0
30	<	35	33	5	12	24.0	12	24.0
35	<	40	38	5	15	30.0	27	54.0
40	<	45	43	5	12	24.0	39	78.0
45	<	50	48	5	7	14.0	46	92.0
50	<	55	53	5	2	4.0	48	96.0
55	<	60	58	5	1	2.0	49	98.0
60	<	65	63	5	0	0.0	49	98.0
65	<	70	68	5	0	0.0	49	98.0
70	<	75	73	5	0	0.0	49	98.0
75	<	80	77	5	1	2.0	50	100.0
					50	100.0

That there are $15$ frequencies for the interval of $35\le x<40$ and there are $12$ frequencies for the interval $40\le x<45$ .

Thus, we have,

  $15+12=27$

To determine

To calculate the probability that the child was less than $50$ months old when first enrolled in preschool, if one child is selected at random from this group of children.

Answer to Problem 1.28E

  $0.92$

Explanation of Solution

To find the probability that the child was less than $50$ months old when first enrolled in preschool, if one child is selected at random from this group of children, we have to;

First calculate the number of children who are less than $50$ months old, i.e.,

The number of children who are less than $50$ months oldis $46$ .

Secondly we have to calculate the total number of children in the group that are $50$ .

Thus, the probability that the child was less than $50$ months old when first enrolled in preschool, if one child is selected at random from this group of children is equal to the number of children who are less than $50$ months old divide by the total number of childrenin the group.

Therefore,

  $\frac{46}{50}=0.92$