Algebra 1, Homework Practice Workbook (MERRILL ALGEBRA 1)
2nd Edition
ISBN: 9780076602919
Author: McGraw-Hill Education
Publisher: McGraw-Hill Education
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Question
Chapter 1.5, Problem 10PPS
(a)
To determine
To describe: The accuracy of the line.
(a)
Expert Solution
Explanation of Solution
Given:
The graph of the mass of marbles is shown in Figure 1.
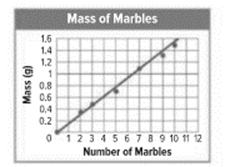
Figure 1
Each point on the graph shows the relation between the number of marble and its mass where the number of marbles is the independent variable and the mass is dependent variable.
The accuracy of the line depends on the closeness of the points to the line.
It can be seen from the given graph that some points are above the line and some are below the line and some are passing through.
The points are close to the line. Therefore, there is high
The points are closeto the line but all points donot pass through the line. In conclusion accuracy is quite good but not perfectly correct.
(b)
To determine
To find: The approximate mass of 7 marbles.
(b)
Expert Solution
Answer to Problem 10PPS
The approximate mass of 7 marbles is 1.1g.
Explanation of Solution
Given:
The graph of the mass of marbles is shown in Figure 1.
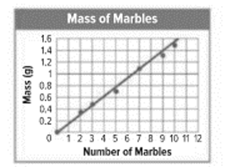
Figure 1
The number of marbles is shown on the horizontal axis that is independent variable and the mass is shown on the vertical axis that is dependent variable.
See the point for 7 marbles on the graph.
It can be observed from Figure 1 that the approximate mass of 7 marbles is 1.1g.
Therefore, the approximate mass of 7 marbles is 1.1g.
(c)
To determine
To explain: The reason of few points is above the line and some are below the line.
(c)
Expert Solution
Explanation of Solution
Given:
The graph of the mass of marbles is shown in Figure 1.
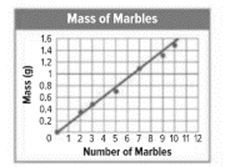
Figure 1
The set of points are the
The line of best fit is the line passes through the points or closely to the point. If half of the data points on the scatter point is below the line and half above the line.
It can be seen from Figure 1 that the data points come close to the line of the best fit that means the
The positive correlation is high since it predicts the values near the line some points are above the line and some are below the line.
(d)
To determine
To make: A conjecture for the approximate mass of 20 marbles and explain the reasoning.
(d)
Expert Solution
Explanation of Solution
Given:
The graph of the mass of marbles is shown in Figure 1.
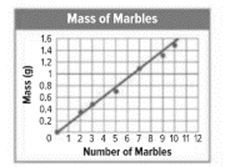
Figure 1
It can be observed from the Figure 1 that the possible set of points are represented in the below table.
| Number of marbles | Mass |
| 0 | 0 |
| 2 | 0.38 |
| 3 | 0.5 |
| 5 | 0.7 |
| 7 | 1.1 |
| 9 | 1.3 |
| 10 | 1.48 |
It can be seen that that the line of the regression is the form of
The point is 1.48 at the time of 10 marbles.
Therefore, there is possibility of the slope is 1.433 and the y- intercept is 0.05 something.
Substitute 1.433 for
Take these points for the line to make a conjecture for the 20 marbles.
Substitute 20 for
Therefore, it would be 2.433 something.
Chapter 1 Solutions
Algebra 1, Homework Practice Workbook (MERRILL ALGEBRA 1)
Ch. 1.1 - Prob. 1AGPCh. 1.1 - Prob. 1BGPCh. 1.1 - Prob. 2AGPCh. 1.1 - Prob. 2BGPCh. 1.1 - Prob. 3GPCh. 1.1 - Prob. 1CYUCh. 1.1 - Prob. 2CYUCh. 1.1 - Prob. 3CYUCh. 1.1 - Prob. 4CYUCh. 1.1 - Prob. 5CYU
Ch. 1.1 - Prob. 6CYUCh. 1.1 - Prob. 7CYUCh. 1.1 - Prob. 8CYUCh. 1.1 - Prob. 9CYUCh. 1.1 - Prob. 10CYUCh. 1.1 - Prob. 11PPSCh. 1.1 - Prob. 12PPSCh. 1.1 - Prob. 13PPSCh. 1.1 - Prob. 14PPSCh. 1.1 - Prob. 15PPSCh. 1.1 - Prob. 16PPSCh. 1.1 - Prob. 17PPSCh. 1.1 - Prob. 18PPSCh. 1.1 - Prob. 19PPSCh. 1.1 - Prob. 20PPSCh. 1.1 - Prob. 21PPSCh. 1.1 - Prob. 22PPSCh. 1.1 - Prob. 23PPSCh. 1.1 - Prob. 24PPSCh. 1.1 - Prob. 25PPSCh. 1.1 - Prob. 26PPSCh. 1.1 - Prob. 27PPSCh. 1.1 - Prob. 28PPSCh. 1.1 - Prob. 29PPSCh. 1.1 - Prob. 30PPSCh. 1.1 - Prob. 31PPSCh. 1.1 - Prob. 32PPSCh. 1.1 - Prob. 33PPSCh. 1.1 - Prob. 34PPSCh. 1.1 - Prob. 35PPSCh. 1.1 - Prob. 36PPSCh. 1.1 - Prob. 37PPSCh. 1.1 - Prob. 38HPCh. 1.1 - Prob. 39HPCh. 1.1 - Prob. 40HPCh. 1.1 - Prob. 41HPCh. 1.1 - Prob. 42HPCh. 1.1 - Prob. 43PFACh. 1.1 - Prob. 44PFACh. 1.1 - Prob. 45PFACh. 1.1 - Prob. 46PFACh. 1.1 - Prob. 47PFACh. 1.1 - Prob. 48PFACh. 1.1 - Prob. 49PFACh. 1.2 - Prob. 1AGPCh. 1.2 - Prob. 1BGPCh. 1.2 - Prob. 1CGPCh. 1.2 - Prob. 2AGPCh. 1.2 - Prob. 2BGPCh. 1.2 - Prob. 3AGPCh. 1.2 - Prob. 3BGPCh. 1.2 - Prob. 3CGPCh. 1.2 - Prob. 4AGPCh. 1.2 - Prob. 4BGPCh. 1.2 - Prob. 5AGPCh. 1.2 - Prob. 5BGPCh. 1.2 - Prob. 1CYUCh. 1.2 - Prob. 2CYUCh. 1.2 - Prob. 3CYUCh. 1.2 - Prob. 4CYUCh. 1.2 - Prob. 5CYUCh. 1.2 - Prob. 6CYUCh. 1.2 - Prob. 7CYUCh. 1.2 - Prob. 8CYUCh. 1.2 - Prob. 9CYUCh. 1.2 - Prob. 10CYUCh. 1.2 - Prob. 11CYUCh. 1.2 - Prob. 12CYUCh. 1.2 - Prob. 13CYUCh. 1.2 - Prob. 14CYUCh. 1.2 - Prob. 15PPSCh. 1.2 - Prob. 16PPSCh. 1.2 - Prob. 17PPSCh. 1.2 - Prob. 18PPSCh. 1.2 - Prob. 19PPSCh. 1.2 - Prob. 20PPSCh. 1.2 - Prob. 21PPSCh. 1.2 - Prob. 22PPSCh. 1.2 - Prob. 23PPSCh. 1.2 - Prob. 24PPSCh. 1.2 - Prob. 25PPSCh. 1.2 - Prob. 26PPSCh. 1.2 - Prob. 27PPSCh. 1.2 - Prob. 28PPSCh. 1.2 - Prob. 29PPSCh. 1.2 - Prob. 30PPSCh. 1.2 - Prob. 31PPSCh. 1.2 - Prob. 32PPSCh. 1.2 - Prob. 33PPSCh. 1.2 - Prob. 34PPSCh. 1.2 - Prob. 35PPSCh. 1.2 - Prob. 36PPSCh. 1.2 - Prob. 37PPSCh. 1.2 - Prob. 38PPSCh. 1.2 - Prob. 39PPSCh. 1.2 - Prob. 40PPSCh. 1.2 - Prob. 41PPSCh. 1.2 - Prob. 42PPSCh. 1.2 - Prob. 43PPSCh. 1.2 - Prob. 44PPSCh. 1.2 - Prob. 45PPSCh. 1.2 - Prob. 46PPSCh. 1.2 - Prob. 47PPSCh. 1.2 - Prob. 48PPSCh. 1.2 - Prob. 49PPSCh. 1.2 - Prob. 50PPSCh. 1.2 - Prob. 51PPSCh. 1.2 - Prob. 52PPSCh. 1.2 - Prob. 53PPSCh. 1.2 - Prob. 54PPSCh. 1.2 - Prob. 55PPSCh. 1.2 - Prob. 56PPSCh. 1.2 - Prob. 57PPSCh. 1.2 - Prob. 58PPSCh. 1.2 - Prob. 59HPCh. 1.2 - Prob. 60HPCh. 1.2 - Prob. 61HPCh. 1.2 - Prob. 62HPCh. 1.2 - Prob. 63HPCh. 1.2 - Prob. 64HPCh. 1.2 - Prob. 65PFACh. 1.2 - Prob. 66PFACh. 1.2 - Prob. 67PFACh. 1.2 - Prob. 68PFACh. 1.2 - Prob. 69PFACh. 1.2 - Prob. 70PFACh. 1.2 - Prob. 71PFACh. 1.3 - Prob. 1AGPCh. 1.3 - Prob. 1BGPCh. 1.3 - Prob. 2GPCh. 1.3 - Prob. 3AGPCh. 1.3 - Prob. 3BGPCh. 1.3 - Prob. 1CYUCh. 1.3 - Prob. 2CYUCh. 1.3 - Prob. 3CYUCh. 1.3 - Prob. 4CYUCh. 1.3 - Prob. 5CYUCh. 1.3 - Prob. 6CYUCh. 1.3 - Prob. 7CYUCh. 1.3 - Prob. 8CYUCh. 1.3 - Prob. 9PPSCh. 1.3 - Prob. 10PPSCh. 1.3 - Prob. 11PPSCh. 1.3 - Prob. 12PPSCh. 1.3 - Prob. 13PPSCh. 1.3 - Prob. 14PPSCh. 1.3 - Prob. 15PPSCh. 1.3 - Prob. 16PPSCh. 1.3 - Prob. 17PPSCh. 1.3 - Prob. 18PPSCh. 1.3 - Prob. 19PPSCh. 1.3 - Prob. 20PPSCh. 1.3 - Prob. 21PPSCh. 1.3 - Prob. 22PPSCh. 1.3 - Prob. 23PPSCh. 1.3 - Prob. 24PPSCh. 1.3 - Prob. 25PPSCh. 1.3 - Prob. 26PPSCh. 1.3 - Prob. 27PPSCh. 1.3 - Prob. 28PPSCh. 1.3 - Prob. 29PPSCh. 1.3 - Prob. 30PPSCh. 1.3 - Prob. 31PPSCh. 1.3 - Prob. 32PPSCh. 1.3 - Prob. 33PPSCh. 1.3 - Prob. 34PPSCh. 1.3 - Prob. 35PPSCh. 1.3 - Prob. 36PPSCh. 1.3 - Prob. 37PPSCh. 1.3 - Prob. 38PPSCh. 1.3 - Prob. 39PPSCh. 1.3 - Prob. 40PPSCh. 1.3 - Prob. 41PPSCh. 1.3 - Prob. 42PPSCh. 1.3 - Prob. 43PPSCh. 1.3 - Prob. 44PPSCh. 1.3 - Prob. 45PPSCh. 1.3 - Prob. 46PPSCh. 1.3 - Prob. 47PPSCh. 1.3 - Prob. 48PPSCh. 1.3 - Prob. 49PPSCh. 1.3 - Prob. 50PPSCh. 1.3 - Prob. 51PPSCh. 1.3 - Prob. 52PPSCh. 1.3 - Prob. 53PPSCh. 1.3 - Prob. 54HPCh. 1.3 - Prob. 55HPCh. 1.3 - Prob. 56HPCh. 1.3 - Prob. 57HPCh. 1.3 - Prob. 58HPCh. 1.3 - Prob. 59HPCh. 1.3 - Prob. 60HPCh. 1.3 - Prob. 61PFACh. 1.3 - Prob. 62PFACh. 1.3 - Prob. 63PFACh. 1.3 - Prob. 64PFACh. 1.3 - Prob. 65PFACh. 1.3 - Prob. 66PFACh. 1.3 - Prob. 67PFACh. 1.4 - Prob. 1GPCh. 1.4 - Prob. 2AGPCh. 1.4 - Prob. 2BGPCh. 1.4 - Prob. 2CGPCh. 1.4 - Prob. 2DGPCh. 1.4 - Prob. 3AGPCh. 1.4 - Prob. 3BGPCh. 1.4 - Prob. 3CGPCh. 1.4 - Prob. 3DGPCh. 1.4 - Prob. 4AGPCh. 1.4 - Prob. 4BGPCh. 1.4 - Prob. 4CGPCh. 1.4 - Prob. 4DGPCh. 1.4 - Prob. 5AGPCh. 1.4 - Prob. 5BGPCh. 1.4 - Prob. 1CYUCh. 1.4 - Prob. 2CYUCh. 1.4 - Prob. 3CYUCh. 1.4 - Prob. 4CYUCh. 1.4 - Prob. 5CYUCh. 1.4 - Prob. 6CYUCh. 1.4 - Prob. 7CYUCh. 1.4 - Prob. 8CYUCh. 1.4 - Prob. 9CYUCh. 1.4 - Prob. 10CYUCh. 1.4 - Prob. 11PPSCh. 1.4 - Prob. 12PPSCh. 1.4 - Prob. 13PPSCh. 1.4 - Prob. 14PPSCh. 1.4 - Prob. 15PPSCh. 1.4 - Prob. 16PPSCh. 1.4 - Prob. 17PPSCh. 1.4 - Prob. 18PPSCh. 1.4 - Prob. 19PPSCh. 1.4 - Prob. 20PPSCh. 1.4 - Prob. 21PPSCh. 1.4 - Prob. 22PPSCh. 1.4 - Prob. 23PPSCh. 1.4 - Prob. 24PPSCh. 1.4 - Prob. 25PPSCh. 1.4 - Prob. 26PPSCh. 1.4 - Prob. 27PPSCh. 1.4 - Prob. 28PPSCh. 1.4 - Prob. 29PPSCh. 1.4 - Prob. 30PPSCh. 1.4 - Prob. 31PPSCh. 1.4 - Prob. 32PPSCh. 1.4 - Prob. 33PPSCh. 1.4 - Prob. 34PPSCh. 1.4 - Prob. 35PPSCh. 1.4 - Prob. 36PPSCh. 1.4 - Prob. 37PPSCh. 1.4 - Prob. 38PPSCh. 1.4 - Prob. 39PPSCh. 1.4 - Prob. 40PPSCh. 1.4 - Prob. 41PPSCh. 1.4 - Prob. 42PPSCh. 1.4 - Prob. 43PPSCh. 1.4 - Prob. 44PPSCh. 1.4 - Prob. 45PPSCh. 1.4 - Prob. 46PPSCh. 1.4 - Prob. 47PPSCh. 1.4 - Prob. 48PPSCh. 1.4 - Prob. 49PPSCh. 1.4 - Prob. 50PPSCh. 1.4 - Prob. 51PPSCh. 1.4 - Prob. 52PPSCh. 1.4 - Prob. 53PPSCh. 1.4 - Prob. 54PPSCh. 1.4 - Prob. 55PPSCh. 1.4 - Prob. 56HPCh. 1.4 - Prob. 57HPCh. 1.4 - Prob. 58HPCh. 1.4 - Prob. 59HPCh. 1.4 - Prob. 60PFACh. 1.4 - Prob. 61PFACh. 1.4 - Prob. 62PFACh. 1.4 - Prob. 63PFACh. 1.4 - Prob. 64PFACh. 1.4 - Prob. 65PFACh. 1.4 - Prob. 66PFACh. 1.4 - Prob. 67PFACh. 1.5 - Prob. 1AGPCh. 1.5 - Prob. 1BGPCh. 1.5 - Prob. 1CGPCh. 1.5 - Prob. 2AGPCh. 1.5 - Prob. 2BGPCh. 1.5 - Prob. 3AGPCh. 1.5 - Prob. 3BGPCh. 1.5 - Prob. 3CGPCh. 1.5 - Prob. 4AGPCh. 1.5 - Prob. 4BGPCh. 1.5 - Prob. 4CGPCh. 1.5 - Prob. 1CYUCh. 1.5 - Prob. 2CYUCh. 1.5 - Prob. 3CYUCh. 1.5 - Prob. 4CYUCh. 1.5 - Prob. 5PPSCh. 1.5 - Prob. 6PPSCh. 1.5 - Prob. 7PPSCh. 1.5 - Prob. 8PPSCh. 1.5 - Prob. 9PPSCh. 1.5 - Prob. 10PPSCh. 1.5 - Prob. 11PPSCh. 1.5 - Prob. 12PPSCh. 1.5 - Prob. 13PPSCh. 1.5 - Prob. 14PPSCh. 1.5 - Prob. 15PPSCh. 1.5 - Prob. 16PPSCh. 1.5 - Prob. 17PPSCh. 1.5 - Prob. 18PPSCh. 1.5 - Prob. 19PPSCh. 1.5 - Prob. 20PPSCh. 1.5 - Prob. 21PPSCh. 1.5 - Prob. 22PPSCh. 1.5 - Prob. 23PPSCh. 1.5 - Prob. 24PPSCh. 1.5 - Prob. 25PPSCh. 1.5 - Prob. 26PPSCh. 1.5 - Prob. 27HPCh. 1.5 - Prob. 28HPCh. 1.5 - Prob. 29PFACh. 1.5 - Prob. 30PFACh. 1.5 - Prob. 31PFACh. 1.5 - Prob. 32PFACh. 1.5 - Prob. 33PFACh. 1.5 - Prob. 34PFACh. 1.5 - Prob. 35PFACh. 1.6 - Prob. 1AGPCh. 1.6 - Prob. 1BGPCh. 1.6 - Prob. 2AGPCh. 1.6 - Prob. 2BGPCh. 1.6 - Prob. 3AGPCh. 1.6 - Prob. 3BGPCh. 1.6 - Prob. 1CYUCh. 1.6 - Prob. 2CYUCh. 1.6 - Prob. 3CYUCh. 1.6 - Prob. 4CYUCh. 1.6 - Prob. 5CYUCh. 1.6 - Prob. 6CYUCh. 1.6 - Prob. 7CYUCh. 1.6 - Prob. 8CYUCh. 1.6 - Prob. 9PPSCh. 1.6 - Prob. 10PPSCh. 1.6 - Prob. 11PPSCh. 1.6 - Prob. 12PPSCh. 1.6 - Prob. 13PPSCh. 1.6 - Prob. 14PPSCh. 1.6 - Prob. 15PPSCh. 1.6 - Prob. 16PPSCh. 1.6 - Prob. 17PPSCh. 1.6 - Prob. 18PPSCh. 1.6 - Prob. 19PPSCh. 1.6 - Prob. 20PPSCh. 1.6 - Prob. 21PPSCh. 1.6 - Prob. 22PPSCh. 1.6 - Prob. 23PPSCh. 1.6 - Prob. 24PPSCh. 1.6 - Prob. 25PPSCh. 1.6 - Prob. 26PPSCh. 1.6 - Prob. 27PPSCh. 1.6 - Prob. 28PPSCh. 1.6 - Prob. 29PPSCh. 1.6 - Prob. 30PPSCh. 1.6 - Prob. 31PPSCh. 1.6 - Prob. 32PPSCh. 1.6 - Prob. 33PPSCh. 1.6 - Prob. 34PPSCh. 1.6 - Prob. 35PPSCh. 1.6 - Prob. 36PPSCh. 1.6 - Prob. 37PPSCh. 1.6 - Prob. 38HPCh. 1.6 - Prob. 39HPCh. 1.6 - Prob. 40HPCh. 1.6 - Prob. 41HPCh. 1.6 - Prob. 42HPCh. 1.6 - Prob. 43PFACh. 1.6 - Prob. 44PFACh. 1.6 - Prob. 45PFACh. 1.6 - Prob. 46PFACh. 1.6 - Prob. 47PFACh. 1.7 - Prob. 1GPCh. 1.7 - Prob. 2AGPCh. 1.7 - Prob. 2BGPCh. 1.7 - Prob. 2CGPCh. 1.7 - Prob. 2DGPCh. 1.7 - Prob. 3AGPCh. 1.7 - Prob. 3BGPCh. 1.7 - Prob. 4AGPCh. 1.7 - Prob. 4BGPCh. 1.7 - Prob. 4CGPCh. 1.7 - Prob. 4DGPCh. 1.7 - Prob. 5AGPCh. 1.7 - Prob. 5BGPCh. 1.7 - Prob. 5CGPCh. 1.7 - Prob. 5DGPCh. 1.7 - Prob. 1CYUCh. 1.7 - Prob. 2CYUCh. 1.7 - Prob. 3CYUCh. 1.7 - Prob. 4CYUCh. 1.7 - Prob. 5CYUCh. 1.7 - Prob. 6CYUCh. 1.7 - Prob. 7CYUCh. 1.7 - Prob. 8CYUCh. 1.7 - Prob. 9CYUCh. 1.7 - Prob. 10CYUCh. 1.7 - Prob. 11CYUCh. 1.7 - Prob. 12CYUCh. 1.7 - Prob. 13CYUCh. 1.7 - Prob. 14CYUCh. 1.7 - Prob. 15CYUCh. 1.7 - Prob. 16CYUCh. 1.7 - Prob. 17CYUCh. 1.7 - Prob. 18CYUCh. 1.7 - Prob. 19CYUCh. 1.7 - Prob. 20PPSCh. 1.7 - Prob. 21PPSCh. 1.7 - Prob. 22PPSCh. 1.7 - Prob. 23PPSCh. 1.7 - Prob. 24PPSCh. 1.7 - Prob. 25PPSCh. 1.7 - Prob. 26PPSCh. 1.7 - Prob. 27PPSCh. 1.7 - Prob. 28PPSCh. 1.7 - Prob. 29PPSCh. 1.7 - Prob. 30PPSCh. 1.7 - Prob. 31PPSCh. 1.7 - Prob. 32PPSCh. 1.7 - Prob. 33PPSCh. 1.7 - Prob. 34PPSCh. 1.7 - Prob. 35PPSCh. 1.7 - Prob. 36PPSCh. 1.7 - Prob. 37PPSCh. 1.7 - Prob. 38PPSCh. 1.7 - Prob. 39PPSCh. 1.7 - Prob. 40PPSCh. 1.7 - Prob. 41PPSCh. 1.7 - Prob. 42PPSCh. 1.7 - Prob. 43PPSCh. 1.7 - Prob. 44PPSCh. 1.7 - Prob. 45PPSCh. 1.7 - Prob. 46PPSCh. 1.7 - Prob. 47PPSCh. 1.7 - Prob. 48PPSCh. 1.7 - Prob. 49HPCh. 1.7 - Prob. 50HPCh. 1.7 - Prob. 51HPCh. 1.7 - Prob. 52HPCh. 1.7 - Prob. 53HPCh. 1.7 - Prob. 54HPCh. 1.7 - Prob. 55HPCh. 1.7 - Prob. 56PFACh. 1.7 - Prob. 57PFACh. 1.7 - Prob. 58PFACh. 1.7 - Prob. 59PFACh. 1.7 - Prob. 60PFACh. 1.7 - Prob. 61PFACh. 1.8 - Prob. 1GPCh. 1.8 - Prob. 2GPCh. 1.8 - Prob. 3GPCh. 1.8 - Prob. 4GPCh. 1.8 - Prob. 1CYUCh. 1.8 - Prob. 2CYUCh. 1.8 - Prob. 3CYUCh. 1.8 - Prob. 4CYUCh. 1.8 - Prob. 5PPSCh. 1.8 - Prob. 6PPSCh. 1.8 - Prob. 7PPSCh. 1.8 - Prob. 8PPSCh. 1.8 - Prob. 9PPSCh. 1.8 - Prob. 10PPSCh. 1.8 - Prob. 11PPSCh. 1.8 - Prob. 12PPSCh. 1.8 - Prob. 13PPSCh. 1.8 - Prob. 14PPSCh. 1.8 - Prob. 15PPSCh. 1.8 - Prob. 16PPSCh. 1.8 - Prob. 17PPSCh. 1.8 - Prob. 18PPSCh. 1.8 - Prob. 19PPSCh. 1.8 - Prob. 20HPCh. 1.8 - Prob. 21HPCh. 1.8 - Prob. 22HPCh. 1.8 - Prob. 23HPCh. 1.8 - Prob. 24HPCh. 1.8 - Prob. 25PFACh. 1.8 - Prob. 26PFACh. 1.8 - Prob. 27PFACh. 1.8 - Prob. 28PFACh. 1.8 - Prob. 29PFACh. 1.8 - Prob. 30PFACh. 1 - Prob. 1MCQCh. 1 - Prob. 2MCQCh. 1 - Prob. 3MCQCh. 1 - Prob. 4MCQCh. 1 - Prob. 5MCQCh. 1 - Prob. 6MCQCh. 1 - Prob. 7MCQCh. 1 - Prob. 8MCQCh. 1 - Prob. 9MCQCh. 1 - Prob. 10MCQCh. 1 - Prob. 11MCQCh. 1 - Prob. 12MCQCh. 1 - Prob. 13MCQCh. 1 - Prob. 14MCQCh. 1 - Prob. 15MCQCh. 1 - Prob. 16MCQCh. 1 - Prob. 17MCQCh. 1 - Prob. 18MCQCh. 1 - Prob. 19MCQCh. 1 - Prob. 20MCQCh. 1 - Prob. 21MCQCh. 1 - Prob. 22MCQCh. 1 - Prob. 23MCQCh. 1 - Prob. 24MCQCh. 1 - Prob. 25MCQCh. 1 - Prob. 1SGRCh. 1 - Prob. 2SGRCh. 1 - Prob. 3SGRCh. 1 - Prob. 4SGRCh. 1 - Prob. 5SGRCh. 1 - Prob. 6SGRCh. 1 - Prob. 7SGRCh. 1 - Prob. 8SGRCh. 1 - Prob. 9SGRCh. 1 - Prob. 10SGRCh. 1 - Prob. 11SGRCh. 1 - Prob. 12SGRCh. 1 - Prob. 13SGRCh. 1 - Prob. 14SGRCh. 1 - Prob. 15SGRCh. 1 - Prob. 16SGRCh. 1 - Prob. 17SGRCh. 1 - Prob. 18SGRCh. 1 - Prob. 19SGRCh. 1 - Prob. 20SGRCh. 1 - Prob. 21SGRCh. 1 - Prob. 22SGRCh. 1 - Prob. 23SGRCh. 1 - Prob. 24SGRCh. 1 - Prob. 25SGRCh. 1 - Prob. 26SGRCh. 1 - Prob. 27SGRCh. 1 - Prob. 28SGRCh. 1 - Prob. 29SGRCh. 1 - Prob. 30SGRCh. 1 - Prob. 31SGRCh. 1 - Prob. 32SGRCh. 1 - Prob. 33SGRCh. 1 - Prob. 34SGRCh. 1 - Prob. 35SGRCh. 1 - Prob. 36SGRCh. 1 - Prob. 37SGRCh. 1 - Prob. 38SGRCh. 1 - Prob. 39SGRCh. 1 - Prob. 40SGRCh. 1 - Prob. 41SGRCh. 1 - Prob. 42SGRCh. 1 - Prob. 43SGRCh. 1 - Prob. 44SGRCh. 1 - Prob. 45SGRCh. 1 - Prob. 46SGRCh. 1 - Prob. 47SGRCh. 1 - Prob. 48SGRCh. 1 - Prob. 49SGRCh. 1 - Prob. 50SGRCh. 1 - Prob. 51SGRCh. 1 - Prob. 52SGRCh. 1 - Prob. 53SGRCh. 1 - Prob. 54SGRCh. 1 - Prob. 55SGRCh. 1 - Prob. 56SGRCh. 1 - Prob. 57SGRCh. 1 - Prob. 58SGRCh. 1 - Prob. 59SGRCh. 1 - Prob. 60SGRCh. 1 - Prob. 61SGRCh. 1 - Prob. 62SGRCh. 1 - Prob. 63SGRCh. 1 - Prob. 64SGRCh. 1 - Prob. 65SGRCh. 1 - Prob. 66SGRCh. 1 - Prob. 67SGRCh. 1 - Prob. 68SGRCh. 1 - Prob. 69SGRCh. 1 - Prob. 70SGRCh. 1 - Prob. 71SGRCh. 1 - Prob. 72SGRCh. 1 - Prob. 73SGRCh. 1 - Prob. 74SGRCh. 1 - Prob. 75SGRCh. 1 - Prob. 1PTCh. 1 - Prob. 2PTCh. 1 - Prob. 3PTCh. 1 - Prob. 4PTCh. 1 - Prob. 5PTCh. 1 - Prob. 6PTCh. 1 - Prob. 7PTCh. 1 - Prob. 8PTCh. 1 - Prob. 9PTCh. 1 - Prob. 10PTCh. 1 - Prob. 11PTCh. 1 - Prob. 12PTCh. 1 - Prob. 13PTCh. 1 - Prob. 14PTCh. 1 - Prob. 15PTCh. 1 - Prob. 16PTCh. 1 - Prob. 17PTCh. 1 - Prob. 18PTCh. 1 - Prob. 19PTCh. 1 - Prob. 20PTCh. 1 - Prob. 21PTCh. 1 - Prob. 1CRCh. 1 - Prob. 2CRCh. 1 - Prob. 3CRCh. 1 - Prob. 4CRCh. 1 - Prob. 5CRCh. 1 - Prob. 6CRCh. 1 - Prob. 7CRCh. 1 - Prob. 8CRCh. 1 - Prob. 9CRCh. 1 - Prob. 10CRCh. 1 - Prob. 11CRCh. 1 - Prob. 12CRCh. 1 - Prob. 13CRCh. 1 - Prob. 14CRCh. 1 - Prob. 15CR
Additional Math Textbook Solutions
Find more solutions based on key concepts
Critical Thinking. For Exercises 5-20, watch out for these little buggers. Each of these exercises involves som...
Elementary Statistics (13th Edition)
Derivatives of bx Find the derivatives of the following functions. 23. y = 8x
Calculus: Early Transcendentals (2nd Edition)
Assessment 1-1A Cookies are sold singly or in packages of 2 or 6. With this packaging, how many ways can you bu...
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
Express the side length of a square as a function of the length d of the square’s diagonal. Then express the ar...
University Calculus: Early Transcendentals (4th Edition)
Compare and contrast scale and scale factor.
Pre-Algebra Student Edition
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, algebra and related others by exploring similar questions and additional content below.Similar questions
- Compare the interest earned from #1 (where simple interest was used) to #5 (where compound interest was used). The principal, annual interest rate, and time were all the same; the only difference was that for #5, interest was compounded quarterly. Does the difference in interest earned make sense? Select one of the following statements. a. No, because more money should have been earned through simple interest than compound interest. b. Yes, because more money was earned through simple interest. For simple interest you earn interest on interest, not just on the amount of principal. c. No, because more money was earned through simple interest. For simple interest you earn interest on interest, not just on the amount of principal. d. Yes, because more money was earned when compounded quarterly. For compound interest you earn interest on interest, not just on the amount of principal.arrow_forwardCompare and contrast the simple and compound interest formulas. Which one of the following statements is correct? a. Simple interest and compound interest formulas both yield principal plus interest, so you must subtract the principal to get the amount of interest. b. Simple interest formula yields principal plus interest, so you must subtract the principal to get the amount of interest; Compound interest formula yields only interest, which you must add to the principal to get the final amount. c. Simple interest formula yields only interest, which you must add to the principal to get the final amount; Compound interest formula yields principal plus interest, so you must subtract the principal to get the amount of interest. d. Simple interest and compound interest formulas both yield only interest, which you must add to the principal to get the final amount.arrow_forwardSara would like to go on a vacation in 5 years and she expects her total costs to be $3000. If she invests $2500 into a savings account for those 5 years at 8% interest, compounding semi-annually, how much money will she have? Round your answer to the nearest cent. Show you work. Will she be able to go on vacation? Why or why not?arrow_forward
- If $8000 is deposited into an account earning simple interest at an annual interest rate of 4% for 10 years, howmuch interest was earned? Show you work.arrow_forward10-2 Let A = 02-4 and b = 4 Denote the columns of A by a₁, a2, a3, and let W = Span {a1, a2, a̸3}. -4 6 5 - 35 a. Is b in {a1, a2, a3}? How many vectors are in {a₁, a₂, a3}? b. Is b in W? How many vectors are in W? c. Show that a2 is in W. [Hint: Row operations are unnecessary.] a. Is b in {a₁, a2, a3}? Select the correct choice below and, if necessary, fill in the answer box(es) to complete your choice. ○ A. No, b is not in {a₁, a2, 3} since it cannot be generated by a linear combination of a₁, a2, and a3. B. No, b is not in (a1, a2, a3} since b is not equal to a₁, a2, or a3. C. Yes, b is in (a1, a2, a3} since b = a (Type a whole number.) D. Yes, b is in (a1, a2, 3} since, although b is not equal to a₁, a2, or a3, it can be expressed as a linear combination of them. In particular, b = + + ☐ az. (Simplify your answers.)arrow_forward14 14 4. The graph shows the printing rate of Printer A. Printer B can print at a rate of 25 pages per minute. How does the printing rate for Printer B compare to the printing rate for Printer A? The printing rate for Printer B is than the rate for Printer A because the rate of 25 pages per minute is than the rate of for Printer A. pages per minute RIJOUT 40 fy Printer Rat Number of Pages 8N WA 10 30 20 Printer A 0 0 246 Time (min) Xarrow_forward
- OR 16 f(x) = Ef 16 χ по x²-2 410 | y = (x+2) + 4 Y-INT: y = 0 X-INT: X=0 VA: x=2 OA: y=x+2 0 X-INT: X=-2 X-INT: y = 2 VA 0 2 whole. 2-2 4 y - (x+2) = 27-270 + xxx> 2 क् above OA (x+2) OA x-2/x²+0x+0 2 x-2x 2x+O 2x-4 4 X<-1000 4/4/2<0 below Of y VA X=2 X-2 OA y=x+2 -2 2 (0,0) 2 χarrow_forwardI need help solving the equation 3x+5=8arrow_forwardWhat is the domain, range, increasing intervals (theres 3), decreasing intervals, roots, y-intercepts, end behavior (approaches four times), leading coffiencent status (is it negative, positivie?) the degress status (zero, undifined etc ), the absolute max, is there a absolute minimum, relative minimum, relative maximum, the root is that has a multiplicity of 2, the multiplicity of 3.arrow_forward
- What is the vertex, axis of symmerty, all of the solutions, all of the end behaviors, the increasing interval, the decreasing interval, describe all of the transformations that have occurred EXAMPLE Vertical shrink/compression (wider). or Vertical translation down, the domain and range of this graph EXAMPLE Domain: x ≤ -1 Range: y ≥ -4.arrow_forward4. Select all of the solutions for x²+x - 12 = 0? A. -12 B. -4 C. -3 D. 3 E 4 F 12 4 of 10arrow_forward2. Select all of the polynomials with the degree of 7. A. h(x) = (4x + 2)³(x − 7)(3x + 1)4 B h(x) = (x + 7)³(2x + 1)^(6x − 5)² ☐ Ch(x)=(3x² + 9)(x + 4)(8x + 2)ª h(x) = (x + 6)²(9x + 2) (x − 3) h(x)=(-x-7)² (x + 8)²(7x + 4)³ Scroll down to see more 2 of 10arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSON
Contemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Algebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSON
Introduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge Press
College Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education
Algebra and Trigonometry (6th Edition)
Algebra
ISBN:9780134463216
Author:Robert F. Blitzer
Publisher:PEARSON
Contemporary Abstract Algebra
Algebra
ISBN:9781305657960
Author:Joseph Gallian
Publisher:Cengage Learning
Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning
Algebra And Trigonometry (11th Edition)
Algebra
ISBN:9780135163078
Author:Michael Sullivan
Publisher:PEARSON
Introduction to Linear Algebra, Fifth Edition
Algebra
ISBN:9780980232776
Author:Gilbert Strang
Publisher:Wellesley-Cambridge Press
College Algebra (Collegiate Math)
Algebra
ISBN:9780077836344
Author:Julie Miller, Donna Gerken
Publisher:McGraw-Hill Education
What is a Linear Equation in One Variable?; Author: Don't Memorise;https://www.youtube.com/watch?v=lDOYdBgtnjY;License: Standard YouTube License, CC-BY
Linear Equation | Solving Linear Equations | What is Linear Equation in one variable ?; Author: Najam Academy;https://www.youtube.com/watch?v=tHm3X_Ta_iE;License: Standard YouTube License, CC-BY