
Concept explainers
The string shown in Figure P13.5 is driven at a frequency of 5.00 Hz. The amplitude of the motion is A = 12.0 cm, and the wave speed is v = 20.0 m/s. Furthermore, the wave is such that y = 0 at x = 0 and t = 0. Determine (a) the angular frequency and (b) the wave number for this wave. (c) Write an expression for the wave function. Calculate (d) the maximum transverse speed and (e) the maximum transverse acceleration of an element of the string.
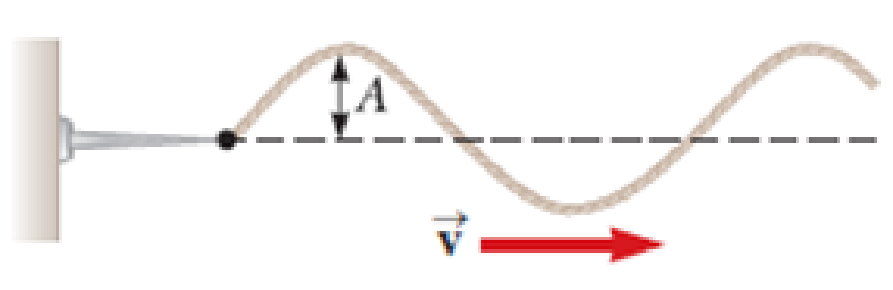
Figure P13.5
(a)
The angular frequency of the wave.
Answer to Problem 5P
The angular frequency of the wave is
Explanation of Solution
Write the expression for the frequency of the string.
Here,
Solve equation (I) for
Conclusion:
Substitute
Therefore, the angular frequency of the wave is
(b)
The wave number of the wave.
Answer to Problem 5P
The wave number of the wave is
Explanation of Solution
Write the expression for the wavelength of the wave.
Here,
Solve equation (III) for
Write the expression for the wavelength in terms of speed of the wave.
Conclusion:
Substitute
Substitute
Therefore, The wave number of the wave is
(c)
Expression for the wave function.
Answer to Problem 5P
Expression for the wave function is
Explanation of Solution
The general form of a wave function can be represented as,
Here,
Conclusion:
Using initial conditions, to make this fit,
In this case, taking initial conditions, substitute
Therefore, Expression for the wave function is
(d)
The maximum transverse speed of the wave.
Answer to Problem 5P
The maximum transverse speed of the wave is
Explanation of Solution
Write the expression for the transverse speed.
Differentiate equation (VI) in equation (VII),
Conclusion:
The maximum magnitude is given by,
Substitute
Therefore, the maximum transverse speed of the wave is
(e)
The maximum transverse acceleration of an element of the string.
Answer to Problem 5P
The maximum transverse acceleration of an element of the string.is
Explanation of Solution
Write the expression for the transverse acceleration.
Use equation (VIII) in equation (VII),
Conclusion:
The maximum magnitude is given by,
Substitute
Therefore, The maximum transverse acceleration of an element of the string.is
Want to see more full solutions like this?
Chapter 13 Solutions
Bundle: Principles of Physics: A Calculus-Based Text, 5th + WebAssign Printed Access Card for Serway/Jewett's Principles of Physics: A Calculus-Based Text, 5th Edition, Multi-Term
- Solve and answer the problem correctly and be sure to check your work. Thank you!!arrow_forwardA 10-m-long glider with a mass of 680 kg (including the passengers) is gliding horizontally through the air at 28 m/s when a 60 kg skydiver drops out by releasing his grip on the glider. What is the glider's speed just after the skydiver lets go?arrow_forwardPROBLEM 2 A cube of mass m is placed in a rotating funnel. (The funnel is rotating around the vertical axis shown in the diagram.) There is no friction between the cube and the funnel but the funnel is rotating at just the right speed needed to keep the cube rotating with the funnel. The cube travels in a circular path of radius r, and the angle between the vertical and the wall of the funnel is 0. Express your answers to parts (b) and (c) in terms of m, r, g, and/or 0. (a) Sketch a free-body diagram for the cube. Show all the forces acting on it, and show the appropriate coordinate system to use for this problem. (b) What is the normal force acting on the cube? FN=mg58 (c) What is the speed v of the cube? (d) If the speed of the cube is different from what you determined in part (c), a force of friction is necessary to keep the cube from slipping in the funnel. If the funnel is rotating slower than it was above, draw a new free-body diagram for the cube to show which way friction…arrow_forward
- Circular turns of radius r in a race track are often banked at an angle θ to allow the cars to achieve higher speeds around the turns. Assume friction is not present. Write an expression for the tan(θ) of a car going around the banked turn in terms of the car's speed v, the radius of the turn r, and g so that the car will not move up or down the incline of the turn. tan(θ) =arrow_forwardThe character Min Min from Arms was a DLC character added to Super Smash Bros. Min Min’s arms are large springs, with a spring constant of 8.53 ⋅ 10^3 N/m, which she uses to punch and fling away her opponents. Min Min pushes her spring arm against Steve, who is not moving, compressing it 1.20 m as shown in figure A. Steve has a mass of 81.6 kg. Assuming she uses only the spring to launch Steve, how fast is Steve moving when the spring is no longer compressed? As Steve goes flying away he goes over the edge of the level, as shown in figure C. What is the magnitude of Steve’s velocity when he is 2.00 m below where he started?arrow_forwardSlinky dog whose middle section is a giant spring with a spring constant of 10.9 N/m. Woody, who has a mass of 0.412 kg, grabs onto the tail end of Slink and steps off the bed with no initial velocity and reaches the floor right as his velocity hits zero again. How high is the bed? What is Woody’s velocity halfway down? Enter just the magnitude of velocity.arrow_forward
- No chatgpt pls will upvotearrow_forwardA positive charge of 91 is located 5.11 m to the left of a negative charge 92. The charges have different magnitudes. On the line through the charges, the net electric field is zero at a spot 2.90 m to the right of the negative charge. On this line there are also two spots where the potential is zero. (a) How far to the left of the negative charge is one spot? (b) How far to the right of the negative charge is the other?arrow_forwardA charge of -3.99 μC is fixed in place. From a horizontal distance of 0.0423 m, a particle of mass 7.31 x 103 kg and charge -9.76 µC is fired with an initial speed of 84.1 m/s directly toward the fixed charge. How far does the particle travel before its speed is zero?arrow_forward
- a) What is the minimum tension in N that the cable must be able to support without breaking? Assume the cable is massless. T = b) If the cable can only support a tension of 10,000 N what is the highest mass the ball can have in kg? mm =arrow_forwardCurve Fitter CURVE FITTER Open Update Fit Save New Exclusion Rules Select Validation Data Polynomial Exponential Logarithmic Auto Fourier Fit Fit Duplicate Data Manual FILE DATA FIT TYPE FIT Harmonic Motion X us 0.45 mi ce 0.4 0.35 0.3 0.25 0.2 Residuals Plot Contour Plot Plot Prediction Bounds None VISUALIZATION Colormap Export PREFERENCES EXPORT Fit Options COA Fourier Equation Fit Plot x vs. t -Harmonic Motion a0+ a1*cos(x*w) + b1*sin(x*w) Number of terms Center and scale 1 ▸ Advanced Options Read about fit options Results Value Lower Upper 0.15 a0 0.1586 0.1551 0.1620 a1 0.0163 0.0115 0.0211 0.1 b1 0.0011 -0.0093 0.0115 W 1.0473 0.9880 1.1066 2 8 10 t 12 14 16 18 20 Goodness of Fit Value Table of Fits SSE 0.2671 Fit State Fit name Data Harmonic Motion x vs. t Fit type fourier1 R-square 0.13345 SSE DFE 0.26712 296 Adj R-sq 0.12467 RMSE 0.030041 # Coeff Valic R-square 0.1335 4 DFE 296.0000 Adj R-sq 0.1247 RMSE 0.0300arrow_forwardWhat point on the spring or different masses should be the place to measure the displacement of the spring? For instance, should you measure to the bottom of the hanging masses?arrow_forward
 Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill
Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University
University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning





