Applied Statistics in Business and Economics
5th Edition
ISBN: 9780077837303
Author: David Doane, Lori Seward Senior Instructor of Operations Management
Publisher: McGraw-Hill Education
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 12.5, Problem 26SE
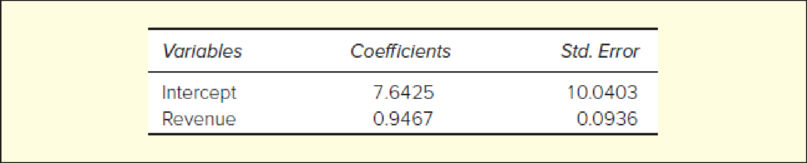
A regression was performed using data on 16 randomly selected charities. The variables were Y = expenses (millions of dollars) and X = revenue (millions of dollars). (a) Write the fitted regression equation. (b) Construct a 95 percent confidence interval for the slope. (c) Perform a right-tailed t test for zero slope at α = .05. State the hypotheses clearly. (d) Use Excel to find the p-value for the t statistic for the slope.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
find the (a) explained variation, (b) unexplained variation, and (c) indicated prediction interval. In each case, there is sujficient evidence to support a claim of a linear correlation, so it is reasonable to use the regression equation when making predictions.
Altitude and Temperature Listed below are altitudes (thousands of feet) and outside air temperatures (°F) recorded by the author during Delta Flight 1053 from New Orleans to Atlanta. For the prediction interval, use a 95% confidence level with the altitude of 6327 ft (or 6.327 thousand feet).
A football coach is looking for a way to identify players that are "under weight". The coach decides to get
data for each player's height (x, in inches) and weight (y, in pounds), then does a linear regression. The
results are:
y = - 58 + 4x,r = 0.95 and the standard error is Se = 13.
Since there is a strong linear correlation the coach, who also majored in Statistics, decides to identify all
"outliers" in the data.
Obviously, any player whose weight is above the regression line is not "under weight". So the only outliers
the coach is interested in are those that are below the regression line.
What is the lowest weight possible for a 67 inch player to not be considered "under weight"? Do not round.
Submit Question
MacBook Air
>>
F2
E3
000 E4
ES
F6
F7
F8
F10
F9
24
4.
%23
*
3.
8.
9.
Y
G
H.
J
K
C
V
M
3 BELK
command
A football coach is looking for a way to identify players that are "under weight". The coach decides to get
data for each player's height (x, in inches) and weight (y, in pounds), then does a linear regression. The
results are:
58+3.7x, r = 0.86 and the standard error is Se
= 10.4.
Since there is a strong linear correlation the coach, who also majored in Statistics, decides to identify all
"outliers" in the data.
Obviously, any player whose weight is above the regression line is not "under weight". So the only outliers
the coach is interested in are those that are below the regression line.
What is the lowest weight possible for a 75 inch player to not be considered "under weight"? Do not round.
Submit Question
ctor
GSearch or type URL
&
%
24
6
7
Chapter 12 Solutions
Applied Statistics in Business and Economics
Ch. 12.1 - Prob. 1SECh. 12.1 - Prob. 2SECh. 12.1 - Prob. 3SECh. 12.1 - Prob. 4SECh. 12.1 - Prob. 5SECh. 12.1 - Prob. 6SECh. 12.2 - (a) Interpret the slope of the fitted regression...Ch. 12.2 - (a) Interpret the slope of the fitted regression...Ch. 12.2 - Prob. 9SECh. 12.2 - (a) Interpret the slope of the fitted regression...
Ch. 12.2 - (a) Interpret the slope of the fitted regression...Ch. 12.3 - Prob. 12SECh. 12.3 - Prob. 13SECh. 12.3 - The regression equation Credits = 15.4 .07 Work...Ch. 12.3 - Below are fitted regressions for Y = asking price...Ch. 12.3 - Refer back to the regression equation in exercise...Ch. 12.3 - Refer back to the regression equation in exercise...Ch. 12.4 - Instructions for exercises 12.18 and 12.19: (a)...Ch. 12.4 - Instructions for exercises 12.18 and 12.19: (a)...Ch. 12.4 - Instructions for exercises 12.2012.22: (a) Use...Ch. 12.4 - Instructions for exercises 12.2012.22: (a) Use...Ch. 12.4 - Instructions for exercises 12.2012.22: (a) Use...Ch. 12.5 - Instructions for exercises 12.23 and 12.24: (a)...Ch. 12.5 - Prob. 24SECh. 12.5 - A regression was performed using data on 32 NFL...Ch. 12.5 - A regression was performed using data on 16...Ch. 12.6 - Prob. 27SECh. 12.6 - Prob. 28SECh. 12.6 - Instructions for exercises 12.2912.31: (a) Use...Ch. 12.6 - Instructions for exercises 12.2912.31: (a) Use...Ch. 12.6 - Instructions for exercises 12.2912.31: (a) Use...Ch. 12.7 - Refer to the Weekly Earnings data set below. (a)...Ch. 12.7 - Prob. 33SECh. 12.8 - Prob. 34SECh. 12.8 - Prob. 35SECh. 12.9 - An estimated regression for a random sample of...Ch. 12.9 - An estimated regression for a random sample of...Ch. 12.9 - Prob. 38SECh. 12.9 - Prob. 39SECh. 12 - (a) How does correlation analysis differ from...Ch. 12 - (a) What is a simple regression model? (b) State...Ch. 12 - (a) Explain how you fit a regression to an Excel...Ch. 12 - (a) Explain the logic of the ordinary least...Ch. 12 - (a) Why cant we use the sum of the residuals to...Ch. 12 - Prob. 6CRCh. 12 - Prob. 7CRCh. 12 - Prob. 8CRCh. 12 - Prob. 9CRCh. 12 - Prob. 10CRCh. 12 - Prob. 11CRCh. 12 - Prob. 12CRCh. 12 - (a) What is heteroscedasticity? Identify its two...Ch. 12 - (a) What is autocorrelation? Identify two main...Ch. 12 - Prob. 15CRCh. 12 - Prob. 16CRCh. 12 - (a) What is a log transform? (b) What are its...Ch. 12 - Prob. 40CECh. 12 - Prob. 41CECh. 12 - Prob. 42CECh. 12 - Prob. 43CECh. 12 - Prob. 44CECh. 12 - Prob. 45CECh. 12 - Prob. 46CECh. 12 - Prob. 47CECh. 12 - Prob. 48CECh. 12 - Prob. 49CECh. 12 - Prob. 50CECh. 12 - Prob. 51CECh. 12 - Prob. 52CECh. 12 - Prob. 53CECh. 12 - Prob. 54CECh. 12 - Prob. 55CECh. 12 - Prob. 56CECh. 12 - Prob. 57CECh. 12 - Prob. 58CECh. 12 - Prob. 59CECh. 12 - In the following regression, X = weekly pay, Y =...Ch. 12 - Prob. 61CECh. 12 - In the following regression, X = total assets (...Ch. 12 - Prob. 63CECh. 12 - Below are percentages for annual sales growth and...Ch. 12 - Prob. 65CECh. 12 - Prob. 66CECh. 12 - Prob. 67CECh. 12 - Simple regression was employed to establish the...Ch. 12 - Prob. 69CECh. 12 - Prob. 70CECh. 12 - Prob. 71CECh. 12 - Below are revenue and profit (both in billions)...Ch. 12 - Below are fitted regressions based on used vehicle...Ch. 12 - Below are results of a regression of Y = average...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, statistics and related others by exploring similar questions and additional content below.Similar questions
- Find the equation of the regression line for the following data set. x 1 2 3 y 0 3 4arrow_forwardFor the following exercises, use Table 4 which shows the percent of unemployed persons 25 years or older who are college graduates in a particular city, by year. Based on the set of data given in Table 5, calculate the regression line using a calculator or other technology tool, and determine the correlation coefficient. Round to three decimal places of accuracyarrow_forwardWhat is the differed annual expenditures of two families if their annual net incomes are differed by 2000? The computed regression line has a value of a=4.32 and b=2.12.arrow_forward
- The number of pounds of steam used per month by a chemical plant is thought to be related to the average ambient temperature (in °F) for that month. The past year's usage and temperatures are in the following table: (a) Find a regression relating steam usage to temperature. (b) Test for significance of regression using a=0.05. (c) Find a 99% confidence interval for B1 Usage/ 1000 Usage/ 1000 Month Temp. Month Temp. Jan. 21 185.79 July. 68 621.55 Feb. 24 214.47 Aug. 74 675.06 Mar. 32 288.03 Sept. 62 562.03 Apr.o 50 452.93 47 424.84 Oct. May 50 454.58 Nov. 41 369.95 June 59 539.03 Dec. 30 a 273.98arrow_forwardAn amusement park owner wants to create a linear regression model to predict the number of ice cream cones sold (Y-variable) based on the day's attendance (X-variable). From his collected data he finds the mean number of ice cream cone sales to be 1,200 cones with a standard deviation of 180. The mean daily attendance is 2,000 people, with a standard deviation of 200. The correlation between ice cream cone sales and attendance is r = 0.65. What is the intercept of the linear regression model predicting ice cream cone sales from daily attendance? 200 80 130 30arrow_forwardThe Heights and Weights of 500 preschoolers have correlation r=0.52. The average height is 30.12 Inches, the average weight is 40.90 Pounds, the standard deviation of their heights is 4.16 inches, and the standard deviation of their weight is 6.42 Pounds. 1. What is the equation of the least squares regression line for predicting their weights from their heights? 2. I'm 35 Inches Tall- Predict my weight.arrow_forward
- When is the coefficient of certainty obtained from the regression equation equal to the square of the coefficient of correlation between the variables?arrow_forwardThe accompanying table lists overhead widths (cm) of seals measured from photographs and the weights (kg) of the seals. Find the (a) explained variation, (b) unexplained variation, and (c) prediction interval for an overhead width of 9.2 cm using a 99% confidence level. There is sufficient evidence to support a claim of a linear correlation, so it is reasonable to use the regression equation when making predictions. Click the icon to view the seal data. a. The explained variation is (Round to the nearest integer as needed.) b. The unexplained variation is (Round to the nearest integer as needed.) c. The 99% prediction interval for an overhead width of 9.2 cm is ☐ kgarrow_forwardListed below are systolic blood pressure measurements (in mm Hg) obtained from the same woman. Find the regression equation, letting the right arm blood pressure be the predictor (x) variable. Find the best predicted systolic blood pressure in the left arm given that the systolic blood pressure in the right arm is 90 mm Hg. Use a significance level of 0.05 Right arm - 102; 101; 94; 79; 80 Left arm - 177; 172; 143; 144; 143 The regression equation is y(carety)= ___+___x. Given that the systolic blood pressure in the right arm is 90mm Hg, the best predicted systolic blood pressure in the left arm is _____ mm Hg.arrow_forwardFour pairs of data yield r= 0.942 and regression equation y=3x.Also, y= 12.75. What is the best predicted value of y for x= 2.9?arrow_forwardA professor obtains SAT scores and freshman grade point averages (GPAs) for a group of n = 15 college students. The SAT scores have a mean of M = 580 with SS = 22,400, and the GPAs have a mean of 3.10 with SS = 1.26, and SP = 84. Find the regression equation for predicting GPA from SAT scores. What percentage of the variance in GPAs is accounted for by the regression equation? (Compute the correlation r, then find r^2.) Does the regression equation account for a significant portion of the variance in GPA? Use α = .05 to evaluate the F-ratio.arrow_forwardThe accompanying table lists overhead widths (cm) of seals measured from photographs and the weights (kg) of the seals. Find the (a) explained variation, (b) unexplained variation, and (c) prediction interval for an overhead width of 8.9 cm using a 99% confidence level. There is sufficient evidence to support a claim of a linear correlation, so it is reasonable to use the regression equation when making predictions. Click the icon to view the seal data. a. The explained variation is (Round to the nearest integer as needed.) b. The unexplained variation is. (Round to the nearest integer as needed.) c. The 99% prediction interval for an overhead width of 8.9 cm is kgarrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_iosRecommended textbooks for you
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning
Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw HillFunctions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage LearningBig Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
Correlation Vs Regression: Difference Between them with definition & Comparison Chart; Author: Key Differences;https://www.youtube.com/watch?v=Ou2QGSJVd0U;License: Standard YouTube License, CC-BY
Correlation and Regression: Concepts with Illustrative examples; Author: LEARN & APPLY : Lean and Six Sigma;https://www.youtube.com/watch?v=xTpHD5WLuoA;License: Standard YouTube License, CC-BY