Chapter 12, Problem 1P

a.

To determine

Construct the decision tree.

Explanation of Solution

Here, there are two decision alternatives d₁ and d₂ and the three states of nature s₁, s₂  and s₃.

Thus, the decision tree is given as follows:

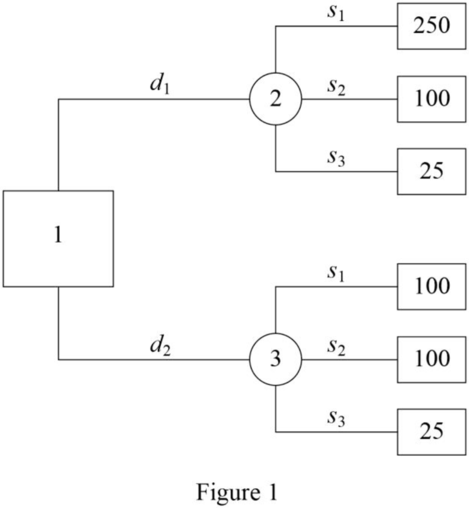
Thus, the decision tree has been constructed.

b.

To determine

Find the decision using the optimistic, conservative and minimax regret approaches.

Answer to Problem 1P

The decision d₁ gives the optimistic approach because it has the largest maximum profit.

The decision d₂ gives the conservative approach because it has the largest minimum profit.

The minimax regret is the decision d₁ because it minimizes the maximum regret.

Explanation of Solution

By using the decision tree in Part (a), the maximum and minimum profit based on the decisions d₁ and d₂.

Decision	Maximum Profit	Minimum Profit
d1	250	25
d2	100	75

Optimistic approach:

The optimistic approach evaluates each decision alternative in terms of best payoff that can occur.

The decision d₁ gives the optimistic approach because it has the largest maximum profit (from the above table).

Conservative approach:

The conservative approach evaluates each decision alternative in terms of worst payoff that can occur.

The decision d₂ gives the conservative approach because it has the largest minimum profit (from the above table).

Minimax Regret approach:

The minimax regret approach is the difference between the payoff associated with a particular alternative and payoff associated with the most decision that would yield the most desirable payoff for a given state of nature.

Regret or opportunity loss table:

Decision	s1	s2	s3
d1	0	0	50
d2	150	0	0

The maximum regret for the decision d₁ is 50 and d₂  is 150.

Here, the decision d₁ has been selected because it minimizes the maximum regret.