
A tall tower of circular cross section is reinforced by horizontal circular disks (like large coins), one meter apart and of negligible thickness. The radius Of the disk at height n is
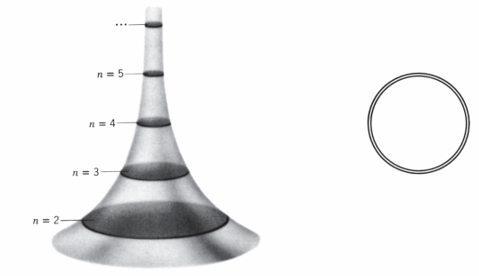
Assuming that the tower is of infinite height:
- Will the total area of the disks be finite or not? Hint: Can you compare the series with a simpler one?
- If the disks are strengthened by wires going around their circumferences like tires, will the total length of wire required be finite or not?
- Explain why there is not a contradiction between your answers in (a) and (b). That is, how is it possible to start with a set of disks of finite area, remove a little strip around the circumference of each, and get an infinite total length of these strips? Hint: Think about units—you can’t compare area and length. Consider two: (1) Make the width of each strip equal to one percent of the radius of the disk from which you cut it. Now the total length is infinite but what about the total area? (2) Try to make the strips all the same width; what happens? Also see Chapter 5, Problem 3.31 (b).
Want to see the full answer?
Check out a sample textbook solution
Chapter 1 Solutions
Mathematical Methods in the Physical Sciences
Additional Math Textbook Solutions
Calculus for Business, Economics, Life Sciences, and Social Sciences (14th Edition)
Precalculus
Basic Business Statistics, Student Value Edition
University Calculus: Early Transcendentals (4th Edition)
Thinking Mathematically (6th Edition)
College Algebra with Modeling & Visualization (5th Edition)
- enter | Infinite Camp ilc 8.3 End-of-Unit Assessment, Op x Pride is the Devil - Google Drive x + 2 sdphiladelphia.ilclassroom.com/assignments/7FQ5923/lesson?card=806642 3 Problem 2 A successful music app tracked the number of song downloads each day for a month for 4 music artists, represented by lines l, j, m, and d over the course of a month. Which line represents an artist whose downloads remained constant over the month? Select the correct choice. = Sidebar Tools M 45 song downloads days d 1 2 3 4 5 6 7 8 00 8 m l RA 9 > КУ Fullscreen G Save & Exit De ☆arrow_forwardQ/Determine the set of points at which - f(z) = 622 2≥ - 4i/z12 i and differentiable analytice is:arrow_forwardsy = f(x) + + + + + + + + + X 3 4 5 7 8 9 The function of shown in the figure is continuous on the closed interval [0, 9] and differentiable on the open interval (0, 9). Which of the following points satisfies conclusions of both the Intermediate Value Theorem and the Mean Value Theorem for f on the closed interval [0, 9] ? (A A B B C Darrow_forward
- = Q6 What will be the allowable bearing capacity of sand having p = 37° and ydry 19 kN/m³ for (i) 1.5 m strip foundation (ii) 1.5 m x 1.5 m square footing and (iii)1.5m x 2m rectangular footing. The footings are placed at a depth of 1.5 m below ground level. Assume F, = 2.5. Use Terzaghi's equations. 0 Ne Na Ny 35 57.8 41.4 42.4 40 95.7 81.3 100.4arrow_forwardQ1 The SPT records versus depth are given in table below. Find qan for the raft 12% foundation with BxB-10x10m and depth of raft D-2m, the allowable settlement is 50mm. Elevation, m 0.5 2 2 6.5 9.5 13 18 25 No.of blows, N 11 15 29 32 30 44 0 estigate shear 12%arrow_forward2arrow_forward
 Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning, Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal LittellAlgebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal LittellAlgebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning
Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,




