
To make a two-way table with conditional relative frequencies based on column totals and conditional relative frequency that the students prefer a cartoon mascot that is a hawk.
Answer to Problem 24E
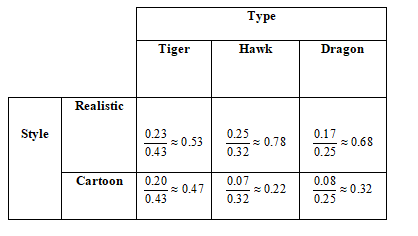
This is the correct two-way table with joint relative frequencies.
The conditional relative frequency that the students prefer a cartoon mascot that is a hawk is 0.22
Explanation of Solution
Given information:
The information provided in the two-way table.
Formula Used:
Joint relative frequencies are found out by dividing each joint frequency by the total number of observations in the two-way table.
Sum up the rows of the joint relative frequencies in order to arrive at the marginal relative frequencies.
Divide the joint relative frequencies with the marginal relative frequencies to get the resulting two-way table.
Calculation:
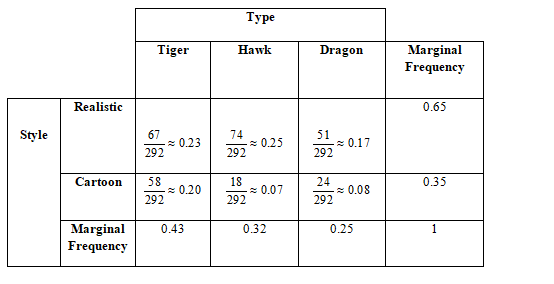
Total number of observations is
Hence the correct table of joint relative frequencies is
For instance, the joint relative frequency of mascot of realistic style of tiger type is
The correct calculation is
Next, divide the joint relative frequencies by the marginal relative frequencies on the column totals.
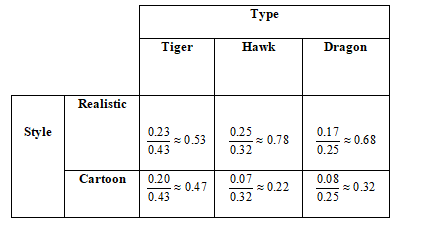
The conditional relative frequency that the students prefer a cartoon mascot that is a hawk is 0.22
Chapter 11 Solutions
BIG IDEAS MATH Integrated Math 1: Student Edition 2016
- TRIANGLES INDEPENDENT PRACTICE ription Criangle write and cow Using each picture or description of triangle write and solve an equation in ordering the number of degrees in each angle TRIANGLE EQUATION & WORK ANGLE MEASURES A B -(7x-2)° (4x) (3x)° (5x − 10) C (5x – 2) (18x) E 3. G 4. H (16x)° LL 2A= 2B= ZE=arrow_forwardi circled the correct answer could you explain how to get that answer, as well as disprove the other optionsarrow_forwardthe answer is A, could you explain how using STOKE'S theoremarrow_forwardcould you explain this pleasethe answer is has sum 1but i dont know how to calculate itarrow_forwardcan you explain why the answer is 1/3arrow_forwardThe position of a particle that moves along the x-axis is defined by x = - 3t^2 + 12^t - 6 f, where t is in seconds. For the time interval t = 0 to t = 3 s, (1) plot the position, velocity, and acceleration as functions of time; (2) calculate the distance traveled; and (3) determine the displacement of the particleshow the graph and write the solution with a penarrow_forwardThe position of a particle that moves along the x-axis is defined by x = - 3t^2 + 12^t - 6 f, where t is in seconds. For the time interval t = 0 to t = 3 s, (1) plot the position, velocity, and acceleration as functions of time; (2) calculate the distance traveled; and (3) determine the displacement of the particleshow the graph and write the solution with a penarrow_forwardThe answer for number 1 is D Could you show me whyarrow_forwardThe path of a particle moving in a straight line is given by s = t^3 - 6t^2+ 9t + 4, where s is in ft and t in seconds. a. Finds and a when v = 0. b. Find s and v when a = 0.show the graph if needed and write the solution with a penarrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_ios
 Discrete Mathematics and Its Applications ( 8th I...MathISBN:9781259676512Author:Kenneth H RosenPublisher:McGraw-Hill Education
Discrete Mathematics and Its Applications ( 8th I...MathISBN:9781259676512Author:Kenneth H RosenPublisher:McGraw-Hill Education Mathematics for Elementary Teachers with Activiti...MathISBN:9780134392790Author:Beckmann, SybillaPublisher:PEARSON
Mathematics for Elementary Teachers with Activiti...MathISBN:9780134392790Author:Beckmann, SybillaPublisher:PEARSON
 Thinking Mathematically (7th Edition)MathISBN:9780134683713Author:Robert F. BlitzerPublisher:PEARSON
Thinking Mathematically (7th Edition)MathISBN:9780134683713Author:Robert F. BlitzerPublisher:PEARSON Discrete Mathematics With ApplicationsMathISBN:9781337694193Author:EPP, Susanna S.Publisher:Cengage Learning,
Discrete Mathematics With ApplicationsMathISBN:9781337694193Author:EPP, Susanna S.Publisher:Cengage Learning, Pathways To Math Literacy (looseleaf)MathISBN:9781259985607Author:David Sobecki Professor, Brian A. MercerPublisher:McGraw-Hill Education
Pathways To Math Literacy (looseleaf)MathISBN:9781259985607Author:David Sobecki Professor, Brian A. MercerPublisher:McGraw-Hill Education





