Physics, Books a la Carte Edition (5th Edition)

5th Edition

ISBN: 9780134020853

Author: James S. Walker

Publisher: PEARSON

See similar textbooks

Concept explainers

Angular speed, acceleration and displacement

Angular acceleration is defined as the rate of change in angular velocity with respect to time. It has both magnitude and direction. So, it is a vector quantity.

Angular Position

Before diving into angular position, one should understand the basics of position and its importance along with usage in day-to-day life. When one talks of position, it’s always relative with respect to some other object. For example, position of earth w…

Videos

Textbook Question

Chapter 11, Problem 95PP

BIO Correcting Torsiversion

Torsiversion is a medical condition in which a tooth is rotated away from its normal position about the long axis of the root Studies show that about 2 percent of the population suffer from this condition to some degree. For those who do, the improper alignment of the tooth can lead to tooth-to-tooth collisions during eating, as well as other problems. Typical patients display a rotation ranging from 20° to 60°, with an average around 30°.

An example is shown in Figure 11-69 (a), where the first premolar is not only displaced slightly from its proper location in the negative y direction, but also rotated clockwise from its normal orientation. To correct this condition an orthodontist might use an archwire and a bracket to apply both a force and a torque to the tooth. In the simplest case, two forces are applied to the tooth in different locations, as indicated by F₁ and F₂ in Figure 11-69 (a). These two forces, if chosen properly, can reposition the tooth by exerting a net force in the positive y direction, and also reorient it by applying a torque in the counterclockwise direction.

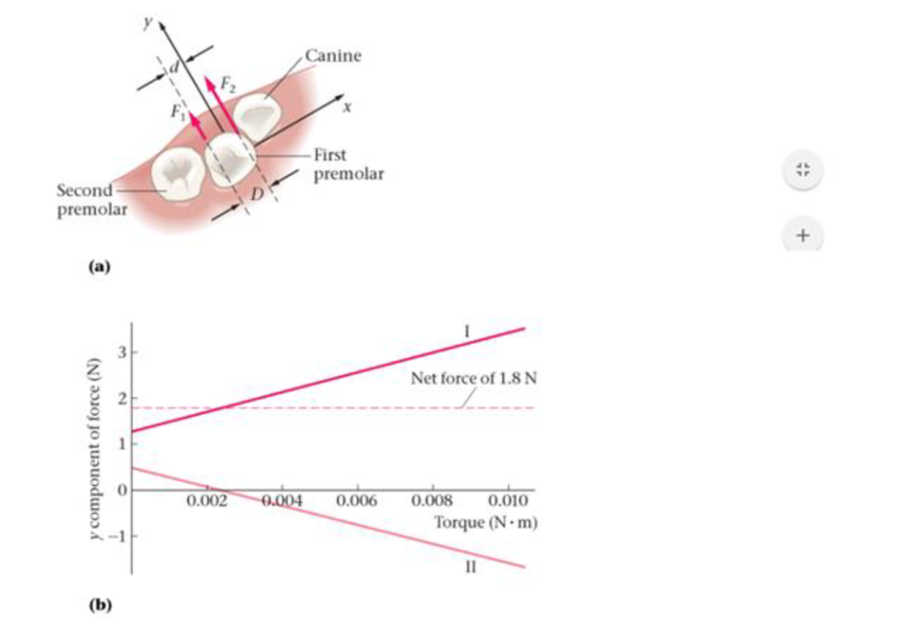
Chapter 11, Problem 95PP, BIO Correcting Torsiversion Torsiversion is a medical condition in which a tooth is rotated away

Figure 11-69 Problems 92, 93, 94, and 95

In a typical case it may be desired to have a net force in the positive y direction of 1.8 N. In addition, the distances in Figure 11-69 (a) can be taken to be d = 3.2 mm and D = 4.5 mm. Given these conditions, a range of torques is possible for various values of the y components of the forces, F_1y and F_2y. For example Figure 11-69 (b) shows the values of F_1y and F_2y necessary to produce a given torque, where the torque is measured about the center of the tooth (which is also the origin of the coordinate system). Notice that the two forces always add to 1.8 N in the positive y direction, though one of the forces changes sign as the torque is increased.

95. •• Find the values of F_1y and F_2y required to give zero net torque of 0.0099 N · m. This is a torque that would be effective at rotating the tooth.

A. F_1y = −1.7 N, F_2y = 3.5 N
B. F_1y = −3.8 N, F_2y = 5.6 N
C. F_1y = −0.23 N, F_2y = 2.0 N
D. F_1y = −4.0 N, F_2y = −2.2N

Expert Solution & Answer

Want to see the full answer?

Check out a sample textbook solution

See solution

Chapter 11, Problem 94PP

Chapter 11, Problem 96PP

Students have asked these similar questions

A rocket is launched at an angle of 60.0° above the horizontal with an initial speed of 97 m/s. The rocket moves for 3.00 s along its initial line of motion with an acceleration of 28.0 m/s². At this time, its engines fail and the rocket proceeds to move as a projectile. (a) Find the maximum altitude reached by the rocket. 1445.46 Your response differs from the correct answer by more than 10%. Double check your calculations. m (b) Find its total time of flight. 36.16 x Your response is within 10% of the correct value. This may be due to roundoff error, or you could have a mistake in your calculation. Carry out all intermediate results to at least four-digit accuracy to minimize roundoff error. s (c) Find its horizontal range. 1753.12 × Your response differs from the correct answer by more than 10%. Double check your calculations. m

Race car driver is cruising down the street at a constant speed of 28.9 m/s (~65 mph; he has a “lead” foot) when the traffic light in front of him turns red. a) If the driver’s reaction time is 160 ms, how far does he and his car travel down the road from the instant he sees the light change to the instant he begins to slow down? b) If the driver’s combined reaction and movement time is 750 ms, how far do he and his car travel down the road from the instant he sees the light change to the instant he slams on her brakes and car begins to slow down? Please answer parts a-B. Show all work. For each question draw a diagram to show the vector/s. Show all the step and provide units in the answers. Provide answer to 2 decimal places. DONT FORGET TO DRAW VECTORS! ONLY USE BASIC FORMULAS TAUGHT IN PHYSICS. distance = speed * time.

Race car driver is cruising down the street at a constant speed of 28.9 m/s (~65 mph; he has a “lead” foot) when the traffic light in front of him turns red. a) If the driver’s reaction time is 160 ms, how far does he and his car travel down the road from the instant he sees the light change to the instant he begins to slow down? b) If the driver’s combined reaction and movement time is 750 ms, how far do he and his car travel down the road from the instant he sees the light change to the instant he slams on her brakes and car begins to slow down? c) If the driver’s average rate of acceleration is -9.5 m/s2 as he slows down, how long does it take him to come to a stop (use information about his speed of 28.9 m/s but do NOT use his reaction and movement time in this computation)? Please answer parts a-c. Show all work. For each question draw a diagram to show the vector/s. Show all the step and provide units in the answers. Provide answer to 2 decimal places unless stated otherwise.…

Chapter 11 Solutions

Physics, Books a la Carte Edition (5th Edition)

Ch. 11.1 - A bicycle wheel is mounted on an axle, as shown in...Ch. 11.2 - Consider two objects with the following...Ch. 11.3 - A Physics sign is supported symmetrically by two...Ch. 11.4 - A mobile made from three piggy banks (A, B, C) is...Ch. 11.5 - Prob. 5EYU Ch. 11.6 - Consider two objects with the following...Ch. 11.7 - Prob. 7EYU Ch. 11.8 - In system 1, a torque of 20 N m acts through an...Ch. 11.9 - The angular velocity of the spinning bicycle wheel...Ch. 11 - Two forces produce the same torque. Does it follow...

Additional Science Textbook Solutions

Find more solutions based on key concepts

Which culture uses NAD+? Use the following choices to answer questions. a. E. coli growing in glucose broth at ...

Microbiology: An Introduction

Q1. Which wavelength of light has the highest frequency? a) 10 nm b) 10 mm c) 1 nm d) 1 mm

Chemistry: A Molecular Approach (4th Edition)

Use the key to classify each of the following described tissue types into one of the four major tissue categori...

Anatomy & Physiology (6th Edition)

In pea plants, plant height, seed shape, and seed color are governed by three independently assorting genes. Th...

Genetic Analysis: An Integrated Approach (3rd Edition)

All of the following processes are involved in the carbon cycle except: a. photosynthesis b. cell respiration c...

Human Biology: Concepts and Current Issues (8th Edition)

1.1 Write a one-sentence definition for each of the following: a. chemistry b. chemical

Chemistry: An Introduction to General, Organic, and Biological Chemistry (13th Edition)

Knowledge Booster

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.