Chapter 11, Problem 19RE

To determine

To find: The center, vertices, foci and asymptotes of the hyperbola.

Explanation of Solution

Given information:

Equation of the hyperbola is given

  $x^2-2y^2=16$

Calculation:

The standard form of the equation of a hyperbola with center $\left(0,0\right)$ and the transverse axis on the x − axis is

  $\frac{x^2}{a^2}-\frac{y^2}{b^2}=1$

Then:-

Length of the transverse axis is $2a$

Coordinates of the vertices are $\left(\pm a,0\right)$

Length of the conjugate axis is $2b$

Coordinate of the co − vertices are $\left(0,\pm b\right)$

Distance between the foci is $2c$

  $c^2=a^2+b^2$

Coordinate of foci are $\left(\pm c,0\right)$

Equations of the asymptotes are $y=\frac{\pm bx}{a}$

Given equation of the hyperbola is:-

  $x^2-2y^2=16$

The hyperbola transverse on the x − axis

Center of the hyperbola $=\left(0,0\right)$

Coordinate of the foci $=\left(\pm c,0\right)$

  $\begin{array}{l}{c}^2=a^2+b^2\\ a^2=16,b^2=8\\ c^2=24\end{array}$

Foci $=\left(\sqrt{24},0\right)\text{ and }\left(-\sqrt{24},0\right)$

Vertex of hyperbola $\left(\pm a,0\right)$

  $\begin{array}{l}{a}^2=16\Rightarrow a=4\\ \Rightarrow \left(4,0\right)\text{ and }\left(-4,0\right)\end{array}$

Equation of the asymptotes $\Rightarrow y=\frac{bxr}{b}$

  $\begin{array}{l}{b}^2=8a^2=16\\ b=\sqrt{8}a=4\\ y=\frac{\pm 2\sqrt{2}x}{4}\\ y=\frac{2\sqrt{2}x}{4}\text{ and }y=\frac{-x}{\sqrt{2}}\end{array}$

  

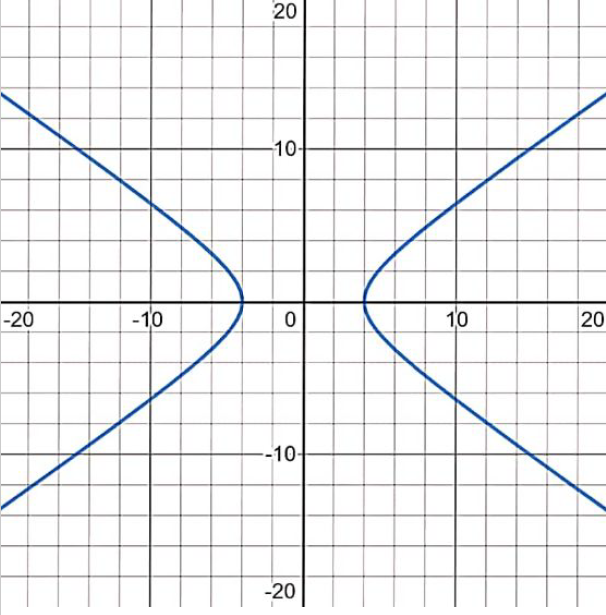
Interpretation :

Through the standard form of the equation of a hyperbola the following values are arrived at.

Center $=\left(0,0\right)$ ,

Vertices $\Rightarrow \left(4,0\right)\text{ and }\left(-4,0\right)$

Foci $\Rightarrow \left(\sqrt{24},0\right)\text{ and }\left(-\sqrt{24},0\right)$

Asymptotes $\Rightarrow y=\frac{x}{\sqrt{2}}\text{ and }y=\frac{-x}{\sqrt{2}}$