
Concept explainers
What is the coordination number of each sphere in (a) a simple cubic cell, (b) a body-centered cubic cell, and (c) a face-centered cubic cell? Assume the spheres are all the same.
(a)
Interpretation:
The co-ordination number of each sphere in simple cubic unit cell, body-centered cubic unit cell and face-centered cubic unit cell have to be identified.
Concept Introduction:
The simplest and basic unit of a crystalline solid is known as unit cell. It is cubic in shape. It is the building block of crystalline solids. The unit cells repeat themselves to build a lattice. Crystalline solids consist of many of such lattices. There are three types of unit cell – simple cubic unit cell, body – centered cubic unit cell and face – centered cubic unit cell.
In packing of the components in a solid, the components are imagined as spheres. A simple cubic unit cell is the simplest form of a cubic unit cell. A cube has eight vertices, twelve edges and six faces. Similarly a cubic unit cell has eight vertices, twelve edges and six faces. If in a cubic unit cell, the components occupy only the eight vertices, then the unit cell is known as simple cubic unit cell. So, each simple cubic unit cell has
In a body – centered cubic unit cell is another type of unit cell in which atoms are arranged in all the eight vertices of the unit cell with one atom per vertex. Further one atom occupies the center of the cube. Thus the number of atoms per unit cell in BCC unit cell is,
In a face – centered cubic unit cell the atoms are arranged in all the eight vertices of the unit cell with one atom per vertex. Further all the six faces of a cubic unit cell are occupied with one atom per face. Thus the number of atoms per unit cell in FCC unit cell is,
Co-ordination number of an atom or sphere is termed as the number of spheres it touches in the unit cell.
Answer to Problem 11.37QP
Co-ordination number of simple cubic unit cell is six.
Explanation of Solution
In simple cubic unit cell each sphere at the corner of the cubic unit cell is shared by eight other spheres. Each sphere is surrounded by six spheres.
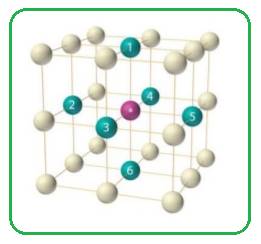
Figure 1
Thus the co-ordination number of simple cubic unit cell is six.
(b)
Interpretation:
The co-ordination number of each sphere in simple cubic unit cell, body-centered cubic unit cell and face-centered cubic unit cell have to be identified.
Concept Introduction:
The simplest and basic unit of a crystalline solid is known as unit cell. It is cubic in shape. It is the building block of crystalline solids. The unit cells repeat themselves to build a lattice. Crystalline solids consist of many of such lattices. There are three types of unit cell – simple cubic unit cell, body – centered cubic unit cell and face – centered cubic unit cell.
In packing of the components in a solid, the components are imagined as spheres. A simple cubic unit cell is the simplest form of a cubic unit cell. A cube has eight vertices, twelve edges and six faces. Similarly a cubic unit cell has eight vertices, twelve edges and six faces. If in a cubic unit cell, the components occupy only the eight vertices, then the unit cell is known as simple cubic unit cell. So, each simple cubic unit cell has
In a body – centered cubic unit cell is another type of unit cell in which atoms are arranged in all the eight vertices of the unit cell with one atom per vertex. Further one atom occupies the center of the cube. Thus the number of atoms per unit cell in BCC unit cell is,
In a face – centered cubic unit cell the atoms are arranged in all the eight vertices of the unit cell with one atom per vertex. Further all the six faces of a cubic unit cell are occupied with one atom per face. Thus the number of atoms per unit cell in FCC unit cell is,
Co-ordination number of an atom or sphere is termed as the number of spheres it touches in the unit cell.
Answer to Problem 11.37QP
Co-ordination number of Body-centered cubic unit cell is eight.
Explanation of Solution
There are two spheres per BCC unit cell. Each sphere touches eight other spheres.
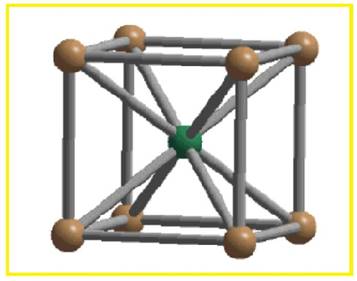
Figure 2
Thus the co-ordination number of body-centered cubic unit cell is eight.
(c)
Interpretation:
The co-ordination number of each sphere in simple cubic unit cell, body-centered cubic unit cell and face-centered cubic unit cell have to be identified.
Concept Introduction:
The simplest and basic unit of a crystalline solid is known as unit cell. It is cubic in shape. It is the building block of crystalline solids. The unit cells repeat themselves to build a lattice. Crystalline solids consist of many of such lattices. There are three types of unit cell – simple cubic unit cell, body – centered cubic unit cell and face – centered cubic unit cell.
In packing of the components in a solid, the components are imagined as spheres. A simple cubic unit cell is the simplest form of a cubic unit cell. A cube has eight vertices, twelve edges and six faces. Similarly a cubic unit cell has eight vertices, twelve edges and six faces. If in a cubic unit cell, the components occupy only the eight vertices, then the unit cell is known as simple cubic unit cell. So, each simple cubic unit cell has
In a body – centered cubic unit cell is another type of unit cell in which atoms are arranged in all the eight vertices of the unit cell with one atom per vertex. Further one atom occupies the center of the cube. Thus the number of atoms per unit cell in BCC unit cell is,
In a face – centered cubic unit cell the atoms are arranged in all the eight vertices of the unit cell with one atom per vertex. Further all the six faces of a cubic unit cell are occupied with one atom per face. Thus the number of atoms per unit cell in FCC unit cell is,
Co-ordination number of an atom or sphere is termed as the number of spheres it touches in the unit cell.
Answer to Problem 11.37QP
Co-ordination number of Face-centered cubic unit cell is twelve.
Explanation of Solution
There are four spheres per FCC unit cell. Each sphere is in contact with twelve spheres.
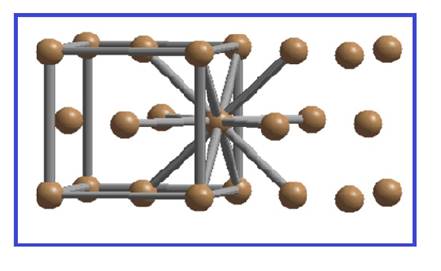
Figure 3
Thus the co-ordination number of simple cubic unit cell is twelve.
Want to see more full solutions like this?
Chapter 11 Solutions
Chemistry
- Please help me find the 1/Time, Log [I^-] Log [S2O8^2-], Log(time) on the data table. With calculation steps. And the average for runs 1a-1b. Please help me thanks in advance. Will up vote!arrow_forwardQ1: Answer the questions for the reaction below: ..!! Br OH a) Predict the product(s) of the reaction. b) Is the substrate optically active? Are the product(s) optically active as a mix? c) Draw the curved arrow mechanism for the reaction. d) What happens to the SN1 reaction rate in each of these instances: 1. Change the substrate to Br "CI 2. Change the substrate to 3. Change the solvent from 100% CH3CH2OH to 10% CH3CH2OH + 90% DMF 4. Increase the substrate concentration by 3-fold.arrow_forwardExperiment 27 hates & Mechanisms of Reations Method I visual Clock Reaction A. Concentration effects on reaction Rates Iodine Run [I] mol/L [S₂082] | Time mo/L (SCC) 0.04 54.7 Log 1/ Time Temp Log [ ] 13,20] (time) / [I] 199 20.06 23.0 30.04 0.04 0.04 80.0 22.8 45 40.02 0.04 79.0 21.6 50.08 0.03 51.0 22.4 60-080-02 95.0 23.4 7 0.08 0-01 1970 23.4 8 0.08 0.04 16.1 22.6arrow_forward
- (15 pts) Consider the molecule B2H6. Generate a molecular orbital diagram but this time using a different approach that draws on your knowledge and ability to put concepts together. First use VSEPR or some other method to make sure you know the ground state structure of the molecule. Next, generate an MO diagram for BH2. Sketch the highest occupied and lowest unoccupied MOs of the BH2 fragment. These are called frontier orbitals. Now use these frontier orbitals as your basis set for producing LGO's for B2H6. Since the BH2 frontier orbitals become the LGOS, you will have to think about what is in the middle of the molecule and treat its basis as well. Do you arrive at the same qualitative MO diagram as is discussed in the book? Sketch the new highest occupied and lowest unoccupied MOs for the molecule (B2H6).arrow_forwardQ8: Propose an efficient synthesis of cyclopentene from cyclopentane.arrow_forwardQ7: Use compound A-D, design two different ways to synthesize E. Which way is preferred? Please explain. CH3I ONa NaOCH 3 A B C D E OCH3arrow_forward
- Predict major product(s) for the following reactions. Note the mechanism(s) of the reactions (SN1, E1, SN2 or E2).arrow_forward(10 pts) The density of metallic copper is 8.92 g cm³. The structure of this metal is cubic close-packed. What is the atomic radius of copper in copper metal?arrow_forwardPredict major product(s) for the following reactions. Note the mechanism(s) of the reactions (SN1, E1, SN2 or E2).arrow_forward
- Predict major product(s) for the following reactions. Note the mechanism(s) of the reactions (SN1, E1, SN2 or E2).arrow_forwardQ3: Rank the following compounds in increasing reactivity of E1 and E2 eliminations, respectively. Br ca. go do A CI CI B C CI Darrow_forwardQ5: Predict major product(s) for the following reactions. Note the mechanism(s) of the reactions (SN1, E1, SN2 or E2). H₂O דיי "Br KN3 CH3CH2OH NaNH2 NH3 Page 3 of 6 Chem 0310 Organic Chemistry 1 HW Problem Sets CI Br excess NaOCH 3 CH3OH Br KOC(CH3)3 DuckDuckGarrow_forward
 Chemistry: Principles and PracticeChemistryISBN:9780534420123Author:Daniel L. Reger, Scott R. Goode, David W. Ball, Edward MercerPublisher:Cengage Learning
Chemistry: Principles and PracticeChemistryISBN:9780534420123Author:Daniel L. Reger, Scott R. Goode, David W. Ball, Edward MercerPublisher:Cengage Learning Principles of Modern ChemistryChemistryISBN:9781305079113Author:David W. Oxtoby, H. Pat Gillis, Laurie J. ButlerPublisher:Cengage Learning
Principles of Modern ChemistryChemistryISBN:9781305079113Author:David W. Oxtoby, H. Pat Gillis, Laurie J. ButlerPublisher:Cengage Learning Physical ChemistryChemistryISBN:9781133958437Author:Ball, David W. (david Warren), BAER, TomasPublisher:Wadsworth Cengage Learning,
Physical ChemistryChemistryISBN:9781133958437Author:Ball, David W. (david Warren), BAER, TomasPublisher:Wadsworth Cengage Learning, ChemistryChemistryISBN:9781305957404Author:Steven S. Zumdahl, Susan A. Zumdahl, Donald J. DeCostePublisher:Cengage Learning
ChemistryChemistryISBN:9781305957404Author:Steven S. Zumdahl, Susan A. Zumdahl, Donald J. DeCostePublisher:Cengage Learning Chemistry: An Atoms First ApproachChemistryISBN:9781305079243Author:Steven S. Zumdahl, Susan A. ZumdahlPublisher:Cengage Learning
Chemistry: An Atoms First ApproachChemistryISBN:9781305079243Author:Steven S. Zumdahl, Susan A. ZumdahlPublisher:Cengage Learning





