Chapter 11, Problem 11.182RP

Students are testing their new drone to see if it can safely deliver packages to different departments on campus. Position data can be approximated using the expressions x(t) = −0.0000225t⁴ + 0.003t³ + 0.01t² and $y(t)=300\left[1-\cos \left(\frac{\pi }{40}t\right)\right]$, where x and y are expressed in meters and t is expressed in seconds. Knowing that the take-off and landing altitudes are the same, plot the path of the drone and determine (a) the duration of the flight, (b) its maximum speed in the x direction, (c) its maximum altitude and the horizontal distance traveled during the flight.

To determine

Plot the path of the drone and find the duration (t) of the flight.

Answer to Problem 11.182RP

The duration (t) of the flight is $\underset{\_}{80\sec }$.

Explanation of Solution

Given information:

The x coordinate is defined by the relation as $x\left(t\right)=-0.0000225t^4+0.003t^3+0.01t^2$.

The y coordinate is defined by the relation as $y\left(t\right)=300\left(1-\cos \left(\frac{\pi }{40}t\right)\right)$.

Calculation:

The x coordinate is defined by the relation:

$x\left(t\right)=-0.0000225t^4+0.003t^3+0.01t^2$ (1)

The y coordinate is defined by the relation:

$y\left(t\right)=300\left(1-\cos \left(\frac{\pi }{40}t\right)\right)$ (1)

Calculate the duration (t) of the flight:

Equate equation (2) to zero.

$\begin{array}{l}y\left(t\right)=300\left(1-\cos \left(\frac{\pi }{40}t\right)\right)=0\\ 300\left(1-\cos \left(\frac{\pi }{40}t\right)\right)=0\\ \cos \left(\frac{\pi }{40}t\right)=1\end{array}$

General solution for $\cos \left(\frac{\pi }{40}t\right)=1$,

$\begin{array}{l}\frac{\pi }{40}t=0+2\pi n\\ \frac{\pi }{40}t=2\pi n\\ 40\times \frac{\pi }{40}t=2\pi n\times 40\end{array}$

$\begin{array}{l}\pi t=80\pi n\\ \frac{\pi t}{\pi }=\frac{80\pi n}{\pi }\\ t=80n\\ t=80\sec \end{array}$

Calculate the x coordinated as time (t) 0 sec.

Substitute 0 for t in Equation (1).

$\begin{array}{c}x\left(0\right)=-0.0000225{\left(0\right)}^4+0.003{\left(0\right)}^3+0.01{\left(0\right)}^2\\ =0\end{array}$

Similarly calculate the x coordinate for time interval of $0\sec <80\sec$.

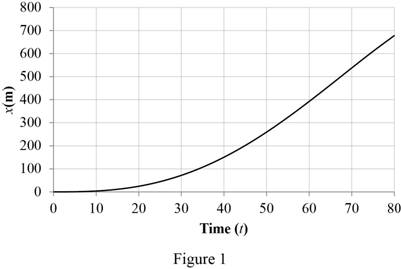
Tabulate the calculated values of x coordinate for time interval $0\sec <80\sec$ as in Table (1).

Time (t)(sec)	x(m)
0	0.00
5	0.61
10	3.78
15	11.24
20	24.40
25	44.34
30	71.78
35	107.11
40	150.40
45	201.36
50	259.38
55	323.49
60	392.40
65	464.49
70	537.78
75	609.96
80	678.40

Plot the graph for time (t) and x coordinate as in Figure (1).

Calculate the y coordinated as time (t) 0 sec.

Substitute 0 for t in equation (1).

$\begin{array}{c}y\left(0\right)=300\left(1-\cos \left(\frac{\pi }{40}\left(0\right)\right)\right)\\ =0\end{array}$

Similarly calculate the y coordinate for time interval of $0\sec <80\sec$.

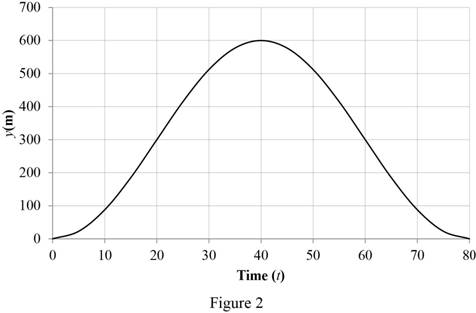
Tabulate the calculated values of y coordinate for time interval $0\sec <80\sec$ as in Table (2).

Time (t)(sec)	y(m)
0	0.00
5	22.84
10	87.87
15	185.19
20	300.00
25	414.81
30	512.13
35	577.16
40	600.00
45	577.16
50	512.13
55	414.81
60	300.00
65	185.19
70	87.87
75	22.84
80	0.00

Plot the graph for time (t) and y coordinate as in Figure (2).

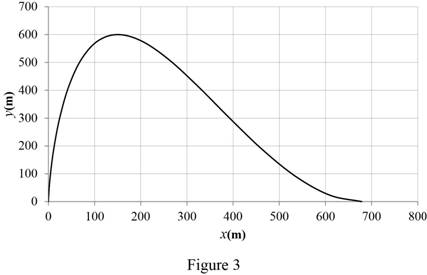
Tabulate the x and y coordinates value as in Table (3):

x(m)	y(m)
0.00	0.00
0.61	22.84
3.78	87.87
11.24	185.19
24.40	300.00
44.34	414.81
71.78	512.13
107.11	577.16
150.40	600.00
201.36	577.16
259.38	512.13
323.49	414.81
392.40	300.00
464.49	185.19
537.78	87.87
609.96	22.84
678.40	0.00

Plot the graph for coordinate x and y as in Figure (3).

Therefore, the duration (t) of the flight is $\underset{\_}{80\sec }$.

To determine

The maximum speed ${\left(v_x\right)}_{\max }$ in the x direction.

Answer to Problem 11.182RP

The maximum speed ${\left(v_x\right)}_{\max }$ in the x direction is $\underset{\_}{14.67\text{m/s}}$.

Explanation of Solution

Given information:

The x coordinate is defined by the relation as $x\left(t\right)=-0.0000225t^4+0.003t^3+0.01t^2$.

The y coordinate is defined by the relation as $y\left(t\right)=300\left(1-\cos \left(\frac{\pi }{40}t\right)\right)$.

Calculation:

Differentiate equation (1) with respective to time (t).

$\begin{array}{l}x\left(t\right)=\left(-0.0000225t^4+0.003t^3+0.01t^2\right)\\ \frac{d\left(x\left(t\right)\right)}{dt}=-0.00009t^3+0.009t^2+0.02t\end{array}$

Since, the rate of change of any coordinate with respect to time is equal to the velocity.

$v_x=-0.00009t^3+0.009t^2+0.02t$ (3)

Differentiate equation (3) with respective to time (t).

$\begin{array}{l}{v}_x=-0.00009t^3+0.009t^2+0.02t\\ \frac{d\left(v_x\right)}{dt}=-0.00027t^2+0.018t+0.02\end{array}$

Since, the rate of change of velocity with respect to time is equal to the acceleration.

$a_x=-0.00027t^2+0.018t+0.02$ (4)

Calculate the time (t) at which the velocity is maximum:

Equate the equation (4) to zero,

$0=-0.00027t^2+0.018t+0.02$

Solve the above quadratic equation for the roots (t),

The roots are -1.093 sec and 67.76 sec.  Reject the negative root.

Calculate the maximum speed ${\left(v_x\right)}_{\max }$ in x direction:

Substitute 67.76 sec for t in equation (3).

$\begin{array}{c}{v}_x=-0.00009{\left(67.76\right)}^3+0.009{\left(67.76\right)}^2+0.02\left(67.76\right)\\ =-28+41.323+1.355\\ =14.67\text{m/s}\end{array}$

Therefore, the maximum speed ${\left(v_x\right)}_{\max }$ in the x direction is $\underset{\_}{14.67\text{m/s}}$.

To determine

The maximum altitude $\left(y_{\max }\right)$ and the horizontal $\left(x_{\max }\right)$ distance travelled during the flight.

Answer to Problem 11.182RP

The maximum altitude $\left(y_{\max }\right)$ and the horizontal $\left(x_{\max }\right)$ distance travelled during the flight $\underset{\_}{600\text{m}}$ and $\underset{\_}{678\text{m}}$.

Explanation of Solution

Given information:

The x coordinate is defined by the relation as $x\left(t\right)=-0.0000225t^4+0.003t^3+0.01t^2$.

The y coordinate is defined by the relation as $y\left(t\right)=300\left(1-\cos \left(\frac{\pi }{40}t\right)\right)$.

Calculation:

Calculate the maximum altitude $\left(y_{\max }\right)$:

Refer Figure 2, the maximum altitude 600m at time 40 sec.

Substitute 40 sec in equation (2).

$\begin{array}{c}y\left(40\right)=300\left(1-\cos \left(\frac{\pi }{40}\left(40\right)\right)\right)\\ =300\left(1-\left(-1\right)\right)\\ =600\text{m}\end{array}$

Calculate the horizontal $\left(x_{\max }\right)$ distance travelled during the flight:

Substitute 80 sec for t in equation (1).

$\begin{array}{c}x\left(80\right)=-0.0000225{\left(80\right)}^4+0.003{\left(80\right)}^3+0.01{\left(80\right)}^2\\ =-921.6+1536+64\\ =678.4\text{m}\end{array}$

Therefore, the maximum altitude $\left(y_{\max }\right)$ and the horizontal $\left(x_{\max }\right)$ distance travelled during the flight $\underset{\_}{600\text{m}}$ and $\underset{\_}{678\text{m}}$.