McDougal Littell Jurgensen Geometry: Student Edition Geometry
McDougal Littell Jurgensen Geometry: Student Edition Geometry
5th Edition
ISBN: 9780395977279
Author: Ray C. Jurgensen, Richard G. Brown, John W. Jurgensen
Publisher: Houghton Mifflin Company College Division
bartleby

Concept explainers

Angles in Circles
Angles within a circle are feasible to create with the help of different properties of the circle such as radii, tangents, and chords. The radius is the distance from the center of the circle to the circumference of the circle. A tangent is a line made p…
Arcs in Circles
A circular arc is the arc of a circle formed by two distinct points. It is a section or segment of the circumference of a circle. A straight line passing through the center connecting the two distinct ends of the arc is termed a semi-circular arc.
bartleby

Videos

Question
Book Icon
Chapter 10.4, Problem 4WE
To determine

To draw: a right angled triangle and then a circumscribed circle.

Expert Solution & Answer
Check Mark

Answer to Problem 4WE

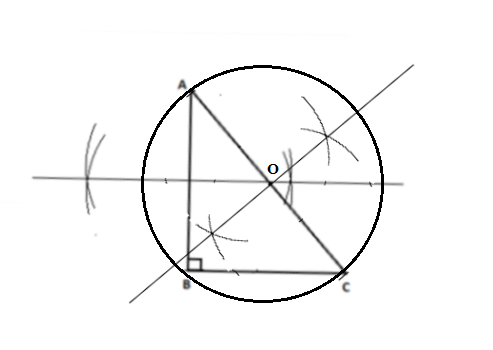
  McDougal Littell Jurgensen Geometry: Student Edition Geometry, Chapter 10.4, Problem 4WE , additional homework tip 1

Explanation of Solution

Given:

A right-angled triangle.

A circumscribed circle is a circle which passes through all the vertices of a given curve.

In this case a circumscribed circle will be a circle which passes through all the vertices of a right angled triangle.

First we draw a right angled triangle.

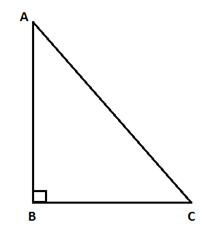
  McDougal Littell Jurgensen Geometry: Student Edition Geometry, Chapter 10.4, Problem 4WE , additional homework tip 2

ABC is a right angled triangle with right angle at B .

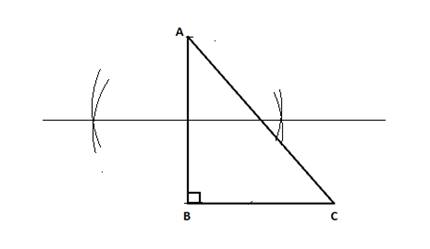
Take any side let’s say AB , draw perpendicular bisector of this line.

Now take an adjacent side of AB, let’s say AC, draw the perpendicular bisector of AC

  McDougal Littell Jurgensen Geometry: Student Edition Geometry, Chapter 10.4, Problem 4WE , additional homework tip 3

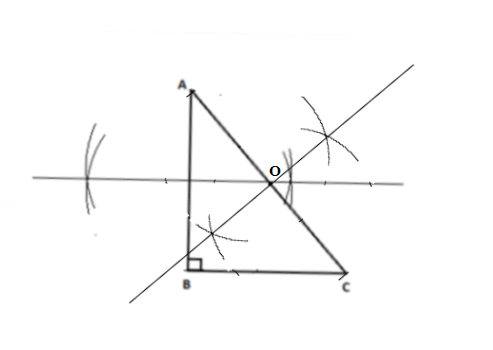
Name the point of intersection of the two bisectors as O.

Now draw a circle by taking O as center and passing through all three vertices A, B and C.

  McDougal Littell Jurgensen Geometry: Student Edition Geometry, Chapter 10.4, Problem 4WE , additional homework tip 4

This is the required figure.

Previous
Chapter 10.4, Problem 3WE
Next
Chapter 10.4, Problem 5WE

Chapter 10 Solutions

McDougal Littell Jurgensen Geometry: Student Edition Geometry

Ch. 10.1 - Prob. 1CECh. 10.1 - Prob. 2CECh. 10.1 - Prob. 3CECh. 10.1 - Prob. 4CECh. 10.1 - Prob. 5CECh. 10.1 - Prob. 6CECh. 10.1 - Prob. 1WECh. 10.1 - Prob. 2WECh. 10.1 - Prob. 3WECh. 10.1 - Prob. 4WE
Ch. 10.1 - Prob. 5WECh. 10.1 - Prob. 6WECh. 10.1 - Prob. 7WECh. 10.1 - Prob. 8WECh. 10.1 - Prob. 9WECh. 10.1 - Prob. 10WECh. 10.1 - Prob. 11WECh. 10.1 - Prob. 12WECh. 10.1 - Prob. 13WECh. 10.1 - Prob. 14WECh. 10.1 - Prob. 15WECh. 10.1 - Prob. 16WECh. 10.1 - Prob. 17WECh. 10.1 - Prob. 18WECh. 10.1 - Prob. 19WECh. 10.1 - Prob. 20WECh. 10.1 - Prob. 21WECh. 10.1 - Prob. 22WECh. 10.1 - Prob. 23WECh. 10.1 - Prob. 24WECh. 10.1 - Prob. 25WECh. 10.1 - Prob. 26WECh. 10.1 - Prob. 1MRECh. 10.1 - Prob. 2MRECh. 10.1 - Prob. 3MRECh. 10.1 - Prob. 4MRECh. 10.1 - Prob. 5MRECh. 10.1 - Prob. 6MRECh. 10.2 - Prob. 1CECh. 10.2 - Prob. 2CECh. 10.2 - Prob. 3CECh. 10.2 - Prob. 4CECh. 10.2 - Prob. 5CECh. 10.2 - Prob. 6CECh. 10.2 - Prob. 7CECh. 10.2 - Prob. 8CECh. 10.2 - Prob. 9CECh. 10.2 - Prob. 10CECh. 10.2 - Prob. 11CECh. 10.2 - Prob. 12CECh. 10.2 - Prob. 13CECh. 10.2 - Prob. 1WECh. 10.2 - Prob. 2WECh. 10.2 - Prob. 3WECh. 10.2 - Prob. 4WECh. 10.2 - Prob. 5WECh. 10.2 - Prob. 6WECh. 10.2 - Prob. 7WECh. 10.2 - Prob. 8WECh. 10.2 - Prob. 9WECh. 10.2 - Prob. 10WECh. 10.2 - Prob. 11WECh. 10.2 - Prob. 12WECh. 10.2 - Prob. 13WECh. 10.2 - Prob. 14WECh. 10.2 - Prob. 15WECh. 10.2 - Prob. 16WECh. 10.2 - Prob. 17WECh. 10.2 - Prob. 18WECh. 10.2 - Prob. 19WECh. 10.2 - Prob. 20WECh. 10.2 - Prob. 21WECh. 10.2 - Prob. 22WECh. 10.2 - Prob. 23WECh. 10.2 - Prob. 24WECh. 10.2 - Prob. 25WECh. 10.2 - Prob. 26WECh. 10.2 - Prob. 27WECh. 10.2 - Prob. 28WECh. 10.2 - Prob. 1EXCh. 10.2 - Prob. 2EXCh. 10.2 - Prob. 3EXCh. 10.3 - Prob. 1CECh. 10.3 - Prob. 2CECh. 10.3 - Prob. 3CECh. 10.3 - Prob. 4CECh. 10.3 - Prob. 5CECh. 10.3 - Prob. 6CECh. 10.3 - Prob. 1WECh. 10.3 - Prob. 2WECh. 10.3 - Prob. 3WECh. 10.3 - Prob. 4WECh. 10.3 - Prob. 5WECh. 10.3 - Prob. 6WECh. 10.3 - Prob. 7WECh. 10.3 - Prob. 8WECh. 10.3 - Prob. 9WECh. 10.3 - Prob. 10WECh. 10.3 - Prob. 11WECh. 10.3 - Prob. 12WECh. 10.3 - Prob. 13WECh. 10.3 - Prob. 14WECh. 10.3 - Prob. 15WECh. 10.3 - Prob. 16WECh. 10.3 - Prob. 17WECh. 10.3 - Prob. 1ST1Ch. 10.3 - Prob. 2ST1Ch. 10.3 - Prob. 3ST1Ch. 10.3 - Prob. 4ST1Ch. 10.3 - Prob. 5ST1Ch. 10.3 - Prob. 6ST1Ch. 10.3 - Prob. 7ST1Ch. 10.3 - Prob. 8ST1Ch. 10.3 - Prob. 1ECh. 10.3 - Prob. 2ECh. 10.3 - Prob. 3ECh. 10.3 - Prob. 1MRECh. 10.3 - Prob. 2MRECh. 10.3 - Prob. 3MRECh. 10.3 - Prob. 1EXCh. 10.3 - Prob. 2EXCh. 10.4 - Prob. 1CECh. 10.4 - Prob. 2CECh. 10.4 - Prob. 3CECh. 10.4 - Prob. 4CECh. 10.4 - Prob. 5CECh. 10.4 - Prob. 6CECh. 10.4 - Prob. 7CECh. 10.4 - Prob. 1WECh. 10.4 - Prob. 2WECh. 10.4 - Prob. 3WECh. 10.4 - Prob. 4WECh. 10.4 - Prob. 5WECh. 10.4 - Prob. 6WECh. 10.4 - Prob. 7WECh. 10.4 - Prob. 8WECh. 10.4 - Prob. 9WECh. 10.4 - Prob. 10WECh. 10.4 - Prob. 11WECh. 10.4 - Prob. 12WECh. 10.4 - Prob. 13WECh. 10.4 - Prob. 14WECh. 10.4 - Prob. 15WECh. 10.4 - Prob. 16WECh. 10.4 - Prob. 17WECh. 10.4 - Prob. 18WECh. 10.4 - Prob. 19WECh. 10.4 - Prob. 20WECh. 10.4 - Prob. 21WECh. 10.5 - Prob. 1CECh. 10.5 - Prob. 2CECh. 10.5 - Prob. 3CECh. 10.5 - Prob. 4CECh. 10.5 - Prob. 5CECh. 10.5 - Prob. 6CECh. 10.5 - Prob. 7CECh. 10.5 - Prob. 8CECh. 10.5 - Prob. 9CECh. 10.5 - Prob. 10CECh. 10.5 - Prob. 11CECh. 10.5 - Prob. 1WECh. 10.5 - Prob. 2WECh. 10.5 - Prob. 3WECh. 10.5 - Prob. 4WECh. 10.5 - Prob. 5WECh. 10.5 - Prob. 6WECh. 10.5 - Prob. 7WECh. 10.5 - Prob. 8WECh. 10.5 - Prob. 9WECh. 10.5 - Prob. 10WECh. 10.5 - Prob. 11WECh. 10.5 - Prob. 12WECh. 10.5 - Prob. 13WECh. 10.5 - Prob. 14WECh. 10.5 - Prob. 15WECh. 10.5 - Prob. 16WECh. 10.5 - Prob. 17WECh. 10.5 - Prob. 18WECh. 10.5 - Prob. 1ST2Ch. 10.5 - Prob. 2ST2Ch. 10.5 - Prob. 3ST2Ch. 10.5 - Prob. 4ST2Ch. 10.5 - Prob. 5ST2Ch. 10.5 - Prob. 6ST2Ch. 10.5 - Prob. 7ST2Ch. 10.6 - Prob. 1CECh. 10.6 - Prob. 2CECh. 10.6 - Prob. 3CECh. 10.6 - Prob. 4CECh. 10.6 - Prob. 5CECh. 10.6 - Prob. 6CECh. 10.6 - Prob. 7CECh. 10.6 - Prob. 8CECh. 10.6 - Prob. 9CECh. 10.6 - Prob. 10CECh. 10.6 - Prob. 11CECh. 10.6 - Prob. 12CECh. 10.6 - Prob. 1WECh. 10.6 - Prob. 2WECh. 10.6 - Prob. 3WECh. 10.6 - Prob. 4WECh. 10.6 - Prob. 5WECh. 10.6 - Prob. 6WECh. 10.6 - Prob. 7WECh. 10.6 - Prob. 8WECh. 10.6 - Prob. 9WECh. 10.6 - Prob. 10WECh. 10.6 - Prob. 11WECh. 10.6 - Prob. 12WECh. 10.6 - Prob. 13WECh. 10.6 - Prob. 14WECh. 10.6 - Prob. 15WECh. 10.6 - Prob. 16WECh. 10.6 - Prob. 17WECh. 10.6 - Prob. 18WECh. 10.6 - Prob. 19WECh. 10.6 - Prob. 20WECh. 10.6 - Prob. 21WECh. 10.6 - Prob. 22WECh. 10.6 - Prob. 23WECh. 10.6 - Prob. 24WECh. 10.7 - Prob. 1CECh. 10.7 - Prob. 2CECh. 10.7 - Prob. 3CECh. 10.7 - Prob. 4CECh. 10.7 - Prob. 5CECh. 10.7 - Prob. 6CECh. 10.7 - Prob. 7CECh. 10.7 - Prob. 8CECh. 10.7 - Prob. 9CECh. 10.7 - Prob. 10CECh. 10.7 - Prob. 1WECh. 10.7 - Prob. 2WECh. 10.7 - Prob. 3WECh. 10.7 - Prob. 4WECh. 10.7 - Prob. 5WECh. 10.7 - Prob. 6WECh. 10.7 - Prob. 7WECh. 10.7 - Prob. 8WECh. 10.7 - Prob. 9WECh. 10.7 - Prob. 10WECh. 10.7 - Prob. 11WECh. 10.7 - Prob. 12WECh. 10.7 - Prob. 13WECh. 10.7 - Prob. 14WECh. 10.7 - Prob. 15WECh. 10.7 - Prob. 16WECh. 10.7 - Prob. 17WECh. 10.7 - Prob. 18WECh. 10.7 - Prob. 19WECh. 10.7 - Prob. 20WECh. 10.7 - Prob. 21WECh. 10.7 - Prob. 22WECh. 10.7 - Prob. 23WECh. 10.7 - Prob. 24WECh. 10.8 - Prob. 1CECh. 10.8 - Prob. 2CECh. 10.8 - Prob. 1WECh. 10.8 - Prob. 2WECh. 10.8 - Prob. 3WECh. 10.8 - Prob. 4WECh. 10.8 - Prob. 5WECh. 10.8 - Prob. 6WECh. 10.8 - Prob. 7WECh. 10.8 - Prob. 8WECh. 10.8 - Prob. 9WECh. 10.8 - Prob. 10WECh. 10.8 - Prob. 11WECh. 10.8 - Prob. 12WECh. 10.8 - Prob. 13WECh. 10.8 - Prob. 14WECh. 10.8 - Prob. 15WECh. 10.8 - Prob. 16WECh. 10.8 - Prob. 17WECh. 10.8 - Prob. 18WECh. 10.8 - Prob. 19WECh. 10.8 - Prob. 1ST3Ch. 10.8 - Prob. 2ST3Ch. 10.8 - Prob. 3ST3Ch. 10.8 - Prob. 4ST3Ch. 10.8 - Prob. 5ST3Ch. 10.8 - Prob. 6ST3Ch. 10.8 - Prob. 7ST3Ch. 10.8 - Prob. 1ECh. 10.8 - Prob. 2ECh. 10.8 - Prob. 3ECh. 10.8 - Prob. 4ECh. 10.8 - Prob. 5ECh. 10.8 - Prob. 6ECh. 10 - Prob. 1CRCh. 10 - Prob. 2CRCh. 10 - Prob. 3CRCh. 10 - Prob. 4CRCh. 10 - Prob. 5CRCh. 10 - Prob. 6CRCh. 10 - Prob. 7CRCh. 10 - Prob. 8CRCh. 10 - Prob. 9CRCh. 10 - Prob. 10CRCh. 10 - Prob. 11CRCh. 10 - Prob. 12CRCh. 10 - Prob. 13CRCh. 10 - Prob. 14CRCh. 10 - Prob. 15CRCh. 10 - Prob. 16CRCh. 10 - Prob. 17CRCh. 10 - Prob. 18CRCh. 10 - Prob. 19CRCh. 10 - Prob. 20CRCh. 10 - Prob. 21CRCh. 10 - Prob. 22CRCh. 10 - Prob. 23CRCh. 10 - Prob. 24CRCh. 10 - Prob. 25CRCh. 10 - Prob. 26CRCh. 10 - Prob. 27CRCh. 10 - Prob. 1CTCh. 10 - Prob. 2CTCh. 10 - Prob. 3CTCh. 10 - Prob. 4CTCh. 10 - Prob. 5CTCh. 10 - Prob. 6CTCh. 10 - Prob. 7CTCh. 10 - Prob. 8CTCh. 10 - Prob. 9CTCh. 10 - Prob. 10CTCh. 10 - Prob. 11CTCh. 10 - Prob. 12CTCh. 10 - Prob. 1ARCh. 10 - Prob. 2ARCh. 10 - Prob. 3ARCh. 10 - Prob. 4ARCh. 10 - Prob. 5ARCh. 10 - Prob. 6ARCh. 10 - Prob. 7ARCh. 10 - Prob. 8ARCh. 10 - Prob. 9ARCh. 10 - Prob. 10ARCh. 10 - Prob. 11ARCh. 10 - Prob. 12ARCh. 10 - Prob. 13ARCh. 10 - Prob. 14ARCh. 10 - Prob. 15ARCh. 10 - Prob. 16ARCh. 10 - Prob. 17ARCh. 10 - Prob. 18ARCh. 10 - Prob. 19ARCh. 10 - Prob. 20ARCh. 10 - Prob. 21ARCh. 10 - Prob. 22ARCh. 10 - Prob. 23ARCh. 10 - Prob. 24ARCh. 10 - Prob. 25ARCh. 10 - Prob. 26ARCh. 10 - Prob. 27ARCh. 10 - Prob. 28ARCh. 10 - Prob. 29ARCh. 10 - Prob. 30ARCh. 10 - Prob. 31ARCh. 10 - Prob. 32ARCh. 10 - Prob. 1CURCh. 10 - Prob. 2CURCh. 10 - Prob. 3CURCh. 10 - Prob. 4CURCh. 10 - Prob. 5CURCh. 10 - Prob. 6CURCh. 10 - Prob. 7CURCh. 10 - Prob. 8CURCh. 10 - Prob. 9CURCh. 10 - Prob. 10CURCh. 10 - Prob. 11CURCh. 10 - Prob. 12CURCh. 10 - Prob. 13CURCh. 10 - Prob. 14CURCh. 10 - Prob. 15CURCh. 10 - Prob. 16CURCh. 10 - Prob. 17CURCh. 10 - Prob. 18CURCh. 10 - Prob. 19CUR

Additional Math Textbook Solutions

Find more solutions based on key concepts
Derivatives Evaluate the derivatives of the following functions. 13. f(x) = tan1 10x

Calculus: Early Transcendentals (2nd Edition)

The four flaws in the given survey.

Elementary Statistics

Assessment 1-1A The following is a magic square all rows, columns, and diagonals sum to the same number. Find t...

A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)

In Problem 19-26, write the function whose graph is the graph of y= x 3 , but is: Shifted up 4 units.

Precalculus

A retail establishment accepts either the American Express or the VISA credit card. A total of 24 percent of it...

A First Course in Probability (10th Edition)

Fill in each blank so that the resulting statement is true. An equation that expresses a relationship between t...

Algebra and Trigonometry (6th Edition)

Knowledge Booster
Background pattern image
Geometry
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, geometry and related others by exploring similar questions and additional content below.
Recommended textbooks for you
Text book image
Elementary Geometry For College Students, 7e
Geometry
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Cengage,
Text book image
Elementary Geometry for College Students
Geometry
ISBN:9781285195698
Author:Daniel C. Alexander, Geralyn M. Koeberlein
Publisher:Cengage Learning
SEE MORE TEXTBOOKS
Finding the length of an arc; Author: Maths Genie;https://www.youtube.com/watch?v=fWGPf5peCc8;License: Standard YouTube License, CC-BY
Circles, Angle Measures, Arcs, Central & Inscribed Angles, Tangents, Secants & Chords - Geometry; Author: The Organic Chemistry Tutor;https://www.youtube.com/watch?v=nd46bA9DKE0;License: Standard Youtube License