Chapter 10.3, Problem 16WE

To determine

To prove: the triangle is isosceles if two medians of that triangle are congruent.

Answer to Problem 16WE

The triangle is isosceles triangle.

Explanation of Solution

Given:

Two medians of triangle are congruent.

Calculation:

Consider a triangle with two equal medians as shown below:

  

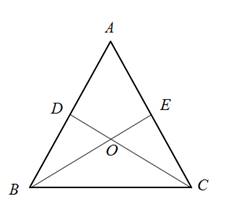
Here, DC and BE are equal. Thus, the point of intersection of medians of a triangle is $\frac{2}{3}$ of the distance from the vertex to the midpoint of opposite side.

Hence,

  $\begin{array}{l}BO=\frac{2}{3}BE\mathrm{......}\left(1\right)\\ CO=\frac{2}{3}CD\mathrm{......}\left(2\right)\end{array}$

In equations (1) and (2), DC and BE are equal. Hence, BO must be equal to CO.

  $BO=CO$

Similarly,

  $DO=OE$

In triangles DOB and EOC,

  $\begin{array}{l}BO=CO\\ DO=OE\\ \angle DOB=\angle EOC\left(\text{Vertical}\text{opposite}\text{angles}\right)\\ \therefore \Delta DOB\cong \Delta EOC\left(SAS\right)\end{array}$

Hence,

  $BD=EC\left(CPCT\right)$

BE and DC are the medians,

Hence,

  $\begin{array}{l}BD=EC\\ \frac{1}{2}AB=\frac{1}{2}AC\\ AB=AC\end{array}$

Hence, the triangle is isosceles triangle.