Calculus Early Transcendentals, Binder Ready Version
11th Edition
ISBN: 9781118883822
Author: Howard Anton, Irl C. Bivens, Stephen Davis
Publisher: WILEY
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 10, Problem 42RE
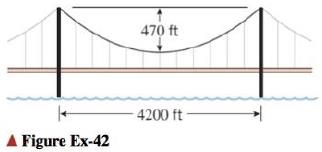
It can be shown in the accompanying figure that hanging cables form parabolic arcs rather than catenaries if they are subjected to uniformly distributed downward forces along their length. For example, if the weight of the roadway in a suspension bridge is assumed to be uniformly distributed along the supporting cables, then the cables can be modeled by parabolas.
(a) Assuming a parabolic model, find an equation for the cable in the accompanying figure, taking the y-axis to be vertical and the origin at the low point of the cable.
(b) Find the length of the cable between the supports.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Use the method of washers to find the volume of the solid that is obtained
when the region between the graphs f(x) = √√2 and g(x) = secx over the
interval ≤x≤ is rotated about the x-axis.
5
Use the method of disks to find the volume of the solid that is obtained
when the region under the curve y = over the interval [4,17] is rotated
about the x-axis.
3. Use the method of washers to find the volume of the solid that is obtained
when the region between the graphs f(x) = √√2 and g(x) = secx over the
interval ≤x≤ is rotated about the x-axis.
Chapter 10 Solutions
Calculus Early Transcendentals, Binder Ready Version
Ch. 10.1 - Prob. 1QCECh. 10.1 - The graph of the curve described by the parametric...Ch. 10.1 - Suppose that a parametric curve C is given by the...Ch. 10.1 - To find dy/dx directly from the parametric...Ch. 10.1 - Let L be the length of the curve x=lnt,y=sint1t An...Ch. 10.1 - (a) By eliminating the parameter, sketch the...Ch. 10.1 - (a) By eliminating the parameter, sketch the...Ch. 10.1 - Sketch the curve by eliminating the parameter, and...Ch. 10.1 - Sketch the curve by eliminating the parameter, and...Ch. 10.1 - Sketch the curve by eliminating the parameter, and...
Ch. 10.1 - Sketch the curve by eliminating the parameter, and...Ch. 10.1 - Prob. 7ESCh. 10.1 - Sketch the curve by eliminating the parameter, and...Ch. 10.1 - Sketch the curve by eliminating the parameter, and...Ch. 10.1 - Sketch the curve by eliminating the parameter, and...Ch. 10.1 - Sketch the curve by eliminating the parameter, and...Ch. 10.1 - Sketch the curve by eliminating the parameter, and...Ch. 10.1 - Prob. 13ESCh. 10.1 - Prob. 14ESCh. 10.1 - Find parametric equations for the curve, and check...Ch. 10.1 - Find parametric equations for the curve, and check...Ch. 10.1 - Evaluation Warning: The document was created with...Ch. 10.1 - Prob. 18ESCh. 10.1 - (a) Use a graphing utility to generate the...Ch. 10.1 - (a) Use a graphing utility to generate the...Ch. 10.1 - Prob. 21ESCh. 10.1 - Graph the equation using a graphing utility....Ch. 10.1 - In each part, match the parametric equation with...Ch. 10.1 - (a) Identify the orientation of the curves in...Ch. 10.1 - (a) Suppose that the line segment from the point...Ch. 10.1 - Find parametric equations for the line segment...Ch. 10.1 - (a) Show that the line segment joining the points...Ch. 10.1 - (a) By eliminating the parameter, show that if a...Ch. 10.1 - Use a graphing utility and parametric equations to...Ch. 10.1 - Use a graphing utility and parametric equations to...Ch. 10.1 - Use a graphing utility and parametric equations to...Ch. 10.1 - Use a graphing utility and parametric equations to...Ch. 10.1 - Determine whether the statement is true or false....Ch. 10.1 - Determine whether the statement is true or false....Ch. 10.1 - Determine whether the statement is true or false....Ch. 10.1 - Prob. 36ESCh. 10.1 - Parametric curves can be defined piecewise by...Ch. 10.1 - Find parametric equations for the rectangle in the...Ch. 10.1 - (a) Find parametric equations for the ellipse that...Ch. 10.1 - We will show later in the text that if a...Ch. 10.1 - (a) Find the slope of the tangent line to the...Ch. 10.1 - (a) Find the slope of the tangent line to the...Ch. 10.1 - For the parametric curve in Exercise 41, make a...Ch. 10.1 - For the parametric curve in Exercise 42, make a...Ch. 10.1 - Find dy/dxandd2y/dx2 at the given point without...Ch. 10.1 - Find dy/dxandd2y/dx2 at the given point without...Ch. 10.1 - Find at the given point without eliminating the...Ch. 10.1 - Find at the given point without eliminating the...Ch. 10.1 - Find dy/dxandd2y/dx2 at the given point without...Ch. 10.1 - Find dy/dxandd2y/dx2 at the given point without...Ch. 10.1 - (a) Find the equation of the tangent line to the...Ch. 10.1 - (a) Find the equation of the tangent line to the...Ch. 10.1 - Find all values of t at which the parametric curve...Ch. 10.1 - Find all values of t at which the parametric curve...Ch. 10.1 - In the and-1850s the French physicist Jules...Ch. 10.1 - The prolate cycloid x=2cost,y=2tsintt crosses...Ch. 10.1 - Show that the curve x=t2,y=t34t intersects itself...Ch. 10.1 - Show that the curve with parametric equations...Ch. 10.1 - Prob. 59ESCh. 10.1 - Verify that the cycloid described by Formula (10)...Ch. 10.1 - (a) What is the slope of the tangent line at time...Ch. 10.1 - Suppose that a bee follows the trajectory...Ch. 10.1 - Consider the family of curves described by the...Ch. 10.1 - (a) Use a graphing utility to study how the curves...Ch. 10.1 - Find the exact arc length of the curve over the...Ch. 10.1 - Find the exact arc length of the curve over the...Ch. 10.1 - Find the exact arc length of the curve over the...Ch. 10.1 - Find the exact arc length of the curve over the...Ch. 10.1 - Find the exact arc length of the curve over the...Ch. 10.1 - Find the exact arc length of the curve over the...Ch. 10.1 - Prob. 71ESCh. 10.1 - Use the parametric equations in Formula (10) to...Ch. 10.1 - Prob. 73ESCh. 10.1 - (a) If a thread is unwound from a fixed circle...Ch. 10.1 - If ftandgt are continuous functions, and if no...Ch. 10.1 - If ftandgt are continuous functions, and if no...Ch. 10.1 - If ftandgt are continuous functions, and if no...Ch. 10.1 - If ftandgt are continuous functions, and if no...Ch. 10.1 - If ftandgt are continuous functions, and if no...Ch. 10.1 - If ftandgt are continuous functions, and if no...Ch. 10.2 - (a) Rectangular coordinates of a point x,y may be...Ch. 10.2 - Find the rectangular coordinates of the points...Ch. 10.2 - Prob. 3QCECh. 10.2 - Prob. 4QCECh. 10.2 - Prob. 1ESCh. 10.2 - Plot the points in polar coordinates....Ch. 10.2 - Find the rectangular coordinates of the points...Ch. 10.2 - Find the rectangular coordinates of the points...Ch. 10.2 - In each part, a point is given in rectangular...Ch. 10.2 - In each part, find polar coordinates satisfying...Ch. 10.2 - Use a calculating utility, where needed, to...Ch. 10.2 - Prob. 8ESCh. 10.2 - Identify the curve by transforming the given polar...Ch. 10.2 - Identify the curve by transforming the given polar...Ch. 10.2 - Express the given equations in polar coordinates....Ch. 10.2 - Express the given equations in polar coordinates....Ch. 10.2 - A graph is given in a rectangular r-coordinate...Ch. 10.2 - A graph is given in a rectangular r-coordinate...Ch. 10.2 - A graph is given in a rectangular r-coordinate...Ch. 10.2 - A graph is given in a rectangular r-coordinate...Ch. 10.2 - Prob. 17ESCh. 10.2 - Prob. 18ESCh. 10.2 - Prob. 19ESCh. 10.2 - Prob. 20ESCh. 10.2 - Prob. 21ESCh. 10.2 - Prob. 22ESCh. 10.2 - Sketch the curve in polar coordinates. r=3Ch. 10.2 - Prob. 24ESCh. 10.2 - Sketch the curve in polar coordinates. r=6sinCh. 10.2 - Sketch the curve in polar coordinates. r2=2cosCh. 10.2 - Prob. 27ESCh. 10.2 - Prob. 28ESCh. 10.2 - Prob. 29ESCh. 10.2 - Sketch the curve in polar coordinates. r=1+2sinCh. 10.2 - Sketch the curve in polar coordinates. r=1cosCh. 10.2 - Sketch the curve in polar coordinates. r=4+3cosCh. 10.2 - Sketch the curve in polar coordinates. r=3sinCh. 10.2 - Sketch the curve in polar coordinates. r=3+4cosCh. 10.2 - Prob. 35ESCh. 10.2 - Prob. 36ESCh. 10.2 - Prob. 37ESCh. 10.2 - Sketch the curve in polar coordinates. r2=cos2Ch. 10.2 - Sketch the curve in polar coordinates. r2=16sin2Ch. 10.2 - Sketch the curve in polar coordinates. r=40Ch. 10.2 - Sketch the curve in polar coordinates. r=40Ch. 10.2 - Prob. 42ESCh. 10.2 - Sketch the curve in polar coordinates. r=2cos2Ch. 10.2 - Sketch the curve in polar coordinates. r=3sin2Ch. 10.2 - Sketch the curve in polar coordinates. r=9sin4Ch. 10.2 - Sketch the curve in polar coordinates. r=2cos3Ch. 10.2 - Determine whether the statement is true or false....Ch. 10.2 - Determine whether the statement is true or false....Ch. 10.2 - Prob. 49ESCh. 10.2 - Prob. 50ESCh. 10.2 - Prob. 51ESCh. 10.2 - Prob. 52ESCh. 10.2 - Prob. 53ESCh. 10.2 - Prob. 54ESCh. 10.2 - Prob. 55ESCh. 10.2 - The accompanying figure shows the graph of the...Ch. 10.2 - The accompanying figure shows the Archimedean...Ch. 10.2 - Find equations for the two families of circles in...Ch. 10.2 - (a) Show that if a varies, then the polar equation...Ch. 10.2 - Prob. 60ESCh. 10.2 - A polar graph of r=f is given over the stated...Ch. 10.2 - A polar graph of r=f is given over the stated...Ch. 10.2 - Use the polar graph from the indicated exercise to...Ch. 10.2 - Use the polar graph from the indicated exercise to...Ch. 10.2 - Prob. 65ESCh. 10.2 - Use the result in Exercise 65 to find an equation...Ch. 10.2 - Prob. 67ESCh. 10.2 - Prob. 68ESCh. 10.2 - Find the highest point on the cardioid r=1+cos.Ch. 10.2 - Find the leftmost point on the upper half of the...Ch. 10.2 - Show that in a polar coordinate system the...Ch. 10.2 - Use the formula obtained in Exercise 71 to find...Ch. 10.2 - Prob. 73ESCh. 10.2 - Prob. 74ESCh. 10.2 - In the late seventeenth century the Italian...Ch. 10.2 - Prob. 76ESCh. 10.2 - Vertical and horizontal asymptotes of polar curves...Ch. 10.2 - Prob. 78ESCh. 10.2 - Prob. 79ESCh. 10.3 - Prob. 1QCECh. 10.3 - Prob. 2QCECh. 10.3 - (a) To find the arc length L of the polar curve...Ch. 10.3 - Prob. 4QCECh. 10.3 - Find the area of the circle r=a by integration.Ch. 10.3 - Find the slope of the tangent line to the polar...Ch. 10.3 - Find the slope of the tangent line to the polar...Ch. 10.3 - Find the slope of the tangent line to the polar...Ch. 10.3 - Find the slope of the tangent line to the polar...Ch. 10.3 - Find the slope of the tangent line to the polar...Ch. 10.3 - Find the slope of the tangent line to the polar...Ch. 10.3 - Calculate the slopes of the tangent lines...Ch. 10.3 - Calculate the slopes of the tangent lines...Ch. 10.3 - Find polar coordinates of all points at which the...Ch. 10.3 - Find polar coordinates of all points at which the...Ch. 10.3 - Use a graphing utility to make a conjecture about...Ch. 10.3 - Use a graphing utility to make a conjecture about...Ch. 10.3 - Prob. 13ESCh. 10.3 - Sketch the polar curve and find polar equations of...Ch. 10.3 - Sketch the polar curve and find polar equations of...Ch. 10.3 - Prob. 16ESCh. 10.3 - Prob. 17ESCh. 10.3 - Sketch the polar curve and find polar equations of...Ch. 10.3 - Use Formula (3) to calculate the arc length of the...Ch. 10.3 - Use Formula (3) to calculate the arc length of the...Ch. 10.3 - Use Formula (3) to calculate the arc length of the...Ch. 10.3 - Use Formula (3) to calculate the arc length of the...Ch. 10.3 - (a) Show that the arc length of one petal of the...Ch. 10.3 - (a) Sketch the spiral r=e/80+. (b) Find an...Ch. 10.3 - Write down, but do not evaluate, an integral for...Ch. 10.3 - Find the area of the shaded region in Exercise...Ch. 10.3 - In each part, find the area of the circle by...Ch. 10.3 - (a) Show that r=2sin+2cos is a circle. (b) Find...Ch. 10.3 - Find the area of the region described. The region...Ch. 10.3 - Find the area of the region described. The region...Ch. 10.3 - Find the area of the region described. The region...Ch. 10.3 - Find the area of the region described. The region...Ch. 10.3 - Find the area of the region described. The region...Ch. 10.3 - Find the area of the region described. The region...Ch. 10.3 - Find the area of the shaded region.Ch. 10.3 - Find the area of the shaded region.Ch. 10.3 - Find the area of the shaded region.Ch. 10.3 - Find the area of the shaded region.Ch. 10.3 - Find the area of the region described. The region...Ch. 10.3 - Find the area of the region described. The region...Ch. 10.3 - Find the area of the region described. The region...Ch. 10.3 - Find the area of the region described. The region...Ch. 10.3 - Find the area of the region described. The region...Ch. 10.3 - Find the area of the region described. The region...Ch. 10.3 - Find the area of the region described. The region...Ch. 10.3 - Find the area of the region described. The region...Ch. 10.3 - Prob. 47ESCh. 10.3 - Determine whether the statement is true or false....Ch. 10.3 - Determine whether the statement is true or false....Ch. 10.3 - Determine whether the statement is true or false....Ch. 10.3 - (a) Find the error: The area that is inside the...Ch. 10.3 - Find the area inside the curve r2=sin2.Ch. 10.3 - Prob. 53ESCh. 10.3 - As illustrated in the accompanying figure, suppose...Ch. 10.3 - (a) Show that the Folium of Descartes x33xy+y3=0...Ch. 10.3 - (a) (Figure 3.1.3a). What is the area that is...Ch. 10.3 - One of the most famous problems in Greek antiquity...Ch. 10.3 - Prob. 58ESCh. 10.3 - Use a graphing utility to generate the graph of...Ch. 10.3 - Use Formula (9) of Section 10.1 to derive the arc...Ch. 10.3 - As illustrated in the accompanying figure, let Pr,...Ch. 10.3 - Use the formula for obtained in Exercise 61. (a)...Ch. 10.3 - Use the formula for obtained in Exercise 61. Show...Ch. 10.3 - (a) In the discussion associated with Exercises...Ch. 10.3 - Sketch the surface, and use the formulas in...Ch. 10.3 - Sketch the surface, and use the formulas in...Ch. 10.3 - Sketch the surface, and use the formulas in...Ch. 10.3 - Sketch the surface, and use the formulas in...Ch. 10.4 - Prob. 1QCECh. 10.4 - (a) The equation of the parabola with focus p,0...Ch. 10.4 - (a) Suppose that an ellipse has semimajor axis a...Ch. 10.4 - (a) Suppose that a hyperbola has semifocal axis a...Ch. 10.4 - Prob. 1ESCh. 10.4 - (a) Find the focus and directrix for each parabola...Ch. 10.4 - Sketch the parabola, and label the focus, vertex,...Ch. 10.4 - Sketch the parabola, and label the focus, vertex,...Ch. 10.4 - Sketch the parabola, and label the focus, vertex,...Ch. 10.4 - Sketch the parabola, and label the focus, vertex,...Ch. 10.4 - Sketch the ellipse, and label the foci, vertices,...Ch. 10.4 - Sketch the ellipse, and label the foci, vertices,...Ch. 10.4 - Sketch the ellipse, and label the foci, vertices,...Ch. 10.4 - Sketch the ellipse, and label the foci, vertices,...Ch. 10.4 - Sketch the hyperbola, and label the vertices,...Ch. 10.4 - Sketch the hyperbola, and label the vertices,...Ch. 10.4 - Sketch the hyperbola, and label the vertices,...Ch. 10.4 - Sketch the hyperbola, and label the vertices,...Ch. 10.4 - Find an equation for the parabola that satisfies...Ch. 10.4 - Find an equation for the parabola that satisfies...Ch. 10.4 - Find an equation for the parabola that satisfies...Ch. 10.4 - Find an equation for the parabola that satisfies...Ch. 10.4 - Find an equation for the ellipse that satisfies...Ch. 10.4 - Find an equation for the ellipse that satisfies...Ch. 10.4 - Find an equation for the ellipse that satisfies...Ch. 10.4 - Find an equation for the ellipse that satisfies...Ch. 10.4 - Find an equation for a hyperbola that satisfies...Ch. 10.4 - Find an equation for a hyperbola that satisfies...Ch. 10.4 - Find an equation for a hyperbola that satisfies...Ch. 10.4 - Find an equation for a hyperbola that satisfies...Ch. 10.4 - Determine whether the statement is true or false....Ch. 10.4 - Determine whether the statement is true or false....Ch. 10.4 - Determine whether the statement is true or false....Ch. 10.4 - Determine whether the statement is true or false....Ch. 10.4 - (a) As illustrated in the accompanying figure, a...Ch. 10.4 - (a) Find an equation for the parabolic arch with...Ch. 10.4 - Show that the vertex is the closest point on a...Ch. 10.4 - As illustrated in the accompanying figure on the...Ch. 10.4 - For the parabolic reflector in the accompanying...Ch. 10.4 - (a) Show that the right and left branches of the...Ch. 10.4 - (a) Show that the right and left branches of the...Ch. 10.4 - An ant is walking on the is such a way that its...Ch. 10.4 - Suppose that the thumbtacks in Figure 10.4.3(b)...Ch. 10.4 - Find the equation of the hyperbola traced by a...Ch. 10.4 - Prob. 41ESCh. 10.4 - Suppose that the base of a solid is elliptical...Ch. 10.4 - Show that an ellipse with semimajor axis a and...Ch. 10.4 - Show that if a plane is not parallel to the axis...Ch. 10.4 - As illustrated in the accompanying figure, a...Ch. 10.4 - As illustrated in the accompanying figure, suppose...Ch. 10.4 - As illustrated in the accompanying figure, suppose...Ch. 10.4 - A nuclear cooling tower is to have a height of h...Ch. 10.4 - Let R be the region that is above the x-axis and...Ch. 10.4 - As illustrated in the accompanying figure, the...Ch. 10.4 - Prove: The line tangent to the parabola x2=4py at...Ch. 10.4 - Prove: The line tangent to the ellipse
at the...Ch. 10.4 - Prove: The line tangent to the hyperbola...Ch. 10.4 - Use the results in Exercises 52 and 53 to show...Ch. 10.4 - Find two values of k such that the line x+2y=k is...Ch. 10.4 - Find the coordinates of all points on the...Ch. 10.4 - A line tangent to the hyperbola 4x2y2=36...Ch. 10.4 - Consider the second-degree equation where A and C...Ch. 10.4 - In each part, use the result in Exercise 58 to...Ch. 10.4 - Derive the equation x2=4py. in Figure 10.4.6.Ch. 10.4 - Derive the equation given in Figure 10.4.14.
Ch. 10.4 - Derive the equation given in Figure 10.4.22.
Ch. 10.4 - Prove Theorem 10.4.4. [Hint: Choose coordinate...Ch. 10.4 - Given two intersecting lines, let be the line...Ch. 10.5 - Prob. 1QCECh. 10.5 - Prob. 2QCECh. 10.5 - In each part, determine a rotation angle that...Ch. 10.5 - Express 2x2+xy+2y2=1 in the xy-coordinate system...Ch. 10.5 - Let an xy-coordinate system be obtained by...Ch. 10.5 - Let an xy-coordinate system be obtained by...Ch. 10.5 - Rotate the coordinate axes to remove the xy-term....Ch. 10.5 - Rotate the coordinate axes to remove the xy-term....Ch. 10.5 - Rotate the coordinate axes to remove the xy-term....Ch. 10.5 - Rotate the coordinate axes to remove the xy-term....Ch. 10.5 - Rotate the coordinate axes to remove the xy-term....Ch. 10.5 - Rotate the coordinate axes to remove the xy-term....Ch. 10.5 - Rotate the coordinate axes to remove the xy-term....Ch. 10.5 - Rotate the coordinate axes to remove the xy-term....Ch. 10.5 - Rotate the coordinate axes to remove the xy-term....Ch. 10.5 - Rotate the coordinate axes to remove the xy-term....Ch. 10.5 - Let an xy-coordinate system be obtained by...Ch. 10.5 - Let an xy-coordinate system be obtained by...Ch. 10.5 - Let an xy-coordinate system be obtained by...Ch. 10.5 - Prob. 16ESCh. 10.5 - Let an xy-coordinate system be obtained by...Ch. 10.5 - Let an xy-coordinate system be obtained by...Ch. 10.5 - Prob. 19ESCh. 10.5 - Show that the graph of the given equation is a...Ch. 10.5 - Show that the graph of the given equation is a...Ch. 10.5 - Show that the graph of the given equation is a...Ch. 10.5 - Show that the graph of the given equation is an...Ch. 10.5 - Show that the graph of the given equation is an...Ch. 10.5 - Show that the graph of the given equation is an...Ch. 10.5 - Show that the graph of the given equation is an...Ch. 10.5 - Show that the graph of the given equation is a...Ch. 10.5 - Show that the graph of the given equation is a...Ch. 10.5 - Show that the graph of the given equation is a...Ch. 10.5 - Show that the graph of the given equation is a...Ch. 10.5 - Show that the graph of the equation x+y=1 is a...Ch. 10.5 - Derive the expression for Bin9.Ch. 10.5 - Use (9) to prove that B24AC=B24AC for all values...Ch. 10.5 - Use (9) to prove that A+C=A+C for all values of .Ch. 10.5 - Prob. 35ESCh. 10.5 - Prove: If B0, then the graph of x2+Bxy+F=0 is a...Ch. 10.6 - Prob. 1QCECh. 10.6 - Prob. 2QCECh. 10.6 - If the distance from a vertex of an ellipse to the...Ch. 10.6 - If the distance from a vertex of a hyperbola to...Ch. 10.6 - Find the eccentricity and the distance from the...Ch. 10.6 - Find the eccentricity and the distance from the...Ch. 10.6 - Prob. 3ESCh. 10.6 - Prob. 4ESCh. 10.6 - Prob. 5ESCh. 10.6 - Prob. 6ESCh. 10.6 - Prob. 7ESCh. 10.6 - Prob. 8ESCh. 10.6 - Find the distance from the pole to the vertices,...Ch. 10.6 - Find the distance from the pole to the vertices,...Ch. 10.6 - Prob. 11ESCh. 10.6 - Prob. 12ESCh. 10.6 - Prob. 13ESCh. 10.6 - Prove that a hyperbola is an equilateral hyperbola...Ch. 10.6 - (a) Show that the coordinates of the point P on...Ch. 10.6 - (a) Show that the eccentricity of an ellipse can...Ch. 10.6 - (a) Show that the eccentricity of a hyperbola can...Ch. 10.6 - Prob. 18ESCh. 10.6 - Determine whether the statement is true or false....Ch. 10.6 - Determine whether the statement is true or false....Ch. 10.6 - Determine whether the statement is true or false....Ch. 10.6 - Prob. 22ESCh. 10.6 - Use the following values, where needed:...Ch. 10.6 - Use the following values, where needed:...Ch. 10.6 - Use the following values, where needed:...Ch. 10.6 - Use the following values, where needed:...Ch. 10.6 - Use the following values, where needed:...Ch. 10.6 - Use the following values, where needed:...Ch. 10 - Prob. 1RECh. 10 - (a) Suppose that the equations x=ft,y=gt describe...Ch. 10 - (a) Find the slope of the tangent line to the...Ch. 10 - Find dy/dx and d2y/dx2att=2 for the parametric...Ch. 10 - Find all values of t at which a tangent line to...Ch. 10 - Find the exact arc length of the curve...Ch. 10 - In each part, find the rectangular coordinates of...Ch. 10 - Prob. 8RECh. 10 - In each part, use a calculating utility to...Ch. 10 - Prob. 10RECh. 10 - In each part, identify the curve by converting the...Ch. 10 - In each part, express the given equation in polar...Ch. 10 - Prob. 13RECh. 10 - Prob. 14RECh. 10 - Prob. 15RECh. 10 - Sketch the curve in polar coordinates. r2=sin2Ch. 10 - Sketch the curve in polar coordinates. r=3cosCh. 10 - Prob. 18RECh. 10 - (a) Find the minimum and maximum x-coordinates of...Ch. 10 - Determine the slope of the tangent line to the...Ch. 10 - A parametric curve of the form...Ch. 10 - (a) Find the arc length of the polar curve...Ch. 10 - Find the area of the region that is enclosed by...Ch. 10 - Find the area of the region in the first quadrant...Ch. 10 - Find the area of the region that is common to the...Ch. 10 - Find the area of the region that is inside the...Ch. 10 - Sketch the parabola, and label the focus, vertex,...Ch. 10 - Sketch the parabola, and label the focus, vertex,...Ch. 10 - Sketch the parabola, and label the focus, vertex,...Ch. 10 - Sketch the parabola, and label the focus, vertex,...Ch. 10 - Sketch the ellipse, and label the foci, the...Ch. 10 - Sketch the ellipse, and label the foci, the...Ch. 10 - Sketch the ellipse, and label the foci, the...Ch. 10 - Sketch the ellipse, and label the foci, the...Ch. 10 - Sketch the hyperbola, and label the vertices,...Ch. 10 - Sketch the hyperbola, and label the vertices,...Ch. 10 - Sketch the hyperbola, and label the vertices,...Ch. 10 - Prob. 38RECh. 10 - Find an equation for the conic described. A...Ch. 10 - Prob. 40RECh. 10 - Find an equation for the conic described. A...Ch. 10 - It can be shown in the accompanying figure that...Ch. 10 - Prob. 43RECh. 10 - Mickey Mantle is recognized as baseball's...Ch. 10 - Prob. 45RECh. 10 - Prob. 46RECh. 10 - Rotate the coordinate axes to remove the xy-term,...Ch. 10 - Rotate the coordinate axes to show that the graph...Ch. 10 - Prob. 49RECh. 10 - Prob. 50RECh. 10 - Prob. 51RECh. 10 - Use the parametric equations x=acost,y=bsint to...Ch. 10 - Use Simpson's rule or the numerical integration...Ch. 10 - (a) Calculate the eccentricity of the Earth's...
Additional Math Textbook Solutions
Find more solutions based on key concepts
Evaluating limits Evaluate the following limits. 31. limx35x4x3
Calculus: Early Transcendentals (2nd Edition)
In Exercises 13–16, find the margin of error for the values of c, ?, and n.
16. e = 0.975, ? = 4.6, n = 100
Elementary Statistics: Picturing the World (7th Edition)
Final Conclusions. In Exercises 25–28, use a significance level of ? = 0.05 and use the given information for t...
Elementary Statistics (13th Edition)
Sampling Method. In Exercises 9-12, determine whether the sampling method appears to be sound or is flawed.
9. ...
Elementary Statistics
Show that the mean, variance, and mgf of the uniform distribution are as given in this section. Also verify tha...
Probability And Statistical Inference (10th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.Similar questions
- 4. Use cylindrical shells to find the volume of the solid generated when the region enclosed by the given curves is revolved about the x-axis. y = √√x, y = 0, y = √√3arrow_forward5 4 3 21 N -5-4-3-2 -1 -2 -3 -4 1 2 3 4 5 -5+ Write an equation for the function graphed above y =arrow_forward6 5 4 3 2 1 -5 -4-3-2-1 1 5 6 -1 23 -2 -3 -4 -5 The graph above is a transformation of the function f(x) = |x| Write an equation for the function graphed above g(x) =arrow_forward
- The graph of y x² is shown on the grid. Graph y = = (x+3)² – 1. +10+ 69 8 7 5 4 9 432 6. 7 8 9 10 1 10 -9 -8 -7 -6 -5 -4 -3 -2 -1 1 2 3 4 5 -2 -3 -4 -5 -6- Clear All Draw:arrow_forwardSketch a graph of f(x) = 2(x − 2)² − 3 4 3 2 1 5 ས་ -5 -4 -3 -2 -1 1 2 3 4 -1 -2 -3 -4 -5+ Clear All Draw:arrow_forward5. Find the arc length of the curve y = 3x³/2 from x = 0 to x = 4.arrow_forward
- -6 -5 * 10 8 6 4 2 -2 -1 -2 1 2 3 4 5 6 -6 -8 -10- The function graphed above is: Concave up on the interval(s) Concave down on the interval(s) There is an inflection point at:arrow_forward6 5 4 3 2 1 -6 -5 -3 -2 3 -1 -2 -3 -4 -5 The graph above is a transformation of the function x² Write an equation for the function graphed above g(x) =arrow_forward6 5 4 3 2 1 -1 -1 -2 -3 -4 A -5 -6- The graph above shows the function f(x). The graph below shows g(x). 6 5 4 3 2 1 3 -1 -2 -3 -4 -5 -6 | g(x) is a transformation of f(x) where g(x) = Af(Bx) where: A = B =arrow_forward
- 5+ 4 3 2 1. -B -2 -1 1 4 5 -1 -2 -3 -4 -5 Complete an equation for the function graphed above y =arrow_forward60 फं + 2 T 2 -2 -3 2 4 5 6 The graph above shows the function f(x). The graph below shows g(x). फ 3 -1 -2 2 g(x) is a transformation of f(x) where g(x) = Af(Bx) where: A = B =arrow_forwardLet f(x) = 4√√ If g(x) is the graph of f(x) shifted up 6 units and right 3 units, write a formula for g(x) g(x)=arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Elementary Linear Algebra (MindTap Course List)AlgebraISBN:9781305658004Author:Ron LarsonPublisher:Cengage Learning
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,
Holt Mcdougal Larson Pre-algebra: Student Edition...
Algebra
ISBN:9780547587776
Author:HOLT MCDOUGAL
Publisher:HOLT MCDOUGAL
Elementary Linear Algebra (MindTap Course List)
Algebra
ISBN:9781305658004
Author:Ron Larson
Publisher:Cengage Learning
Area Between The Curve Problem No 1 - Applications Of Definite Integration - Diploma Maths II; Author: Ekeeda;https://www.youtube.com/watch?v=q3ZU0GnGaxA;License: Standard YouTube License, CC-BY