Chapter 10, Problem 2.2CQ

Summary Introduction

To determine: The difference shown by the second set of sample from the first one.

Introduction

Company TT is a division of company DM. It was about to launch a new product. Ms. MY, the production manager, asked her assistant JM to check the capability of the oven. JM collected 18 random samples from each of the 20 pieces. After the analysis, the samples proved to be incapable based on the specification width of 1.44 cm.

Table 1

Sample	Mean	Range
1	45.01	0.85
2	44.99	0.89
3	45.02	0.86
4	45	0.91
5	45.04	0.87
6	44.98	0.9
7	44.91	0.86
8	45.04	0.89
9	45	0.85
10	44.97	0.91
11	45.11	0.84
12	44.96	0.87
13	45	0.86
14	44.92	0.89
15	45.06	0.87
16	44.94	0.86
17	45	0.85
18	45.03	0.88

Quiet disappointed with the end results, the manager was figuring out ways to improve the process and free the capital expenditure of $10,000. A former professor suggested going for more samples with less sample sizes. JM conducted the analysis on 27 samples of 5 observations each and the results are tabulated below:

Table 2

Sample	Mean	Range
1	44.96	0.42
2	44.98	0.39
3	44.96	0.41
4	44.97	0.37
5	45.02	0.39
6	45.03	0.4
7	45.04	0.39
8	45.02	0.42
9	45.08	0.38
10	45.12	0.4
11	45.07	0.41
12	45.02	0.38
13	45.01	0.41
14	44.98	0.4
15	45	0.39
16	44.95	0.41
17	44.94	0.43
18	44.94	0.4
19	44.87	0.38
20	44.95	0.41
21	44.93	0.39
22	44.96	0.41
23	44.99	0.4
24	45	0.44
25	45.03	0.42
26	45.04	0.38
27	45.03	0.4

Answer to Problem 2.2CQ

The second sample reveals the changes in the process more clearly than the first set of data.

Explanation of Solution

Given information:

Table 3

Sample	Mean	Range
1	45.01	0.85
2	44.99	0.89
3	45.02	0.86
4	45	0.91
5	45.04	0.87
6	44.98	0.9
7	44.91	0.86
8	45.04	0.89
9	45	0.85
10	44.97	0.91
11	45.11	0.84
12	44.96	0.87
13	45	0.86
14	44.92	0.89
15	45.06	0.87
16	44.94	0.86
17	45	0.85
18	45.03	0.88

Formula:

Mean Chart:

$\begin{array}{l}\text{UCL}=\overline{\overline{\text{X}}}+{\text{A}}_{\text{2}}\overline{\text{R}}\\ \text{LCL}=\overline{\overline{\text{X}}}-{\text{A}}_{\text{2}}\overline{\text{R}}\end{array}$

Difference shown by the second set of sample from the first one:

Date set:1

Table 4

Sample	Mean	Range
1	45.01	0.85
2	44.99	0.89
3	45.02	0.86
4	45	0.91
5	45.04	0.87
6	44.98	0.9
7	44.91	0.86
8	45.04	0.89
9	45	0.85
10	44.97	0.91
11	45.11	0.84
12	44.96	0.87
13	45	0.86
14	44.92	0.89
15	45.06	0.87
16	44.94	0.86
17	45	0.85
18	45.03	0.88
	45	0.872777778

Excel worksheet:

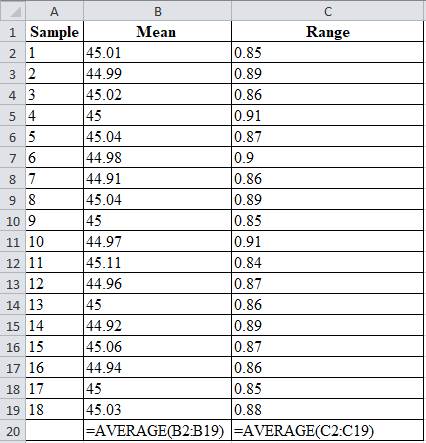
From factors of three-sigma chart, for n=20, A₂ = 0.18; D₃ = 0.41; D₄ = 1.59

Mean control chart:

$\begin{array}{c}\overline{\overline{\text{X}}}=\frac{{\displaystyle \sum _{\text{i}=\text{1}}^{18}\overline{\text{X}}}}{\text{n}}\\ =\frac{809.98}{18}\\ =45\end{array}$

Range control chart:

$\begin{array}{c}\overline{\text{R}}=\frac{{\displaystyle \sum _{i=1}^{18}\text{R}}}{\text{n}}\\ =\frac{15.71}{18}\\ =0.873\end{array}$

Upper control limit:

$\begin{array}{c}\text{UCL}=\overline{\overline{\text{X}}}+{\text{A}}_{\text{2}}\overline{\text{R}}\\ =45+\left(0.18\times 0.873\right)\\ =45.156\end{array}$

The upper control limit is calculated by adding the product of 0.18 and 0.873 with 45, which yields 45.156.

Lower control limit:

$\begin{array}{c}\text{UCL}=\overline{\overline{\text{X}}}-{\text{A}}_{\text{2}}\overline{\text{R}}\\ =45-\left(0.18\times 0.873\right)\\ =44.842\end{array}$

The lower control limit is calculated by subtracting the product of 0.18 and 0.873 with 45, which yields 44.842.

A graph is plotted using the UCL, LCL and samples values.

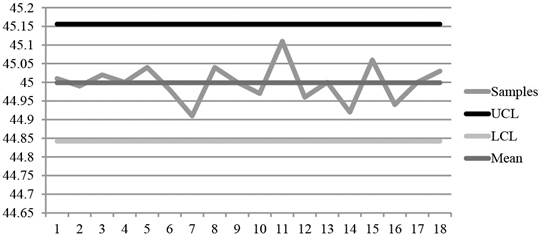
Diagram 1

Date set: 2

Table 5

Sample	Mean	Range
1	44.96	0.42
2	44.98	0.39
3	44.96	0.41
4	44.97	0.37
5	45.02	0.39
6	45.03	0.4
7	45.04	0.39
8	45.02	0.42
9	45.08	0.38
10	45.12	0.4
11	45.07	0.41
12	45.02	0.38
13	45.01	0.41
14	44.98	0.4
15	45	0.39
16	44.95	0.41
17	44.94	0.43
18	44.94	0.4
19	44.87	0.38
20	44.95	0.41
21	44.93	0.39
22	44.96	0.41
23	44.99	0.4
24	45	0.44
25	45.03	0.42
26	45.04	0.38
27	45.03	0.4
	44.9959	0.40111

Excel worksheet:

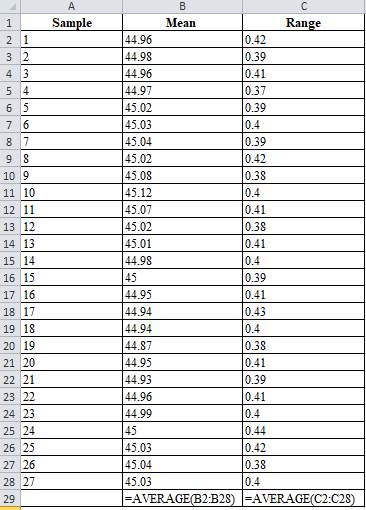
From factors of three-sigma chart, for n=20, A₂ = 0.58

Mean control chart:

$\begin{array}{c}\overline{\overline{\text{X}}}=\frac{{\displaystyle \sum _{\text{i}=\text{1}}^{27}\overline{\text{X}}}}{\text{n}}\\ =\frac{1214.89}{27}\\ =44.99\end{array}$

Range control chart:

$\begin{array}{c}\overline{\text{R}}=\frac{{\displaystyle \sum _{i=1}^{27}\text{R}}}{\text{n}}\\ =\frac{10.83}{27}\\ =0.4011\end{array}$

Upper control limit:

$\begin{array}{c}\text{UCL}=\overline{\overline{\text{X}}}+{\text{A}}_{\text{2}}\overline{\text{R}}\\ =44.99+\left(0.58\times 0.401\right)\\ =45.229\end{array}$

The upper control limit is calculated by adding the product of 0.58 and 0.401 with 44.99, which yields 45.229.

Lower control limit:

$\begin{array}{c}\text{UCL}=\overline{\overline{\text{X}}}{\text{-A}}_{\text{2}}\overline{\text{R}}\\ =44.99-\left(0.58\times 0.401\right)\\ =44.763\end{array}$

The lower control limit is calculated by subtracting the product of 0.58 and 0.401 with 44.99, which yields 44.763.

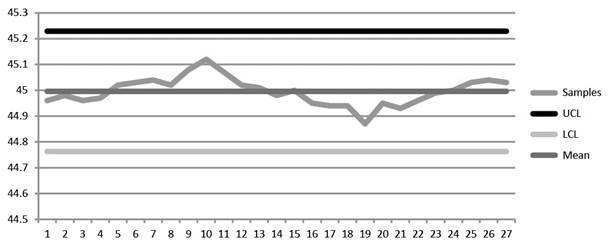
Diagram 2

On comparing Diagrams 1 and 2, it is evident that the second set of data has closer range of changes while the first set of data is scattered and reveals no information about the changes in the process.

Hence, the second sample reveals the changes in the process changes more clearly than the first set of data.