
Concept explainers
Interpretation:
The degeneracies for all energies of a
Concept introduction:
The Schrodinger equation is used to find the allowed energy levels for electronic transitions in the
Where,
•
•
•
The energy obtained after applying the operator on wavefunction is known as the eigen value for the wavefunction.
Answer to Problem 10.81E
The degeneracies for all energies of a
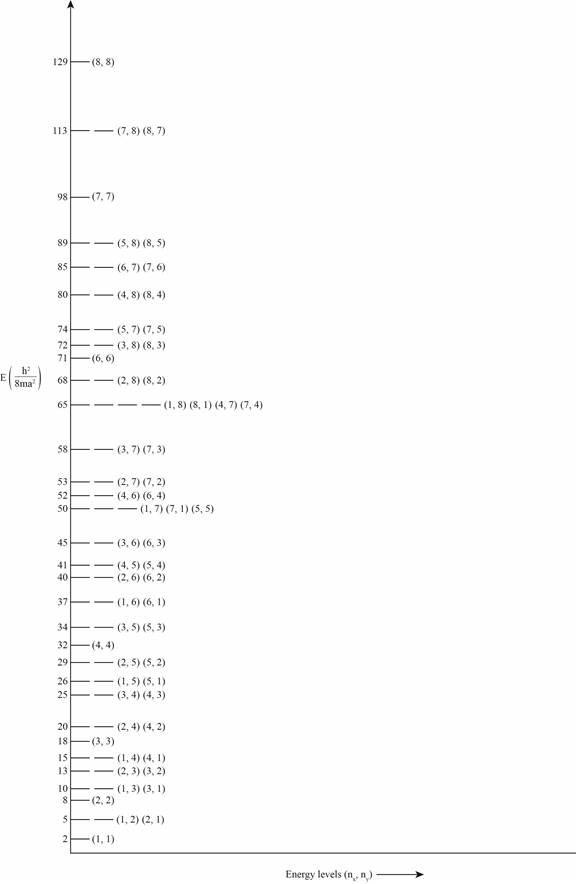
Explanation of Solution
The formula to calculate energy for
Assuming
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energies corresponding to energy levels
Similarly, energy corresponding to energy levels
Similarly, energy corresponding to energy levels
Similarly, energy corresponding to energy levels
Similarly, energy corresponding to energy levels
Similarly, energy corresponding to energy levels
Similarly, energy corresponding to energy levels
The plot of energy versus energy levels is shown in the figure 1.
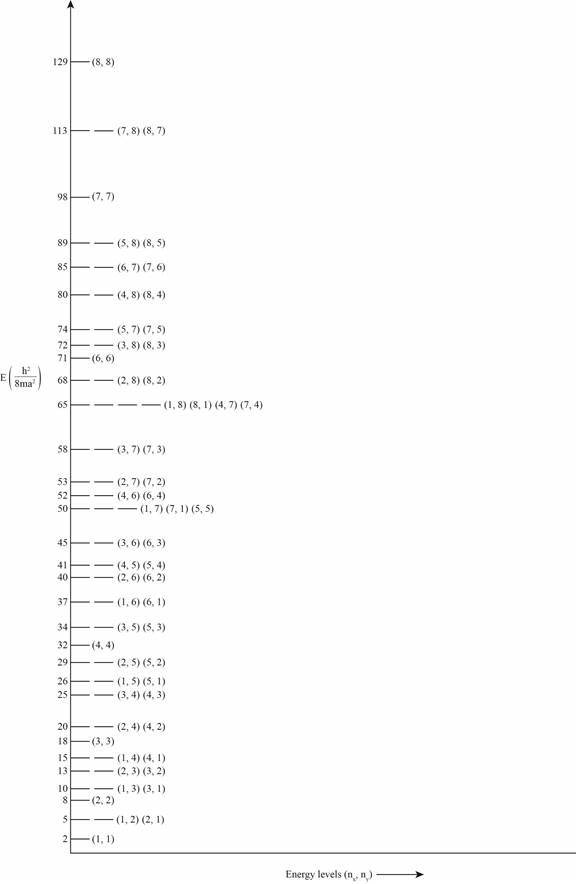
Figure 1
The degeneracies for all energies of a
Want to see more full solutions like this?
Chapter 10 Solutions
Physical Chemistry
- pls helparrow_forwardpls helparrow_forward35) Complete the following equation by drawing the line the structure of the products that are formed. Please note that in some cases more than one product is possible. You must draw all possible products to recive full marks! a. ethanol + 2-propanol + H2SO4 → b. OH conc. H2SO4 CH2 H3C CH + K2Cr2O7 C. d. H3C A pressure CH3 + H2 CH Pt catalystarrow_forward
- 21) The rate of reaction depends upon: a. the concentration and nature of reactants b. the temperature of the reaction C. whether or not a catalyst was used d. all of the above 22) A Maxwell-Boltzmann curve shows the distribution of molecular energies in a reaction system. When the temperature in this system is increased, the peak is a. higher and further to the right. b. higher and further to the left. c. lower and further to the right. d. lower and further to the left. 23) Which of the following correctly describes the reaction represented by the reaction below? CaCO3 (s) + energy → CaO (s) + CO2 (g) a. It is exothermic and the potential energy is greater in the reactants than the products. b. c. It is exothermic and the potential energy is greater in the products than the reactants. It is endothermic and the potential energy is greater in the products than the reactants. d. It is endothermic and the potential energy is equal for the products and reactants.arrow_forwardpls helparrow_forward27) Draw the energy level diagram and write the full and shorthand electron configuration for a neutral sulfur atom.arrow_forward
- Indicate whether these compounds are isomers, enantiomers, or tautomers. OCH OCH محمد ممدarrow_forward30) Substance A to E below are listed with several of their properties. The identities of the substances are identified in random order below: Iron, ethane, ethanol, sodium nitrate, graphite First classify each substance as either a polar covalent compound, non-polar covalent compound, ionic compound, metallic solid, or network solid. Write your predictions in the sixth coloumn of the chart, under "type of substance." Then, identify the identity of the substance in the last coloumn. Substance Melting Point Boiling Point Solubility in H₂O Electrical Conductivity Type of Substance Identity of Substance (°C) (°C) as: Solid, Liquids, Solution A -182 -88 Insoluble No/No/- B 1538 2862 Insoluble Yes/Yes/- C 308 380 Soluble Yes/Yes/Yes Ꭰ 3456 Insoluble No/-/- E -114 78 Soluble No/No/Noarrow_forwardpls helparrow_forward
 Physical ChemistryChemistryISBN:9781133958437Author:Ball, David W. (david Warren), BAER, TomasPublisher:Wadsworth Cengage Learning,
Physical ChemistryChemistryISBN:9781133958437Author:Ball, David W. (david Warren), BAER, TomasPublisher:Wadsworth Cengage Learning, Principles of Modern ChemistryChemistryISBN:9781305079113Author:David W. Oxtoby, H. Pat Gillis, Laurie J. ButlerPublisher:Cengage LearningChemistry: Matter and ChangeChemistryISBN:9780078746376Author:Dinah Zike, Laurel Dingrando, Nicholas Hainen, Cheryl WistromPublisher:Glencoe/McGraw-Hill School Pub Co
Principles of Modern ChemistryChemistryISBN:9781305079113Author:David W. Oxtoby, H. Pat Gillis, Laurie J. ButlerPublisher:Cengage LearningChemistry: Matter and ChangeChemistryISBN:9780078746376Author:Dinah Zike, Laurel Dingrando, Nicholas Hainen, Cheryl WistromPublisher:Glencoe/McGraw-Hill School Pub Co


