College Algebra
10th Edition
ISBN: 9781337282291
Author: Ron Larson
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Question
Chapter 1, Problem 5RE
To determine
The symmetry with respect to both axes and the origin for the equation,
The equation,
Explanation:
Consider the equation,
To check for symmetry about
If the equation after simplifying is equivalent to the original equation, then the given equation will be symmetric about
Replace
Since the new equation is not equivalent to the original equation, the given equation is not symmetric about
To check for symmetry about
If the equation after simplifying is equivalent to the original equation, then the given equation will be symmetric about
Replace
Since the new equation is not equivalent to the original equation, the given equation is not symmetric about
To check for symmetry about the origin, replace
If the equation after simplifying is equivalent to the original equation, then the given equation will be symmetric about the origin.
Replace
Since the new equation is not equivalent to the original equation, the given equation is not symmetric about the origin.
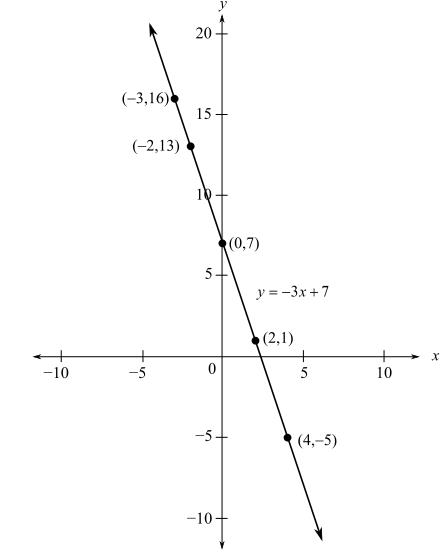
Plot the graph of the equation,
Now plot the points,
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
??!!
rections: For problem
rough 3, read
each question carefully and be sure to
show all work.
1. Determine if
9(4a²-4ab+b²) = (6a-3b)² is a
polynomial identity.
2. Is (2x-y) (8x3+ y³) equivalent to
16x4-y4?
3. Find an expression that is equivalent to
(a - b)³.
Directions: For problems 4 and 5,
algebraically prove that the following
equations are polynomial identities. Show
all of your work and explain each step.
4. (2x+5)² = 4x(x+5)+25
5. (4x+6y)(x-2y)=2(2x²-xy-6y²)
Name:
Mussels & bem
A section of a river currently has a population of 20 zebra mussels. The
population of zebra mussels increases 60 % each month. What will be the
population of zebra mussels after 2 years?
9
10
# of
months
# of
mussels
1
2
3
4
5
6
7
8
o
Graph your data. Remember to title your graph.
What scale should be used on the y-axis?
What scale should be used on the x-axis?
Exponential Growth Equation
y = a(1+r)*
Chapter 1 Solutions
College Algebra
Ch. 1.1 - Determine whether (a)(3,5)and(b)(2,26) lie on the...Ch. 1.1 - Sketch the graph of each equation a.3x+y=2...Ch. 1.1 - Sketch the graph of each equation. a.y=x2+3...Ch. 1.1 - Identify the x- and y-intercepts of the graph of...Ch. 1.1 - Test y2=6x for symmetry with respect to both axes...Ch. 1.1 - Use symmetry to sketch the graph of xy2=1.Ch. 1.1 - Sketch the graph of y=| x2 |.Ch. 1.1 - Prob. 8ECPCh. 1.1 - Prob. 9ECPCh. 1.1 - Fill in the blanks. An ordered pair (a, b) is a ...
Ch. 1.1 - Fill in the blanks. The set of all solution points...Ch. 1.1 - Fill in the blanks. The points at which a graph...Ch. 1.1 - Prob. 4ECh. 1.1 - Prob. 5ECh. 1.1 - Prob. 6ECh. 1.1 - Determining Solution Points In Exercises 7-14,...Ch. 1.1 - Determining Solution Points In Exercises 7-14,...Ch. 1.1 - Determining Solution Points In Exercises 7-14,...Ch. 1.1 - Determining Solution Points In Exercises 7-14,...Ch. 1.1 - Determining Solution Points In Exercises 7-14,...Ch. 1.1 - Determining Solution Points In Exercises 7-14,...Ch. 1.1 - Determining Solution Points In Exercises 7-14,...Ch. 1.1 - Determining Solution Points In Exercises 7-14,...Ch. 1.1 - Sketching the Graph of an Equation In Exercises...Ch. 1.1 - Sketching the Graph of an Equation In Exercises...Ch. 1.1 - Sketching the Graph of an Equation In Exercises...Ch. 1.1 - Sketching the Graph of an Equation In Exercises...Ch. 1.1 - Identifying x- and y-Intercepts In Exercises...Ch. 1.1 - Identifying x- and y-Intercepts In Exercises...Ch. 1.1 - Identifying x- and y-Intercepts In Exercises...Ch. 1.1 - Identifying x- and y-Intercepts In Exercises...Ch. 1.1 - Identifying x- and y-Intercepts In Exercises...Ch. 1.1 - Identifying x- and y-Intercepts In Exercises...Ch. 1.1 - Prob. 25ECh. 1.1 - Prob. 26ECh. 1.1 - Prob. 27ECh. 1.1 - Prob. 28ECh. 1.1 - Prob. 29ECh. 1.1 - Prob. 30ECh. 1.1 - Prob. 31ECh. 1.1 - Prob. 32ECh. 1.1 - Prob. 33ECh. 1.1 - Prob. 34ECh. 1.1 - Prob. 35ECh. 1.1 - Prob. 36ECh. 1.1 - Prob. 37ECh. 1.1 - Prob. 38ECh. 1.1 - Prob. 39ECh. 1.1 - Prob. 40ECh. 1.1 - Prob. 41ECh. 1.1 - Prob. 42ECh. 1.1 - Prob. 43ECh. 1.1 - Prob. 44ECh. 1.1 - Prob. 45ECh. 1.1 - Prob. 46ECh. 1.1 - Prob. 47ECh. 1.1 - Prob. 48ECh. 1.1 - Using Technology In Exercises 49–58, use a...Ch. 1.1 - Prob. 50ECh. 1.1 - Prob. 51ECh. 1.1 - Prob. 52ECh. 1.1 - Prob. 53ECh. 1.1 - Prob. 54ECh. 1.1 - Prob. 55ECh. 1.1 - Prob. 56ECh. 1.1 - Prob. 57ECh. 1.1 - Prob. 58ECh. 1.1 - Prob. 59ECh. 1.1 - Prob. 60ECh. 1.1 - Prob. 61ECh. 1.1 - Prob. 62ECh. 1.1 - Prob. 63ECh. 1.1 - Prob. 64ECh. 1.1 - Prob. 65ECh. 1.1 - Prob. 66ECh. 1.1 - Prob. 67ECh. 1.1 - Prob. 68ECh. 1.1 - Prob. 69ECh. 1.1 - Prob. 70ECh. 1.1 - Prob. 71ECh. 1.1 - Prob. 72ECh. 1.1 - Depreciation A hospital purchases a new magnetic...Ch. 1.1 - Depreciation You purchase an all-terrain vehicle...Ch. 1.1 - Architecture The arch support of a bridge is...Ch. 1.1 - Population Statistics The table shows the life...Ch. 1.1 - Electronics The resistance y (in ohms) of 1000...Ch. 1.1 - Prob. 79ECh. 1.1 - Prob. 80ECh. 1.1 - Prob. 81ECh. 1.1 - Prob. 82ECh. 1.1 - Think About It Find a and b when the graph of...Ch. 1.2 - Solve each equation. a.72x=15 b.7x9=5x+7Ch. 1.2 - Solve 4(x+2)12=5(x6) and check your solution.Ch. 1.2 - Solve 4x913=x+53.Ch. 1.2 - Prob. 4ECPCh. 1.2 - Find the x- and y-intercepts of the graph of each...Ch. 1.2 - The number y (in thousands) of male participants...Ch. 1.2 - Fill in the blanks. An is a statement that...Ch. 1.2 - Fill in the blanks. There are three types of...Ch. 1.2 - Prob. 3ECh. 1.2 - Prob. 4ECh. 1.2 - Fill in the blanks. A equation is an equation...Ch. 1.2 - Prob. 6ECh. 1.2 - Prob. 7ECh. 1.2 - Prob. 8ECh. 1.2 - Classifying an Equation In Exercises 7-14,...Ch. 1.2 - Classifying an Equation In Exercises 7-14,...Ch. 1.2 - Prob. 11ECh. 1.2 - Prob. 12ECh. 1.2 - Prob. 13ECh. 1.2 - Classifying an Equation In Exercises 7-14,...Ch. 1.2 - Solving a Linear Equation In Exercises 15-26,...Ch. 1.2 - Prob. 16ECh. 1.2 - Prob. 17ECh. 1.2 - Prob. 18ECh. 1.2 - Prob. 19ECh. 1.2 - Prob. 20ECh. 1.2 - Prob. 21ECh. 1.2 - Solving a Linear Equation In Exercises 15-26,...Ch. 1.2 - Prob. 23ECh. 1.2 - Prob. 24ECh. 1.2 - Prob. 25ECh. 1.2 - Prob. 26ECh. 1.2 - Prob. 27ECh. 1.2 - Solving a Rational Equation In Exercises 27-44,...Ch. 1.2 - Solving a Rational Equation In Exercises 27-44,...Ch. 1.2 - Solving a Rational Equation In Exercises 27-44,...Ch. 1.2 - Solving a Rational Equation In Exercises 27-44,...Ch. 1.2 - Solving a Rational Equation In Exercises 27-44,...Ch. 1.2 - Solving a Rational Equation In Exercises 27-44,...Ch. 1.2 - Solving a Rational Equation In Exercises 27-44,...Ch. 1.2 - Solving a Rational Equation In Exercises 27-44,...Ch. 1.2 - Prob. 36ECh. 1.2 - Prob. 37ECh. 1.2 - Prob. 38ECh. 1.2 - Solving a Rational Equation In Exercises 27–44,...Ch. 1.2 - Prob. 40ECh. 1.2 - Prob. 41ECh. 1.2 - Prob. 42ECh. 1.2 - Solving a Rational Equation In Exercises 27–44,...Ch. 1.2 - Prob. 44ECh. 1.2 - Prob. 45ECh. 1.2 - Prob. 46ECh. 1.2 - Prob. 47ECh. 1.2 - Prob. 48ECh. 1.2 - Prob. 49ECh. 1.2 - Prob. 50ECh. 1.2 - Prob. 51ECh. 1.2 - Prob. 52ECh. 1.2 - Prob. 53ECh. 1.2 - Prob. 54ECh. 1.2 - Prob. 55ECh. 1.2 - Prob. 56ECh. 1.2 - Prob. 57ECh. 1.2 - Prob. 58ECh. 1.2 - Prob. 59ECh. 1.2 - Prob. 60ECh. 1.2 - Prob. 61ECh. 1.2 - Prob. 62ECh. 1.2 - Prob. 63ECh. 1.2 - Prob. 64ECh. 1.2 - Geometry The surface area S of the circular...Ch. 1.2 - Prob. 66ECh. 1.2 - Prob. 67ECh. 1.2 - Prob. 68ECh. 1.2 - Population The population y (in thousands) of...Ch. 1.2 - Population The population y (in thousands) of...Ch. 1.2 - Operating Cost A delivery company has a fleet of...Ch. 1.2 - Flood Control A river is 8 feet above its flood...Ch. 1.2 - Prob. 73ECh. 1.2 - Prob. 74ECh. 1.2 - Prob. 75ECh. 1.2 - Prob. 76ECh. 1.2 - Prob. 77ECh. 1.2 - Prob. 78ECh. 1.2 - Prob. 79ECh. 1.2 - Prob. 80ECh. 1.2 - Prob. 81ECh. 1.2 - Prob. 82ECh. 1.2 - Prob. 83ECh. 1.2 - Finding Intercepts Consider the linear equation...Ch. 1.3 - You accept a job with an annual income of $58,400...Ch. 1.3 - Prob. 2ECPCh. 1.3 - Your family has annual loan payments equal to 28...Ch. 1.3 - A rectangular family room is 3 times as long as it...Ch. 1.3 - A boat travels at a constant speed to an island 14...Ch. 1.3 - Prob. 6ECPCh. 1.3 - Prob. 7ECPCh. 1.3 - Prob. 8ECPCh. 1.3 - A cylindrical container has a volume of 84 cubic...Ch. 1.3 - Fill in the blanks. The process of translating...Ch. 1.3 - Fill in the blanks. A good approach to...Ch. 1.3 - Writing a Verbal Description In Exercises 3-12,...Ch. 1.3 - Writing a Verbal Description In Exercises 3-12,...Ch. 1.3 - Prob. 5ECh. 1.3 - Prob. 6ECh. 1.3 - Prob. 7ECh. 1.3 - Prob. 8ECh. 1.3 - Prob. 9ECh. 1.3 - Prob. 10ECh. 1.3 - Prob. 11ECh. 1.3 - Prob. 12ECh. 1.3 - Writing an Algebraic Expression In Exercises...Ch. 1.3 - Writing an Algebraic Expression In Exercises...Ch. 1.3 - Prob. 15ECh. 1.3 - Writing an Algebraic Expression In Exercises...Ch. 1.3 - Writing an Algebraic Expression In Exercises...Ch. 1.3 - Writing an Algebraic Expression In Exercises...Ch. 1.3 - Writing an Algebraic Expression In Exercises...Ch. 1.3 - Writing an Algebraic Expression In Exercises...Ch. 1.3 - Writing an Algebraic Expression In Exercises...Ch. 1.3 - Writing an Algebraic Expression In Exercises...Ch. 1.3 - Writing an Algebraic Expression In Exercises...Ch. 1.3 - Writing an Algebraic Expression In Exercises...Ch. 1.3 - Translating a Statement In Exercises 25-28,...Ch. 1.3 - Translating a Statement In Exercises 25-28,...Ch. 1.3 - Translating a Statement In Exercises 25-28,...Ch. 1.3 - Translating a Statement In Exercises 25-28,...Ch. 1.3 - Writing an Expression In Exercises 29 and 30,...Ch. 1.3 - Writing an Expression In Exercises 29 and 30,...Ch. 1.3 - Number Problems In Exercises 31-36, write a...Ch. 1.3 - Number Problems In Exercises 31-36, write a...Ch. 1.3 - Number Problems In Exercises 31-36, write a...Ch. 1.3 - Prob. 34ECh. 1.3 - Number Problems In Exercises 31-36, write a...Ch. 1.3 - Number Problems In Exercises 31-36, write a...Ch. 1.3 - Finance A salesperson’s weekly paycheck is 15 less...Ch. 1.3 - Discount The sale price of a swimming pool after a...Ch. 1.3 - Prob. 39ECh. 1.3 - Finance A family has a monthly mortgage payment of...Ch. 1.3 - Dimensions A rectangular room is 1.5 times as long...Ch. 1.3 - Dimensions A rectangular picture frame has a...Ch. 1.3 - Prob. 43ECh. 1.3 - Course Grade You are taking a course that has four...Ch. 1.3 - Travel Time You are driving on a Canadian freeway...Ch. 1.3 - Average Speed A truck driver travels at an average...Ch. 1.3 - Prob. 47ECh. 1.3 - Radio Waves Radio waves travel at the same speed...Ch. 1.3 - Height of a Building You measure the shadow cast...Ch. 1.3 - Height of a Tree You measure a tree’s shadow and...Ch. 1.3 - Flagpole Height A person who is 6 feet tall walks...Ch. 1.3 - Shadow Length A person who is 6 feet tall walks...Ch. 1.3 - Simple Interest You invested a total of $12,000 at...Ch. 1.3 - Simple Interest You invested a total of $25,000 at...Ch. 1.3 - Inventory A nursery has $40,000 of inventory in...Ch. 1.3 - Inventory An automobile dealer has $600,000 of...Ch. 1.3 - A forester is making a gasoline-oil mixture for a...Ch. 1.3 - Mixture Problem A grocer mixes peanuts that cost...Ch. 1.3 - Prob. 59ECh. 1.3 - Prob. 60ECh. 1.3 - Prob. 61ECh. 1.3 - Prob. 62ECh. 1.3 - Prob. 63ECh. 1.3 - Prob. 64ECh. 1.3 - Prob. 65ECh. 1.3 - Prob. 66ECh. 1.3 - Prob. 67ECh. 1.3 - Chemistry The boiling point of bromine is 58.8C....Ch. 1.3 - Volume of a Billiard Ball A billiard ball has a...Ch. 1.3 - Length of a Tank The diameter of a cylindrical...Ch. 1.3 - Physics You have a uniform beam of length L with a...Ch. 1.3 - HOW DO YOU SEE IT? To determine a building’s...Ch. 1.3 - Prob. 73ECh. 1.3 - Prob. 74ECh. 1.3 - True or False? In Exercises 73-76, determine...Ch. 1.3 - True or False? In Exercises 73-76, determine...Ch. 1.4 - Solve 2x23x+1=6 by factoring.Ch. 1.4 - Solve each equation by extracting square roots. (a...Ch. 1.4 - Prob. 3ECPCh. 1.4 - Prob. 4ECPCh. 1.4 - Prob. 5ECPCh. 1.4 - Prob. 6ECPCh. 1.4 - A rectangular kitchen is 6 feet longer than it is...Ch. 1.4 - You drop a rock from a height of 196 feet. How...Ch. 1.4 - Prob. 9ECPCh. 1.4 - Prob. 10ECPCh. 1.4 - Prob. 1ECh. 1.4 - Prob. 2ECh. 1.4 - Prob. 3ECh. 1.4 - Prob. 4ECh. 1.4 - Fill in the blanks. The general equation that...Ch. 1.4 - Fill in the blanks. An important theorem that is...Ch. 1.4 - Solving a Quadratic Equation by Factoring In...Ch. 1.4 - Solving a Quadratic Equation by Factoring In...Ch. 1.4 - Prob. 9ECh. 1.4 - Solving a Quadratic Equation by Factoring In...Ch. 1.4 - Prob. 11ECh. 1.4 - Solving a Quadratic Equation by Factoring In...Ch. 1.4 - Prob. 13ECh. 1.4 - Prob. 14ECh. 1.4 - Solving a Quadratic Equation by Factoring In...Ch. 1.4 - Prob. 16ECh. 1.4 - Solving a Quadratic Equation by Factoring In...Ch. 1.4 - Prob. 18ECh. 1.4 - Extracting Square Roots In Exercises 19-32, solve...Ch. 1.4 - Extracting Square Roots In Exercises 19-32, solve...Ch. 1.4 - Prob. 21ECh. 1.4 - Prob. 22ECh. 1.4 - Prob. 23ECh. 1.4 - Prob. 24ECh. 1.4 - Prob. 25ECh. 1.4 - Extracting Square Roots In Exercises 19-32, solve...Ch. 1.4 - Prob. 27ECh. 1.4 - Extracting Square Roots In Exercises 19-32, solve...Ch. 1.4 - Prob. 29ECh. 1.4 - Prob. 30ECh. 1.4 - Prob. 31ECh. 1.4 - Prob. 32ECh. 1.4 - Prob. 33ECh. 1.4 - Prob. 34ECh. 1.4 - Prob. 35ECh. 1.4 - Prob. 36ECh. 1.4 - Prob. 37ECh. 1.4 - Prob. 38ECh. 1.4 - Prob. 39ECh. 1.4 - Prob. 40ECh. 1.4 - Prob. 41ECh. 1.4 - Prob. 42ECh. 1.4 - Prob. 43ECh. 1.4 - Prob. 44ECh. 1.4 - Rewriting an Expression In Exercises 43-50,...Ch. 1.4 - Prob. 46ECh. 1.4 - Prob. 47ECh. 1.4 - Prob. 48ECh. 1.4 - Prob. 49ECh. 1.4 - Prob. 50ECh. 1.4 - Prob. 51ECh. 1.4 - Prob. 52ECh. 1.4 - Prob. 53ECh. 1.4 - Prob. 54ECh. 1.4 - Prob. 55ECh. 1.4 - Prob. 56ECh. 1.4 - Prob. 57ECh. 1.4 - Prob. 58ECh. 1.4 - Prob. 59ECh. 1.4 - Prob. 60ECh. 1.4 - Prob. 61ECh. 1.4 - Prob. 62ECh. 1.4 - Prob. 63ECh. 1.4 - Prob. 64ECh. 1.4 - Prob. 65ECh. 1.4 - Prob. 66ECh. 1.4 - Prob. 67ECh. 1.4 - Prob. 68ECh. 1.4 - Prob. 69ECh. 1.4 - Using the Quadratic Formula In Exercises 69-90,...Ch. 1.4 - Prob. 71ECh. 1.4 - Prob. 72ECh. 1.4 - Prob. 73ECh. 1.4 - Prob. 74ECh. 1.4 - Prob. 75ECh. 1.4 - Prob. 76ECh. 1.4 - Prob. 77ECh. 1.4 - Using the Quadratic Formula In Exercises 69-90,...Ch. 1.4 - Prob. 79ECh. 1.4 - Using the Quadratic Formula In Exercises 69-90,...Ch. 1.4 - Prob. 81ECh. 1.4 - Prob. 82ECh. 1.4 - Prob. 83ECh. 1.4 - Prob. 84ECh. 1.4 - Prob. 85ECh. 1.4 - Prob. 86ECh. 1.4 - Prob. 87ECh. 1.4 - Prob. 88ECh. 1.4 - Prob. 89ECh. 1.4 - Prob. 90ECh. 1.4 - Prob. 91ECh. 1.4 - Prob. 92ECh. 1.4 - Prob. 93ECh. 1.4 - Prob. 94ECh. 1.4 - Prob. 95ECh. 1.4 - Prob. 96ECh. 1.4 - Prob. 97ECh. 1.4 - Prob. 98ECh. 1.4 - Prob. 99ECh. 1.4 - Prob. 100ECh. 1.4 - Prob. 101ECh. 1.4 - Prob. 102ECh. 1.4 - Prob. 103ECh. 1.4 - Prob. 104ECh. 1.4 - Dimensions of a Floor The floor of a one-story...Ch. 1.4 - Dimensions of a Garden A gardener has 100 meters...Ch. 1.4 - Geometry You construct an open box with a square...Ch. 1.4 - Geometry You construct an open box from a square...Ch. 1.4 - Geometry An above-ground swimming pool contains...Ch. 1.4 - Seating A rectangular classroom seats 72 students....Ch. 1.4 - Using the Position Equation In Exercises 111-114,...Ch. 1.4 - Using the Position Equation In Exercises 111-114,...Ch. 1.4 - Using the Position Equation In Exercises 111-114,...Ch. 1.4 - Using the Position Equation In Exercises 111-114,...Ch. 1.4 - Public Debt The total public debt D (in trillions...Ch. 1.4 - Doctors treated a patient at an emergency room...Ch. 1.4 - Biology The metabolic rate of an ectothermic...Ch. 1.4 - Boating A winch tows a boat to a dock. The rope is...Ch. 1.4 - Flying Speed Two planes leave simultaneously from...Ch. 1.4 - Prob. 121ECh. 1.4 - Prob. 122ECh. 1.4 - Prob. 123ECh. 1.4 - Prob. 124ECh. 1.4 - Prob. 125ECh. 1.4 - Prob. 126ECh. 1.4 - Prob. 127ECh. 1.4 - Prob. 128ECh. 1.4 - Prob. 129ECh. 1.4 - Prob. 130ECh. 1.5 - Prob. 1ECPCh. 1.5 - Prob. 2ECPCh. 1.5 - Prob. 3ECPCh. 1.5 - Prob. 4ECPCh. 1.5 - Prob. 5ECPCh. 1.5 - Prob. 6ECPCh. 1.5 - Prob. 1ECh. 1.5 - Prob. 2ECh. 1.5 - Prob. 3ECh. 1.5 - Prob. 4ECh. 1.5 - Fill in the blanks. When a is a positive real...Ch. 1.5 - Prob. 6ECh. 1.5 - Prob. 7ECh. 1.5 - Equality of Complex Numbers In Exercises 7-10,...Ch. 1.5 - Prob. 9ECh. 1.5 - Equality of Complex Numbers In Exercises 7-10,...Ch. 1.5 - Prob. 11ECh. 1.5 - Prob. 12ECh. 1.5 - Prob. 13ECh. 1.5 - Prob. 14ECh. 1.5 - Prob. 15ECh. 1.5 - Prob. 16ECh. 1.5 - Prob. 17ECh. 1.5 - Prob. 18ECh. 1.5 - Prob. 19ECh. 1.5 - Prob. 20ECh. 1.5 - Prob. 21ECh. 1.5 - Prob. 22ECh. 1.5 - Prob. 23ECh. 1.5 - Prob. 24ECh. 1.5 - Prob. 25ECh. 1.5 - Prob. 26ECh. 1.5 - Prob. 27ECh. 1.5 - Prob. 28ECh. 1.5 - Prob. 29ECh. 1.5 - Prob. 30ECh. 1.5 - Prob. 31ECh. 1.5 - Prob. 32ECh. 1.5 - Prob. 33ECh. 1.5 - Prob. 34ECh. 1.5 - Prob. 35ECh. 1.5 - Prob. 36ECh. 1.5 - Prob. 37ECh. 1.5 - Prob. 38ECh. 1.5 - Prob. 39ECh. 1.5 - Prob. 40ECh. 1.5 - Prob. 41ECh. 1.5 - Prob. 42ECh. 1.5 - Prob. 43ECh. 1.5 - Prob. 44ECh. 1.5 - Prob. 45ECh. 1.5 - Prob. 46ECh. 1.5 - Prob. 47ECh. 1.5 - Prob. 48ECh. 1.5 - Prob. 49ECh. 1.5 - Prob. 50ECh. 1.5 - Prob. 51ECh. 1.5 - Prob. 52ECh. 1.5 - Prob. 53ECh. 1.5 - Prob. 54ECh. 1.5 - Prob. 55ECh. 1.5 - Prob. 56ECh. 1.5 - Prob. 57ECh. 1.5 - Prob. 58ECh. 1.5 - Prob. 59ECh. 1.5 - Prob. 60ECh. 1.5 - Prob. 61ECh. 1.5 - Prob. 62ECh. 1.5 - Prob. 63ECh. 1.5 - Prob. 64ECh. 1.5 - Prob. 65ECh. 1.5 - Prob. 66ECh. 1.5 - Prob. 67ECh. 1.5 - Prob. 68ECh. 1.5 - Prob. 69ECh. 1.5 - Prob. 70ECh. 1.5 - Prob. 71ECh. 1.5 - Prob. 72ECh. 1.5 - Prob. 73ECh. 1.5 - Prob. 74ECh. 1.5 - Prob. 75ECh. 1.5 - Prob. 76ECh. 1.5 - Prob. 77ECh. 1.5 - Prob. 78ECh. 1.5 - Prob. 79ECh. 1.5 - Prob. 80ECh. 1.5 - Prob. 81ECh. 1.5 - Prob. 82ECh. 1.5 - Prob. 83ECh. 1.5 - Prob. 84ECh. 1.5 - Prob. 85ECh. 1.5 - Prob. 86ECh. 1.5 - The opposition to current in an electrical circuit...Ch. 1.5 - Prob. 88ECh. 1.5 - Prob. 89ECh. 1.5 - Prob. 90ECh. 1.5 - Prob. 91ECh. 1.5 - Prob. 92ECh. 1.5 - Prob. 93ECh. 1.5 - Prob. 94ECh. 1.5 - Prob. 95ECh. 1.5 - Prob. 96ECh. 1.5 - Proof Prove that the complex conjugate of the sum...Ch. 1.6 - Solve 9x412x2=0 and check your solution(s).Ch. 1.6 - Solve each equation. a.x35x22x+10=0b.6x327x254x=0Ch. 1.6 - Solve each equation. a.x47x2+12=0b.9x437x2+4=0Ch. 1.6 - Prob. 4ECPCh. 1.6 - Prob. 5ECPCh. 1.6 - Prob. 6ECPCh. 1.6 - Prob. 7ECPCh. 1.6 - A high school charters a bus for $560 to take a...Ch. 1.6 - You deposit $2500 in a long-term investment in...Ch. 1.6 - Fill in the blanks. Fill in the blanks. The...Ch. 1.6 - Fill in the blanks. An equation is of when it can...Ch. 1.6 - Prob. 3ECh. 1.6 - Prob. 4ECh. 1.6 - Solving a Polynomial Equation In Exercises 5-16,...Ch. 1.6 - Solving a Polynomial Equation In Exercises 5-16,...Ch. 1.6 - Solving a Polynomial Equation In Exercises 5-16,...Ch. 1.6 - Solving a Polynomial Equation In Exercises 5-16,...Ch. 1.6 - Solving a Polynomial Equation In Exercises 5-16,...Ch. 1.6 - Prob. 10ECh. 1.6 - Prob. 11ECh. 1.6 - Solving a Polynomial Equation In Exercises 5-16,...Ch. 1.6 - Solving a Polynomial Equation In Exercises 5-16,...Ch. 1.6 - Prob. 14ECh. 1.6 - Prob. 15ECh. 1.6 - Prob. 16ECh. 1.6 - Prob. 17ECh. 1.6 - Solving an Equation of Quadratic Type In Exercises...Ch. 1.6 - Prob. 19ECh. 1.6 - Prob. 20ECh. 1.6 - Prob. 21ECh. 1.6 - Prob. 22ECh. 1.6 - Prob. 23ECh. 1.6 - Prob. 24ECh. 1.6 - Prob. 25ECh. 1.6 - Prob. 26ECh. 1.6 - Prob. 27ECh. 1.6 - Prob. 28ECh. 1.6 - Prob. 29ECh. 1.6 - Prob. 30ECh. 1.6 - Prob. 31ECh. 1.6 - Solving a Radical Equation In Exercises 31-44,...Ch. 1.6 - Prob. 33ECh. 1.6 - Solving a Radical Equation In Exercises 31-44,...Ch. 1.6 - Prob. 35ECh. 1.6 - Prob. 36ECh. 1.6 - Prob. 37ECh. 1.6 - Prob. 38ECh. 1.6 - Prob. 39ECh. 1.6 - Prob. 40ECh. 1.6 - Prob. 41ECh. 1.6 - Prob. 42ECh. 1.6 - Prob. 43ECh. 1.6 - Prob. 44ECh. 1.6 - Prob. 45ECh. 1.6 - Prob. 46ECh. 1.6 - Prob. 47ECh. 1.6 - Prob. 48ECh. 1.6 - Prob. 49ECh. 1.6 - Prob. 50ECh. 1.6 - Solving a Rational Equation In Exercises 51-58,...Ch. 1.6 - Solving a Rational Equation In Exercises 51-58,...Ch. 1.6 - Prob. 53ECh. 1.6 - Solving a Rational Equation In Exercises 51-58,...Ch. 1.6 - Solving a Rational Equation In Exercises 51-58,...Ch. 1.6 - Prob. 56ECh. 1.6 - Prob. 57ECh. 1.6 - Prob. 58ECh. 1.6 - Solving an Absolute Value Equation In Exercises...Ch. 1.6 - Solving an Absolute Value Equation In Exercises...Ch. 1.6 - Prob. 61ECh. 1.6 - Solving an Absolute Value Equation In Exercises...Ch. 1.6 - Prob. 63ECh. 1.6 - Prob. 64ECh. 1.6 - Prob. 65ECh. 1.6 - Prob. 66ECh. 1.6 - Prob. 67ECh. 1.6 - Prob. 68ECh. 1.6 - Prob. 69ECh. 1.6 - Prob. 70ECh. 1.6 - Prob. 71ECh. 1.6 - Prob. 72ECh. 1.6 - Prob. 73ECh. 1.6 - Prob. 74ECh. 1.6 - Solving an Equation In Exercises 75-82, find the...Ch. 1.6 - Prob. 76ECh. 1.6 - Prob. 77ECh. 1.6 - Solving an Equation In Exercises 75-82, find the...Ch. 1.6 - Prob. 79ECh. 1.6 - Prob. 80ECh. 1.6 - Solving an Equation In Exercises 75-82, find the...Ch. 1.6 - Prob. 82ECh. 1.6 - Think About It In Exercises 83-92, write an...Ch. 1.6 - Think About It In Exercises 83-92, write an...Ch. 1.6 - Prob. 85ECh. 1.6 - Prob. 86ECh. 1.6 - Think About It In Exercises 83-92, write an...Ch. 1.6 - Prob. 88ECh. 1.6 - Prob. 89ECh. 1.6 - Think About It In Exercises 83-92, write an...Ch. 1.6 - Prob. 91ECh. 1.6 - Prob. 92ECh. 1.6 - Prob. 93ECh. 1.6 - Reduced Rates Three students plan to divide the...Ch. 1.6 - Average Speed An airline runs a commuter flight...Ch. 1.6 - Average Speed A family drives 1080 miles to a...Ch. 1.6 - Compound Interest You deposit $2500 in a long-term...Ch. 1.6 - Compound Interest You deposit $6000 in a long-term...Ch. 1.6 - Airline Passengers An airline offers daily flights...Ch. 1.6 - Nursing The number of registered nursing graduates...Ch. 1.6 - The temperature T (in degrees Fahrenheit) of...Ch. 1.6 - Voting-Age Population The total voting-age...Ch. 1.6 - Demand The demand for a video game can be modeled...Ch. 1.6 - Power Line A power station is on one side of a...Ch. 1.6 - Prob. 105ECh. 1.6 - Painting a Fence Working alone, you can paint a...Ch. 1.6 - Prob. 107ECh. 1.6 - Circumference of an Ellipse The circumference C of...Ch. 1.6 - Prob. 109ECh. 1.6 - Prob. 110ECh. 1.6 - Prob. 111ECh. 1.6 - Prob. 112ECh. 1.6 - Prob. 113ECh. 1.6 - HOW DO YOU SEE IT? The figure shows a glass cube...Ch. 1.6 - Prob. 115ECh. 1.6 - Prob. 116ECh. 1.6 - Prob. 117ECh. 1.6 - Prob. 118ECh. 1.7 - Write an inequality that represents each interval....Ch. 1.7 - Prob. 2ECPCh. 1.7 - Prob. 3ECPCh. 1.7 - Prob. 4ECPCh. 1.7 - Prob. 5ECPCh. 1.7 - Prob. 6ECPCh. 1.7 - Prob. 7ECPCh. 1.7 - Prob. 1ECh. 1.7 - Prob. 2ECh. 1.7 - Fill in the blanks. It is sometimes possible to...Ch. 1.7 - Prob. 4ECh. 1.7 - Intervals and Inequalities In Exercises 5-12,...Ch. 1.7 - Intervals and Inequalities In Exercises 5-12,...Ch. 1.7 - Intervals and Inequalities In Exercises 5-12,...Ch. 1.7 - Prob. 8ECh. 1.7 - Intervals and Inequalities In Exercises 5-12,...Ch. 1.7 - Prob. 10ECh. 1.7 - Intervals and Inequalities In Exercises 5-12,...Ch. 1.7 - Intervals and Inequalities In Exercises 5-12,...Ch. 1.7 - Solving a Linear Inequality In Exercises 13-30,...Ch. 1.7 - Prob. 14ECh. 1.7 - Solving a Linear Inequality In Exercises 13-30,...Ch. 1.7 - Prob. 16ECh. 1.7 - Solving a Linear Inequality In Exercises 13-30,...Ch. 1.7 - Solving a Linear Inequality In Exercises 13-30,...Ch. 1.7 - Solving a Linear Inequality In Exercises 13-30,...Ch. 1.7 - Prob. 20ECh. 1.7 - Solving a Linear Inequality In Exercises 13-30,...Ch. 1.7 - Prob. 22ECh. 1.7 - Solving a Linear Inequality In Exercises 13-30,...Ch. 1.7 - Solving a Linear Inequality In Exercises 13-30,...Ch. 1.7 - Solving a Linear Inequality In Exercises 13-30,...Ch. 1.7 - Prob. 26ECh. 1.7 - Solving a Linear Inequality In Exercises 13-30,...Ch. 1.7 - Prob. 28ECh. 1.7 - Solving a Linear Inequality In Exercises 13-30,...Ch. 1.7 - Solving a Linear Inequality In Exercises 13-30,...Ch. 1.7 - Solving a Double Inequality In Exercises 31-42,...Ch. 1.7 - Prob. 32ECh. 1.7 - Solving a Double Inequality In Exercises 31-42,...Ch. 1.7 - Prob. 34ECh. 1.7 - Solving a Double Inequality In Exercises 31-42,...Ch. 1.7 - Solving a Double Inequality In Exercises 31-42,...Ch. 1.7 - Solving a Double Inequality In Exercises 31-42,...Ch. 1.7 - Prob. 38ECh. 1.7 - Solving a Double Inequality In Exercises 31-42,...Ch. 1.7 - Prob. 40ECh. 1.7 - Solving a Double Inequality In Exercises 31-42,...Ch. 1.7 - Solving a Double Inequality In Exercises 31-42,...Ch. 1.7 - Solving an Absolute Value Inequality In Exercises...Ch. 1.7 - Prob. 44ECh. 1.7 - Solving an Absolute Value Inequality In Exercises...Ch. 1.7 - Prob. 46ECh. 1.7 - Solving an Absolute Value Inequality In Exercises...Ch. 1.7 - Solving an Absolute Value Inequality In Exercises...Ch. 1.7 - Solving an Absolute Value Inequality In Exercises...Ch. 1.7 - Prob. 50ECh. 1.7 - Solving an Absolute Value Inequality In Exercises...Ch. 1.7 - Prob. 52ECh. 1.7 - Solving an Absolute Value Inequality In Exercises...Ch. 1.7 - Solving an Absolute Value Inequality In Exercises...Ch. 1.7 - Solving an Absolute Value Inequality In Exercises...Ch. 1.7 - Prob. 56ECh. 1.7 - Solving an Absolute Value Inequality In Exercises...Ch. 1.7 - Prob. 58ECh. 1.7 - Using Technology In Exercises 59-68, use a...Ch. 1.7 - Using Technology In Exercises 59-68, use a...Ch. 1.7 - Using Technology In Exercises 59-68, use a...Ch. 1.7 - Prob. 62ECh. 1.7 - Using Technology In Exercises 59-68, use a...Ch. 1.7 - Prob. 64ECh. 1.7 - Using Technology In Exercises 59-68, use a...Ch. 1.7 - Using Technology In Exercises 59-68, use a...Ch. 1.7 - Using Technology In Exercises 59-68, use a...Ch. 1.7 - Prob. 68ECh. 1.7 - Using Technology In Exercises 69-74, use a...Ch. 1.7 - Prob. 70ECh. 1.7 - Prob. 71ECh. 1.7 - Using Technology In Exercises 69-74, use a...Ch. 1.7 - Prob. 73ECh. 1.7 - Prob. 74ECh. 1.7 - Think About It The graph of x53 can be described...Ch. 1.7 - Prob. 76ECh. 1.7 - Prob. 77ECh. 1.7 - Using Absolute Value In Exercises 77-84, use...Ch. 1.7 - Prob. 79ECh. 1.7 - Prob. 80ECh. 1.7 - Prob. 81ECh. 1.7 - Prob. 82ECh. 1.7 - Prob. 83ECh. 1.7 - Prob. 84ECh. 1.7 - Prob. 85ECh. 1.7 - Prob. 86ECh. 1.7 - Prob. 87ECh. 1.7 - Prob. 88ECh. 1.7 - Prob. 89ECh. 1.7 - Prob. 90ECh. 1.7 - Job Offers You are considering two job offers. The...Ch. 1.7 - Job Offers You are considering two job offers. The...Ch. 1.7 - Prob. 93ECh. 1.7 - Prob. 94ECh. 1.7 - Prob. 95ECh. 1.7 - Prob. 96ECh. 1.7 - Daily Sales A doughnut shop sells a dozen...Ch. 1.7 - Prob. 98ECh. 1.7 - Prob. 99ECh. 1.7 - Prob. 100ECh. 1.7 - Chemists’ Wages The mean hourly wage W (in...Ch. 1.7 - Milk Production Milk production M (in billions of...Ch. 1.7 - Prob. 103ECh. 1.7 - Prob. 104ECh. 1.7 - Prob. 105ECh. 1.7 - Accuracy of Measurement You stop at a self-service...Ch. 1.7 - Geometry The side length of a square is 10.4...Ch. 1.7 - Prob. 108ECh. 1.7 - Prob. 109ECh. 1.7 - Prob. 110ECh. 1.7 - Prob. 111ECh. 1.7 - Prob. 112ECh. 1.7 - Prob. 113ECh. 1.7 - Prob. 114ECh. 1.7 - Prob. 115ECh. 1.8 - Solve x2x200. Then graph the solution set.Ch. 1.8 - Prob. 2ECPCh. 1.8 - Prob. 3ECPCh. 1.8 - Prob. 4ECPCh. 1.8 - Prob. 5ECPCh. 1.8 - Prob. 6ECPCh. 1.8 - Prob. 7ECPCh. 1.8 - Prob. 1ECh. 1.8 - Prob. 2ECh. 1.8 - Prob. 3ECh. 1.8 - Prob. 4ECh. 1.8 - Checking Solutions In Exercises 5-8, determine...Ch. 1.8 - Prob. 6ECh. 1.8 - Checking Solutions In Exercises 5-8, determine...Ch. 1.8 - Checking Solutions In Exercises 5-8, determine...Ch. 1.8 - Finding Key Numbers In Exercises 9-12, find the...Ch. 1.8 - Prob. 10ECh. 1.8 - Finding Key Numbers In Exercises 9-12, find the...Ch. 1.8 - Prob. 12ECh. 1.8 - Solving a Polynomial Inequality In Exercises...Ch. 1.8 - Solving a Polynomial Inequality In Exercises...Ch. 1.8 - Solving a Polynomial Inequality In Exercises...Ch. 1.8 - Prob. 16ECh. 1.8 - Solving a Polynomial Inequality In Exercises...Ch. 1.8 - Prob. 18ECh. 1.8 - Solving a Polynomial Inequality In Exercises...Ch. 1.8 - Prob. 20ECh. 1.8 - Solving a Polynomial Inequality In Exercises...Ch. 1.8 - Prob. 22ECh. 1.8 - Solving a Polynomial Inequality In Exercises...Ch. 1.8 - Prob. 24ECh. 1.8 - Solving a Polynomial Inequality In Exercises...Ch. 1.8 - Prob. 26ECh. 1.8 - Solving a Polynomial Inequality In Exercises...Ch. 1.8 - Prob. 28ECh. 1.8 - Solving a Polynomial Inequality In Exercises...Ch. 1.8 - Prob. 30ECh. 1.8 - Prob. 31ECh. 1.8 - Prob. 32ECh. 1.8 - Prob. 33ECh. 1.8 - Prob. 34ECh. 1.8 - Prob. 35ECh. 1.8 - Prob. 36ECh. 1.8 - Prob. 37ECh. 1.8 - Prob. 38ECh. 1.8 - Prob. 39ECh. 1.8 - Prob. 40ECh. 1.8 - Solving a Rational Inequality In Exercises 41-52,...Ch. 1.8 - Solving a Rational Inequality In Exercises 41-52,...Ch. 1.8 - Solving a Rational Inequality In Exercises 41-52,...Ch. 1.8 - Solving a Rational Inequality In Exercises 41-52,...Ch. 1.8 - Solving a Rational Inequality In Exercises 41-52,...Ch. 1.8 - Prob. 46ECh. 1.8 - Prob. 47ECh. 1.8 - Prob. 48ECh. 1.8 - Prob. 49ECh. 1.8 - Prob. 50ECh. 1.8 - Prob. 51ECh. 1.8 - Solving a Rational Inequality In Exercises 41-52,...Ch. 1.8 - Prob. 53ECh. 1.8 - Prob. 54ECh. 1.8 - Prob. 55ECh. 1.8 - Prob. 56ECh. 1.8 - Prob. 57ECh. 1.8 - Prob. 58ECh. 1.8 - Prob. 59ECh. 1.8 - Prob. 60ECh. 1.8 - Solving an Inequality In Exercises 61-66, solve...Ch. 1.8 - Prob. 62ECh. 1.8 - Prob. 63ECh. 1.8 - Prob. 64ECh. 1.8 - Prob. 65ECh. 1.8 - Prob. 66ECh. 1.8 - Prob. 67ECh. 1.8 - Prob. 68ECh. 1.8 - Prob. 69ECh. 1.8 - Prob. 70ECh. 1.8 - Prob. 71ECh. 1.8 - Prob. 72ECh. 1.8 - Prob. 73ECh. 1.8 - Prob. 74ECh. 1.8 - Prob. 75ECh. 1.8 - Prob. 76ECh. 1.8 - Prob. 77ECh. 1.8 - Safe Load The maximum safe load uniformly...Ch. 1.8 - Geometry A rectangular playing field with a...Ch. 1.8 - Geometry A rectangular parking lot with a...Ch. 1.8 - Resistors When two resistors of resistances R1 and...Ch. 1.8 - Prob. 82ECh. 1.8 - Prob. 83ECh. 1.8 - Prob. 84ECh. 1.8 - Prob. 85ECh. 1.8 - Prob. 86ECh. 1.8 - Prob. 87ECh. 1.8 - Prob. 88ECh. 1.8 - Prob. 89ECh. 1.8 - Prob. 90ECh. 1 - Sketching the Graph of an Equation In Exercises 1...Ch. 1 - Sketching the Graph of an Equation In Exercises 1...Ch. 1 - Identifying x-andy-Intercepts In Exercises 3 and...Ch. 1 - Identifying x-andy-Intercepts In Exercises 3 and...Ch. 1 - Prob. 5RECh. 1 - Prob. 6RECh. 1 - Prob. 7RECh. 1 - Prob. 8RECh. 1 - Prob. 9RECh. 1 - Prob. 10RECh. 1 - Prob. 11RECh. 1 - Prob. 12RECh. 1 - Prob. 13RECh. 1 - Prob. 14RECh. 1 - Prob. 15RECh. 1 - Prob. 16RECh. 1 - Prob. 17RECh. 1 - Prob. 18RECh. 1 - Sales The sales S (in millions of dollars) for...Ch. 1 - Physics The force F (in pounds) required to...Ch. 1 - Classifying an Equation In Exercises 21-24,...Ch. 1 - Classifying an Equation In Exercises 21-24,...Ch. 1 - Classifying an Equation In Exercises 21-24,...Ch. 1 - Classifying an Equation In Exercises 21-24,...Ch. 1 - Solving an Equation In Exercises 25-32, solve the...Ch. 1 - Solving an Equation In Exercises 25-32, solve the...Ch. 1 - Solving an Equation In Exercises 25-32, solve the...Ch. 1 - Solving an Equation In Exercises 25-32, solve the...Ch. 1 - Solving an Equation In Exercises 25-32, solve the...Ch. 1 - Solving an Equation In Exercises 25-32, solve the...Ch. 1 - Solving an Equation In Exercises 25-32, solve the...Ch. 1 - Solving an Equation In Exercises 25-32, solve the...Ch. 1 - Finding Intercepts Algebraically In Exercises...Ch. 1 - Finding Intercepts Algebraically In Exercises...Ch. 1 - Finding Intercepts Algebraically In Exercises...Ch. 1 - Finding Intercepts Algebraically In Exercises...Ch. 1 - Finding Intercepts Algebraically In Exercises...Ch. 1 - Finding Intercepts Algebraically In Exercises...Ch. 1 - Geometry The surface area S of the cylinder shown...Ch. 1 - Temperature The Fahrenheit and Celsius temperature...Ch. 1 - Profit In 2014, LinkedIn’s total revenue was 45.2...Ch. 1 - Discount The sale price of a digital camera after...Ch. 1 - Business Venture You are starting a small...Ch. 1 - Average Speed You commute 56 miles one way to...Ch. 1 - Mixture Problem A car radiator contains 10 liters...Ch. 1 - Simple Interest You invest $6000 at 412 and 512...Ch. 1 - Volume of a Cone The volume V of a cone can be...Ch. 1 - Kinetic Energy The kinetic energy E of an object...Ch. 1 - Choosing a Method In Exercises 49-58, solve the...Ch. 1 - Choosing a Method In Exercises 49-58, solve the...Ch. 1 - Choosing a Method In Exercises 49-58, solve the...Ch. 1 - Choosing a Method In Exercises 49-58, solve the...Ch. 1 - Choosing a Method In Exercises 49-58, solve the...Ch. 1 - Choosing a Method In Exercises 49-58, solve the...Ch. 1 - Choosing a Method In Exercises 49-58, solve the...Ch. 1 - Prob. 56RECh. 1 - Prob. 57RECh. 1 - Prob. 58RECh. 1 - Simply Supported Beam A simply supported 20-foot...Ch. 1 - Sports You throw a softball straight up into the...Ch. 1 - Prob. 61RECh. 1 - Prob. 62RECh. 1 - Prob. 63RECh. 1 - Prob. 64RECh. 1 - Prob. 65RECh. 1 - Prob. 66RECh. 1 - Prob. 67RECh. 1 - Prob. 68RECh. 1 - Prob. 69RECh. 1 - Prob. 70RECh. 1 - Prob. 71RECh. 1 - Prob. 72RECh. 1 - Prob. 73RECh. 1 - Prob. 74RECh. 1 - Prob. 75RECh. 1 - Prob. 76RECh. 1 - Complex Solutions of a Quadratic Equation In...Ch. 1 - Prob. 78RECh. 1 - Prob. 79RECh. 1 - Prob. 80RECh. 1 - Prob. 81RECh. 1 - Prob. 82RECh. 1 - Prob. 83RECh. 1 - Prob. 84RECh. 1 - Prob. 85RECh. 1 - Prob. 86RECh. 1 - Prob. 87RECh. 1 - Prob. 88RECh. 1 - Prob. 89RECh. 1 - Prob. 90RECh. 1 - Prob. 91RECh. 1 - Prob. 92RECh. 1 - Prob. 93RECh. 1 - Prob. 94RECh. 1 - Prob. 95RECh. 1 - Prob. 96RECh. 1 - Prob. 97RECh. 1 - Prob. 98RECh. 1 - Prob. 99RECh. 1 - Intervals and Inequalities In Exercises 99-102,...Ch. 1 - Prob. 101RECh. 1 - Prob. 102RECh. 1 - Prob. 103RECh. 1 - Prob. 104RECh. 1 - Prob. 105RECh. 1 - Prob. 106RECh. 1 - Prob. 107RECh. 1 - Prob. 108RECh. 1 - Cost, Revenue, and Profit The revenue for selling...Ch. 1 - Prob. 110RECh. 1 - Prob. 111RECh. 1 - Prob. 112RECh. 1 - Prob. 113RECh. 1 - Prob. 114RECh. 1 - Prob. 115RECh. 1 - Prob. 116RECh. 1 - Prob. 117RECh. 1 - Prob. 118RECh. 1 - Prob. 119RECh. 1 - True or False? In Exercises 119 and 120, determine...Ch. 1 - Prob. 121RECh. 1 - Prob. 122RECh. 1 - Prob. 1TCh. 1 - In Exercises 1-6, use the algebraic tests to check...Ch. 1 - Prob. 3TCh. 1 - Prob. 4TCh. 1 - Prob. 5TCh. 1 - In Exercises 1-6, use the algebraic tests to check...Ch. 1 - In Exercises 7-12, solve the equation and check...Ch. 1 - Prob. 8TCh. 1 - In Exercises 7-12, solve the equation and check...Ch. 1 - Prob. 10TCh. 1 - Prob. 11TCh. 1 - Prob. 12TCh. 1 - In Exercises 13-16, solve the inequality. Then...Ch. 1 - Prob. 14TCh. 1 - In Exercises 13-16, solve the inequality. Then...Ch. 1 - Prob. 16TCh. 1 - Perform each operation and write the result in...Ch. 1 - Prob. 18TCh. 1 - Prob. 19TCh. 1 - Prob. 20TCh. 1 - A basketball has a volume of about 455.9 cubic...Ch. 1 - On the first part of a 350-kilometer trip, a...Ch. 1 - Prob. 23TCh. 1 - Prob. 1PSCh. 1 - Prob. 2PSCh. 1 - Prob. 3PSCh. 1 - Prob. 4PSCh. 1 - Prob. 5PSCh. 1 - Water Height For a bathtub with a rectangular...Ch. 1 - Prob. 7PSCh. 1 - Prob. 8PSCh. 1 - Prob. 9PSCh. 1 - Prob. 10PSCh. 1 - Prob. 11PSCh. 1 - Prob. 12PSCh. 1 - Prob. 13PSCh. 1 - Proof Prove that the product of a complex number...Ch. 1 - The Mandelbrot Set A fractal is a geometric figure...
Knowledge Booster
Similar questions
- In a national park, the current population of an endangered species of bear is 80. Each year, the population decreases by 10%. How can you model the population of bears in the park? # of years # of bears 9 10 2 3 4 5 6 7 8 ° 1 Graph your data. Remember to title your graph. What scale should be used on the y-axis? What scale should be used on the x-axis? SMOKY 19 OUNTAINS NATIONAL Exponential Decay Equation y = a(1-r)* PARKarrow_forwardOn Feb. 8, this year, at 6am in the morning all UiB meteorology professors met to discuss a highly unfortunate and top-urgent crisis: Their most precious instrument, responsible for measuring the air temperature hour-by- hour, had failed - what if the Bergen public would find out? How would they plan their weekend without up-to-date air temperature readings? Silent devastation - and maybe a hint of panic, also - hung in the room. Apprentice Taylor, who - as always - was late to the meeting, sensed that this was his chance to shine! Could they fake the data? At least for some hours (until the measurements would work again)? He used to spend a lot of time online and thus knew the value of fake data, especially when it spread fast! He reminded the crying professors of a prehistoric project with the title "Love your derivatives as you love yourself!" - back then, they had installed top-modern technology that not only measured the air temperature itself, but also its 1st, 2nd, 3rd, 4th, and…arrow_forwardConsider a forest where the population of a particular plant species grows exponentially. In a real-world scenario, we often deal with systems where the analytical function describing the phenomenon is not available. In such cases, numerical methods come in handy. For the sake of this task, however, you are provided with an analytical function so that you can compare the results of the numerical methods to some ground truth. The population P(t) of the plants at time t (in years) is given by the equation: P(t) = 200 0.03 t You are tasked with estimating the rate of change of the plant population at t = 5 years using numerical differentiation methods. First, compute the value of P'(t) at t = 5 analytically. Then, estimate P'(t) at t = 5 years using the following numerical differentiation methods: ⚫ forward difference method (2nd-order accurate) 3 ⚫ backward difference method (2nd-order accurate) ⚫ central difference method (2nd-order accurate) Use h = 0.5 as the step size and round all…arrow_forward
- Nicole organized a new corporation. The corporation began business on April 1 of year 1. She made the following expenditures associated with getting the corporation started: Expense Date Amount Attorney fees for articles of incorporation February 10 $ 40,500 March 1-March 30 wages March 30 6,550 March 1-March 30 rent Stock issuance costs March 30 2,850 April 1-May 30 wages Note: Leave no answer blank. Enter zero if applicable. April 1 May 30 24,000 16,375 c. What amount can the corporation deduct as amortization expense for the organizational expenditures and for the start-up costs for year 1 [not including the amount determined in part (b)]? Note: Round intermediate calculations to 2 decimal places and final answer to the nearest whole dollar amount. Start-up costs amortized Organizational expenditures amortizedarrow_forwardLast Chance Mine (LCM) purchased a coal deposit for $2,918,300. It estimated it would extract 18,950 tons of coal from the deposit. LCM mined the coal and sold it, reporting gross receipts of $1.24 million, $13 million, and $11 million for years 1 through 3, respectively. During years 1-3, LCM reported net income (loss) from the coal deposit activity in the amount of ($11,400), $550,000, and $502,500, respectively. In years 1-3, LCM extracted 19,950 tons of coal as follows: (1) Tons of Coal 18,950 Depletion (2) Basis (2)(1) Rate $2,918,300 $154.00 Tons Extracted per Year Year 1 4,500 Year 2 8,850 Year 3 6,600 Note: Leave no answer blank. Enter zero if applicable. Enter your answers in dollars and not in millions of dollars. a. What is LCM's cost depletion for years 1, 2, and 3? Cost Depletion Year 1 Year 2 Year 3arrow_forwardConsider the following equation. log1/9' =6 Find the value of x. Round your answer to the nearest thousandth. x = ✓arrow_forward
- Expanding a logarithmic expression: Problem type 3 Use the properties of logarithms to expand the following expression. 4(8+x)² log 5 ) Your answer should not have radicals or exponents. You may assume that all variables are positive. log 4(8 + X 5 -x)²arrow_forwardUse the properties of logarithms to expand the following expression. log 6(x+5)² 3/24 Your answer should not have radicals or exponents. You may assume that all variables are positive. log 6(x + 3 I 4 5)² log Xarrow_forwardExpanding a logarithmic expression: Problem type 2 Use the properties of logarithms to expand the following expression. 3 yz log 5 x 0/3 An Each logarithm should involve only one variable and should not have any radicals or exponents. You may assume that all variables are positive. log yz 3 厚 5 Explanation Check log ☑ 2025 MG ¿W MIII LLC. All Rights Reserved. Terms of Use | Privacy Centerarrow_forward
- Expanding a logarithmic expression: Problem type 2 Use the properties of logarithms to expand the following expression. 3 yz log 5 x 0/3 An Each logarithm should involve only one variable and should not have any radicals or exponents. You may assume that all variables are positive. log yz 3 厚 5 Explanation Check log ☑ 2025 MG ¿W MIII LLC. All Rights Reserved. Terms of Use | Privacy Centerarrow_forwardWhat is the domain and range, thank you !!arrow_forwardAssume a bivariate patch p(u, v) over the unit square [0, 1]² that is given as a tensor product patch where u-sections (u fixed to some constant û; v varying across [0, 1]) are quadratic polynomials Pu:û(v) = p(û, v) while v-sections are lines pv:ô (u) = p(u, v). The boundary lines pv:o(u) and pv:1 (u) are specified by their end points p(0,0) 0.8 and p(1,0) 0.2 as well as p(0, 1) 0.3 and p(1, 1) = 0.8. The boundary quadratics pu:o(v) and pu:1 (v) interpolate p(0,0.5) = 0.1 and p(1, 0.5) = 0.9 in addition to the above given four corner-values. = = = Use Pu:û(v) = (1, v, v² ) Mq (Pu:û(0), Pu:û (0.5), Pu:û(1)) with Ma = 1 0 0 -3 4-1 2 4 2 (Pv:ô as well as pu: (u) = (1, u) M₁ (pv:v (0), P: (1)) with M₁ = = (19) 0 to formulate p(u, v) using the "geometric input" G with G = = (P(0,0%) p(0,0) p(0,0.5) p(0,1) ) = ( 0.39 0.8 0.1 0.3 0.2 0.9 0.8 p(1,0) p(1, 0.5) p(1, 1) See the figure below for (left) a selection of iso-lines of p(u, v) and (right) a 3D rendering of p(u, v) as a height surface…arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
College AlgebraAlgebraISBN:9781305115545Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Holt Mcdougal Larson Pre-algebra: Student Edition...
Algebra
ISBN:9780547587776
Author:HOLT MCDOUGAL
Publisher:HOLT MCDOUGAL
Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning
Algebra and Trigonometry (MindTap Course List)
Algebra
ISBN:9781305071742
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning
College Algebra (MindTap Course List)
Algebra
ISBN:9781305652231
Author:R. David Gustafson, Jeff Hughes
Publisher:Cengage Learning
College Algebra
Algebra
ISBN:9781305115545
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning