Y Submit Part D-Clockwise Rotation of a Stress Element with No Shear Stress The state of stress at a point in a member is shown on the rectangular stress element in (Eigure 6) where the magnitudes of the stresses are lo₂-13 ksi and lo, 12 ksi Determine the state of stress on an element rotated such that the +2' axis is 60° below the - axis of the original stress element. Express your answers, separated by commas, to three significant figures. View Available Hint(s) Ợ Uy Tây Submit < Return to Assignment LIVE ΑΣΦ. 11 | vec Provide Feedback 1 ksi, ksi, ksi a ? ksi, ksi, ksi 2 W + W {0 Figure ▼ Part A State of Stress on an Inclined Plane 0₂ < 6 of 6 >
Y Submit Part D-Clockwise Rotation of a Stress Element with No Shear Stress The state of stress at a point in a member is shown on the rectangular stress element in (Eigure 6) where the magnitudes of the stresses are lo₂-13 ksi and lo, 12 ksi Determine the state of stress on an element rotated such that the +2' axis is 60° below the - axis of the original stress element. Express your answers, separated by commas, to three significant figures. View Available Hint(s) Ợ Uy Tây Submit < Return to Assignment LIVE ΑΣΦ. 11 | vec Provide Feedback 1 ksi, ksi, ksi a ? ksi, ksi, ksi 2 W + W {0 Figure ▼ Part A State of Stress on an Inclined Plane 0₂ < 6 of 6 >
Elements Of Electromagnetics
7th Edition
ISBN:9780190698614
Author:Sadiku, Matthew N. O.
Publisher:Sadiku, Matthew N. O.
ChapterMA: Math Assessment
Section: Chapter Questions
Problem 1.1MA
Related questions
Question
![**Topic: Clockwise Rotation of a Stress Element with No Shear Stress**
**Part D:**
The state of stress at a point in a member is shown on the rectangular stress element in the figure. The magnitudes of the stresses are \(\sigma_x = 13 \, \text{ksi}\) and \(\sigma_y = 12 \, \text{ksi}\).
Determine the state of stress on an element rotated such that the \(+x'\) axis is \(60^\circ\) below the \(-x\) axis of the original stress element.
**Instructions:**
Express your answers, separated by commas, to three significant figures.
[**Hint Section Available**]
**Input Fields for Solutions:**
\[ \sigma_{x'} \), \sigma_{y'}, τ_{x'y'} = \quad \text{ksi, ksi, ksi}
[Submit Button Below]
---
**Figure Explanation:**
The figure represents a rectangular stress element with:
- \(\sigma_x\) acting horizontally to the right,
- \(\sigma_y\) acting vertically downward.
This is to assess the stresses resulting from rotation, crucial for understanding stress transformations in materials.
---
**Navigation Links:**
- Return to Assignment
- Provide Feedback](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F462ae637-bd5b-4659-9142-cd2b96956849%2Ff1788972-78d2-48a0-829c-56cabe725f5a%2Fmz6bnq_processed.jpeg&w=3840&q=75)
Transcribed Image Text:**Topic: Clockwise Rotation of a Stress Element with No Shear Stress**
**Part D:**
The state of stress at a point in a member is shown on the rectangular stress element in the figure. The magnitudes of the stresses are \(\sigma_x = 13 \, \text{ksi}\) and \(\sigma_y = 12 \, \text{ksi}\).
Determine the state of stress on an element rotated such that the \(+x'\) axis is \(60^\circ\) below the \(-x\) axis of the original stress element.
**Instructions:**
Express your answers, separated by commas, to three significant figures.
[**Hint Section Available**]
**Input Fields for Solutions:**
\[ \sigma_{x'} \), \sigma_{y'}, τ_{x'y'} = \quad \text{ksi, ksi, ksi}
[Submit Button Below]
---
**Figure Explanation:**
The figure represents a rectangular stress element with:
- \(\sigma_x\) acting horizontally to the right,
- \(\sigma_y\) acting vertically downward.
This is to assess the stresses resulting from rotation, crucial for understanding stress transformations in materials.
---
**Navigation Links:**
- Return to Assignment
- Provide Feedback

Transcribed Image Text:## Part C - Counterclockwise Rotation of a Stress Element
The state of stress at a point in a member is shown on the rectangular stress element in **Figure 5**, where the magnitudes of the stresses are:
- \(\sigma_x = 16 \, \text{ksi}\)
- \(\sigma_y = 29 \, \text{ksi}\)
- \(\tau_{xy} = 8 \, \text{ksi}\)
Determine the state of stress on an element rotated \(50^\circ\) counterclockwise from the element shown.
**Instructions:**
Express your answers, separated by commas, to three significant figures.
**Input Box:**
The user is prompted to enter the rotated stress values in the form: \(\sigma_x', \sigma_y', \tau_{xy}'\) in \(\text{ksi}\).
## Part D - Clockwise Rotation of a Stress Element with No Shear Stress
The state of stress at a point in a member is shown on the rectangular stress element in **Figure 6**, where the magnitudes of the stresses are:
- \(\sigma_x' = 13 \, \text{ksi}\)
- \(\sigma_y' = 12 \, \text{ksi}\)
---
## Figure Explanation
The illustrated figure represents a rectangular stress element with applied stresses, depicted as follows:
- The horizontal and vertical arrows indicate normal stresses \(\sigma_x\) and \(\sigma_y\).
- The diagonal arrow represents shear stress \(\tau_{xy}\).
The element serves as a visual aid for understanding how stresses act on different planes of the element and how they transform when the element is rotated.
This educational resource supports the understanding of stress transformations in mechanics of materials.
Expert Solution
Step 1: Determining the given data
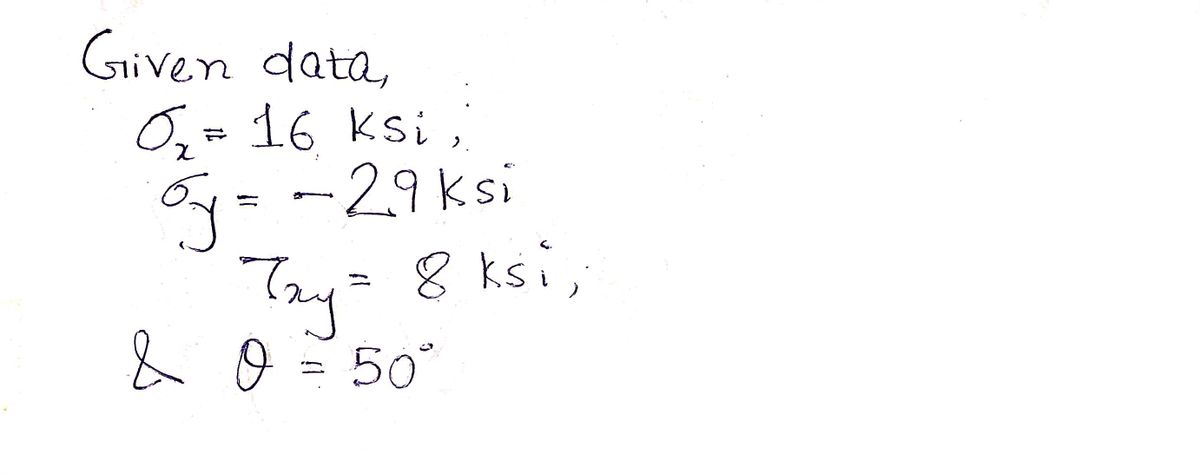
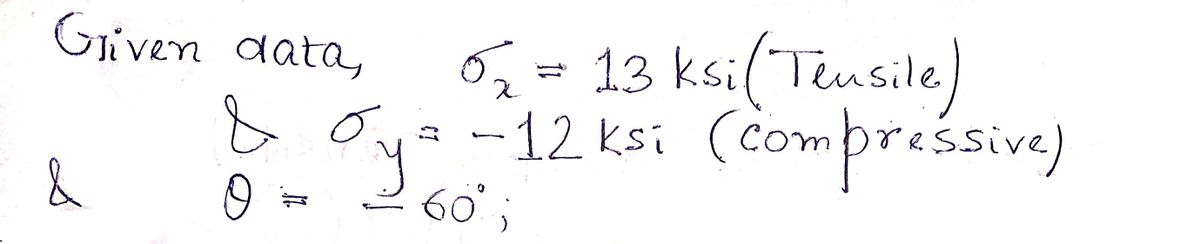
Step by step
Solved in 4 steps with 5 images

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Recommended textbooks for you

Elements Of Electromagnetics
Mechanical Engineering
ISBN:
9780190698614
Author:
Sadiku, Matthew N. O.
Publisher:
Oxford University Press

Mechanics of Materials (10th Edition)
Mechanical Engineering
ISBN:
9780134319650
Author:
Russell C. Hibbeler
Publisher:
PEARSON

Thermodynamics: An Engineering Approach
Mechanical Engineering
ISBN:
9781259822674
Author:
Yunus A. Cengel Dr., Michael A. Boles
Publisher:
McGraw-Hill Education

Elements Of Electromagnetics
Mechanical Engineering
ISBN:
9780190698614
Author:
Sadiku, Matthew N. O.
Publisher:
Oxford University Press

Mechanics of Materials (10th Edition)
Mechanical Engineering
ISBN:
9780134319650
Author:
Russell C. Hibbeler
Publisher:
PEARSON

Thermodynamics: An Engineering Approach
Mechanical Engineering
ISBN:
9781259822674
Author:
Yunus A. Cengel Dr., Michael A. Boles
Publisher:
McGraw-Hill Education

Control Systems Engineering
Mechanical Engineering
ISBN:
9781118170519
Author:
Norman S. Nise
Publisher:
WILEY

Mechanics of Materials (MindTap Course List)
Mechanical Engineering
ISBN:
9781337093347
Author:
Barry J. Goodno, James M. Gere
Publisher:
Cengage Learning

Engineering Mechanics: Statics
Mechanical Engineering
ISBN:
9781118807330
Author:
James L. Meriam, L. G. Kraige, J. N. Bolton
Publisher:
WILEY