worker, and the company decides to eliminate this effect by selecting 12 workers at random and timing each worker on each assembly process. rkers are chosen at random to use Process 1 first, and the rest use Process 2 first. For each worker and each process, the assembly time (in min corded, as shown in the table below. Worker Process 1 Process 2 Difference (Process 1 - Process 2) Send data to calculator 1 90 Explanation 78 Check 12 2 41 22 19 3 N 40 32 8 4 65 38 5 65 52 (a) State the null hypothesis Ho and the alternative hypothesis H₁. H₁ :0 H₁ :0 Start 2 6 35 7 7 8 32 38 39 23 9 76 10 43 38 33 11 55 27 13 28 -7 15 38 10 -4 59 Based on these data, can the company conclude, at the 0.05 level of significance, that the mean assembly times for the two processes differ? Answer question by performing a hypothesis test regarding H (which is u with a letter "d" subscript), the population mean difference in assembly times for t processes. Assume that this population of differences (Process 1 minus Process 2) is normally distributed. Perform a two-tailed test. Then complete the parts below. Carry your intermediate computations to three or more decimal places and round your answ specified. (If necessary, consult a list of formulas.) 12 LL 81 X 50 31 O S 2022 McGraw Hill LLC. All Rights Reserved. P ê Terms of Use | Priva
worker, and the company decides to eliminate this effect by selecting 12 workers at random and timing each worker on each assembly process. rkers are chosen at random to use Process 1 first, and the rest use Process 2 first. For each worker and each process, the assembly time (in min corded, as shown in the table below. Worker Process 1 Process 2 Difference (Process 1 - Process 2) Send data to calculator 1 90 Explanation 78 Check 12 2 41 22 19 3 N 40 32 8 4 65 38 5 65 52 (a) State the null hypothesis Ho and the alternative hypothesis H₁. H₁ :0 H₁ :0 Start 2 6 35 7 7 8 32 38 39 23 9 76 10 43 38 33 11 55 27 13 28 -7 15 38 10 -4 59 Based on these data, can the company conclude, at the 0.05 level of significance, that the mean assembly times for the two processes differ? Answer question by performing a hypothesis test regarding H (which is u with a letter "d" subscript), the population mean difference in assembly times for t processes. Assume that this population of differences (Process 1 minus Process 2) is normally distributed. Perform a two-tailed test. Then complete the parts below. Carry your intermediate computations to three or more decimal places and round your answ specified. (If necessary, consult a list of formulas.) 12 LL 81 X 50 31 O S 2022 McGraw Hill LLC. All Rights Reserved. P ê Terms of Use | Priva
MATLAB: An Introduction with Applications
6th Edition
ISBN:9781119256830
Author:Amos Gilat
Publisher:Amos Gilat
Chapter1: Starting With Matlab
Section: Chapter Questions
Problem 1P
Related questions
Question
100%

Transcribed Image Text:### Comparing Assembly Times for Keyboard Manufacturing Processes
A company is investigating whether there is a difference in assembly times between two keyboard assembly processes. Since assembly times can vary significantly from one worker to another, the company selects 12 workers at random to control this variability. For each worker, assembly times are recorded for both processes. The table below shows these times in minutes:
| Worker | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 |
|--------------|----|----|----|----|----|----|----|----|----|----|----|----|
| Process 1 | 90 | 41 | 42 | 78 | 45 | 39 | 32 | 91 | 30 | 75 | 45 | 81 |
| Process 2 | 78 | 22 | 32 | 38 | 52 | 43 | 35 | 30 | 40 | 65 | 49 | 50 |
| Difference (Process 1 - Process 2) | 12 | 19 | 10 | 40 | -7 | -4 | -3 | 61 | -10 | 10 | -4 | 31 |
### Hypothesis Testing
Using these data, the company wants to determine if there is a statistically significant difference in assembly times between the two processes. They will do this at the 0.05 level of significance.
#### Steps:
1. **Formulate Hypotheses:**
- Null Hypothesis \( H_0 \): The mean difference in assembly times between the two processes is zero (\(\mu_d = 0\)).
- Alternative Hypothesis \( H_1 \): The mean difference in assembly times between the two processes is not zero (\(\mu_d \neq 0\)).
2. **Perform a Two-tailed Test:**
- Assume the population of differences is normally distributed.
- Calculate necessary statistics and test the hypothesis.
3. **Conclude:**
- Interpret the results based on the p-value or critical value approach.
Given these methods and data, the company will be able to ascertain whether the two processes significantly differ in terms of assembly time.

Transcribed Image Text:### Hypothesis Testing Exercise
This exercise guides you through the steps of hypothesis testing using a specified significance level. Below are the steps involved:
#### (a) State the Hypotheses
- **Null Hypothesis (H₀):**
- Input box for defining the null hypothesis.
- **Alternative Hypothesis (H₁):**
- Input box for defining the alternative hypothesis.
#### (b) Determine the Type of Test Statistic
- **Type of Test Statistic:**
- Dropdown menu for selecting the appropriate test statistic to use.
#### (c) Find the Test Statistic
- Calculate the value of the test statistic and round it to three or more decimal places.
- Input box for entering the calculated value.
#### (d) Find Critical Values
- Determine the two critical values at a 0.05 level of significance, rounded to three or more decimal places.
- Input boxes for entering the two critical values.
#### (e) Conclusion at 0.05 Level
- Question: At the 0.05 level, can the company conclude that the mean assembly times for the two processes differ?
- Options: Yes / No (radio buttons)
### Symbols and Tools
- The right panel includes various symbols and tools useful for input such as:
- Greek letters (e.g., μ, σ)
- Mathematical operators (e.g., ≤, ≠)
- Statistical symbols (e.g., x̄, s)
This exercise helps in understanding the practical application of hypothesis testing by identifying null and alternative hypotheses, selecting the correct test statistic, calculating the test statistic value, finding critical values, and making conclusions based on statistical evidence.
© 2022 McGraw Hill LLC. All Rights Reserved. Terms of Use
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution!
Trending now
This is a popular solution!
Step by step
Solved in 2 steps with 1 images

Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
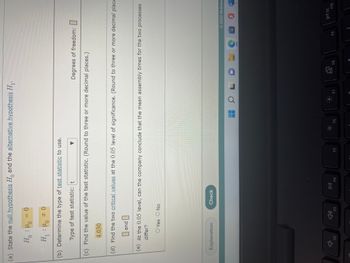
Transcribed Image Text:(a) State the null hypothesis and the alternative hypothesis H₁
H₁ = μ₁ = 0
H₁: Po # 0
1
(b) Determine the type of test statistic to use.
Type of test statistic: t
(c) Find the value of the test statistic. (Round to three or more decimal places.)
4.030
(d) Find the two critical values at the 0.05 level of significance. (Round to three or more decimal place
and
(e) At the 0.05 level, can the company conclude that the mean assembly times for the two processes
differ?
F2
OYes O No
Explanation
F3
Check
DII
F4
Degrees of freedom:
F5
F6
OLO
F7
8
F8
g
F9
Ⓒ2022 McGraw
prt sc
W
F10
Solution
Recommended textbooks for you

MATLAB: An Introduction with Applications
Statistics
ISBN:
9781119256830
Author:
Amos Gilat
Publisher:
John Wiley & Sons Inc

Probability and Statistics for Engineering and th…
Statistics
ISBN:
9781305251809
Author:
Jay L. Devore
Publisher:
Cengage Learning

Statistics for The Behavioral Sciences (MindTap C…
Statistics
ISBN:
9781305504912
Author:
Frederick J Gravetter, Larry B. Wallnau
Publisher:
Cengage Learning

MATLAB: An Introduction with Applications
Statistics
ISBN:
9781119256830
Author:
Amos Gilat
Publisher:
John Wiley & Sons Inc

Probability and Statistics for Engineering and th…
Statistics
ISBN:
9781305251809
Author:
Jay L. Devore
Publisher:
Cengage Learning

Statistics for The Behavioral Sciences (MindTap C…
Statistics
ISBN:
9781305504912
Author:
Frederick J Gravetter, Larry B. Wallnau
Publisher:
Cengage Learning

Elementary Statistics: Picturing the World (7th E…
Statistics
ISBN:
9780134683416
Author:
Ron Larson, Betsy Farber
Publisher:
PEARSON

The Basic Practice of Statistics
Statistics
ISBN:
9781319042578
Author:
David S. Moore, William I. Notz, Michael A. Fligner
Publisher:
W. H. Freeman

Introduction to the Practice of Statistics
Statistics
ISBN:
9781319013387
Author:
David S. Moore, George P. McCabe, Bruce A. Craig
Publisher:
W. H. Freeman