
Concept explainers
Two cover plates are welded to the rolled-steel beam as shown. Using E = 29 × 106 psi, determine (a) the slope at end C, (b) the deflection at end C.
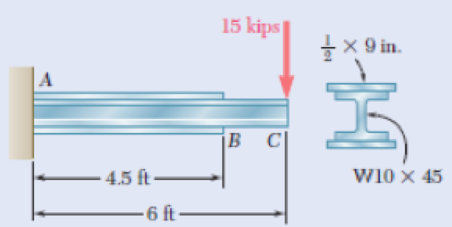
Fig. P9.108
(a)
Find the slope
Answer to Problem 108P
The slope
Explanation of Solution
Given information:
The elastic modulus (E) is
The section of the beam is
The dimension of the top plate and bottom plate is
Calculation:
Refer Appendix C, “Properties of Rolled steel shapes”.
The moment of inertia (I) for the given section is
The depth of the section (D) is
The width of the section (b) is
Use moment area method:
Consider from bottom.
Calculate the neutral axis
Substitute
Top plate:
Calculate the area of the top plate
Since the dimension of the top plate is
Calculate the depth of neutral axis (d) using the formula:
Substitute
Calculate the product of
Substitute
Calculate the moment of inertia (I) using the formula:
Here, b is the width the top plate and h is the height of the top plate.
Substitute
Bottom plate:
Top plate:
Calculate the area of the bottom plate
Since the dimension of the bottom plate is
Calculate the depth of neutral axis (d) using the formula:
Substitute
Calculate the product of
Substitute
Calculate the moment of inertia (I) using the formula:
Here, b is the width the top plate and h is the height of the top plate.
Substitute
Tabulate the calculated values and compute the moment of inertia (I) as in Table (1).
| Segments | Area, A | Depth, d (in.) | ||
| Top plate | 4.5 | 5.3 | 126.405 | 0.09375 |
| 248 | ||||
| Bottom plate | 4.5 | 5.3 | 126.405 | 0.09375 |
| Summation | 252.81 | 248 |
Take the greater value of moment of inertia from the three segments is
Calculate the moment of inertia (I) using the relation:
Substitute
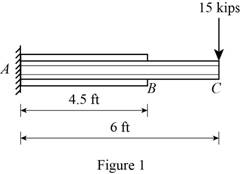
Show the free body diagram of beam by considering the point load as in Figure 1.
Draw the moment diagram of the above beam as in Figure 2.
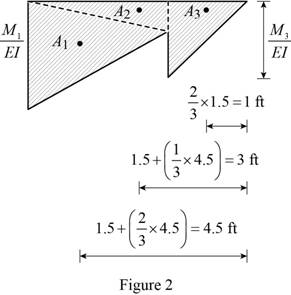
Calculate the moment
Calculate the ratio of
Substitute
Calculate the area
Here,
Substitute 4.5 ft for
Calculate the area
Substitute
Calculate the moment
Calculate the ratio of
Substitute
Calculate the area
Here,
Substitute 1.5 m for
Show the tangent slope and deflection at point C related to reference tangent as in Figure 3.
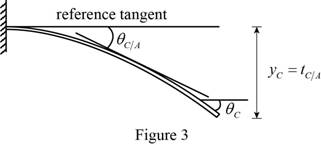
Since the support A has fixed support, the slope
Calculate the slope at the end C related to the fixed end A
Substitute
Calculate the slope at the point C
Substitute 0 for
Thus, the slope
(b)
Find the deflection
Answer to Problem 108P
The deflection
Explanation of Solution
Given information:
The elastic modulus (E) is
The section of the beam is
The dimension of the top plate and bottom plate is
Calculation:
Calculate the deflection at end C related to the fixed end A
Substitute
Calculate the deflection at the point C
Substitute 0 for
Thus, the deflection
Want to see more full solutions like this?
Chapter 9 Solutions
EBK MECHANICS OF MATERIALS
- [Q2]: The cost information supplied by the cost accountant is as follows:Sales 20,00 units, $ 10 per unitCalculate the (a/ newsale guantity and (b) new selling price to earn the sameVariable cost $ 6 per unit, Fixed Cost $ 30,000, Profit $ 50,000profit ifi) Variable cost increases by $ 2 per unitil) Fixed cost increase by $ 10,000Ili) Variable cost increase by $ 1 per unit and fixed cost reduces by $ 10,000arrow_forwardcan you please help me perform Visual Inspection and Fractography of the attatched image: Preliminary examination to identify the fracture origin, suspected fatigue striation, and corrosion evidences.arrow_forwardcan you please help[ me conduct Causal Analysis (FTA) on the scenario attatched: FTA diagram which is a fault tree analysis diagram will be used to gain an overview of the entire path of failure from root cause to the top event (i.e., the swing’s detachment) and to identify interactions between misuse, material decay and inspection errors.arrow_forward
- hi can you please help me in finding the stress intensity factor using a k-calcluator for the scenario attathced in the images.arrow_forwardHi, can you please help me .Identify and justify suitable analytical techniques of the scenario below, bearing in mind the kinds of information being handled to reach a conclusion (methodology). A child swing set was discovered to have failed at the fixing at the top of the chains connecting the seat to the top of the swing set. A 12 mm threaded steel bolt, connecting the shackle to the top beam, failed at the start of the threaded region on the linkage closest to the outside side of the swing set . The linkage and bolts were made of electro galvanised mild steel . The rigid bar chain alternatives and fixings were of the same material and appeared to be fitted in accordance with guidelines. The yield strength of the steel used is 260 MPa and the UTS is 380 MPa. The bolt that failed was threaded using a standard thread with a pitch (distance between threads) of 1.75 mm and a depth of approximately 1.1 mm. The swing set in question had been assigned to ‘toddlers’ with the application of…arrow_forwardHi, can you please define and calculate the failure mode of the linkage that failed on the swing (images added) : A child swing set was discovered to have failed at the fixing at the top of the chains connecting the seat to the top of the swing set. A 12 mm threaded steel bolt, connecting the shackle to the top beam, failed at the start of the threaded region on the linkage closest to the outside side of the swing set . The linkage and bolts were made of electro galvanised mild steel . The rigid bar chain alternatives and fixings were of the same material and appeared to be fitted in accordance with guidelines. The yield strength of the steel used is 260 MPa and the UTS is 380 MPa. The bolt that failed was threaded using a standard thread with a pitch (distance between threads) of 1.75 mm and a depth of approximately 1.1 mm. The swing set in question had been assigned to ‘toddlers’ with the application of a caged-type seat. However, the location was within the play area not…arrow_forward
- Page 11-68. The rectangular plate shown is subjected to a uniaxial stress of 2000 psi. Compute the shear stress and the tensile developed on a plane forming an angle of 30° with the longitud axis of the member. (Hint: Assume a cross-sectional area of unity) 2000 psi 2000 psi hparrow_forward11-70. A shear stress (pure shear) of 5000 psi exists on an element. (a) Determine the maximum tensile and compressive stresses caused in the element due to this shear. (b) Sketch the element showing the planes on which the maximum tensile and compressive stresses act.arrow_forward11-20. An aluminum specimen of circular cross section, 0.50 in. in diameter, ruptured under a tensile load of 12,000 lb. The plane of failure was found to be at 48° with a plane perpendicular to the longitudinal axis of the specimen. (a) Compute the shear stress on the failure plane. (b) Compute the maximum tensile stress. (c) Compute the tensile stress on the failure plane. hparrow_forward
- A long flat steel bar 13 mm thick and 120 mm wide has semicircular grooves as shown and carries a tensile load of 50 kN Determine the maximum stress if plate r= 8mm r=21mm r=38mmarrow_forwardProblem 13: F₁ = A =250 N 30% Determine the moment of each of the three forces about point B. F₂ = 300 N 60° 2 m -3 m B 4 m F3=500 Narrow_forward3 kN 3 kN 1.8 kN/m 80 mm B 300 mm D an 1.5 m-1.5 m--1.5 m- PROBLEM 5.47 Using the method of Sec. 5.2, solve Prob. 5.16 PROBLEM 5.16 For the beam and loading shown, determine the maximum normal stress due to bending on a transverse section at C.arrow_forward
 Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY





