Chapter 9.4, Problem 36ES

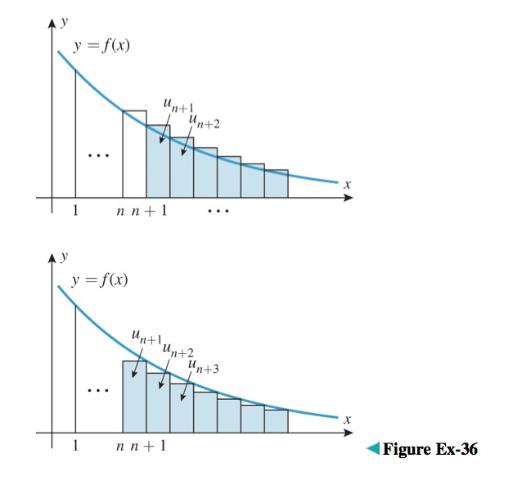
Exercise 36 will show how a partial sum can be used to obtain upper and lower bounds on the sum of a series when the hypotheses of the integral test are satisfied. This result will be needed in Exercises 37-40.

(a) Let ${\displaystyle {\sum }_{k=1}^{\infty }{u}_k}$ be a convergent series with positive terms, and let $f$ be a function that is decreasing and continuous on $\left[n,+\infty \right)$ and such that $u_k=f\left(k\right)\text{for}k\ge n.$ Use an area argument and the accompanying figure to show that ${\displaystyle {\int }_{n+1}^{+\infty }f\left(x\right)dx}<{\displaystyle \underset{k=n+1}{\overset{\infty }{\sum }}{u}_k}<{\displaystyle {\int }_n^{+\infty }f\left(x\right)dx}$

(b) Show that if S is the sum of the series ${\displaystyle {\sum }_{k=1}^{\infty }{u}_k}$ and $s_n$ is the $n\text{th}$ partial sum, then $s_n+{\displaystyle {\int }_{n+1}^{+\infty }f\left(x\right)dx<S<s_n+{\displaystyle {\int }_n^{+\infty }f\left(x\right)}}dx$