Chapter 9.1, Problem 10CYU

To determine

To Find:

The vertex , axis of symmetry and y − intercept.

Answer to Problem 10CYU

Vertex : (1,2)

Axis of Symmetry : x = 1

y-intercept : 1

Explanation of Solution

Given Information:

Given equation is :

  $y=-x^2+2x+1$

Concept Used :

• Quadratic Functions:
• Quadratic functions are non-linear and are of the form:

  $f(x)=a{x}^2+bx+c;a\ne 0$

This is the standard form of Quadratic function.

• Shape of the graph of a Quadratic function is called Parabola.
• Parabolas are symmetric about a central line called Axis Of Symmetry.
• The axis of symmetry intersects a parabola only at one point , called the Vertex.
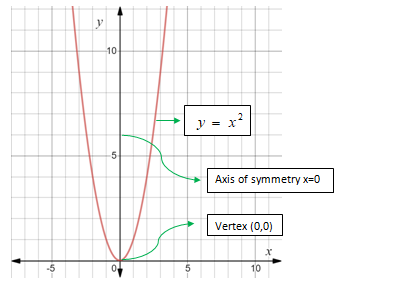
• Parent Function:

  $y=x^2$

• Standard Form :

  $f(x)=a{x}^2+bx+c;a\ne 0$

• Type of Graph:

Parabola

• Axis of Symmetry: Passes through the vertex and divides the parabola into two congruent halves.

  $x=-\frac{b}{2a}$

• y-intercept = The y coordinate of the point at which the graph intersects y-axis = c
• Graph:

  

• If a > 0 ,
• The graph of $a{x}^2+bx+c$ opens upward.
• The lowest point on the graph is the minimum.
• If a < 0 ,
• The graph of $a{x}^2+bx+c$ opens downward.
• The highest point on the graph is the maximum.
• The maximum or the minimum is the vertex.

Calculation:

• In the equation $y=-x^2+2x+1$ ; comparing it with standard form of quadratic equation , we have a = -1 , b = 2 , c = 1 .
• Find Axis of Symmetry :

  $\begin{array}{l}y=a{x}^2+bx+c\mathrm{...........................}[s\tan dard\_form]\\ x=-\frac{b}{2a}\mathrm{.....................................}[formula\_of\_axis\_of\_symmetry]\\ x=-\frac{2}{2(-1)}=\mathrm{1..........................}[a=-1,b=2]\end{array}$

The equation of axis of symmetry is x = 1

• Find the Vertex :

Since axis of symmetry passes through the vertex , we have x coordinate of the vertex (x,y) is x=1

Substituting the value of x =1 in the equation $y=-x^2+2x+1$

  $\begin{array}{l}y=-x^2+2x+1\\ $ y=-{(1)}^2+2(1)+\mathrm{1.....................}[x=1]$y=-1+2+\mathrm{1................................}[simplify]\\ y=2\end{array}$

The vertex is at (1,2)

• Find y-intercept :

The y-coordinate of the point at which the graph cuts the y-axis is the y-intercept.

Hence , substituting x=0 in the equation $y=-x^2+2x+1$ , we will get the y-intercept.

  $\begin{array}{l}y=-x^2+2x+1\\ $ y=-{(0)}^2+2(0)+\mathrm{1.....................}[x=0]$y=1\end{array}$

Or

y-intercept always occurs at (0,c) and c = 1 here.

So, y-intercept = 1 and is located at (0,1)