
The expression for the steady state displacement x(t)for a cam.
The steady stat response for the given Fourier series is given by
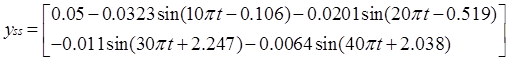
Given:
The displacement produced by the cam is given by the following figure
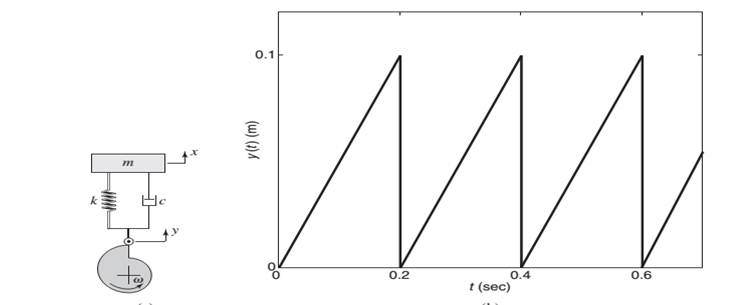
Figure 1 Figure 2
Where m = 1 Kg, 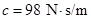 and k = 4900N/m.
and k = 4900N/m.
Fourier series approximation = 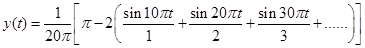
Concept Used:
We first derive an expression for transfer function of the given system and then calculate 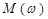 magnitude of the given transfer function, phase angle, bandwidth, and magnitude of phase angles of respective frequencies, and finally calculate the expression for steady state response.
magnitude of the given transfer function, phase angle, bandwidth, and magnitude of phase angles of respective frequencies, and finally calculate the expression for steady state response.
Calculation:
Fourier series approximation = 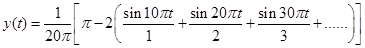
From Figure 1 equation of motion is given by,
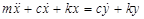 (1)
(1)
Substituting for m = 1 Kg, 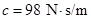 and k = 4900N/m in equation (1)
and k = 4900N/m in equation (1)
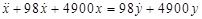
Applying Laplace transform for the above equation
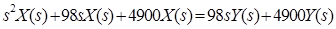
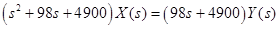
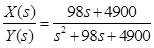
The transfer function for the given system
T(s) 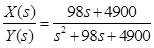 (2)
(2)
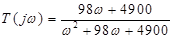
Therefore magnitude of above transfer function is given by
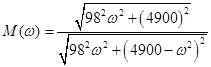 (3)
(3)
The phase angle is given by
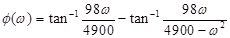 (4)
(4)
From the equation (3) we can observe that 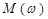 attains a peak of 1 when
attains a peak of 1 when 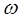 0. Hence
0. Hence 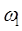 = 0 and
= 0 and 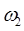 is calculated as follows
is calculated as follows
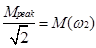
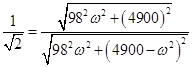
Squaring on both sides we get,
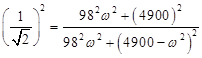
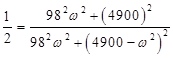
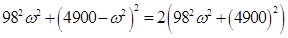
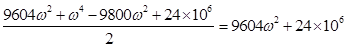
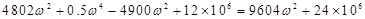
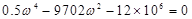
On further simplification we get
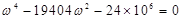
The roots of the equation are 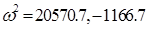
Taking only the positive value 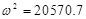
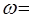 143.42 rad/s
143.42 rad/s
Therefore, the bandwidth lies between 0 and 143.42 rad/s that is 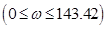
As 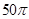 is greater than 143.42 rad/s which is outside the required bandwidth, we consider only 0, 10
is greater than 143.42 rad/s which is outside the required bandwidth, we consider only 0, 10 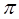 20
20 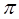 30
30 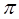 and 40
and 40 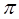 for frequency values.
for frequency values.
From equation (3) 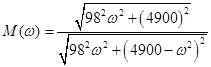
Substituting for 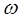 0, 10
0, 10 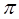 20
20 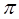 30
30 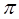 and 40
and 40 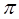 respectively we get
respectively we get

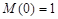 .... (5)
.... (5)
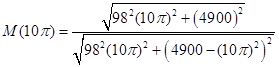
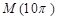 =
= 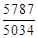
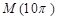 =1.015
=1.015
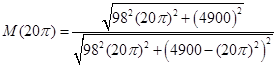
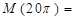
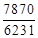
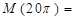 1.263
1.263
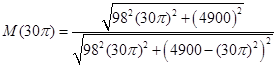

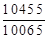
 1.038
1.038
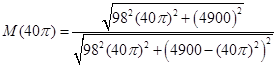
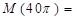
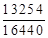
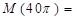 0.806
0.806
From equation (4) we have 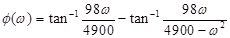
Now calculating the phase angles for corresponding frequencies 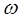 0, 10
0, 10 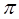 20
20 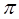 30
30 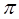 and 40
and 40 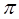 respectively we get,
respectively we get,
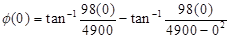
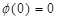 rad
rad
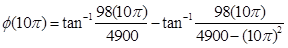
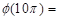
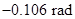
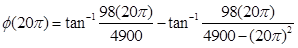
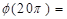
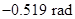
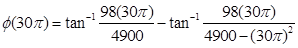
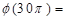 2.247 rad
2.247 rad
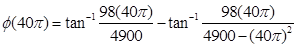
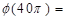 2.038 rad
2.038 rad
The steady state response for the given Fourier series
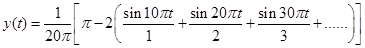
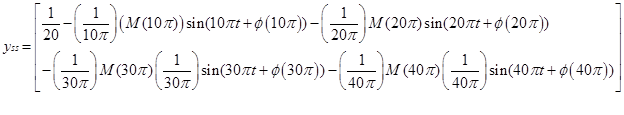
Substituting the values of 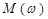 and
and 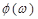 for corresponding frequencies
for corresponding frequencies 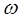 0, 10
0, 10 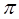 20
20 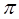 30
30 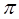 and 40
and 40 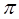 respectively we get,
respectively we get,
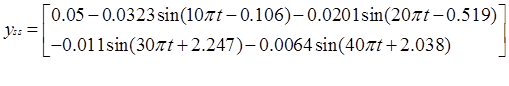
Conclusion:
Therefore, the steady stat response for the given function is given by
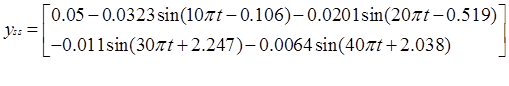
Answer to Problem 9.33P
The steady stat response for the given Fourier series is given by
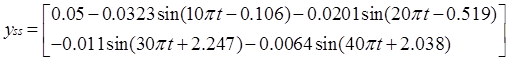
Explanation of Solution
Given:
The displacement produced by the cam is given by the following figure

Figure 1 Figure 2
Where m = 1 Kg, 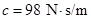 and k = 4900N/m.
and k = 4900N/m.
Fourier series approximation = 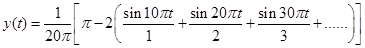
Concept Used:
We first derive an expression for transfer function of the given system and then calculate  magnitude of the given transfer function, phase angle, bandwidth, and magnitude of phase angles of respective frequencies, and finally calculate the expression for steady state response.
magnitude of the given transfer function, phase angle, bandwidth, and magnitude of phase angles of respective frequencies, and finally calculate the expression for steady state response.
Calculation:
Fourier series approximation = 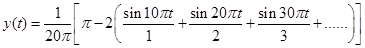
From Figure 1 equation of motion is given by,
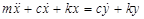 (1)
(1)
Substituting for m = 1 Kg, 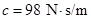 and k = 4900N/m in equation (1)
and k = 4900N/m in equation (1)
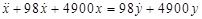
Applying Laplace transform for the above equation
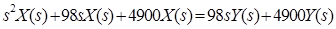
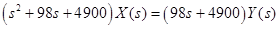
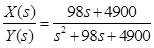
The transfer function for the given system
T(s) 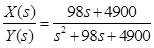 (2)
(2)
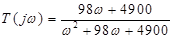
Therefore magnitude of above transfer function is given by
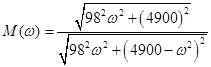 (3)
(3)
The phase angle is given by
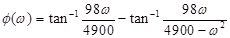 (4)
(4)
From the equation (3) we can observe that 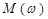 attains a peak of 1 when
attains a peak of 1 when 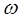 0. Hence
0. Hence 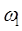 = 0 and
= 0 and 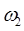 is calculated as follows
is calculated as follows
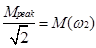
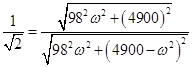
Squaring on both sides we get,
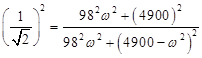
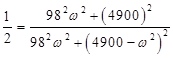
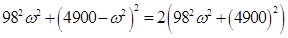
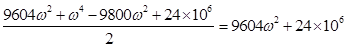
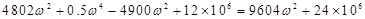
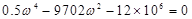
On further simplification we get
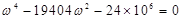
The roots of the equation are 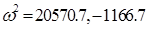
Taking only the positive value 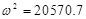
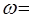 143.42 rad/s
143.42 rad/s
Therefore, the bandwidth lies between 0 and 143.42 rad/s that is 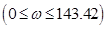
As 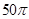 is greater than 143.42 rad/s which is outside the required bandwidth, we consider only 0, 10
is greater than 143.42 rad/s which is outside the required bandwidth, we consider only 0, 10 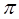 20
20 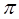 30
30 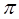 and 40
and 40 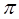 for frequency values.
for frequency values.
From equation (3) 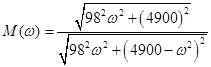
Substituting for 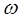 0, 10
0, 10 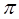 20
20 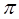 30
30 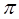 and 40
and 40 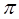 respectively we get
respectively we get

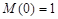 .... (5)
.... (5)
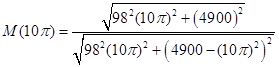
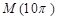 =
= 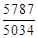
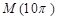 =1.015
=1.015
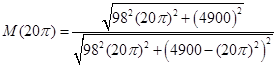
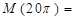
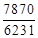
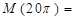 1.263
1.263
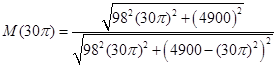

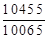
 1.038
1.038
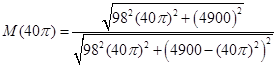
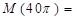
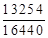
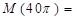 0.806
0.806
From equation (4) we have 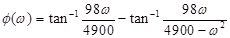
Now calculating the phase angles for corresponding frequencies 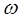 0, 10
0, 10 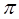 20
20 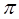 30
30 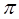 and 40
and 40 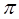 respectively we get,
respectively we get,
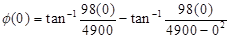
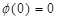 rad
rad
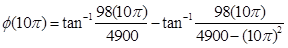
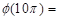
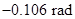
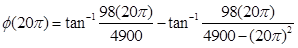
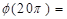
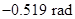
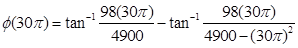
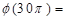 2.247 rad
2.247 rad
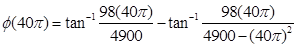
 2.038 rad
2.038 rad
The steady state response for the given Fourier series
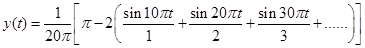
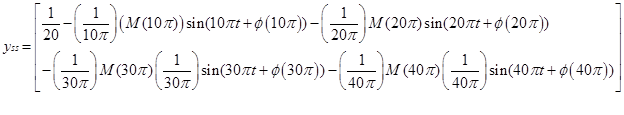
Substituting the values of 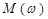 and
and 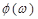 for corresponding frequencies
for corresponding frequencies 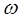 0, 10
0, 10 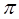 20
20 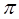 30
30 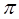 and 40
and 40 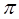 respectively we get,
respectively we get,
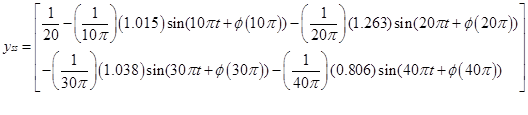
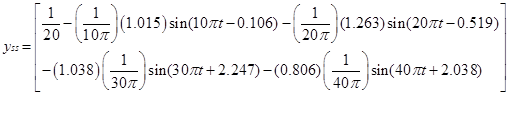
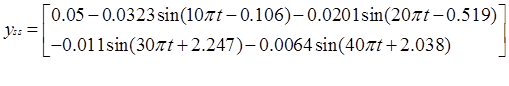
Conclusion:
Therefore, the steady stat response for the given function is given by
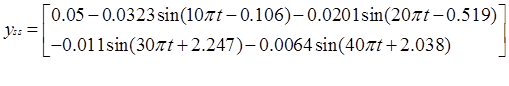
Want to see more full solutions like this?
Chapter 9 Solutions
EBK SYSTEM DYNAMICS
- I want the kinematic diagram to be draw like this plsarrow_forwardAccording to the principles and steps above, draw the kinematic diagram of following mechanisms. Mark the appropriate scale, calculates the degree of freedom. NO.1 NO.2 NO: 3 NO.: 4arrow_forwardAn office building is planned with a lateral-force-resisting system designed for earthquake resistance in aseismic zone. The seismic capacity of the proposed system, expressed as a force factor, is assumed tofollow a lognormal distribution with a median of 6.5 and a standard deviation of 1.5. The ground motionfrom the largest expected earthquake at the site is estimated to correspond to an equivalent force factor of 5.5.(a) What is the estimated probability that the building will experience damage when subjected to the largest expected earthquake? (b) If the building survives (i.e., experiences no damage) during a previous moderate earthquake with aforce factor of 4.0, what is the updated probability of failure of the building under the largest expectedearthquake?(c) Suppose future occurrences of the largest expected earthquake follow a Poisson process with a mean return period of 500 years. Assuming that damage events from different earthquakes are statisticallyindependent,…arrow_forward
- During a plant visit, it was noticed that a 12-m-long section of a 10-cm-diameter steam pipe is completely exposed to the ambient air. The temperature measurements indicate that the average temperature of the outer surface of the steam pipe is 75°C when the ambient temperature is 5°C. There are also light winds in the area at 10 km/h. The emissivity of the outer surface of the pipe is 0.8, and the average temperature of the surfaces surrounding the pipe, including the sky, is estimated to be 0°C. Determine the amount of heat lost from the steam during a 10-h-long work day. Steam is supplied by a gas-fired steam generator that has an efficiency of 80 percent, and the plant pays $1.05/therm of natural gas. If the pipe is insulated and 90 percent of the heat loss is saved, determine the amount of money this facility will save a year as a result of insulating the steam pipes. Assume the plant operates every day of the year for 10 h. State your assumptions.arrow_forwardAn old fashioned ice cream kit consists of two concentric cylinders of radii Ra and Rb. The inner cylinder is filled with milk and ice cream ingredients while the space between the two cylinders is filled with an ice-brine mixture. Ice cream begins to form on the inner surface of the inner cylinder. To expedite the process, would you recommend rotating the inner cylinder? Justify your recommendation. icecream/ ice-brine Ra Rbarrow_forwardFind temperatures STRICTLY USING RITZ APPROXIMATION METHODarrow_forward
- Solve this Problem using RITZ APPROXIMATION. STEP BY STEParrow_forwardB/40 The body is constructed of a uniform square plate, a uniform straight rod, a uniform quarter‐circular rod, and a particle (negligible dimensions). If each part has the indicated mass, determine the mass moments of inertia of the body about the x‐, y‐, and z‐axes. Answer Given.arrow_forward(read image) Answer:arrow_forward
- (read image) Answer Givenarrow_forwardB/16. The plane area shown in the top portion of the figure is rotated 180° about the x‐axis to form the body of revolution of mass m shown in the lower portion of the figure. Determine the mass moment of inertia of the body about the x‐axis. Answer Givenarrow_forward(read image) Answer:arrow_forward
 Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY





