
The profile of a gear tooth shown in Fig. P9.1 (a) is approximated by the
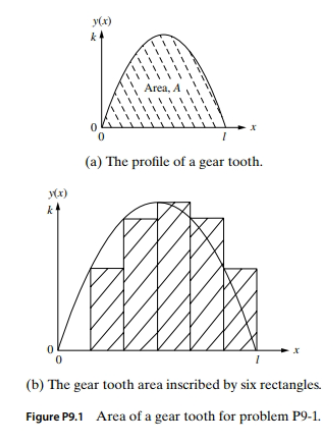
(a) Estimate the area A using six rectangles of equal width
(b) Calculate the exact area by evaluating the definite
(a)
The estimated area of given function
Answer to Problem 1P
The estimated area of given function by rectangle method is
Explanation of Solution
Given:
The expression of profile of gear tooth is given as:
Number of rectangles given are 6.
Width of the rectangle is given as:
Concept used:
The expression of area is given as:
Here,
Calculation:
For
Substitute
Therefore,
For
Substitute
Therefore,
For
Substitute
Therefore,
For
Substitute
Therefore,
For
Substitute
Therefore,
Substitute
Therefore,
Substitute
Equation (3) can be written as:
Substitute
Conclusion:
Thus, the estimated area of the given function by rectangle method is
(b)
The exact area of given function
Answer to Problem 1P
The exact area of given function by integration method is
Explanation of Solution
Concept used:
The expression of area is given as:
Here,
Calculation:
Substitute
Further simplify for
Conclusion:
Thus, the exact area of given function by integration method is
Want to see more full solutions like this?
Chapter 9 Solutions
Introductory Mathematics for Engineering Applications
Additional Math Textbook Solutions
Pre-Algebra Student Edition
Basic Business Statistics, Student Value Edition
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
Elementary and Intermediate Algebra: Concepts and Applications (7th Edition)
Elementary Statistics: Picturing the World (7th Edition)
Calculus: Early Transcendentals (2nd Edition)
- Main/84589a48-b934-4b6e-a6e1-a5d75f559df9?transferToken=MxNewDS NGIFSFh5aZ5MQMzuGzB.. Critics review films out of 5 based on three attributes: the story, the special effects and the acting. The ratings of four critics for a film are collected in the table below. B Special Critic Critic 1 Story rating Effects rating Acting rating 4.4 4.3 4.5 Critic 2 4.1 4.2 3.9 Critic 3 3.9 4 3.4 Critic 4 4.2 4.1 4.2 44° X Critic 1 also gave the film a rating for the Director's ability. If the average of Critic 1's ratings was 4.3 what rating did they give to the Director's ability? 3.9 4.0 OOOO Esc 4.1 4.2 Q Search L 2 A AR F2 +4 % x Ö- 1+ FS F4 F5 F6 F7 F8 £ %5 14 96 T 27 F9 화요 4 * 8 F10 U F11 61 UTROGENA apid Cle 2-in-1 & Fade Toner TOCLEAR FADES The look of ENG 17:51 UK 25/01/2025 TYLITTLETHING DY MIST ONIKI ALM # 공☆ Home End F12 Insert Delete +11 K Parrow_forwardeminder to complete the Case X T Civil Service Numerical Test Sec X C T Casework Skills Practice Test x + euauthoring.panpowered.com/DeliveryWeb/Civil Service Main/84589a48-b934-4b6e-a6e1-a5d75f559df9?transferToken=MxNewOS NGIFSFH5aZSMQMzuGzB The table shows the value of the FTSE 100 share index over 6-month periods for 2 years. Month Value (£) January 2017 7,147 July 2017 7,455 January 2018 7,692 July 2018 7,668 January 2019 7,154 If the value of shares held in the FTSE 100 was £2,450 in July 2017, what would it be in July 2018? £2,478 £2,505 O O O O C £2,520 £2,553 £2,560 EN Q Search பல் L -☀- F5 0+ F6 C F8 27 8 화요 * 0 F10 E 61 F12arrow_forward(c) Suppose that all the pages are black and white, but each group of 25 con- secutive pages (1-25, 26-50, 51-75, 76-100, 101-125) must be assigned to the same printer. Each printer can be assigned 0, 25, 50, 75, 100, or 125 pages to print. How many ways are there for the 125 pages to be assigned to the five printers?arrow_forward
- Ruff, Inc. makes dog food out of chicken and grain. Chicken has 10 grams of protein and 5 grams of fat per ounce, and grain has 2 grams of protein and 2 grams of fat per ounce. A bag of dog food must contain at least 222 grams of protein and at least 162 grams of fat. If chicken costs 11¢ per ounce and grain costs 1¢ per ounce, how many ounces of each should Ruff use in each bag of dog food to minimize cost? (If an answer does not exist, enter DNE.)arrow_forwardSolve the linear system of equations attached using Gaussian elimination (not Gauss-Jordan) and back subsitution. Remember that: A matrix is in row echelon form if Any row that consists only of zeros is at the bottom of the matrix. The first non-zero entry in each other row is 1. This entry is called aleading 1. The leading 1 of each row, after the first row, lies to the right of the leading 1 of the previous row.arrow_forward7. Show that for R sufficiently large, the polynomial P(z) in Example 3, Sec. 5, satisfies the inequality |P(z)| R. Suggestion: Observe that there is a positive number R such that the modulus of each quotient in inequality (9), Sec. 5, is less than |an|/n when |z| > R.arrow_forward
- 9. Establish the identity 1- 1+z+z² + 2n+1 ... +z" = 1- z (z1) and then use it to derive Lagrange's trigonometric identity: 1 1+ cos cos 20 +... + cos no = + 2 sin[(2n+1)0/2] 2 sin(0/2) (0 < 0 < 2л). Suggestion: As for the first identity, write S = 1+z+z² +...+z" and consider the difference S - zS. To derive the second identity, write z = eie in the first one.arrow_forward8. Prove that two nonzero complex numbers z₁ and Z2 have the same moduli if and only if there are complex numbers c₁ and c₂ such that Z₁ = c₁C2 and Z2 = c1c2. Suggestion: Note that (i≤ exp (101+0) exp (01-02) and [see Exercise 2(b)] 2 02 Ꮎ - = = exp(i01) exp(101+0) exp (i 01 - 02 ) = exp(102). i 2 2arrow_forwardnumerical anaarrow_forward
 Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning
Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning
 Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt





