
Concept explainers
a.
To write:
An equation describing the number of hours per month each type of plane should be rented if the flight school is to meet its goal.
a.
Answer to Problem 29E
The equation
Explanation of Solution
Given:
At a flight school, pilots in training can rent single-engine airplanes for $60 per hour and twin-engine airplanes for $180 per hour. The flight school’s goal is to take in $9000 in rental fee each month.
Calculation:
Let x represent number of hours for which single-engine airplanes were rented and y represent number of hours for which twin-engine airplanes were rented.
We have been given that pilots in training can rent single-engine airplanes for $60 per hour, so amount charged for x hours of single-engine airplanes would be 60x .
We have been given that pilots in training can rent twin-engine airplanes for $180 per hour, so amount charged for yhours of twin-engine airplanes would be 180x .
The total amount charged for renting all airplanes will be equal to $9000. We can represent this information in an equation as:
Therefore, the equation
b.
To graph:
An equation from part (a) using the intercepts.
b.
Answer to Problem 29E
x -intercept:
y -intercept:
Explanation of Solution
Given:
At a flight school, pilots in training can rent single-engine airplanes for $60 per hour and twin-engine airplanes for $180 per hour. The flight school’s goal is to take in $9000 in rental fee each month.
Calculation:
To find x -intercept, we will substitute
Therefore, the x -intercept of our given equation is
To find y- intercept, we will substitute
Therefore, the y -intercept of our given equation is
Upon graphing our given equation, we will get our required graph as shown below:
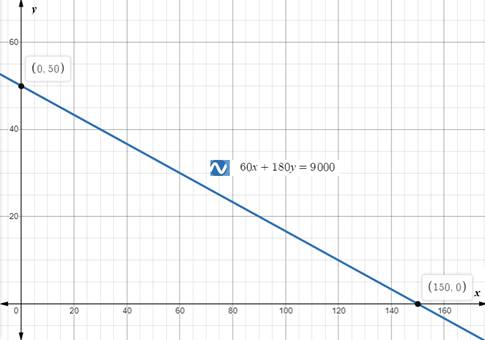
c.
To use:
Your graph to estimate how many hours the single-engine plane must be rented if the flight school is to meet its goal.
c.
Answer to Problem 29E
The single-engine planes must be rented for 60 hoursif the flight school is to meet its goal.
Explanation of Solution
Given:
During one month, the twin-engine airplanes were rented for 30 hours.
Calculation:
Upon looking at our graph, we can see that the value of x is 60, when
Therefore, the single-engine planes must be rented for 60 hoursif the flight school is to meet its goal.
d.
To check:
Your answer to part
d.
Answer to Problem 29E
The answer to part
Explanation of Solution
Given:
During one month, the twin-engine airplanes were rented for 30 hours.
Calculation:
To solve our given problem, we will substitute
Since we got same value, therefore, the single-engine planes must be rented for 60 hoursif the flight school is to meet its goal.
Chapter 8 Solutions
ELEMENTARY+INTERMEDIATE ALGEBRA
Additional Math Textbook Solutions
University Calculus: Early Transcendentals (4th Edition)
Intro Stats, Books a la Carte Edition (5th Edition)
Elementary Statistics (13th Edition)
Calculus: Early Transcendentals (2nd Edition)
College Algebra (7th Edition)
- Solve the following systems of equations and show all work.y = x2 + 3y = x + 5 Please type out answerarrow_forwardSolve the following system of equations. Show all work and solutions.y = 2x2 + 6x + 1y = −4x2 + 1 Please type out answerarrow_forwardDalia buys 20 collectible gems per month. Grace sells 10 gems from her collection of 120 each month. When will Dalia have more gems than Grace? Show your work. Dear Student If You Face any issue let me know i will solve your all doubt. I will provide solution again in more detail systematic and organized way. I would also like my last 3 questions credited to mearrow_forward
- Dalia buys 20 collectible gems per month. Grace sells 10 gems from her collection of 120 each month. When will Dalia have more gems than Grace? Show your work.arrow_forwardSolve the following system of equations. Show all work and solutions.y = 2x2 + 6x + 1y = −4x2 + 1arrow_forwardSolve the following systems of equations and show all work.y = x2 + 3y = x + 5arrow_forward
- Write an equation for the function shown. You may assume all intercepts and asymptotes are on integers. The blue dashed lines are the asymptotes. 10 9- 8- 7 6 5 4- 3- 2 4 5 15-14-13-12-11-10 -9 -8 -7 -6 -5 -4 -3 -2 1 1 2 3 -1 -2 -3 -4 1 -5 -6- -7 -8- -9 -10+ 60 7 8 9 10 11 12 13 14 15arrow_forwardUse the graph of the polynomial function of degree 5 to identify zeros and multiplicity. Order your zeros from least to greatest. -6 3 6+ 5 4 3 2 1 2 -1 -2 -3 -4 -5 3 4 6 Zero at with multiplicity Zero at with multiplicity Zero at with multiplicityarrow_forwardUse the graph to identify zeros and multiplicity. Order your zeros from least to greatest. 6 5 4 -6-5-4-3-2 3 21 2 1 2 4 5 ૪ 345 Zero at with multiplicity Zero at with multiplicity Zero at with multiplicity Zero at with multiplicity པ་arrow_forward
- Use the graph to write the formula for a polynomial function of least degree. -5 + 4 3 ♡ 2 12 1 f(x) -1 -1 f(x) 2 3. + -3 12 -5+ + xarrow_forwardUse the graph to identify zeros and multiplicity. Order your zeros from least to greatest. 6 -6-5-4-3-2-1 -1 -2 3 -4 4 5 6 a Zero at with multiplicity Zero at with multiplicity Zero at with multiplicity Zero at with multiplicityarrow_forwardUse the graph to write the formula for a polynomial function of least degree. 5 4 3 -5 -x 1 f(x) -5 -4 -1 1 2 3 4 -1 -2 -3 -4 -5 f(x) =arrow_forward
 Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSON
Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSON Contemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage Learning
Contemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage Learning Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning Algebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSON
Algebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSON Introduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge Press
Introduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge Press College Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education
College Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education





