Applied Statics and Strength of Materials (6th Edition)
6th Edition
ISBN: 9780133840544
Author: George F. Limbrunner, Craig D'Allaird, Leonard Spiegel
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 8, Problem 8.29SP
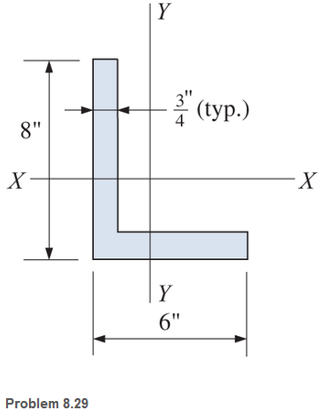
Calculate the moments of inertia of the area shown with respect to both cetroidal axes.
Expert Solution & Answer
Learn your wayIncludes step-by-step video
schedule13:15
Students have asked these similar questions
Determine the moment of Inertia of the quarter of the circle having a radius of 6m shown in the figure with respect to the given x- axis.
Determine the moments of inertia of the shaded area about the x- and y-axes. Also determine the polar moment of inertia about point
O.
-0.80h
Answers:
lx = i
ly= i
lo = i
0.67h
-0.80h
h4
h4
h4
Determine the moment of inertia with respect to the x and y axes of the area bounded by (y-1)2 =4-2x and the y-axis. Show complete graph and solution with strips.
Chapter 8 Solutions
Applied Statics and Strength of Materials (6th Edition)
Ch. 8 - Calculate the moment of intertia with respect to...Ch. 8 - Calculate the moment of inertia of the triangular...Ch. 8 - A structural steel wide-flange section is...Ch. 8 - The concrete block shown has wall thicknesses of...Ch. 8 - A rectangle has a base of 6 in. and a height of 12...Ch. 8 - For the area of Problem 8.5 , calculate the exact...Ch. 8 - Check the tabulated moment of inertia for a 300610...Ch. 8 - For the cross section of Problem 8.3 , calculate...Ch. 8 - Calculate the moments of intertia with respect to...Ch. 8 - Calculate the moments of intertia with respect to...
Ch. 8 - The rectangular area shown has a square hole cut...Ch. 8 - For the built-up structural steel member shown,...Ch. 8 - Calculate the moments of inertia about both...Ch. 8 - Calculate the moment of inertia with respect to...Ch. 8 - For the two channels shown, calculated the spacing...Ch. 8 - Compute the radii of gyration about both...Ch. 8 - Two C1015.3 channels area welded together at their...Ch. 8 - Compute the radii of gyration with respect to the...Ch. 8 - Compute the radii of gyration with respect to the...Ch. 8 - Compute the radii of gyration with respect to the...Ch. 8 - Compute the radii of gyration with respect to the...Ch. 8 - Calculate the polar moment of inertia for a...Ch. 8 - Calculate the polar moment of inertia for a...Ch. 8 - For the areas (a) aid (b) of Problem 8.9 ,...Ch. 8 - For the following computer problems, any...Ch. 8 - For the following computer problems, any...Ch. 8 - For the following computer problems, any...Ch. 8 - For the cross section shown, calculate the moments...Ch. 8 - Calculate the moments of inertia of the area shown...Ch. 8 - For the cross-sectional areas shown, calculate the...Ch. 8 - For the cross-sectional areas shown, calculate the...Ch. 8 - Calculate the moments of intertia of the built-up...Ch. 8 - Calculate the moments of inertia about both...Ch. 8 - Calculate lx and ly of the built-up steel members...Ch. 8 - Calculate the least radius of gyration for the...Ch. 8 - A structural steel built-up section is fabricated...Ch. 8 - Calculate the polar moment of inertia for the...Ch. 8 - Determine the polar moment of inertia for the...Ch. 8 - Compute the radii of gyration with respect to the...Ch. 8 - Calculate the polar moment of inertia about the...Ch. 8 - The area of the welded member shown is composed of...
Additional Engineering Textbook Solutions
Find more solutions based on key concepts
Comprehension check 12-10
The preceding graph shows the ideal gas Jaw relationship (PV =nRT) between pressure (...
Thinking Like an Engineer: An Active Learning Approach (4th Edition)
What parts are included in the vehicle chassis?
Automotive Technology: Principles, Diagnosis, And Service (6th Edition) (halderman Automotive Series)
Water at 15C is flowing downward in a vertical tube 7.5m long. The pressure is 550kPa at the top and 585kPa at ...
Applied Fluid Mechanics (7th Edition)
The sign has a mass of 100 kg with center of mass at G. Determine the x, y, z components of reaction at the bal...
Statics and Mechanics of Materials (5th Edition)
When force P is applied to the rigid arm ABC, point B displaces vertically downward through a distance of 0.2 m...
Mechanics of Materials (10th Edition)
1.1 What is the difference between an atom and a molecule? A molecule and a crystal?
Manufacturing Engineering & Technology
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- Compute the principal centroidal moments of inertia for the plane area.arrow_forwardThe moments of inertia of the plane region about the x- and u-axes are Ix=0.4ft4 and Iu=0.6ft4, respectively. Determine y (the y-coordinate of the centroid C) and Ix (the moment of inertia about the centroidal x-axis).arrow_forwardThe moment of inertia of the plane region about the x-axis and the centroidal x-axis are Ix=0.35ft4 and Ix=0.08in.4, respectively. Determine the coordinate y of the centroid and the moment of inertia of the region about the u-axis.arrow_forward
- Determine the product of inertia with respect to the x- and y-axes for the quarter circular, thin ring (tR) by integration.arrow_forwardUsing integration, compute the polar moment of inertia about point O for the circular sector. Check your result with Table 9.2.arrow_forwardThe product of inertia of triangle (a) with respect to its centroid is Ixy=b2h2/72. What is Ixy for triangles (b)-(d)? (Hint: Investigate the signs in the expression Ixy=IxyAxy.)arrow_forward
- 3. Determine the moment of inertia with respect to the x-x and y-y centroidal axes for the following figure: Objects are Cen tured E3.5" 2"arrow_forwardDetermine the moment of inertia about x and y axes for the area bounded by the curves as shown in figure by direct integration method.arrow_forwardDetermine the moments of inertia of the shaded area about the x- and y-axes. Also determine the polar moment of inertia about point O. -0.58h -0.58h 0.63h harrow_forward
- Determine the moments of inertia of the shaded area about the x- and y-axes. Also determine the polar moment of inertia about point O. -0.56h Answers: 1x = i ly= i lo= i 0.60h -0.56h h h4 h4 hearrow_forwardDetermine the moments of inertia of the shaded area about the x- and y-axes. Also determine the polar moment of inertia about point O. -0.57h -0.57h 0.59h h Answers: Ix = h4 Iy = h4 Io = h4arrow_forwardDo it neatly pleasearrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
International Edition---engineering Mechanics: St...Mechanical EngineeringISBN:9781305501607Author:Andrew Pytel And Jaan KiusalaasPublisher:CENGAGE L
International Edition---engineering Mechanics: St...
Mechanical Engineering
ISBN:9781305501607
Author:Andrew Pytel And Jaan Kiusalaas
Publisher:CENGAGE L
moment of inertia; Author: NCERT OFFICIAL;https://www.youtube.com/watch?v=A4KhJYrt4-s;License: Standard YouTube License, CC-BY