Chapter 8, Problem 1DA

The Data Bank is found in Appendix B, or on the World Wide Web by following links from www.mhhe.com/math/stats/bluman/

1. From the Data Bank, select a random sample of at least 30 individuals, and test one or more of the following hypotheses by using the z test. Use α = 0.05.

a. For serum cholesterol, H₀: μ = 220 milligram percent (mg%). Use σ = 5.

b. For systolic pressure, H₀: μ = 120 millimeters of mercury (mm Hg). Use σ = 13.

c. For IQ, H₀: μ = 100. Use σ = 15.

d. For sodium level, H₀: μ = 140 milliequivalents per liter (mEq/l). Use σ = 6.

To determine

To test: The claim that $H_0:\mu =220$.

Answer to Problem 1DA

The conclusion is that there is sufficient evidence to infer that the average serum cholesterol level is differs from 220 milligram percent (mg%).

Explanation of Solution

Answer will vary. One of the possible answers is given below:

Given info:

Claim: $H_0:\mu =220$, $n=35$, $\sigma =5$ and $\alpha =0.05$.

Calculation:

State the null and alternative hypotheses:

Null hypothesis:

$H_0:\mu =220$

Alternative hypothesis:

$H_1:\mu \ne 220$

Test statistic value and P-value:

Software procedure:

Step by step procedure to obtain the test value using the MINITAB software:

• Choose Stat > Basic Statistics > 1-Sample Z.
• In Samples in Column, enter the column of Serum cholesterol.
• In Standard deviation, enter 5.
• In Perform hypothesis test, enter the test mean as 220.
• Check Options; enter Confidence level as 95%.
• Choose not equal in alternative.
• Click OK in all dialogue boxes.

Output using the MINITAB software is given below:

From the output, the test value is –10.68 and the P-value is 0.000.

Make the Decision:

Decision rule:

If $P\text{-value}\le \alpha$, reject the null hypothesis

If $P\text{-value}>\alpha$, do not reject the null hypothesis.

Here, the P-value is lesser than the level of significance.

That is, $P\text{-value}\left(=0.000\right)<\alpha \left(=0.05\right)$.

By the decision rule, the null hypothesis is rejected.

Thus, the decision is “reject the null hypothesis”.

Summarize the result:

There is sufficient evidence to infer that the average serum cholesterol level is differs from 220 milligram percent (mg%).

To determine

To test: The claim that $H_0:\mu =120$.

Answer to Problem 1DA

The conclusion is that there is sufficient evidence to infer that the average systolic pressure is differs from 120 millimeters of mercury (mm Hg).

Explanation of Solution

Given info:

Claim: $H_0:\mu =120$, $n=35$, $\sigma =13$ and $\alpha =0.05$.

Calculation:

State the null and alternative hypotheses:

Null hypothesis:

$H_0:\mu =120$

Alternative hypothesis:

$H_1:\mu \ne 120$

Test statistic value and P-value:

Software procedure:

Step by step procedure to obtain the test value using the MINITAB software:

• Choose Stat > Basic Statistics > 1-Sample Z.
• In Samples in Column, enter the column of Systolic pressure.
• In Standard deviation, enter 13.
• In Perform hypothesis test, enter the test mean as 120.
• Check Options; enter Confidence level as 95%.
• Choose not equal in alternative.
• Click OK in all dialogue boxes.

Output using the MINITAB software is given below:

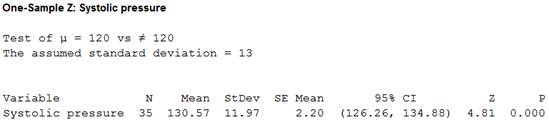
From the output, the test value is 4.81 and the P-value is 0.000.

Make the Decision:

Here, the P-value is lesser than the level of significance.

That is, $P\text{-value}\left(=0.000\right)<\alpha \left(=0.05\right)$.

By the decision rule, the null hypothesis is rejected.

Thus, the decision is “reject the null hypothesis”.

Summarize the result:

There is sufficient evidence to infer that the average systolic pressure is differs from 120 millimetres of mercury (mm Hg).

To determine

To test: The claim that $H_0:\mu =100$.

Answer to Problem 1DA

The conclusion is that there is sufficient evidence to infer that the average IQ score is differs from 100.

Explanation of Solution

Given info:

Claim: $H_0:\mu =100$, $n=35$, $\sigma =15$ and $\alpha =0.05$.

Calculation:

State the null and alternative hypotheses:

Null hypothesis:

$H_0:\mu =100$

Alternative hypothesis:

$H_1:\mu \ne 100$

Test statistic value and P-value:

Software procedure:

Step by step procedure to obtain the test value using the MINITAB software:

• Choose Stat > Basic Statistics > 1-Sample Z.
• In Samples in Column, enter the column of IQ.
• In Standard deviation, enter 15.
• In Perform hypothesis test, enter the test mean as 100.
• Check Options; enter Confidence level as 95%.
• Choose not equal in alternative.
• Click OK in all dialogue boxes.

Output using the MINITAB software is given below:

From the output, the test value is 3.49 and the P-value is 0.000.

Make the Decision:

Here, the P-value is lesser than the level of significance.

That is, $P\text{-value}\left(=0.000\right)<\alpha \left(=0.05\right)$.

By the decision rule, the null hypothesis is rejected.

Thus, the decision is “reject the null hypothesis”.

Summarize the result:

There is sufficient evidence to infer that the average IQ score is differs from 100.

To determine

To test: The claim that $H_0:\mu =140$.

Answer to Problem 1DA

The conclusion is that there is sufficient evidence to infer that the average sodium level is 140.

Explanation of Solution

Given info:

Claim: $H_0:\mu =140$, $n=35$, $\sigma =6$ and $\alpha =0.05$.

Calculation:

State the null and alternative hypotheses:

Null hypothesis:

$H_0:\mu =140$

Alternative hypothesis:

$H_1:\mu \ne 140$

Test statistic value and P-value:

Software procedure:

Step by step procedure to obtain the test value using the MINITAB software:

• Choose Stat > Basic Statistics > 1-Sample Z.
• In Samples in Column, enter the column of Sodium level.
• In Standard deviation, enter 6.
• In Perform hypothesis test, enter the test mean as 140.
• Check Options; enter Confidence level as 95%.
• Choose not equal in alternative.
• Click OK in all dialogue boxes.

Output using the MINITAB software is given below:

From the output, the test value is 0.31 and the P-value is 0.757.

Make the Decision:

Here, the P-value is greater than the level of significance.

That is, $P\text{-value}\left(=0.757\right)>\alpha \left(=0.05\right)$.

By the decision rule, the null hypothesis is not rejected.

Thus, the decision is “fail to reject the null hypothesis”.

Summarize the result:

There is sufficient evidence to infer that the average sodium level is 140.