Chapter 7, Problem 7.13P

To determine

(a)

To prove:

H is not the function of $\varphi$ or zusing law of Ampere's circuital.

Explanation of Solution

Given:

The radius of the hollow cylindrical shell is 'a' which is centered on the z -axis and it carries a surface charge density of $K_a{a}_{\varphi }$.

Concept Used:

In this concept, Ampere's circuital law will be used. The law of Ampere states the relationship between the magnetic field and the current one. It says that the closed integration of the magnetic field along the path is equal to the current product contained in the path and the medium. The mathematical expression is given as-

   ${\displaystyle \underset{L}{\oint }H\cdot dl={\mu }_0{I}_{enclosed}}$

Where,

H = magnetic field

   ${\mu }_0$ = permittivity of the medium

I_enclosed = current within the path

Calculation:

As given a hollow cylinder with a radius of 'a' which is centered on the z-axis.

The surface charge density of this cylinder is $K_a{a}_{\varphi }$.

Hollow cylinder along with amperian loop around it shown below-

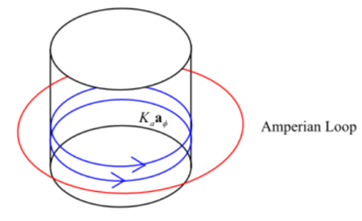
From the above diagram, the value net charge within the loop is zero.

Amperian loop, when the surface charge current element within this loop is given below-

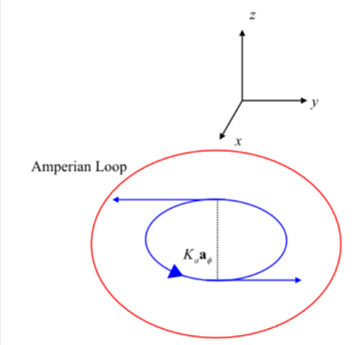
From the above diagram, the direction of the tangent of the current element is a_ywhich is situated at the diametrically opposite end of the direction of the current element which is -a_y.In the same way, every current element's tangent is situated exactly opposite to the direction of the current element.

With this condition, the total charge is canceled out and it will become zero. Therefore,

$I_{enclosed}=0$

From, Ampere's circuital law-

   ${\displaystyle \underset{L}{\oint }H.dl=I_{enclosed}}=0$

So, H =0. This explains that there is no magnetic field outside the cylinder.

Now, create a loop around the hollow cylinder which passes through its axis and also the outside of the hollow cylinder. This is given as-

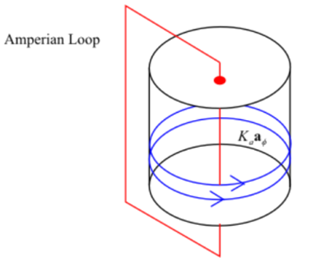
From the above diagram, the direction of surface current is $a_{\varphi }$ and the surface current crossing unit normal length remains the same for any value of $\varphi .$ Thus, the net current within amperian loop remains the same for any value of $\varphi$.

Therefore, magnetic field intensity is independent of any value of $\varphi .$

From the same diagram, assume the length of the cylinder is infinite and the length of the loop is the finite dimension. At this condition, amperian loop cuts the infinite cylinder. Therefore, the net current remainsthe same for the amperian loop of the same dimension in the direction of z.

Therefore magnetic field intensity is independent of any value of z.

Conclusion:

Thus, it is proved that the H is not the function of $\varphi$ and z.

To determine

(b)

To show:

The value of $H_{\varphi }$ and $H_{\rho }$ is zero everywhere.

Answer to Problem 7.13P

It is proved that $H_{\varphi }$ is zero everywhere, by using Ampere's circuital law

It is proved that $H_{\rho }$ is zero everywhere, by using Biot Savarts law.

Explanation of Solution

Given:

Radius of hollow cylindrical shell is 'a' which is centered on z- axis and carries uniform surface charge density of $K_a{a}_{\varphi }$.

Concept Used:

The concept of Ampere's circuital law and Biot's Savarts will be used. Ampere's circuital law says the closed integration of magnetic field along the path is equal to the product of current within that path and the medium. The mathematical expression is given as-

   ${\displaystyle \underset{L}{\oint }H\cdot dl={\mu }_0{I}_{enclosed}}$

Where,

H = magnetic field

μ₀ = permittivity of the medium

I_enclosed = current within the path

Biot Savart's law is used to calculate the magnetic field generated by the current carrying wire and helps to determine its strength at different points. It helps to relate magnetic field, direction, length, and proximity of electric current. Biot Savart's equation in terms of surface charge density is given as-

$H(r)={\displaystyle \int \frac{K(r)\times \widehat{r}}{4\pi r^2}da}$

Where,

K = surface charge density

$\widehat{r}$ = unit vector

Calculation:

As the component $\varphi$ of H doesn't exist, if it exists then the amperian loop would be encircling the complete hollow cylinder.

The magnetic field produces the direction of a_z because the amperian loop encloses the current flowing the direction of a_z, but there is no current in the direction of a_z.

Therefore, $H_{\varphi }=0$

The unit vector of the inside loop of the cylinder is given as-

$a_R=\frac{-\rho a_{\rho }+z{a}_z}{{\rho }^2+z^2}$

By using Biot Savart's law, we can get the direction of the field which is obtained by K×a_R. The component a_z of a_R results in the radial component of magnetic field intensity which is given as-

$K_a{a}_{\varphi }\times a_z=K_a{a}_{\rho }$

Where, charge density is $K_a{a}_{\varphi }$.

Obtained radial components are opposite to the point at the end of the diameter of the hollow cylinder for constant z.

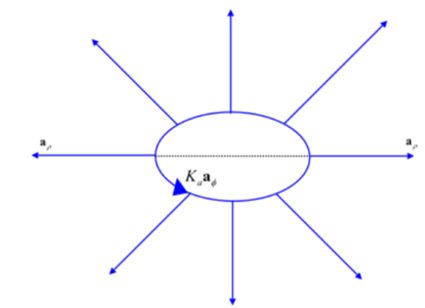
For each current element there is radial component, but these radial elements cancel out by the current element which is located diametrically opposite of radial element at same z. Therefore, the radial component for hollow cylinder shell is

$H_{\rho }=0$

Conclusion:

It is proved that the value of $H_{\varphi }$ and $H_{\rho }$ is zero everywhere, by using Ampere's circuital law and radial component of Biot Savarts law.

To determine

(c)

To prove:

The value of H_z = 0 for $\rho >a$.

Answer to Problem 7.13P

By usingAmpere's circuital law,

The current within cylinder is zero and the electric field outside the cylinder is zero. Therefore, the value of H_z = 0 for $\rho >a$.

Explanation of Solution

Given:

The radius of the hollow cylindrical shell is 'a' which is centered on z -axis and carries uniform surface charge density of $K_a{a}_{\varphi }$.

Concept Used:

In this concept of Ampere's circuital law will be used. Ampere's law states the relationship between the current and the magnetic field. It says the closed integration of magnetic field along the path is equal to the product of current within that path and the medium. The mathematical expression is given as-

   ${\displaystyle \underset{L}{\oint }H\cdot dl={\mu }_0{I}_{enclosed}}$

Where,

H = magnetic field

μ₀ = permittivity of the medium

I_enclosed = current within the path

Calculation:

Hollow cylinder along with amperian loop around it is shown below-

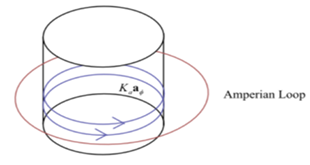
Amperian loop is when the surface charge current element within this loop is given below-

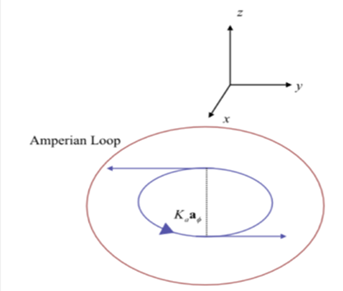
From the above two diagrams, the Amperian loop encompasses the hollow cylindrical shell completely. Therefore, the current within cylinder is zero. So, the electric field outside the cylinder is zero. Thus, the value of the magnetic field is zero for $\rho >a$.

$H_z=0,\rho >a$

Conclusion:

By using Ampere's circuital law,

The current within cylinder is zero and the electric filed outside the cylinder is zero. Therefore, the value of $H_z=0,\rho >a$.

To determine

(d)

To prove:

The value of $H_z=K_a\text{for}\rho >a$.

Answer to Problem 7.13P

By using Biot Savarts equation in terms of surface charge density, the value of magnetic field is $H=K_a{a}_z$ or $H_z=K_a\text{for}\rho >a$ in the direction of z.

Explanation of Solution

Given:

Radius of hollow cylindrical shell is 'a' which is centered on z -axis and carries uniform surface charge density of $K_a{a}_{\varphi }$.

Concept Used:

Biot Savart's law is used to calculate the magnetic field generated by the current carrying wire and helps to determine its strength at different points. It helps to relate magnetic field, direction, length, and proximity of electric current. Biot Savart's equation in terms of surface charge density is given as-

$H(r)={\displaystyle \int \frac{K(r)\times \widehat{r}}{4\pi r^2}da}$

Where,

K = surface charge density

$\widehat{r}$ = unit vector

H = magnetic field

Calculation:

Here, a magnetic field is to be calculated at the inside points of the cylinder as it doesn't exist outside the cylinder.

The unit vector of the inside loop of the cylinder is given as-

$a_R=\frac{-\rho a_{\rho }+z{a}_z}{{\rho }^2+z^2}$

Biot Savart equation can be written in terms of surface charge density, which is given as-

$H={\displaystyle {\int }_s\frac{K\times a_r}{4\pi R^2}dS}$

Where, K is surface charge density, which given as-

$K=K_a{a}_{\varphi }$

Put the value of K and a_r in the equation-

   $H={\displaystyle {\int }_s\frac{K_a{a}_{\varphi }\times \left(-\rho a_{\rho }+z{a}_z\right)}{4\pi {\left({\rho }^2+z^2\right)}^{\frac{3}{2}}}dS}$

As it is already proved that H does not vary with z.

   $H={\displaystyle {\int }_s\frac{K_a{a}_{\varphi }\times \left(-\rho a_{\rho }\right)}{4\pi {\left({\rho }^2+z^2\right)}^{\frac{3}{2}}}dS}$

   $H=K_a{a}_z{\displaystyle {\int }_s\frac{\rho }{4\pi {\left({\rho }^2+z^2\right)}^{\frac{3}{2}}}\rho d\varphi dz}$

   $H=K_a{a}_z{\displaystyle {\int }_s\frac{{\rho }^2}{4\pi {\left({\rho }^2+z^2\right)}^{\frac{3}{2}}}d\varphi dz}$

In above equation, put $z =\rho tan\theta$ , therefore, $dz=\rho se{c}^2\theta d\theta$

   $H=K_a{a}_z{\displaystyle {\int }_s\frac{{\rho }^2}{4\pi {\left({\rho }^2+{\rho }^{2}ta{n}^2\theta \right)}^{\frac{3}{2}}}d\varphi \rho se{c}^2\theta d\theta }$

   $H=K_a{a}_z{\displaystyle {\int }_s\frac{{\rho }^{3}se{c}^2\theta }{4\pi {\left({\rho }^2+{\rho }^{2}ta{n}^2\theta \right)}^{\frac{3}{2}}}d\varphi d\theta }$

   $H=K_a{a}_z{\displaystyle {\int }_s\frac{{\rho }^{3}se{c}^2\theta }{4\pi {\rho }^{3}se{c}^3\theta }d\varphi d\theta }$

   $H=\frac{K_a{a}_z}{4\pi }{\displaystyle {\int }_s\cos \theta d\varphi d\theta }$

Limits of θ

   $z=+\infty \Rightarrow \theta =+\frac{\pi }{2}$

   $z=-\infty \Rightarrow \theta =-\frac{\pi }{2}$

   $H=\frac{K_a{a}_z}{4\pi }{\displaystyle {\int }_0^{2\pi }{\displaystyle {\int }_{-\frac{\pi }{2}}^{\frac{\pi }{2}}\cos \theta d\varphi d\theta }}$

On integration

   $H=\frac{K_a{a}_z}{4\pi }\times 2\pi \times 2$

   $H=K_a{a}_z$

Conclusion:

The value of the magnetic field is $H=K_a{a}_z$ or H_z = K_a for ρ> a in the direction of z.

To determine

(e)

The value of H everywhere.

Answer to Problem 7.13P

Value of H is

Magnetic field for $\rho <a=\left(K_a+K_b\right)a_z$

Magnetic field for $a<\rho <b=K_b{a}_{\varphi }$

Magnetic field for $\rho >b=0$

Explanation of Solution

Given:

The radius of the hollow cylindrical shell is 'a' which is centered on z -axis and carries uniform surface charge density of $K_a{a}_{\varphi }$ . In the second shell $\rho =b$ , and the value of current is $K_b{a}_{\varphi }$.

Concept Used:

In this concept of Ampere's circuital law will be used. Ampere's law states the relationship between the current and the magnetic field. It says the closed integration of magnetic field along the path is equal to the product of current within that path and the medium. The mathematical expression is given as-

   ${\displaystyle \underset{L}{\oint }H\cdot dl={\mu }_0{I}_{enclosed}}$

Where,

H = magnetic field

μ₀ = permitivity of the medium

I_enclosed = current within the path

Calculation:

Plot the amperian loop around the hollow cylinder to get the magnetic field everywhere-

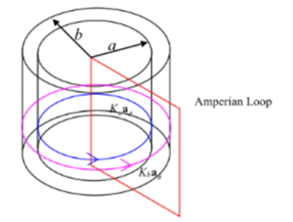
From the above diagram, the magnetic field for $\rho <a\text{ is }\left(K_a+K_b\right)a_z$

Magnetic field for $a<\rho <b$

Amperian loop for this is $K_b{a}_{\varphi }$ , therefore magnetic field at this radius is $K_b{a}_{\varphi }$.

Magnetic field for radius $\rho >b$

In this case, no current is within the amperian loop, therefore magnetic field for this case is zero.

Conclusion:

Magnetic field for $\rho <a=\left(K_a+K_b\right)a_z$

Magnetic field for $a<\rho <b=K_b{a}_{\varphi }$

Magnetic field for $\rho >b=0$