
Concept explainers
A particle of mass m = 1.18 kg is attached between two identical springs on a frictionless, horizontal tabletop. Both springs have spring constant k and are initially unstressed, and the particle is at x = 0. (a) The particle is pulled a distance x along a direction perpendicular to the initial configuration of the springs as shown in Figure P7.50. Show that the force exerted by the springs on the particle is
(b) Show that the potential energy of the system is
(c) Make a plot of U(x) versus x and identify all equilibrium points. Assume L = 1.20 m and k = 40.0 N/m. (d) If the panicle is pulled 0.500 m to the right and then released, what is its speed when it reaches x = 0?
Figure P7.50
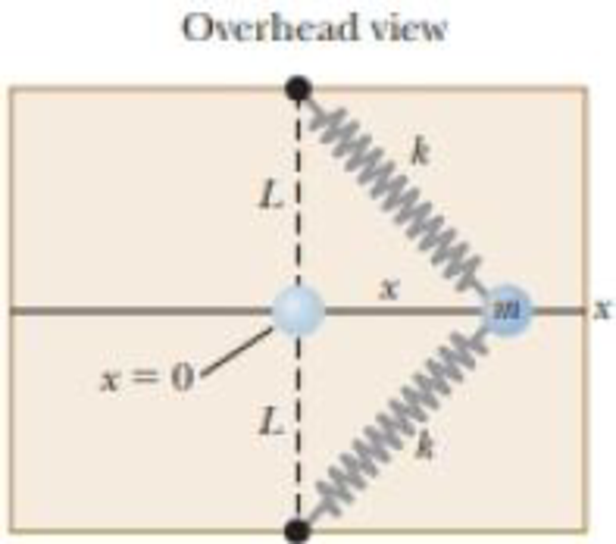
(a)
To show: The force exerted by the spring on the particle is
Answer to Problem 50CP
The force exerted by the spring on the particle is
Explanation of Solution
Given info: The mass of the particle is
The free body diagram of the given case is as shown in the figure below.
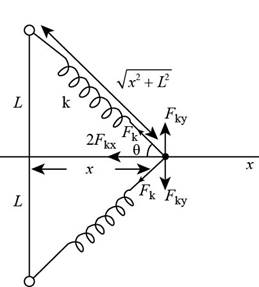
Figure (1)
The extension in the spring is,
Here,
The new length after stretching is,
Here,
Substitute
From the figure (1) the net force in
The net force in
The negative sign is due to the direction of the force in the negative direction.
Here,
From the free body diagram the value of
The formula for the spring force is,
Substitute
Substitute
Write the expression for the force exerted by the spring on the particle.
Substitute
Conclusion:
Therefore, the force exerted by the spring on the particle is
(b)
To show: The potential energy of the system is
Answer to Problem 50CP
The potential energy of the system is
Explanation of Solution
Given info: The mass of the particle is
From part (a) the force exerted by the spring on the particle is,
The potential energy of a system is,
Substitute
Conclusion:
Therefore, the potential energy of the system is
(c)
To draw: The plot of
Answer to Problem 50CP
The plot for
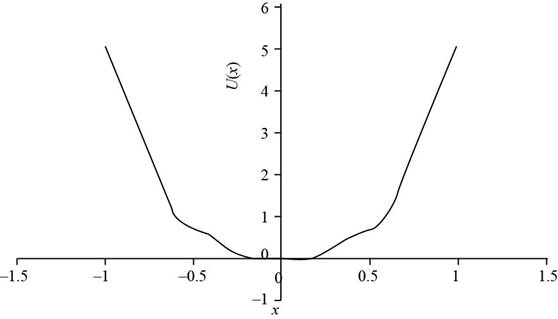
Explanation of Solution
Introduction:
The equilibrium points are the points at which the values of the force and the potential energy have the minimum value in order to have higher stability in the system.
Given info: The mass of the particle is
The potential energy of the system is,
Substitute
Thus, the potential energy of the system is,
The plot
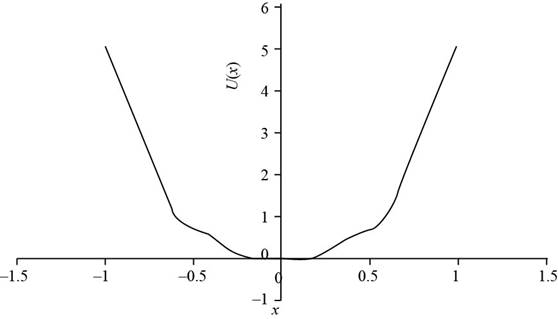
Figure (2)
The equilibrium points are the point at the potential energy is zero. In the above plot the minimum potential energy is at
Form the graph the equilibrium point is for
Conclusion:
Therefore, the plot for
(d)
The speed of the particle.
Answer to Problem 50CP
The speed of the particle is
Explanation of Solution
Given info: The mass of the particle is
The potential energy of the system is,
Substitute
Thus, the potential energy of the system is
The potential energy is converted in to the kinetic energy to follow the law of conservation of momentum.
Here,
Rearrange the above equation for
Substitute
Conclusion:
Therefore, speed of the particle is
Want to see more full solutions like this?
Chapter 7 Solutions
Physics for Scientists and Engineers with Modern Physics
Additional Science Textbook Solutions
College Physics: A Strategic Approach (3rd Edition)
Laboratory Manual For Human Anatomy & Physiology
SEELEY'S ANATOMY+PHYSIOLOGY
Fundamentals Of Thermodynamics
Chemistry: A Molecular Approach (4th Edition)
Chemistry & Chemical Reactivity
- For what type of force is it not possible to define a potential energy expression?arrow_forward10. Imagine you have a system in which you have 54 grams of ice. You can melt this ice and then vaporize it all at 0 C. The melting and vaporization are done reversibly into a balloon held at a pressure of 0.250 bar. Here are some facts about water you may wish to know. The density of liquid water at 0 C is 1 g/cm³. The density of ice at 0 C is 0.917 g/cm³. The enthalpy of vaporization of liquid water is 2.496 kJ/gram and the enthalpy of fusion of solid water is 333.55 J/gram.arrow_forwardConsider 1 mole of supercooled water at -10°C. Calculate the entropy change of the water when the supercooled water freezes at -10°C and 1 atm. Useful data: Cp (ice) = 38 J mol-1 K-1 Cp (water) 75J mol −1 K -1 Afus H (0°C) 6026 J mol −1 Assume Cp (ice) and Cp (water) to be independent of temperature.arrow_forward
- The molar enthalpy of vaporization of benzene at its normal boiling point (80.09°C) is 30.72 kJ/mol. Assuming that AvapH and AvapS stay constant at their values at 80.09°C, calculate the value of AvapG at 75.0°C, 80.09°C, and 85.0°C. Hint: Remember that the liquid and vapor phases will be in equilibrium at the normal boiling point.arrow_forward3. The entropy of an ideal gas is S = Nkg In V. Entropy is a state function rather than a path function, and in this problem, you will show an example of the entropy change for an ideal gas being the same when you go between the same two states by two different pathways. A. Express ASV = S2 (V2) - S₁(V1), the change in entropy upon changing the volume from V₁to V2, at fixed particle number N and energy, U. B. Express ASN = S₂(N₂) - S₁ (N₁), the change in entropy upon changing the particle number from N₁ to N2, at fixed volume V and energy U. C. Write an expression for the entropy change, AS, for a two-step process (V₁, N₁) → (V2, N₁) → (V2, N₂) in which the volume changes first at fixed particle number, then the particle number changes at fixed volume. Again, assume energy is constant.arrow_forwardPlease don't use Chatgpt will upvote and give handwritten solutionarrow_forward
- 6. We used the constant volume heat capacity, Cv, when we talked about thermodynamic cycles. It acts as a proportionality constant between energy and temperature: dU = C₁dT. You can also define a heat capacity for constant pressure processes, Cp. You can think of enthalpy playing a similar role to energy, but for constant pressure processes δαρ C = (37) - Sup Ср ат P = ат Starting from the definition of enthalpy, H = U + PV, find the relationship between Cy and Cp for an ideal gas.arrow_forwardPure membranes of dipalmitoyl lecithin phospholipids are models of biological membranes. They melt = 41°C. Reversible melting experiments indicate that at Tm AHm=37.7 kJ mol-1. Calculate: A. The entropy of melting, ASm- B. The Gibbs free energy of melting, AGm- C. Does the membrane become more or less ordered upon melting? D. There are 32 rotatable CH2 CH2 bonds in each molecule that can rotate more freely if the membrane melts. What is the increase in multiplicity on melting a mole of bonds?arrow_forward5. Heat capacity often has a temperature dependence for real molecules, particularly if you go over a large temperature range. The heat capacity for liquid n-butane can be fit to the equation Cp(T) = a + bT where a = 100 J K₁₁ mol¹ and b = 0.1067 J K² mol¹ from its freezing point (T = 140 K) to its boiling point (T₁ = 270 K). A. Compute AH for heating butane from 170 K to 270 K. B. Compute AS for the same temperature range.arrow_forward
- 4. How much energy must be transferred as heat to cause the quasi-static isothermal expansion of one mole of an ideal gas at 300 K from PA = 1 bar to PB = 0.5 bar? A. What is VA? B. What is VB? C. What is AU for the process? D. What is AH for the process? E. What is AS for the process?arrow_forward1. The diagram shows the tube used in the Thomson experiment. a. State the KE of the electrons. b. Draw the path of the electron beam in the gravitational field of the earth. C. If the electric field directed upwards, deduce the direction of the magnetic field so it would be possible to balance the forces. electron gun 1KVarrow_forwardas a hiker in glacier national park, you need to keep the bears from getting at your food supply. You find a campground that is near an outcropping of ice. Part of the outcropping forms a feta=51.5* slopeup that leads to a verticle cliff. You decide that this is an idea place to hang your food supply out of bear reach. You put all of your food into a burlap sack, tie a rope to the sack, and then tie a bag full of rocks to the other end of the rope to act as an anchor. You currently have 18.5 kg of food left for the rest of your trip, so you put 18.5 kg of rocks in the anchor bag to balance it out. what happens when you lower the food bag over the edge and let go of the anchor bag? Determine the acceleration magnitude a of the two-bag system when you let go of the anchor bag?arrow_forward
 Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University
University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning





