
The state of plane stress shown occurs in a machine component made of a steel with σy = 30 ksi. Using the maximum- distortion-energy criterion, determine whether yield will occur when (a) τXV = 6 ksi, (b) τXV = 12 ksi, (c) τXV = 14 ksi. If yield does not occur, determine the corresponding factor of safety.
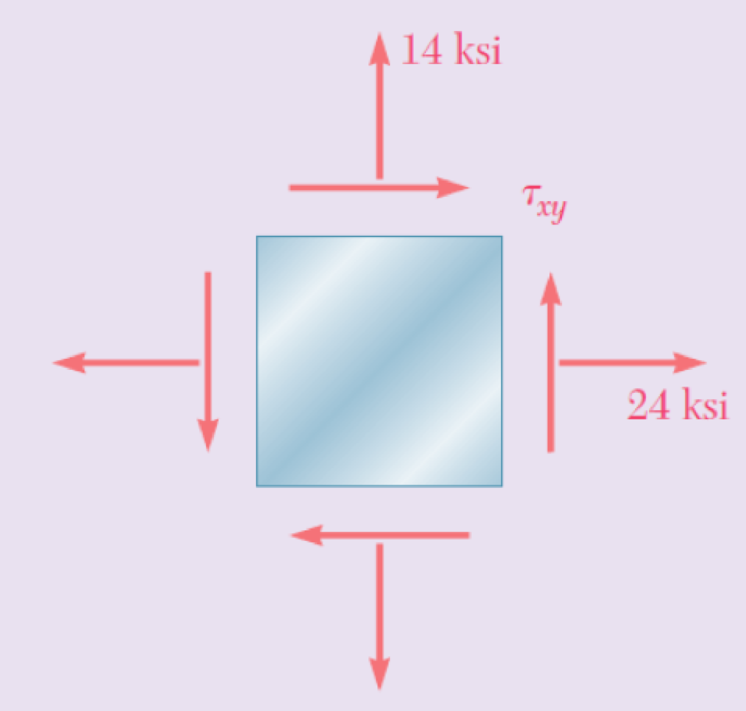
Fig. P7.164
(a)
Check the yield will occur for the given condition or not?.
Find the corresponding factor of safety for not occurring the yield.
Answer to Problem 164RP
The yielding will
Explanation of Solution
Given information:
The normal stress in x-axis is
The normal stress in y-axis is
The shearing stress in xy-plane is
The allowable yield strength of the steel is
Use maximum distortion-energy theory.
Calculation:
Consider the normal stress in z-axis is
The minimum principal stress is
Find the average normal stress
Substitute 24 ksi for
Find the radius of the Mohr circle (R) using the equation.
Substitute 24 ksi for
Find the maximum principal stress
Substitute 19 ksi for
Find the minimum principal stress
Substitute 19 ksi for
Check the yielding condition using the Maximum-distortion-energy criteria as follows;
Substitute 26.81 ksi for
The yielding will not occur.
Find the factor of safety (FOS) using the relation.
Substitute 30 ksi for
Therefore, the yielding will
(b)
Check the yield will occur for the given condition or not?.
Find the corresponding factor of safety for not occurring the yield.
Answer to Problem 164RP
The yielding will
Explanation of Solution
Given information:
The normal stress in x-axis is
The normal stress in y-axis is
The shearing stress in xy-plane is
The allowable yield strength of the steel is
Use maximum distortion-energy theory.
Calculation:
Consider the normal stress in z-axis is
The minimum principal stress is
Find the average normal stress
Substitute 24 ksi for
Find the radius of the Mohr circle (R) using the equation.
Substitute 24 ksi for
Find the maximum principal stress
Substitute 19 ksi for
Find the minimum principal stress
Substitute 19 ksi for
Check the yielding condition using the Maximum-distortion-energy criteria as follows;
Substitute 32 ksi for
The yielding will not occur.
Find the factor of safety (FOS) using the relation.
Substitute 30 ksi for
Therefore, the yielding will
(c)
Check the yield will occur for the given condition or not?.
Find the corresponding factor of safety for not occurring the yield.
Answer to Problem 164RP
The yielding will occur.
Explanation of Solution
Given information:
The normal stress in x-axis is
The normal stress in y-axis is
The shearing stress in xy-plane is
The allowable yield strength of the steel is
Use maximum distortion-energy theory.
Calculation:
Consider the normal stress in z-axis is
The minimum principal stress is
Find the average normal stress
Substitute 24 ksi for
Find the radius of the Mohr circle (R) using the equation.
Substitute 24 ksi for
Find the maximum principal stress
Substitute 19 ksi for
Find the minimum principal stress
Substitute 19 ksi for
Check the yielding condition using the Maximum-distortion-energy criteria as follows;
Substitute 33.866 ksi for
The yielding will occur.
Therefore, the yielding will occur.
Want to see more full solutions like this?
Chapter 7 Solutions
EBK MECHANICS OF MATERIALS
- Solve this problem and show all of the workarrow_forwardNo chatgpt pls will upvotearrow_forwardreading is 0.4 mas SHOWN. Assume h₁ = 0.4 m, h₂ = 0.5 m. (a) Do you know the specific weight of mercury? (b) Do you know the specific weight of gasoline? (c) Do you know the specific weight of oil? (a) YHg = 133,000 (b) Ygas = 6867 (c) Yoil = 8829 eTextbook and Media Part 2 N/m³ N/m³ N/m³ A+ Gasoline t +B Oil -Mercury Attempts: unlimited Did you calculate the pressure difference between two locations using the correct specific weight? Did you assume that the pressures in fluid are the same in a horizontal plane even though they are in different tubes? Are the calculated pressures in a column of fluid always higher at lower elevations? Did you account for the fact that the two horizontal tubes of the U-tube are above the ground? Concepts: The pressure in a fluid is a function of the specific weight of the fluid and the height relative to a reference. Pressure is constant in a horizontal plane of a continuous mass of fluid. (a) What is the initial pressure difference? (PA-PB) (b) What is…arrow_forward
- Find the solution of the following Differential Equations 1) "-4y+3y=0 3) "+16y=0 2) y"-16y=0 4) y"-y-6y=0 5) y"+2y=0 7) y"+y=0, (#0) 9) y"-y=0, y(0) = 6, y'(0) = -4 11) y"-4y+3y=0, y(0)=-1, 13) y'(0) = -5 "+2y+2y=0 15) y"-9y=0 17) y"-4y=0 6) y"-2y+2y=0 8) "+4y+5y=0 10) y"-9y=0, y(0) = 2, y'(0) = 0 12) y"-3y+2y= 0, y(0)=-1, y'(0) = 0 14) 4y+4y+y=0 16) "+6y+12y=0 18) 4y+4y+17y=0arrow_forwardAccess Pearson Mastering Engineering Back to my courses Course Home Course Home Scoresarrow_forwardAccess Pearson Mastering Engineering Back to my courses Course Home Course Home Scores Review Next >arrow_forward
- Access Pearson Course Home Scoresarrow_forwardAccess Pearson Mastering Engineering Back to my courses Course Home Course Home Scoresarrow_forwardAccess Pearson Mastering Engineering Back to my courses Course Home Course Home Scores ■Review Next >arrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_ios
 Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY





