
Single Variable Calculus Format: Unbound (saleable)
3rd Edition
ISBN: 9780134765761
Author: Briggs, William L.^cochran, Lyle^gillett, Bernard^
Publisher: Prentice Hall
expand_more
expand_more
format_list_bulleted
Concept explainers
Textbook Question
Chapter 6.4, Problem 8E
Let R be the region bounded by the curves
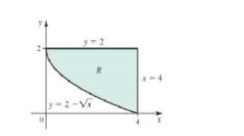
8. Suppose the shell method is used to determine the volume of the solid generated by revolving R about the line x = 4.
- a. What is the radius of a cylindrical shell at a point x in [0, 4]?
- b. What is the height of a cylindrical shell at a point x in [0, 4]?
- c. Write an
integral for the volume of the solid using the shell method.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Find the given derivative.
Dx 7x
1
6
2
이에
Question
Consider the graph of f(x) below. Over what open interval is f'(x) positive? Give your answer in interval notation.
Provide your answer below:
0
Question
Find the open interval(s) of f(x), graph given below, where f'(x) is negative.
-3-2-1
1 2
4
5
6
Give your answer in interval notation. For example, (2, 4) U (6,∞).
Provide your answer below:
Chapter 6 Solutions
Single Variable Calculus Format: Unbound (saleable)
Ch. 6.1 - A police officer leaves his station on a...Ch. 6.1 - Describe a possible motion of an object along a...Ch. 6.1 - Is the position s(t) a number or a function? For...Ch. 6.1 - Without doing further calculations, what are the...Ch. 6.1 - Prob. 5QCCh. 6.1 - Prob. 6QCCh. 6.1 - Explain the meaning of position, displacement, and...Ch. 6.1 - Suppose the velocity of an object moving along a...Ch. 6.1 - Given the velocity function v of an object moving...Ch. 6.1 - Prob. 4E
Ch. 6.1 - Prob. 5ECh. 6.1 - What is the result of integrating a population...Ch. 6.1 - Displacement and distance from velocity Consider...Ch. 6.1 - Displacement and distance from velocity Consider...Ch. 6.1 - Velocity graphs The figures show velocity...Ch. 6.1 - Prob. 10ECh. 6.1 - Prob. 11ECh. 6.1 - Prob. 12ECh. 6.1 - Displacement from velocity Consider an object...Ch. 6.1 - Displacement from velocity Consider an object...Ch. 6.1 - Displacement from velocity Consider an object...Ch. 6.1 - Displacement from velocity Assume t is time...Ch. 6.1 - Position from velocity Consider an object moving...Ch. 6.1 - Prob. 18ECh. 6.1 - Position from velocity Consider an object moving...Ch. 6.1 - Prob. 20ECh. 6.1 - Prob. 21ECh. 6.1 - Prob. 22ECh. 6.1 - Prob. 23ECh. 6.1 - Prob. 24ECh. 6.1 - Flying into a headwind The velocity (in mi/hr) of...Ch. 6.1 - Day hike The velocity (in mi/hr) of a hiker...Ch. 6.1 - Piecewise velocity The velocity of a (fast)...Ch. 6.1 - Probe speed A data collection probe is dropped...Ch. 6.1 - Position and velocity from acceleration Find the...Ch. 6.1 - Position and velocity from acceleration Find the...Ch. 6.1 - Prob. 31ECh. 6.1 - Prob. 32ECh. 6.1 - Position and velocity from acceleration Find the...Ch. 6.1 - Prob. 34ECh. 6.1 - Prob. 35ECh. 6.1 - Prob. 36ECh. 6.1 - Prob. 37ECh. 6.1 - Prob. 38ECh. 6.1 - Prob. 39ECh. 6.1 - Prob. 40ECh. 6.1 - Prob. 41ECh. 6.1 - Prob. 42ECh. 6.1 - Population growth 43. A culture of bacteria in a...Ch. 6.1 - Prob. 44ECh. 6.1 - Oil production An oil refinery produces oil at a...Ch. 6.1 - Prob. 46ECh. 6.1 - Prob. 47ECh. 6.1 - Prob. 48ECh. 6.1 - Prob. 49ECh. 6.1 - Prob. 50ECh. 6.1 - Prob. 51ECh. 6.1 - Prob. 52ECh. 6.1 - Prob. 53ECh. 6.1 - Prob. 54ECh. 6.1 - Prob. 55ECh. 6.1 - Prob. 56ECh. 6.1 - Marginal cost Consider the following marginal cost...Ch. 6.1 - Prob. 58ECh. 6.1 - Explain why or why not Determine whether the...Ch. 6.1 - Prob. 60ECh. 6.1 - Prob. 61ECh. 6.1 - Prob. 62ECh. 6.1 - Prob. 63ECh. 6.1 - Prob. 64ECh. 6.1 - Prob. 65ECh. 6.1 - Prob. 66ECh. 6.1 - Prob. 67ECh. 6.1 - Variable gravity At Earths surface, the...Ch. 6.1 - Prob. 69ECh. 6.1 - Prob. 70ECh. 6.1 - Another look at the Fundamental Theorem 71. Use...Ch. 6.1 - Prob. 72ECh. 6.2 - In the area formula for a region between two...Ch. 6.2 - Prob. 2QCCh. 6.2 - Prob. 3QCCh. 6.2 - Prob. 4QCCh. 6.2 - Set up a sum of two integrals that equals the area...Ch. 6.2 - Set up an integral that equals the area of the...Ch. 6.2 - Make a sketch to show a case in which the area...Ch. 6.2 - Make a sketch to show a case in which the area...Ch. 6.2 - Find the area of the region (see figure) in two...Ch. 6.2 - Find the area of the region (see figure) in two...Ch. 6.2 - Express the area of the shaded region in Exercise...Ch. 6.2 - Prob. 8ECh. 6.2 - Finding area Determine the area of the shaded...Ch. 6.2 - Prob. 10ECh. 6.2 - Finding area Determine the area of the shaded...Ch. 6.2 - Prob. 12ECh. 6.2 - Finding area Determine the area of the shaded...Ch. 6.2 - Prob. 14ECh. 6.2 - Finding area Determine the area of the shaded...Ch. 6.2 - Prob. 16ECh. 6.2 - Finding area Determine the area of the shaded...Ch. 6.2 - Prob. 18ECh. 6.2 - Finding area Determine the area of the shaded...Ch. 6.2 - Finding area Determine the area of the shaded...Ch. 6.2 - Finding area Determine the area of the shaded...Ch. 6.2 - Prob. 22ECh. 6.2 - Finding area Determine the area of the shaded...Ch. 6.2 - Prob. 24ECh. 6.2 - Finding area Determine the area of the shaded...Ch. 6.2 - Prob. 26ECh. 6.2 - Finding area Determine the area of the shaded...Ch. 6.2 - Finding area Determine the area of the shaded...Ch. 6.2 - Finding area Determine the area of the shaded...Ch. 6.2 - Prob. 30ECh. 6.2 - Prob. 31ECh. 6.2 - Prob. 32ECh. 6.2 - Area between velocity curves Two runners, starting...Ch. 6.2 - Prob. 34ECh. 6.2 - Prob. 35ECh. 6.2 - Calculus and geometry For the given regions R1 and...Ch. 6.2 - Regions between curves Find the area of the region...Ch. 6.2 - Prob. 38ECh. 6.2 - Regions between curves Find the area of the region...Ch. 6.2 - Regions between curves Find the area of the region...Ch. 6.2 - Regions between curves Find the area of the region...Ch. 6.2 - Regions between curves Find the area of the region...Ch. 6.2 - Regions between curves Find the area of the region...Ch. 6.2 - Prob. 44ECh. 6.2 - Regions between curves Find the area of the region...Ch. 6.2 - Regions between curves Find the area of the region...Ch. 6.2 - Prob. 47ECh. 6.2 - Prob. 48ECh. 6.2 - Any method Use any method (including geometry) to...Ch. 6.2 - Prob. 50ECh. 6.2 - Prob. 51ECh. 6.2 - Prob. 52ECh. 6.2 - Regions between curves Find the area of the region...Ch. 6.2 - Regions between curves Find the area of the region...Ch. 6.2 - Regions between curves Find the area of the region...Ch. 6.2 - Prob. 56ECh. 6.2 - Regions between curves Find the area of the region...Ch. 6.2 - Prob. 58ECh. 6.2 - Prob. 59ECh. 6.2 - Prob. 60ECh. 6.2 - Regions between curves Find the area of the region...Ch. 6.2 - Prob. 62ECh. 6.2 - Prob. 63ECh. 6.2 - Prob. 64ECh. 6.2 - Prob. 65ECh. 6.2 - Prob. 66ECh. 6.2 - Prob. 67ECh. 6.2 - Prob. 68ECh. 6.2 - Prob. 69ECh. 6.2 - Prob. 70ECh. 6.2 - Prob. 71ECh. 6.2 - Prob. 72ECh. 6.2 - Prob. 73ECh. 6.2 - Prob. 74ECh. 6.2 - Prob. 75ECh. 6.2 - Prob. 76ECh. 6.2 - Prob. 77ECh. 6.2 - Prob. 78ECh. 6.3 - Why is the volume as given by the general slicing...Ch. 6.3 - In Example 2 what is the cross-sectional area...Ch. 6.3 - What solid results when the region R is revolved...Ch. 6.3 - Prob. 4QCCh. 6.3 - Prob. 5QCCh. 6.3 - Prob. 6QCCh. 6.3 - Suppose a cut is made through a solid object...Ch. 6.3 - A solid has a circular base and cross sections...Ch. 6.3 - Consider a solid whose base is the region in the...Ch. 6.3 - Why is the disk method a special case of the...Ch. 6.3 - Prob. 5ECh. 6.3 - Prob. 6ECh. 6.3 - Use the region R that is bounded by the graphs of...Ch. 6.3 - Use the region R that is bounded by the graphs of...Ch. 6.3 - Use the region R that is bounded by the graphs of...Ch. 6.3 - Prob. 10ECh. 6.3 - General slicing method Use the general slicing...Ch. 6.3 - General slicing method Use the general slicing...Ch. 6.3 - General slicing method Use the general slicing...Ch. 6.3 - General slicing method Use the general slicing...Ch. 6.3 - General slicing method Use the general slicing...Ch. 6.3 - Prob. 16ECh. 6.3 - Disk method Let R be the region bounded by the...Ch. 6.3 - Disk method Let R be the region bounded by the...Ch. 6.3 - Disk method Let R be the region bounded by the...Ch. 6.3 - Solids of revolution Let R be the region bounded...Ch. 6.3 - Solids of revolution Let R be the region bounded...Ch. 6.3 - Disks/washers about the y-axis Let R be the region...Ch. 6.3 - Washer method Let R be the region bounded by the...Ch. 6.3 - Washer method Let R be the region bounded by the...Ch. 6.3 - Washer method Let R be the region bounded by the...Ch. 6.3 - Washer method Let R be the region bounded by the...Ch. 6.3 - Solids of revolution Let R be the region bounded...Ch. 6.3 - Disks/washers about the y-axis Let R be the region...Ch. 6.3 - Disk method Let R be the region bounded by the...Ch. 6.3 - Prob. 30ECh. 6.3 - Disk method Let R be the region bounded by the...Ch. 6.3 - Solids of revolution Let R be the region bounded...Ch. 6.3 - Solids of revolution Let R be the region bounded...Ch. 6.3 - Washer method Let R be the region bounded by the...Ch. 6.3 - Washer method Let R be the region bounded by the...Ch. 6.3 - Washer method Let R be the region bounded by the...Ch. 6.3 - Disks/washers about the y-axis Let R be the region...Ch. 6.3 - Solids of revolution Let R be the region bounded...Ch. 6.3 - Solids of revolution Let R be the region bounded...Ch. 6.3 - Solids of revolution Let R be the region bounded...Ch. 6.3 - Solids of revolution Let R be the region bounded...Ch. 6.3 - Solids of revolution Let R be the region bounded...Ch. 6.3 - Solids of revolution Let R be the region bounded...Ch. 6.3 - Solids of revolution Let R be the region bounded...Ch. 6.3 - Which is greater? For the following regions R,...Ch. 6.3 - Which is greater? For the following regions R,...Ch. 6.3 - Prob. 47ECh. 6.3 - Prob. 48ECh. 6.3 - Revolution about other axes Let R be the region...Ch. 6.3 - Prob. 50ECh. 6.3 - Revolution about other axes Let R be the region...Ch. 6.3 - Prob. 52ECh. 6.3 - Prob. 53ECh. 6.3 - Prob. 54ECh. 6.3 - Revolution about other axes Find the volume of the...Ch. 6.3 - Revolution about other axes Find the volume of the...Ch. 6.3 - Prob. 57ECh. 6.3 - Prob. 58ECh. 6.3 - Prob. 59ECh. 6.3 - Prob. 60ECh. 6.3 - Prob. 61ECh. 6.3 - Prob. 62ECh. 6.3 - Prob. 63ECh. 6.3 - Prob. 64ECh. 6.3 - Prob. 65ECh. 6.3 - Prob. 66ECh. 6.3 - Prob. 67ECh. 6.3 - Volume of a wooden object A solid wooden object...Ch. 6.3 - Prob. 69ECh. 6.3 - Water in a bowl A hemispherical bowl of radius 8...Ch. 6.3 - Prob. 71ECh. 6.3 - Prob. 72ECh. 6.3 - Cavalieris principle Cavalieris principle states...Ch. 6.3 - Prob. 74ECh. 6.4 - The triangle bounded by the x-axis, the line y =...Ch. 6.4 - Prob. 2QCCh. 6.4 - Prob. 3QCCh. 6.4 - Assume f and g are continuous with f(x) g(x) on...Ch. 6.4 - Fill in the blanks: A region R is revolved about...Ch. 6.4 - Fill in the blanks: A region R is revolved about...Ch. 6.4 - Look again at the region R in Figure 6.38 (p 439)....Ch. 6.4 - Let R be the region in the first quadrant bounded...Ch. 6.4 - Let R be the region bounded by the curves...Ch. 6.4 - Prob. 7ECh. 6.4 - Let R be the region bounded by the curves...Ch. 6.4 - Shell method Let R be the region bounded by the...Ch. 6.4 - Prob. 10ECh. 6.4 - Shell method Let R be the region bounded by the...Ch. 6.4 - Prob. 12ECh. 6.4 - Shell method Let R be the region bounded by the...Ch. 6.4 - Prob. 14ECh. 6.4 - Shell method Let R be the region bounded by the...Ch. 6.4 - Prob. 16ECh. 6.4 - Shell method Let R be the region bounded by the...Ch. 6.4 - Prob. 18ECh. 6.4 - Shell method Let R be the region bounded by the...Ch. 6.4 - Prob. 20ECh. 6.4 - Shell method Let R be the region bounded by the...Ch. 6.4 - Shell method Let R be the region bounded by the...Ch. 6.4 - Shell method Let R be the region bounded by the...Ch. 6.4 - Shell method Let R be the region bounded by the...Ch. 6.4 - Prob. 25ECh. 6.4 - Prob. 26ECh. 6.4 - Shell method Let R be the region bounded by the...Ch. 6.4 - Prob. 28ECh. 6.4 - Prob. 29ECh. 6.4 - Prob. 30ECh. 6.4 - Prob. 31ECh. 6.4 - Prob. 32ECh. 6.4 - Prob. 33ECh. 6.4 - Prob. 34ECh. 6.4 - Washers vs. shells Let R be the region bounded by...Ch. 6.4 - Prob. 36ECh. 6.4 - Washers vs. shells Let R be the region bounded by...Ch. 6.4 - Prob. 38ECh. 6.4 - Shell method about other lines Let R be the region...Ch. 6.4 - Prob. 40ECh. 6.4 - Prob. 41ECh. 6.4 - Prob. 42ECh. 6.4 - Prob. 43ECh. 6.4 - Prob. 44ECh. 6.4 - Prob. 45ECh. 6.4 - Prob. 46ECh. 6.4 - Prob. 47ECh. 6.4 - Prob. 48ECh. 6.4 - Volume of a sphere Let R be the region bounded by...Ch. 6.4 - Prob. 50ECh. 6.4 - A torus (doughnut) A torus is formed when a circle...Ch. 6.4 - Prob. 52ECh. 6.4 - Prob. 53ECh. 6.4 - Prob. 54ECh. 6.4 - Choose your method Find the volume of the...Ch. 6.4 - Prob. 56ECh. 6.4 - Prob. 57ECh. 6.4 - Prob. 58ECh. 6.4 - Choose your method Let R be the region bounded by...Ch. 6.4 - Prob. 60ECh. 6.4 - Choose your method Let R be the region bounded by...Ch. 6.4 - Prob. 62ECh. 6.4 - Prob. 63ECh. 6.4 - Prob. 64ECh. 6.4 - Prob. 65ECh. 6.4 - Prob. 66ECh. 6.4 - Prob. 67ECh. 6.4 - Prob. 68ECh. 6.4 - Prob. 69ECh. 6.4 - Prob. 70ECh. 6.4 - Prob. 71ECh. 6.4 - Equal integrals Without evaluating integrals,...Ch. 6.4 - Volumes without calculus Solve the following...Ch. 6.4 - Prob. 74ECh. 6.4 - Prob. 75ECh. 6.4 - Prob. 76ECh. 6.5 - What does the arc length formula give for the...Ch. 6.5 - Prob. 2QCCh. 6.5 - Prob. 3QCCh. 6.5 - Prob. 1ECh. 6.5 - Prob. 2ECh. 6.5 - Setting up arc length integrals Write and...Ch. 6.5 - Setting up arc length integrals Write and...Ch. 6.5 - Setting up arc length integrals Write and...Ch. 6.5 - Prob. 6ECh. 6.5 - Prob. 7ECh. 6.5 - Prob. 8ECh. 6.5 - Arc lezngth calculations Find the arc length of...Ch. 6.5 - Arc length calculations Find the arc length of the...Ch. 6.5 - Arc length calculations Find the arc length of the...Ch. 6.5 - Arc length calculations Find the arc length of the...Ch. 6.5 - Arc length calculations Find the arc length of the...Ch. 6.5 - Arc length calculations Find the arc length of the...Ch. 6.5 - Arc length calculations Find the arc length of the...Ch. 6.5 - Arc length calculations Find the arc length of the...Ch. 6.5 - Arc length calculations with respect to y Find the...Ch. 6.5 - Arc length calculations with respect to y Find the...Ch. 6.5 - Arc length calculations with respect to y Find the...Ch. 6.5 - Arc length calculations with respect to y Find the...Ch. 6.5 - Arc length by calculator a. Write and simplify the...Ch. 6.5 - Prob. 22ECh. 6.5 - Prob. 23ECh. 6.5 - Prob. 24ECh. 6.5 - Arc length by calculator a. Write and simplify the...Ch. 6.5 - Arc length by calculator a. Write and simplify the...Ch. 6.5 - Arc length by calculator a. Write and simplify the...Ch. 6.5 - Prob. 28ECh. 6.5 - Arc length by calculator a. Write and simplify the...Ch. 6.5 - Arc length by calculator a.Write and simplify the...Ch. 6.5 - Prob. 31ECh. 6.5 - Prob. 32ECh. 6.5 - Explain why or why not Determine whether the...Ch. 6.5 - Prob. 34ECh. 6.5 - Functions from arc length What differentiable...Ch. 6.5 - Function from arc length Find a curve that passes...Ch. 6.5 - Prob. 37ECh. 6.5 - Prob. 38ECh. 6.5 - Prob. 39ECh. 6.5 - Prob. 40ECh. 6.5 - Prob. 41ECh. 6.5 - Bernoullis parabolas Johann Bernoulli (16671748)...Ch. 6.6 - Which is greater the surface area of a cone of...Ch. 6.6 - Prob. 2QCCh. 6.6 - Prob. 3QCCh. 6.6 - Prob. 1ECh. 6.6 - Prob. 2ECh. 6.6 - Prob. 3ECh. 6.6 - Prob. 4ECh. 6.6 - Prob. 5ECh. 6.6 - Prob. 6ECh. 6.6 - Prob. 7ECh. 6.6 - Prob. 8ECh. 6.6 - Computing surface areas Find the area of the...Ch. 6.6 - Computing surface areas Find the area of the...Ch. 6.6 - Revolving about the y-axis Find the area of the...Ch. 6.6 - Prob. 12ECh. 6.6 - Computing surface areas Find the area of the...Ch. 6.6 - Prob. 14ECh. 6.6 - Prob. 15ECh. 6.6 - Prob. 16ECh. 6.6 - Prob. 17ECh. 6.6 - Prob. 18ECh. 6.6 - Prob. 19ECh. 6.6 - Prob. 20ECh. 6.6 - Painting surfaces A 1.5-mm layer of paint is...Ch. 6.6 - Prob. 22ECh. 6.6 - Explain why or why not Determine whether the...Ch. 6.6 - T 2629. Surface area using technology Consider the...Ch. 6.6 - T 2629. Surface area using technology Consider the...Ch. 6.6 - Surface area using technology Consider the...Ch. 6.6 - Surface area using technology Consider the...Ch. 6.6 - Surface area using technology Consider the...Ch. 6.6 - Revolving an astroid Consider the upper half of...Ch. 6.6 - Prob. 30ECh. 6.6 - Prob. 31ECh. 6.6 - Prob. 32ECh. 6.6 - Prob. 33ECh. 6.6 - Prob. 34ECh. 6.6 - Prob. 35ECh. 6.6 - Prob. 36ECh. 6.6 - Prob. 37ECh. 6.6 - Prob. 38ECh. 6.6 - Surface-area-to-volume ratio (SAV) In the design...Ch. 6.6 - Prob. 40ECh. 6.6 - Prob. 41ECh. 6.6 - Surface plus cylinder Suppose f is a nonnegative...Ch. 6.7 - In Figure 6.69, suppose a = 0, b = 3, and the...Ch. 6.7 - Prob. 2QCCh. 6.7 - Prob. 3QCCh. 6.7 - Prob. 4QCCh. 6.7 - In Example 3b, the bucket occupies the interval...Ch. 6.7 - Prob. 6QCCh. 6.7 - Prob. 7QCCh. 6.7 - Suppose a 1-m cylindrical bar has a constant...Ch. 6.7 - Explain how to find the mass of a one-dimensional...Ch. 6.7 - How much work is required to move an object from x...Ch. 6.7 - Why is integration used to find the work done by a...Ch. 6.7 - Why is integration used to find the work required...Ch. 6.7 - Prob. 6ECh. 6.7 - What is the pressure on a horizontal surface with...Ch. 6.7 - Prob. 8ECh. 6.7 - Consider the cylindrical tank in Example 4 that...Ch. 6.7 - Consider the cylindrical tank in Example 4 that...Ch. 6.7 - Consider the cylindrical tank in Example 4 that...Ch. 6.7 - Prob. 12ECh. 6.7 - Mass of one-dimensional objects Find the mass of...Ch. 6.7 - Mass of one-dimensional objects Find the mass of...Ch. 6.7 - Mass of one-dimensional objects Find the mass of...Ch. 6.7 - Prob. 16ECh. 6.7 - Mass of one-dimensional objects Find the mass of...Ch. 6.7 - Mass of one-dimensional objects Find the mass of...Ch. 6.7 - Mass of one-dimensional objects Find the mass of...Ch. 6.7 - Prob. 20ECh. 6.7 - Work from force How much work is required to move...Ch. 6.7 - Prob. 22ECh. 6.7 - Compressing and stretching a spring Suppose a...Ch. 6.7 - Compressing and stretching a spring Suppose a...Ch. 6.7 - Prob. 25ECh. 6.7 - Prob. 26ECh. 6.7 - Prob. 27ECh. 6.7 - Prob. 28ECh. 6.7 - Calculating work for different springs Calculate...Ch. 6.7 - Prob. 30ECh. 6.7 - Winding a chain A 30-m-long chain hangs vertically...Ch. 6.7 - Prob. 32ECh. 6.7 - Winding part of a chain A 20-m-long, 50-kg chain...Ch. 6.7 - Leaky Bucket A 1-kg bucket resting on the ground...Ch. 6.7 - Emptying a swimming pool A swimming pool has the...Ch. 6.7 - Prob. 36ECh. 6.7 - Emptying a half-full cylindrical tank Suppose the...Ch. 6.7 - Prob. 38ECh. 6.7 - Emptying a conical tank A water tank is shaped...Ch. 6.7 - Prob. 40ECh. 6.7 - Filling a spherical tank A spherical water tank...Ch. 6.7 - Emptying a water trough A water trough has a...Ch. 6.7 - Emptying a water trough A cattle trough has a...Ch. 6.7 - Prob. 44ECh. 6.7 - Emptying a conical tank An inverted cone is 2 m...Ch. 6.7 - Force on dams The following figures show the shape...Ch. 6.7 - Force on dams The following figures show the shape...Ch. 6.7 - Force on dams The following figures show the shape...Ch. 6.7 - Force on dams The following figures show the shape...Ch. 6.7 - Prob. 50ECh. 6.7 - Prob. 51ECh. 6.7 - Prob. 52ECh. 6.7 - Prob. 53ECh. 6.7 - Prob. 54ECh. 6.7 - Prob. 55ECh. 6.7 - Prob. 56ECh. 6.7 - Prob. 57ECh. 6.7 - Prob. 58ECh. 6.7 - Prob. 59ECh. 6.7 - Prob. 60ECh. 6.7 - Prob. 61ECh. 6.7 - Prob. 62ECh. 6.7 - Drinking juice A glass has circular cross sections...Ch. 6.7 - Prob. 64ECh. 6.7 - Prob. 65ECh. 6.7 - Prob. 66ECh. 6.7 - Prob. 67ECh. 6.7 - Work by two different integrals A rigid body with...Ch. 6.7 - Work in a gravitational field For large distances...Ch. 6.7 - Prob. 70ECh. 6 - Explain why or why not Determine whether the...Ch. 6 - Prob. 2RECh. 6 - Prob. 3RECh. 6 - Prob. 4RECh. 6 - Prob. 5RECh. 6 - Prob. 6RECh. 6 - Prob. 7RECh. 6 - Prob. 8RECh. 6 - Prob. 9RECh. 6 - Prob. 10RECh. 6 - Prob. 11RECh. 6 - Prob. 12RECh. 6 - Prob. 13RECh. 6 - Prob. 14RECh. 6 - Prob. 15RECh. 6 - Prob. 16RECh. 6 - Prob. 17RECh. 6 - Prob. 18RECh. 6 - Prob. 19RECh. 6 - Prob. 20RECh. 6 - Prob. 21RECh. 6 - Prob. 22RECh. 6 - Prob. 23RECh. 6 - Prob. 24RECh. 6 - Prob. 25RECh. 6 - Prob. 26RECh. 6 - Prob. 27RECh. 6 - Multiple regions The regions R1, R2, and R3 (see...Ch. 6 - Prob. 29RECh. 6 - Prob. 30RECh. 6 - Prob. 31RECh. 6 - Prob. 32RECh. 6 - Prob. 33RECh. 6 - Prob. 34RECh. 6 - Prob. 35RECh. 6 - Area and volume Let R be the region in the first...Ch. 6 - Prob. 37RECh. 6 - Prob. 38RECh. 6 - Prob. 39RECh. 6 - Prob. 40RECh. 6 - Prob. 41RECh. 6 - Prob. 42RECh. 6 - Prob. 43RECh. 6 - Prob. 44RECh. 6 - Prob. 45RECh. 6 - Prob. 46RECh. 6 - Prob. 47RECh. 6 - Prob. 48RECh. 6 - Prob. 49RECh. 6 - Prob. 50RECh. 6 - Prob. 51RECh. 6 - Prob. 52RECh. 6 - Prob. 53RECh. 6 - Prob. 54RECh. 6 - Prob. 55RECh. 6 - Prob. 56RECh. 6 - Prob. 57RECh. 6 - Prob. 58RECh. 6 - Prob. 59RECh. 6 - Prob. 60RECh. 6 - Prob. 61RECh. 6 - Prob. 62RECh. 6 - Prob. 63RECh. 6 - Prob. 64RECh. 6 - Prob. 65RECh. 6 - Surface area and volume Let f(x)=13x3 and let R be...Ch. 6 - Surface area and volume Let f(x)=3xx2 and let R be...Ch. 6 - Prob. 68RECh. 6 - Surface area and more Let f(x)=x42+116x2 and let R...Ch. 6 - Prob. 70RECh. 6 - Prob. 71RECh. 6 - Prob. 72RECh. 6 - Prob. 73RECh. 6 - Leaky bucket A 1-kg bucket resting on the ground...Ch. 6 - Prob. 75RECh. 6 - Prob. 76RECh. 6 - Pumping water A water tank has the shape of a box...Ch. 6 - Prob. 78RECh. 6 - Prob. 79RECh. 6 - Prob. 80RECh. 6 - Prob. 81RECh. 6 - Prob. 82RECh. 6 - Fluid Forces Suppose the Mowing plates are placed...Ch. 6 - Prob. 84RECh. 6 - Prob. 85RECh. 6 - Prob. 86RE
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.Similar questions
- the correct answer is Ccould you please show me how to do it using the residue theoremarrow_forwardUse the information to find and compare Δy and dy. (Round your answers to four decimal places.) y = x4 + 7 x = −3 Δx = dx = 0.01 Δy = dy =arrow_forward4. A car travels in a straight line for one hour. Its velocity, v, in miles per hour at six minute intervals is shown in the table. For each problem, approximate the distance the car traveled (in miles) using the given method, on the provided interval, and with the given number of rectangles or trapezoids, n. Time (min) 0 6 12 18|24|30|36|42|48|54|60 Speed (mph) 0 10 20 40 60 50 40 30 40 40 65 a.) Left Rectangles, [0, 30] n=5 b.) Right Rectangles, [24, 42] n=3 c.) Midpoint Rectangles, [24, 60] n=3 d.) Trapezoids, [0, 24] n=4arrow_forward
- The bracket BCD is hinged at C and attached to a control cable at B. Let F₁ = 275 N and F2 = 275 N. F1 B a=0.18 m C A 0.4 m -0.4 m- 0.24 m Determine the reaction at C. The reaction at C N Z F2 Darrow_forwardThe correct answer is C,i know that we need to use stokes theorem and parametrize the equations then write the equation F with respect to the curve but i cant seem to find a way to do it, the integral should be from 0 to 2pi but i might be wrongcould you show me the steps to get to 18piarrow_forwardA 10-ft boom is acted upon by the 810-lb force as shown in the figure. D 6 ft 6 ft E B 7 ft C 6 ft 4 ft W Determine the tension in each cable and the reaction at the ball-and-socket joint at A. The tension in cable BD is lb. The tension in cable BE is lb. The reaction at A is ( lb) i + Ib) j. (Include a minus sign if necessary.)arrow_forward
- the correct answer is A could you show me whyarrow_forwardGood Day, Kindly assist me with this query.arrow_forwardon donne f(x) da fonction derive dhe do fonction fcsos calcule f'(x) orans chacun des Cas sulants: 3 1) f(x)=5x-11, 2- f (x) = ->³ 3-1(x) = x² 12x +π; 4-f(x)=- 5-f(x) = 33-4x6-609)=-3x²+ 7= f(x) = x + 1.8-f(x) = 4 s-f(x) = x++ X+1 -x-1 2 I 3x-4 девоarrow_forward
- The correct answer is Ccould you show me how to do it by finding a0 and and akas well as setting up the piecewise function and integratingarrow_forwardT 1 7. Fill in the blanks to write the calculus problem that would result in the following integral (do not evaluate the interval). Draw a graph representing the problem. So π/2 2 2πxcosx dx Find the volume of the solid obtained when the region under the curve on the interval is rotated about the axis.arrow_forward38,189 5. Draw a detailed graph to and set up, but do not evaluate, an integral for the volume of the solid obtained by rotating the region bounded by the curve: y = cos²x_for_ |x| ≤ and the curve y y = about the line x = =플 2 80 F3 a FEB 9 2 7 0 MacBook Air 3 2 stv DGarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
 Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage, Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning
Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning
Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,

Elementary Geometry For College Students, 7e
Geometry
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Cengage,

Functions and Change: A Modeling Approach to Coll...
Algebra
ISBN:9781337111348
Author:Bruce Crauder, Benny Evans, Alan Noell
Publisher:Cengage Learning

Elementary Geometry for College Students
Geometry
ISBN:9781285195698
Author:Daniel C. Alexander, Geralyn M. Koeberlein
Publisher:Cengage Learning

Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning

Holt Mcdougal Larson Pre-algebra: Student Edition...
Algebra
ISBN:9780547587776
Author:HOLT MCDOUGAL
Publisher:HOLT MCDOUGAL

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,
Area Between The Curve Problem No 1 - Applications Of Definite Integration - Diploma Maths II; Author: Ekeeda;https://www.youtube.com/watch?v=q3ZU0GnGaxA;License: Standard YouTube License, CC-BY