
Equal area properties for parabolas Consider the parabola y = x2. Let P, Q, and R be points on the parabola with R between P and Q on the curve. Let ℓP, ℓQ, and ℓR be the lines tangent to the parabola at P, Q, and R, respectively (see figure). Let P′ be the intersection point of ℓQ and ℓR, let Q′ be the intersection point of ℓP and ℓR, and let R′ be the intersection point of ℓP and ℓQ. Prove that Area ΔPQR = 2 · Area ΔP′Q′R′ in the following cases. (In fact, the property holds for any three points on any parabola.) (Source: Mathematics Magazine 81, 2, Apr 2008)
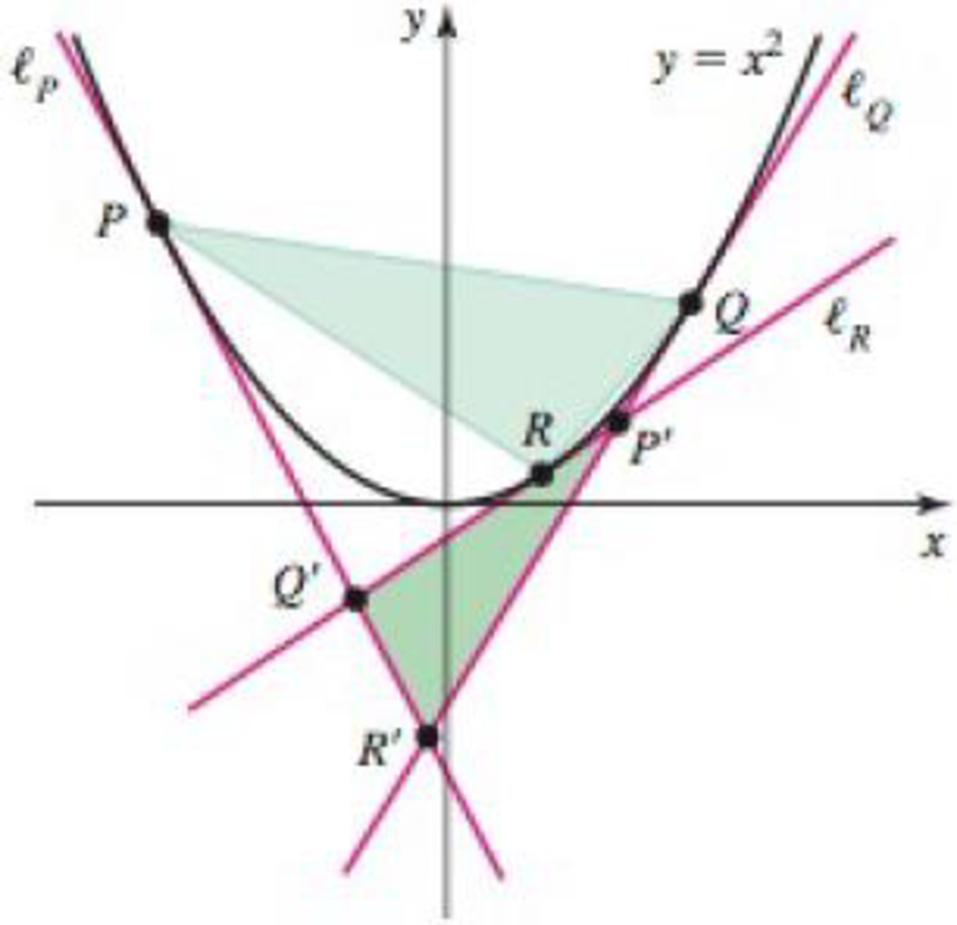
- a. P(−a, a2), Q(a, a2), and R(0, 0), where a is a positive real number
- b. P(−a, a2), Q(b, b2), and R(0, 0), where a and b are positive real numbers
- c. P(−a, a2), Q(b, b2), and R is any point between P and Q on the curve
Want to see the full answer?
Check out a sample textbook solution
Chapter 6 Solutions
Single Variable Calculus: Early Transcendentals (2nd Edition) - Standalone book
Additional Math Textbook Solutions
Elementary Statistics: Picturing the World (7th Edition)
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
Pre-Algebra Student Edition
A First Course in Probability (10th Edition)
College Algebra with Modeling & Visualization (5th Edition)
University Calculus: Early Transcendentals (4th Edition)
- help pleasearrow_forwardIn each of Problems 1 through 4, draw a direction field for the given differential equation. Based on the direction field, determine the behavior of y as t → ∞. If this behavior depends on the initial value of y at t = 0, describe the dependency.1. y′ = 3 − 2yarrow_forwardB 2- The figure gives four points and some corresponding rays in the xy-plane. Which of the following is true? A B Angle COB is in standard position with initial ray OB and terminal ray OC. Angle COB is in standard position with initial ray OC and terminal ray OB. C Angle DOB is in standard position with initial ray OB and terminal ray OD. D Angle DOB is in standard position with initial ray OD and terminal ray OB.arrow_forward
- temperature in degrees Fahrenheit, n hours since midnight. 5. The temperature was recorded at several times during the day. Function T gives the Here is a graph for this function. To 29uis a. Describe the overall trend of temperature throughout the day. temperature (Fahrenheit) 40 50 50 60 60 70 5 10 15 20 25 time of day b. Based on the graph, did the temperature change more quickly between 10:00 a.m. and noon, or between 8:00 p.m. and 10:00 p.m.? Explain how you know. (From Unit 4, Lesson 7.) 6. Explain why this graph does not represent a function. (From Unit 4, Lesson 8.)arrow_forwardFind the area of the shaded region. (a) 5- y 3 2- (1,4) (5,0) 1 3 4 5 6 (b) 3 y 2 Decide whether the problem can be solved using precalculus, or whether calculus is required. If the problem can be solved using precalculus, solve it. If the problem seems to require calculus, use a graphical or numerical approach to estimate the solution. STEP 1: Consider the figure in part (a). Since this region is simply a triangle, you may use precalculus methods to solve this part of the problem. First determine the height of the triangle and the length of the triangle's base. height 4 units units base 5 STEP 2: Compute the area of the triangle by employing a formula from precalculus, thus finding the area of the shaded region in part (a). 10 square units STEP 3: Consider the figure in part (b). Since this region is defined by a complicated curve, the problem seems to require calculus. Find an approximation of the shaded region by using a graphical approach. (Hint: Treat the shaded regi as…arrow_forwardSolve this differential equation: dy 0.05y(900 - y) dt y(0) = 2 y(t) =arrow_forward
- Suppose that you are holding your toy submarine under the water. You release it and it begins to ascend. The graph models the depth of the submarine as a function of time. What is the domain and range of the function in the graph? 1- t (time) 1 2 4/5 6 7 8 -2 -3 456700 -4 -5 -6 -7 d (depth) -8 D: 00 t≤ R:arrow_forward0 5 -1 2 1 N = 1 to x = 3 Based on the graph above, estimate to one decimal place the average rate of change from x =arrow_forwardComplete the description of the piecewise function graphed below. Use interval notation to indicate the intervals. -7 -6 -5 -4 30 6 5 4 3 0 2 1 -1 5 6 + -2 -3 -5 456 -6 - { 1 if x Є f(x) = { 1 if x Є { 3 if x Єarrow_forwardComplete the description of the piecewise function graphed below. 6 5 -7-6-5-4-3-2-1 2 3 5 6 -1 -2 -3 -4 -5 { f(x) = { { -6 if -6x-2 if -2< x <1 if 1 < x <6arrow_forwardLet F = V where (x, y, z) x2 1 + sin² 2 +z2 and let A be the line integral of F along the curve x = tcost, y = t sint, z=t, starting on the plane z = 6.14 and ending on the plane z = 4.30. Then sin(3A) is -0.598 -0.649 0.767 0.278 0.502 0.010 -0.548 0.960arrow_forwardLet C be the intersection of the cylinder x² + y² = 2.95 with the plane z = 1.13x, with the clockwise orientation, as viewed from above. Then the value of cos (₤23 COS 2 y dx xdy+3 z dzis 3 z dz) is 0.131 -0.108 -0.891 -0.663 -0.428 0.561 -0.332 -0.387arrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_ios
- Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
