
To show that side ST is perpendicular to side BD.
Explanation of Solution
Given information:
A, B, C and D lie in plane m .
D is any point on
Formula used:
The below properties are used:
If a segment divides a segment into two congruent segments, it is a bisector.
A point on a perpendicular bisector of a segment is equal to distance from ends of the segments.
Two
Two
A point equal distance from endpoints of a segment is on the perpendicular bisector of the segment.
Proof:
It is given that,
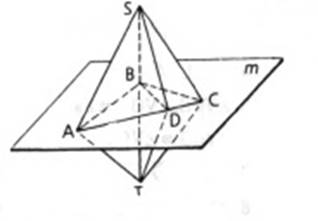
If a segment divides a segment into two congruent segments, it is a bisector.
A point on a perpendicular bisector of a segment is equal to distance from ends of the segments.
By reflexive property, we get
By SSS congruence rule, we get
As corresponding parts of congruent triangles are congruent, we get
By reflexive property, we get
By SAS congruence rule, we get
As corresponding parts of congruent triangles are congruent, we get
A point equal distance from endpoints of a segment is on the perpendicular bisector of the segment.
According to definition of perpendicular bisector
Chapter 6 Solutions
Geometry For Enjoyment And Challenge
Additional Math Textbook Solutions
Precalculus
Elementary Statistics: Picturing the World (7th Edition)
A First Course in Probability (10th Edition)
Elementary Statistics
Elementary Statistics (13th Edition)
Intro Stats, Books a la Carte Edition (5th Edition)
- Door 87.5in to 47 living 44.75 Closet 96in Window ISS.Sin 48in Train Table 96in 48in 132:2 Windowarrow_forward39 Two sides of one triangle are congruent to two sides of a second triangle, and the included angles are supplementary. The area of one triangle is 41. Can the area of the second triangle be found?arrow_forwardA parallelogram with an area of 211.41 m^2 hast a base Thatcher measures 24.3m. Find ist height.arrow_forward
 Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage, Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning
Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning

