
(a)
Interpretation:
Calculate values of
Concept Introduction:
The Gibbs free energy is calculated as:
(a)
Answer to Problem 6.46P
Explanation of Solution
Given information:
It is given that pressure is
From steam tables of saturated steam in Appendix E, table E.1
At pressure
Since, pressure
From linear interpolation, if
Temperature corresponding to
Enthalpy of saturated liquid and entropy of saturated liquid and vapor is
And,
Entropy of saturated liquid and entropy of saturated liquid and vapor is
And,
So,
And
Both values of
(b)
Interpretation:
Calculate values for
Concept Introduction:
The change in enthalpy and entropy in ideal gas is defined as:
And
(b)
Answer to Problem 6.46P
Explanation of Solution
From subpart (a), values of enthalpies of saturated vapor and liquid as well as values of entropies of saturated vapor and liquid are:
And temperature is:
So,
And
Both values of
(c)
Interpretation:
Calculate values for
Concept Introduction:
The residual properties
The residual properties
The residual properties
(c)
Answer to Problem 6.46P
Explanation of Solution
At
So,
Now, for the hypothetical ideal gas values of volume, enthalpy and entropy at same temperature and pressure, it cannot find using steam table because steam tables only give values of real gases not ideal gases. However, we can make an approximation of low pressure in real gases at the same temperature to convert real gas into ideal one. So, we are considering low pressure or
Since,
Hence from linear interpolation, at
But we want values of volume, enthalpy and entropy of ideal gas at
And
Enthalpy of ideal gas is defined as
Which is not the function of pressure, so enthalpy of ideal gas at
Now,
For entropy we know that
Since temperature s constant so first term at right hand side will be zero and hence,
So,
Residual properties are:
And
And
Now calculations of residual properties of volume, enthalpy and entropy for saturated vapor from generalized correlations are given below,
....(1a)
Where,
....(1b)
....(2a)
Where,
....(2b)
And
....(3a)
Where
....(3b)
Properties of pure species of steam are given in Table B.1 Appendix B as water,
So,
And
For differentiative terms in equation (2b) and (3b),
And,
For residual volume calculations, From equation (1b)
So, from equation (1a)
For residual enthalpy calculations, From equation (2b)
So, from equation (2a)
For residual entropy calculations, from equation (3b)
So, from equation (3a)
Results do not agree but approximately they do agree.
(d)
Interpretation:
Calculate values for
Concept Introduction:
First draw a graph between
The Clapeyron equation is:
(d)
Answer to Problem 6.46P
Explanation of Solution
From saturated steam table in Appendix E, table E.2
Now, draw a graph between
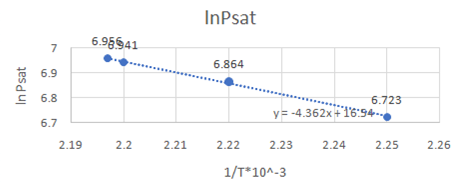
Slope of the graph from equation of graph is
Or,
So,
Also,
Volume of saturated liquid and entropy of saturated liquid and vapor at pressure
Since, pressure
And,
So,
Now from Clapeyron equation,
The value of
So, the results approximately match with each other.
Want to see more full solutions like this?
Chapter 6 Solutions
EBK INTRODUCTION TO CHEMICAL ENGINEERIN
- 1. The settling chamber, shown schematically in Figure 2E1.1, is used as a primary separation device in the removal of dust particles of density 1500 kg/m³ from a gas of density 0:7 kg/m³ and viscosity 1.90 x 10-5 Pa s. Gas inlet Elevation Gas Gas exit exit H Collection surface -W Section X-X Dimensions: H=3m L = 10 m W=2m Figure 2E1.1 Schematic diagram of settling chamber Assuming Stokes' law applies, show that the efficiency of collection of particles of size x is given by the expression collection efficiency, x = x²8(pp - Pi)L 18μHU where U is the uniform gas velocity through the parallel-sided section of the chamber. State any other assumptions made. (b) What is the upper limit of particle size for which Stokes' law applies? (c) When the volumetric flow rate of gas is 0.9 m³/s, and the dimensions of the chamber are those shown in Figure 2E1.1, determine the collection efficiency for spherical particles of diameter 30 mm.arrow_forwardCan you answer this sequantially correct like show me the full process. Also, since it is chemical engineering related problem a perry's handbook is used. Thank youarrow_forwardchemical engineering Demonstrate how each specific enthalpy was calculated, from the reference state to the process state. Be thorough to the fullest. This is a material-energy balance. The answers are H(1) = 35.7 KJ/kmol, H(2) = 32.0 KJ/kmol, and H(3) = -1.26 KJ/kmol.arrow_forward
- Do question 9 please! Question 7 Is just there for reference!!arrow_forward7) You are tasked with separating two proteins by ion exchange chromatography on a 30 cm long column with an inner diameter of 2 cm. The resin has a diameter of 100 μm and a void fraction of 0.3, and your mobile phase flows through the column at a rate of Q = 5 cm³/min. The Van Deemter coefficients A, B, and C have been determined to be 0.0228 cm, 0.0036 cm²/min, and 0.00053 min, respectively, for both proteins. Protein A elutes from the column with an average retention time of 27 min and standard deviation of 0.8 min. Protein B elutes from the column. with an average retention time of 33.8 min and standard deviation of 1.0. a) How many theoretical plates does the column contain? b) What flow rate (Q) will give you the maximum resolution? c) What is the minimum height of a theoretical plate for the system?arrow_forward4) A fixed bed adsorption unit contains rigid (incompressible) silica particles with a diameter of 120 um and porosity of 0.3. The resin bed is 200 cm long and has a diameter of 15 cm. A protein solution is pumped into the column at a rate of 50 L/min, and the mobile phase has a viscosity of 1.2 CP. a) What is the pressure drop for this system (in bar)? b) What would be the pressure drop if the particle diameter were decreased to 30 μm?arrow_forward
- You are part of a team constructing a pipeline to transfer shale gas produced at the oceanfloor to the coastline. The temperature of the pipeline is nearly constant at 2 oC. The pipelineis made of smooth stainless steel and is 0.3 m in diameter and 100 m long. The averagevelocity of shale gas is 10 m/s and the inlet temperature is 20 oC ** Useful shale gas properties at 20 oC (Table A-12 for propane gas):(use these values for calculations and validate them later)• Density (ρ) = 18.13 kg/m3• Cp = 1974 J/kg-K• Viscosity (μ) = 8.54*10-6 kg/m-s• Pr = 0.918• k = 0.01836 W/m-Ka) Is the flow laminar or turbulent? Is the flow hydrodynamically and thermally fully developed?(circle your answer below and provide justification. • Laminar vs. Turbulent• Hydrodynamically developing vs. developed• Thermally developing vs. fully developedJustification: b) Calculate convective heat transfer coefficient (h). c) Calculate the exit temperature of the shale gas. d) Are the shale gas properties…arrow_forward3) A pilot-plant Podbielniak centrifugal extractor operating at 11,400 x g (this is G₁) is capable of processing 500 mL/min of filtered fermentation broth and 125 mL/min organic solvent, giving a recovery of 95%. The rotating cylinder inside the extractor has a diameter of 20 cm and is 2.5 cm wide. You need to scale up this extraction by using a larger Podbielniak extractor that has a diameter of 91 cm and width of 91 cm and delivers 2,300 x g (G2). What flow rates (in L/min) should be used in the larger extractor to achieve the same recovery efficiency?arrow_forward7) You are tasked with separating two proteins by ion exchange chromatography on a 30 cm long column with an inner diameter of 2 cm. The resin has a diameter of 100 μm and a void fraction of 0.3, and your mobile phase flows through the column at a rate of Q = 5 cm³/min. The Van Deemter coefficients A, B, and C have been determined to be 0.0228 cm, 0.0036 cm²/min, and 0.00053 min, respectively, for both proteins. Protein A elutes from the column with an average retention time of 27 min and standard deviation of 0.8 min. Protein B elutes from the column. with an average retention time of 33.8 min and standard deviation of 1.0. a) How many theoretical plates does the column contain? b) What flow rate (Q) will give you the maximum resolution? c) What is the minimum height of a theoretical plate for the system?arrow_forward
 Introduction to Chemical Engineering Thermodynami...Chemical EngineeringISBN:9781259696527Author:J.M. Smith Termodinamica en ingenieria quimica, Hendrick C Van Ness, Michael Abbott, Mark SwihartPublisher:McGraw-Hill Education
Introduction to Chemical Engineering Thermodynami...Chemical EngineeringISBN:9781259696527Author:J.M. Smith Termodinamica en ingenieria quimica, Hendrick C Van Ness, Michael Abbott, Mark SwihartPublisher:McGraw-Hill Education Elementary Principles of Chemical Processes, Bind...Chemical EngineeringISBN:9781118431221Author:Richard M. Felder, Ronald W. Rousseau, Lisa G. BullardPublisher:WILEY
Elementary Principles of Chemical Processes, Bind...Chemical EngineeringISBN:9781118431221Author:Richard M. Felder, Ronald W. Rousseau, Lisa G. BullardPublisher:WILEY Elements of Chemical Reaction Engineering (5th Ed...Chemical EngineeringISBN:9780133887518Author:H. Scott FoglerPublisher:Prentice Hall
Elements of Chemical Reaction Engineering (5th Ed...Chemical EngineeringISBN:9780133887518Author:H. Scott FoglerPublisher:Prentice Hall
 Industrial Plastics: Theory and ApplicationsChemical EngineeringISBN:9781285061238Author:Lokensgard, ErikPublisher:Delmar Cengage Learning
Industrial Plastics: Theory and ApplicationsChemical EngineeringISBN:9781285061238Author:Lokensgard, ErikPublisher:Delmar Cengage Learning Unit Operations of Chemical EngineeringChemical EngineeringISBN:9780072848236Author:Warren McCabe, Julian C. Smith, Peter HarriottPublisher:McGraw-Hill Companies, The
Unit Operations of Chemical EngineeringChemical EngineeringISBN:9780072848236Author:Warren McCabe, Julian C. Smith, Peter HarriottPublisher:McGraw-Hill Companies, The





