
Concept explainers
A single bead can slide with negligible friction on a stiff wire that has been bent into a circular loop of radius 15.0 cm as shown in Figure P6.48. The circle is always in a vertical plane and rotates steadily about its vertical diameter with a period of 0.450 s. The position of the bead is described by the angle θ that the radial line, from the center of the loop to the bead, makes with the vertical. (a) At what angle up from the bottom of the circle can the bead slay motionless relative to the turning circle? (b) What If? Repeat the problem, this time taking the period of the circle’s rotation as 0.850 s. (c) Describe how the solution to part (b) is different from the solution to part (a). (d) For any period or loop size, is there always an angle at which the bead can stand still relative to the loop? (e) Are there ever more than two angles? Arnold Arons suggested the idea for this problem.
Figure P6.48
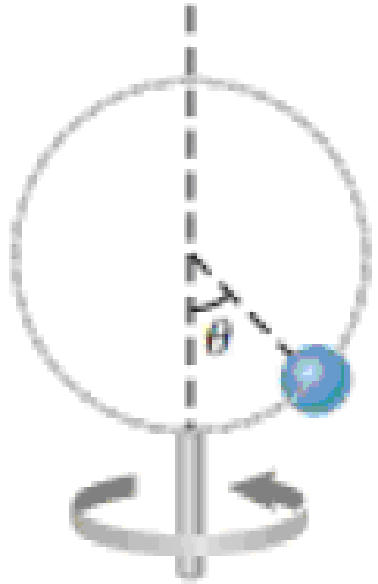
(a)
The angle from the bottom of the circle for which the bead can stay motionless.
Answer to Problem 48CP
The angles from the bottom of the circle for which the bead can stay motionless are
Explanation of Solution
Given info: The radius of the circular loop is
The acceleration due to gravity is
The rough sketch of the force body diagram of the situation is shown below,
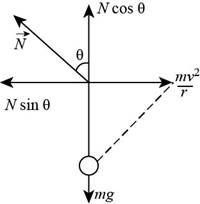
Figure (1)
The bead moves in a circle,
Here,
The speed of the bead is,
Here,
From the figure (1) the net force in
Here,
The net force in
Substitute
Substitute
The two possible solutions are,
and,
Substitute
Conclusion:
Therefore, the angles from the bottom of the circle for which the bead can stay motionless are
(b)
The angles from the bottom of the circle for which the bead can stay motionless for the time period as
Answer to Problem 48CP
The only one possible angle from the bottom of the circle for which the bead can stay motionless for time period
Explanation of Solution
Given info: The radius of the circular loop is
The acceleration due to gravity is
Form the part (a) equation (1).
The possible solutions are,
and,
Substitute
The above value is not possible.
Conclusion:
Therefore, the only one possible angle from the bottom of the circle for which the bead can stay motionless at time period
(c)
The difference between the solution of the part (a) and part (b).
Answer to Problem 48CP
The part (b) has only one solution as the time period is very large.
Explanation of Solution
Given info: The radius of the circular loop is
Form equation (1)
The possible solutions are,
and,
As the second solution depends directly on the square of the amplitude of the time period so, as the time period increases the value of
Conclusion:
Therefore, the part (b) has only one solution as the time period is very large.
(d)
The angle at which the bead can stand still relative to the loop.
Answer to Problem 48CP
The angle for which condition
Explanation of Solution
Given info: The radius of the circular loop is
Form equation (1)
The possible solutions are,
and,
As the range of the cosine function is
So, the value of the cosine of the angle is always less than
Conclusion:
Therefore, for the condition
(e)
Whether there are more than two angles.
Answer to Problem 48CP
The number of possible angles are
Explanation of Solution
Given info: The radius of the circular loop is
Form equation (1)
The possible solutions are,
and,
Form the above expression the second solution depends on time period, acceleration due to gravity and the radius of the hoop and for a given case the parameters has only one value so the second solution has only one answer.
Conclusion:
Therefore, the number of possible angles are
Want to see more full solutions like this?
Chapter 6 Solutions
Bundle: Physics For Scientists And Engineers With Modern Physics, Loose-leaf Version, 10th + Webassign Printed Access Card For Serway/jewett's Physics For Scientists And Engineers, 10th, Single-term
Additional Science Textbook Solutions
College Physics: A Strategic Approach (3rd Edition)
Campbell Biology (11th Edition)
Human Biology: Concepts and Current Issues (8th Edition)
Introductory Chemistry (6th Edition)
Fundamentals Of Thermodynamics
- Ho propel 9-kN t. Boat 27. An elevator accelerates downward at 2.4 m/s². What force does the elevator's floor exert on a 52-kg passenger?arrow_forward16. 17 A CUIN Starting from rest and undergoing constant acceleration, a 940-kg racing car covers 400 m in 4.95 s. Find the force on the car.arrow_forward----- vertical diste Section 4.6 Newton's Third Law 31. What upward gravitational force does a 5600-kg elephant exert on Earth?arrow_forward
- 64. Two springs have the same unstretched length but different spring constants, k₁ and k₂. (a) If they're connected side by side and stretched a distance x, as shown in Fig. 4.24a, show that the force exerted by the combination is (k₁ + k₂)x. (b) If they're con- nected end to end (Fig. 4.24b) and the combination is stretched a distance x, show that they exert a force k₁k2x/(k₁ + k₂). www (a) FIGURE 4.24 Problem 65 www (b)arrow_forward65. Although we usually write Newton's second law for one-dimensional motion in the form F =ma, which holds when mass is constant, d(mv) a more fundamental version is F = . Consider an object dt whose mass is changing, and use the product rule for derivatives to show that Newton's law then takes the form F dm = ma + v dtarrow_forwardIf a proton is located on the x-axis in some coordinate system at x0 = -3.2 x 10-5 meters, what is the x-component of the Electric Field due to this proton at a position x = +3.2 x 10-5 meters and on the x axis as the y-axis is 0 giving a number of Newtons/Coulomb?arrow_forward
- Consider a single square loop of wire of area A carrying a current I in a uniform magnetic field of strength B. The field is pointing directly up the page in the plane of the page. The loop is oriented so that the plane of the loop is perpendicular to the plane of the page (this means that the normal vector for the loop is always in the plane of the page!). In the illustrations below the magnetic field is shown in red and the current through the current loop is shown in blue. The loop starts out in orientation (i) and rotates clockwise, through orientations (ii) through (viii) before returning to (i). (i) Ø I N - - I N - (iii) (iv) (v) (vii) (viii) a) [3 points] For each of the eight configurations, draw in the magnetic dipole moment vector μ of the current loop and indicate whether the torque on the dipole due to the magnetic field is clockwise (CW), counterclockwise (CCW), or zero. In which two orientations will the loop experience the maximum magnitude of torque? [Hint: Use the…arrow_forwardPlease help with calculating the impusle, thanks! Having calculated the impact and rebound velocities of the ping pong ball and the tennis ball calculate the rebounding impulse: 1.Measure the weight of the balls and determine their mass. Tennis ball: 0.57 kg Ping Pong Ball: 0.00246 kg The impulse, I, is equal to the change in momentum, Pf-Pi. Note the sign change, i.e., going down is negative and up is positive. The unit for momentum is kg-m/s. The change is momentum, impulse, is often givens the equivalent unit of N-S, Newton-Secondarrow_forward5. Three blocks, each with mass m, are connected by strings and are pulled to the right along the surface of a frictionless table with a constant force of magnitude F. The tensions in the strings connecting the masses are T1 and T2 as shown. m T1 T2 F m m How does the magnitude of tension T₁ compare to F? A) T₁ = F B) T₁ = (1/2)F C) T₁ = (1/3)F D) T₁ = 2F E) T₁ = 3Farrow_forward
- Using Coulombs Law, what is the magnitude of the electrical force between two protons located 1 meter apart from each other in Newtons?arrow_forwardCalculate the magnitude of the gravitational force between 2 protons located 1 meter apart from each other in Newtons using Newton's Law of Universal Gravitation.arrow_forwardIf the metal sphere on the Van de Graff has a charge of 0.14 Coulombs and the person has a mass of 62 kg, how much excess charge would the person need in order to levitate at a distance 25 cm from the center of the charged metal sphere if there is a distance 25 cm from the person to the sphere using Coulomb's Law to calculate the electrical force. Give your answer as the number of Coulombs (with no unit label, as usual).arrow_forward
 Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill
Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University
University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University





