
Concept explainers
Find the area under the standard
a. Between 0 and 1.50
b. Between 0 and −1.25
c. Between 1.56 and 1.96
d. Between −1.20 and −2.25
e. Between −0.06 and 0.73
f. Between 1.10 and −1.80
g. To the right of z = 1.75
h. To the right of z = −1.28
i. To the left of z = −2.12
j. To the left of z = 1.36
(a)
To find: The area under the standard normal distribution curve for
Answer to Problem 18CQ
The area under the standard normal distribution curve for
Explanation of Solution
Calculation:
Software procedure:
Use Minitab; find the area under the normal curve between 0 and 1.50 with the help of following instructions:
- Choose Graph > Probability Distribution Plot choose View Probability> OK.
- From Distribution, choose ‘Normal’ distribution.
- Enter the Mean as 0.0 and Standard deviation as 1.0.
- Click the Shaded Area tab.
- Click the picture for Middle.
- Type in the smaller value 0 for X value1 and then the larger value 1.50 for the X value2.
- Click OK.
Output using the MINITAB software is given below:
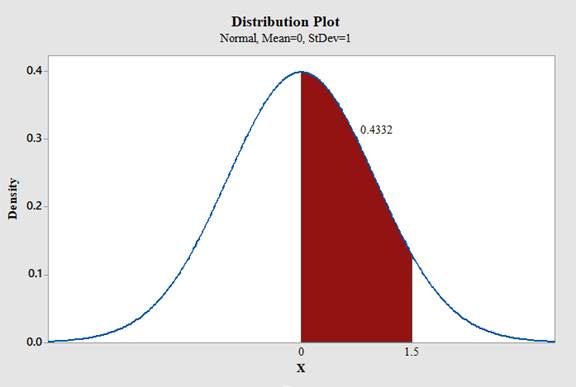
Therefore,
Conclusion:
The area under the standard normal distribution curve for
(b)
To find: The area under the standard normal distribution curve for
–1.25. That is,
Answer to Problem 18CQ
The area under the standard normal distribution curve for
Explanation of Solution
Calculation:
Software procedure:
Use Minitab; find the area under the normal curve between 0 and -1.25 with the help of following instructions:
- Choose Graph > Probability Distribution Plot choose View Probability> OK.
- From Distribution, choose ‘Normal’ distribution.
- Enter the Mean as 0.0 and Standard deviation as 1.0.
- Click the Shaded Area tab.
- Click the picture for Middle.
- Type in the smaller value -1.25 for X value1 and then the larger value 0 for the X value2.
- Click OK.
Output using the MINITAB software is given below:
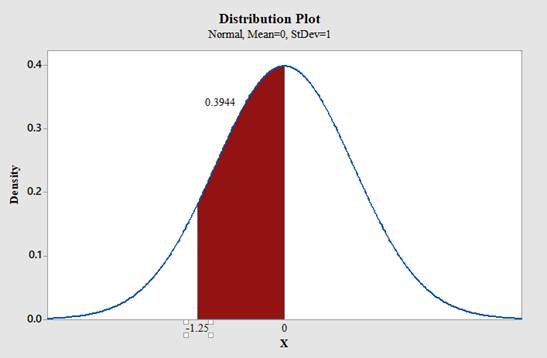
Therefore,
Conclusion:
The area under the standard normal distribution curve for
(c)
To find: The area under the standard normal distribution curve for
Answer to Problem 18CQ
The area under the standard normal distribution curve for
Explanation of Solution
Calculation:
Software procedure:
Use Minitab; find the area under the normal curve between 1.56 and 1.96 with the help of following instructions:
- Choose Graph > Probability Distribution Plot choose View Probability> OK.
- From Distribution, choose ‘Normal’ distribution.
- Enter the Mean as 0.0 and Standard deviation as 1.0.
- Click the Shaded Area tab.
- Click the picture for Middle.
- Type in the smaller value 1.56 for X value1 and then the larger value 1.96 for the X value2.
- Click OK.
Output using the MINITAB software is given below:
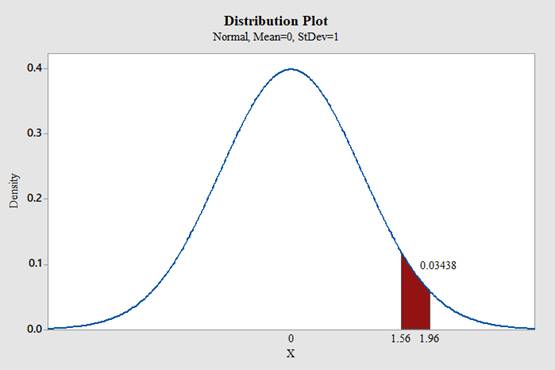 .
.
Therefore,
Conclusion:
The area under the standard normal distribution curve for
(d)
To find: The area under the standard normal distribution curve for
Answer to Problem 18CQ
The area under the standard normal distribution curve for
Explanation of Solution
Calculation:
Software procedure:
Use Minitab; find the area under the normal curve between -1.20 and -2.25 with the help of following instructions:
- Choose Graph > Probability Distribution Plot choose View Probability> OK.
- From Distribution, choose ‘Normal’ distribution.
- Enter the Mean as 0.0 and Standard deviation as 1.0.
- Click the Shaded Area tab.
- Click the picture for Middle.
- Type in the smaller value -2.25 for X value1 and then the larger value -1.20 for the X value2.
- Click OK.
Output using the MINITAB software is given below:
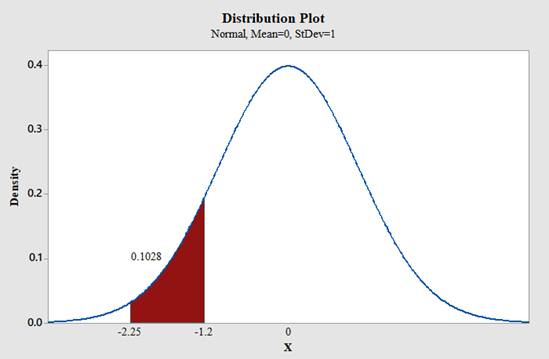
Therefore,
Conclusion:
The area under the standard normal distribution curve for
(e)
To find: The area under the standard normal distribution curve for
Answer to Problem 18CQ
The area under the standard normal distribution curve for
Explanation of Solution
Calculation:
Software procedure:
Use Minitab; find the area under the normal curve between -0.06 and 0.73 with the help of following instructions:
- Choose Graph > Probability Distribution Plot choose View Probability> OK.
- From Distribution, choose ‘Normal’ distribution.
- Enter the Mean as 0.0 and Standard deviation as 1.0.
- Click the Shaded Area tab.
- Click the picture for Middle.
- Type in the smaller value -0.06 for X value1 and then the larger value 0.73 for the X value2.
- Click OK.
Output using the MINITAB software is given below:
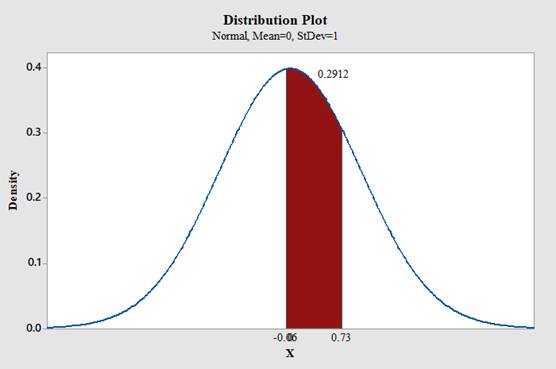 .
.
Therefore,
Conclusion:
The area under the standard normal distribution curve for
(f)
To find: The area under the standard normal distribution curve for
Answer to Problem 18CQ
The area under the standard normal distribution curve for
Explanation of Solution
Calculation:
Software procedure:
Use Minitab; find the area under the normal curve between 1.10 and -1.80 with the help of following instructions:
- Choose Graph > Probability Distribution Plot choose View Probability> OK.
- From Distribution, choose ‘Normal’ distribution.
- Enter the Mean as 0.0 and Standard deviation as 1.0.
- Click the Shaded Area tab.
- Click the picture for Middle.
- Type in the smaller value -1.80 for X value1 and then the larger value 1.10 for the X value2.
- Click OK.
Output using the MINITAB software is given below:
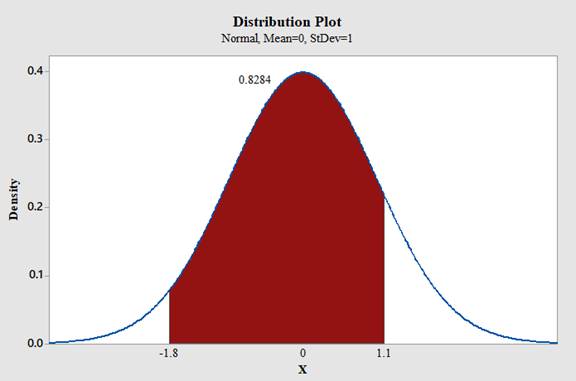
Therefore,
Conclusion:
The area under the standard normal distribution curve for
(g)
To find: The area under the standard normal curve to the right of
Answer to Problem 18CQ
The area under the standard normal curve to the right of
Explanation of Solution
Calculation:
Software procedure:
Use Minitab; find the area under the normal curve to the right of 1.75 with the help of following instructions:
- Choose Graph > Probability Distribution Plot choose View Probability> OK.
- From Distribution, choose ‘Normal’ distribution.
- Enter the Mean as 0.0 and Standard deviation as 1.0.
- Click the Shaded Area tab.
- Click the picture for Right Trail.
- Type in the Z value of 1.75 and click OK.
Output using the MINITAB software is given below:
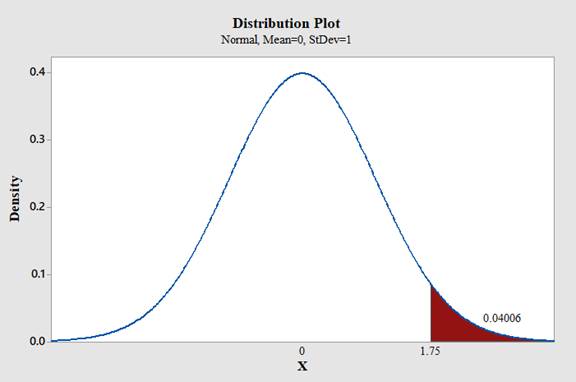
Therefore,
Conclusion:
The area under the standard normal curve to the right of
(h)
To find: The area under the standard normal curve to the right of
Answer to Problem 18CQ
The area under the standard normal curve to the right of
Explanation of Solution
Calculation:
Software procedure:
Use Minitab; find the area under the normal curve to the right of -1.28 with the help of following instructions:
- Choose Graph > Probability Distribution Plot choose View Probability> OK.
- From Distribution, choose ‘Normal’ distribution.
- Enter the Mean as 0.0 and Standard deviation as 1.0.
- Click the Shaded Area tab.
- Click the picture for Right Trail.
- Type in the Z value of -1.28 and click OK.
Output using the MINITAB software is given below:
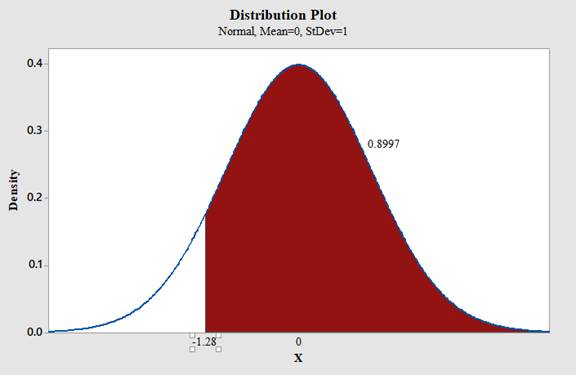
Therefore,
Conclusion:
The area under the standard normal curve to the right of
(i)
To find: The area under the standard normal curve to the left of
Answer to Problem 18CQ
The area under the standard normal curve to the left of
Explanation of Solution
Calculation:
Software procedure:
Use Minitab; find the area under the normal curve to the left of -2.12 with the help of following instructions:
- Choose Graph > Probability Distribution Plot choose View Probability> OK.
- From Distribution, choose ‘Normal’ distribution.
- Enter the Mean as 0.0 and Standard deviation as 1.0.
- Click the Shaded Area tab.
- Click the picture for Left Trail.
- Type in the Z value of -2.12 and click OK.
Output using the MINITAB software is given below:
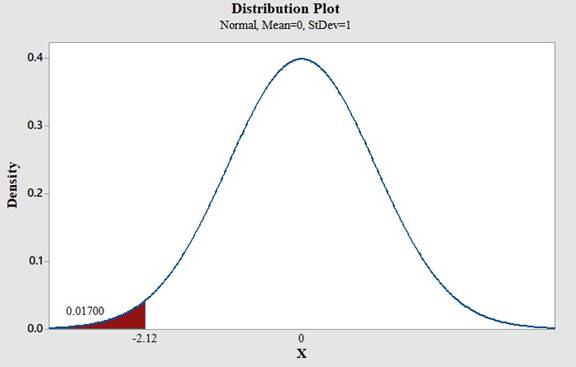
Therefore,
Conclusion:
The area under the standard normal curve to the left of
(j)
To find: The area under the standard normal curve to the left of
Answer to Problem 18CQ
The area under the standard normal curve to the left of
Explanation of Solution
Calculation:
Software procedure:
Use Minitab; find the area under the normal curve to the left of 1.36 with the help of following instructions:
- Choose Graph > Probability Distribution Plot choose View Probability> OK.
- From Distribution, choose ‘Normal’ distribution.
- Enter the Mean as 0.0 and Standard deviation as 1.0.
- Click the Shaded Area tab.
- Click the picture for Left Trail.
- Type in the Z value of 1.36 and click OK.
Output using the MINITAB software is given below:
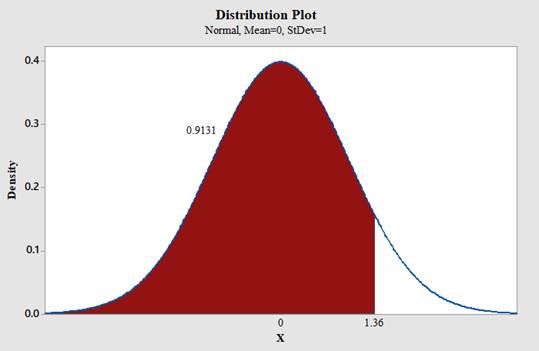
Therefore,
Conclusion:
The area under the standard normal curve to the left of
Want to see more full solutions like this?
Chapter 6 Solutions
Elementary Statistics: A Step By Step Approach
- A survey of 581 citizens found that 313 of them favor a new bill introduced by the city. We want to find a 95% confidence interval for the true proportion of the population who favor the bill. What is the lower limit of the interval? Enter the result as a decimal rounded to 3 decimal digits. Your Answer:arrow_forwardLet X be a continuous RV with PDF where a > 0 and 0 > 0 are parameters. verify that f-∞ /x (x)dx = 1. Find the CDF, Fx (7), of X.arrow_forward6. [20] Let X be a continuous RV with PDF 2(1), 1≤x≤2 fx(x) = 0, otherwisearrow_forward
- A survey of 581 citizens found that 313 of them favor a new bill introduced by the city. We want to find a 95% confidence interval for the true proportion of the population who favor the bill. What is the lower limit of the interval? Enter the result as a decimal rounded to 3 decimal digits. Your Answer:arrow_forwardA survey of 581 citizens found that 313 of them favor a new bill introduced by the city. We want to find a 95% confidence interval for the true proportion of the population who favor the bill. What is the lower limit of the interval? Enter the result as a decimal rounded to 3 decimal digits. Your Answer:arrow_forward2. The SMSA data consisting of 141 observations on 10 variables is fitted by the model below: 1 y = Bo+B1x4 + ẞ2x6 + ẞ3x8 + √1X4X8 + V2X6X8 + €. See Question 2, Tutorial 3 for the meaning of the variables in the above model. The following results are obtained: Estimate Std. Error t value Pr(>|t|) (Intercept) 1.302e+03 4.320e+02 3.015 0.00307 x4 x6 x8 x4:x8 x6:x8 -1.442e+02 2.056e+01 -7.013 1.02e-10 6.340e-01 6.099e+00 0.104 0.91737 -9.455e-02 5.802e-02 -1.630 0.10550 2.882e-02 2.589e-03 11.132 1.673e-03 7.215e-04 2.319 F) x4 1 3486722 3486722 17.9286 4.214e-05 x6 1 14595537 x8 x4:x8 x6:x8 1 132.4836 < 2.2e-16 1045693 194478 5.3769 0.02191 1 1198603043 1198603043 6163.1900 < 2.2e-16 1 25765100 25765100 1045693 Residuals 135 26254490 Estimated variance matrix (Intercept) x4 x6 x8 x4:x8 x6:x8 (Intercept) x4 x6 x8 x4:x8 x6:x8 0.18875694 1.866030e+05 -5.931735e+03 -2.322825e+03 -16.25142055 0.57188953 -5.931735e+03 4.228816e+02 3.160915e+01 0.61621781 -0.03608028 -0.00445013 -2.322825e+03…arrow_forward
- In some applications the distribution of a discrete RV, X resembles the Poisson distribution except that 0 is not a possible value of X. Consider such a RV with PMF where 1 > 0 is a parameter, and c is a constant. (a) Find the expression of c in terms of 1. (b) Find E(X). (Hint: You can use the fact that, if Y ~ Poisson(1), the E(Y) = 1.)arrow_forwardSuppose that X ~Bin(n,p). Show that E[(1 - p)] = (1-p²)".arrow_forwardI need help with this problem and an explanation of the solution for the image described below. (Statistics: Engineering Probabilities)arrow_forward
- I need help with this problem and an explanation of the solution for the image described below. (Statistics: Engineering Probabilities)arrow_forwardThis exercise is based on the following data on four bodybuilding supplements. (Figures shown correspond to a single serving.) Creatine(grams) L-Glutamine(grams) BCAAs(grams) Cost($) Xtend(SciVation) 0 2.5 7 1.00 Gainz(MP Hardcore) 2 3 6 1.10 Strongevity(Bill Phillips) 2.5 1 0 1.20 Muscle Physique(EAS) 2 2 0 1.00 Your personal trainer suggests that you supplement with at least 10 grams of creatine, 39 grams of L-glutamine, and 90 grams of BCAAs each week. You are thinking of combining Xtend and Gainz to provide you with the required nutrients. How many servings of each should you combine to obtain a week's supply that meets your trainer's specifications at the least cost? (If an answer does not exist, enter DNE.) servings of xtend servings of gainzarrow_forwardI need help with this problem and an explanation of the solution for the image described below. (Statistics: Engineering Probabilities)arrow_forward
 College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
