Elementary Linear Algebra
8th Edition
ISBN: 9780357156100
Author: Ron Larson
Publisher: Cengage Limited
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 5.CR, Problem 71CR
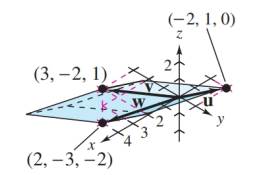
Finding the Volume of a Parallelepiped In Exercises 69-72, find the volume
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Solve for the matrix X:
X
(2 7³) x + ( 2 ) - (112)
6
14
8
5.
Solve for the matrix X. (Hint: we can solve AX
-1
= B whenever A is invertible)
2
3
0
Χ
2
=
3
1
Write p(x) = 6+11x+6x² as a linear combination of ƒ (x) = 2+x+4x² and g(x) = 1−x+3x²
and h(x)=3+2x+5x²
Chapter 5 Solutions
Elementary Linear Algebra
Ch. 5.1 - Finding the Length of a Vector. In Exercises 1-4,...Ch. 5.1 - Finding the Length of a Vector. In Exercises 1-4,...Ch. 5.1 - Finding the Length of a Vector. In Exercises 1-4,...Ch. 5.1 - Finding the Length of a Vector. In Exercises 1-4,...Ch. 5.1 - Finding the Length of a Vector. In Exercises 5-8,...Ch. 5.1 - Finding the Length of a Vector. In Exercises 58,...Ch. 5.1 - Exercises Finding the Length of a Vector In...Ch. 5.1 - Exercises Finding the Length of a Vector In...Ch. 5.1 - Exercises Finding a Unit Vector. In Exercises 912,...Ch. 5.1 - Exercises Finding a Unit Vector. In Exercises 912,...
Ch. 5.1 - Exercises Finding a Unit Vector. In Exercises 912,...Ch. 5.1 - Exercises Finding a Unit Vector. In Exercises 912,...Ch. 5.1 - Exercises Finding a Vector. In Exercises 1316,...Ch. 5.1 - Prob. 14ECh. 5.1 - Prob. 15ECh. 5.1 - Finding a VectorIn Exercises 13-16, find the...Ch. 5.1 - Consider the vector v=(1,3,0,4). Find u such that...Ch. 5.1 - For what values of c is c(1,2,3)=1?Ch. 5.1 - Finding the Distance Between Two VectorsIn...Ch. 5.1 - Finding the Distance Between Two VectorsIn...Ch. 5.1 - Finding the Distance Between Two VectorsIn...Ch. 5.1 - Finding the Distance Between Two VectorsIn...Ch. 5.1 - Prob. 23ECh. 5.1 - Prob. 24ECh. 5.1 - Prob. 25ECh. 5.1 - Prob. 26ECh. 5.1 - Find (u+v)(2uv) when uu=4, uv=5, and vv=10.Ch. 5.1 - Find (3uv)(u3v) when uu=8, uv=7, and vv=6.Ch. 5.1 - Finding Lengths, Unit Vectors, and Dot Products In...Ch. 5.1 - Finding Lengths, Unit Vectors, and Dot Products In...Ch. 5.1 - Finding Lengths, Unit Vectors, and Dot Products In...Ch. 5.1 - Prob. 32ECh. 5.1 - Finding Lengths, Unit Vectors, and Dot Products In...Ch. 5.1 - Prob. 34ECh. 5.1 - Verifying the Cauchy-Schwarz Inequality In...Ch. 5.1 - Verifying the Cauchy-Schwarz Inequality In...Ch. 5.1 - Prob. 37ECh. 5.1 - Prob. 38ECh. 5.1 - Finding the Angle Between Two Vectors In Exercises...Ch. 5.1 - Finding the Angle Between Two Vectors In Exercises...Ch. 5.1 - Prob. 41ECh. 5.1 - Prob. 42ECh. 5.1 - Finding the Angle Between Two Vectors In Exercises...Ch. 5.1 - Finding the Angle Between Two Vectors In Exercises...Ch. 5.1 - Finding the Angle Between Two Vectors In Exercises...Ch. 5.1 - Prob. 46ECh. 5.1 - Determining a Relationship Between Two Vectors In...Ch. 5.1 - Determining a Relationship Between Two Vectors In...Ch. 5.1 - Prob. 49ECh. 5.1 - Determining a Relationship Between Two Vectors In...Ch. 5.1 - Prob. 51ECh. 5.1 - Determining a Relationship Between Two Vectors In...Ch. 5.1 - Exercises Determining a relationship Between Two...Ch. 5.1 - Prob. 54ECh. 5.1 - Prob. 55ECh. 5.1 - Exercises Finding orthogonal Vectors In Exercises...Ch. 5.1 - Exercises Finding orthogonal Vectors In Exercises...Ch. 5.1 - Prob. 58ECh. 5.1 - Prob. 59ECh. 5.1 - Verifying the Triangle Inequality. In Exercises...Ch. 5.1 - Prob. 61ECh. 5.1 - Prob. 62ECh. 5.1 - Prob. 63ECh. 5.1 - Verifying the Pythagorean Theorem In Exercises...Ch. 5.1 - Prob. 65ECh. 5.1 - Prob. 66ECh. 5.1 - Rework Exercise 23 using matrix multiplication....Ch. 5.1 - Rework Exercise 24 using matrix multiplication....Ch. 5.1 - Prob. 69ECh. 5.1 - Prob. 70ECh. 5.1 - Writing In Exercises 71 and 72, determine whether...Ch. 5.1 - Prob. 72ECh. 5.1 - True or False?In Exercises 73 and 74, determine...Ch. 5.1 - Prob. 74ECh. 5.1 - Prob. 75ECh. 5.1 - Prob. 76ECh. 5.1 - Orthogonal Vectors In Exercises 77 and 78, let...Ch. 5.1 - Orthogonal Vectors In Exercises 77 and 78, let...Ch. 5.1 - Prob. 79ECh. 5.1 - Prob. 80ECh. 5.1 - Prob. 81ECh. 5.1 - Prob. 82ECh. 5.1 - Guided Proof Prove that if u is orthogonal to v...Ch. 5.1 - Prob. 84ECh. 5.1 - Prob. 85ECh. 5.1 - Proof Prove that u+v=u+v if and only if u and v...Ch. 5.1 - Proof Use the properties of matrix multiplication...Ch. 5.1 - Prob. 88ECh. 5.1 - Writing Let x be a solution to mn homogeneous...Ch. 5.2 - Showing That a Function Is an Inner Product In...Ch. 5.2 - Showing That a Function Is an Inner Product In...Ch. 5.2 - Showing That a Function Is an Inner Product In...Ch. 5.2 - Showing That a Function Is an Inner Product In...Ch. 5.2 - Showing That a Function Is an Inner Product In...Ch. 5.2 - Showing That a Function Is an Inner Product In...Ch. 5.2 - Showing That a Function Is an Inner Product In...Ch. 5.2 - Showing That a Function Is an Inner ProductIn...Ch. 5.2 - Showing That a Function Is Not an Inner Product In...Ch. 5.2 - Showing That a Function Is Not an Inner Product In...Ch. 5.2 - Showing That a Function Is Not an Inner Product In...Ch. 5.2 - Showing That a Function Is Not an Inner Product In...Ch. 5.2 - Showing That a Function Is Not an Inner Product In...Ch. 5.2 - Showing That a Function Is Not an Inner Product In...Ch. 5.2 - Showing That a Function Is Not an Inner Product In...Ch. 5.2 - Prob. 16ECh. 5.2 - Finding Inner Product, Length, and DistanceIn...Ch. 5.2 - Prob. 18ECh. 5.2 - Finding Inner Product, Length, and DistanceIn...Ch. 5.2 - Finding Inner Product, Length, and DistanceIn...Ch. 5.2 - Finding Inner Product, Length, and DistanceIn...Ch. 5.2 - Prob. 22ECh. 5.2 - Finding Inner Product, Length, and DistanceIn...Ch. 5.2 - Finding Inner Product, Length, and DistanceIn...Ch. 5.2 - Finding Inner Product, Length, and DistanceIn...Ch. 5.2 - Prob. 26ECh. 5.2 - Showing That a Function Is an Inner ProductIn...Ch. 5.2 - Showing That a Function Is an Inner ProductIn...Ch. 5.2 - Finding Inner Product, Length, and DistanceIn...Ch. 5.2 - Prob. 30ECh. 5.2 - Finding Inner Product, Length, and DistanceIn...Ch. 5.2 - Finding Inner Product, Length, and Distance In...Ch. 5.2 - Showing That a Function Is an Inner Product In...Ch. 5.2 - Prob. 34ECh. 5.2 - Finding Inner Product, Length, and Distance In...Ch. 5.2 - Finding Inner Product, Length, and Distance In...Ch. 5.2 - Finding Inner Product, Length, and Distance In...Ch. 5.2 - Prob. 38ECh. 5.2 - Calculus In Exercises 39-42, use the functions f...Ch. 5.2 - Prob. 40ECh. 5.2 - Calculus In Exercises 39-42, use the functions f...Ch. 5.2 - Prob. 42ECh. 5.2 - Finding the Angle Between Two Vectors In Exercises...Ch. 5.2 - Finding the Angle Between Two Vectors In Exercises...Ch. 5.2 - Finding the Angle Between Two Vectors In Exercises...Ch. 5.2 - Finding the Angle Between Two Vectors In Exercises...Ch. 5.2 - Finding the Angle Between Two Vectors In Exercises...Ch. 5.2 - Prob. 48ECh. 5.2 - Finding the Angle Between Two Vectors In Exercises...Ch. 5.2 - Finding the Angle Between Two Vectors In Exercises...Ch. 5.2 - Prob. 51ECh. 5.2 - Prob. 52ECh. 5.2 - Prob. 53ECh. 5.2 - Verifying Inequalities In Exercises 53-64, verify...Ch. 5.2 - Prob. 55ECh. 5.2 - Prob. 56ECh. 5.2 - Prob. 57ECh. 5.2 - Prob. 58ECh. 5.2 - Prob. 59ECh. 5.2 - Verifying Inequalities In Exercises 53-64, verify...Ch. 5.2 - Verifying InequalitiesIn Exercises 53-64, verify a...Ch. 5.2 - Prob. 62ECh. 5.2 - Prob. 63ECh. 5.2 - Prob. 64ECh. 5.2 - Calculus In Exercises 65-68, show that f and g are...Ch. 5.2 - Prob. 66ECh. 5.2 - Calculus In Exercises 65-68, show that f and g are...Ch. 5.2 - Prob. 68ECh. 5.2 - Prob. 69ECh. 5.2 - Finding and Graphing Orthogonal Projections in R2...Ch. 5.2 - Prob. 71ECh. 5.2 - Prob. 72ECh. 5.2 - Prob. 73ECh. 5.2 - Finding Orthogonal Projections In Exercises 7376,...Ch. 5.2 - Finding Orthogonal Projections In Exercises 7376,...Ch. 5.2 - Prob. 76ECh. 5.2 - Prob. 77ECh. 5.2 - Calculus In Exercises 77-84, find the orthogonal...Ch. 5.2 - Calculus In Exercises 77-84, find the orthogonal...Ch. 5.2 - Prob. 80ECh. 5.2 - Prob. 81ECh. 5.2 - Prob. 82ECh. 5.2 - Prob. 83ECh. 5.2 - Prob. 84ECh. 5.2 - True or false?In Exercises 85 and 86, determine...Ch. 5.2 - Prob. 86ECh. 5.2 - Prob. 87ECh. 5.2 - Prob. 88ECh. 5.2 - Prob. 89ECh. 5.2 - Proof Let u and v be a nonzero vectors in an inner...Ch. 5.2 - Prob. 91ECh. 5.2 - Prob. 92ECh. 5.2 - Prob. 93ECh. 5.2 - Prob. 94ECh. 5.2 - Guided proofLet u,v be the Euclidean inner product...Ch. 5.2 - CAPSTONE (a) Explain how to determine whether a...Ch. 5.2 - Prob. 97ECh. 5.2 - Prob. 98ECh. 5.2 - Prob. 99ECh. 5.2 - Prob. 100ECh. 5.2 - Consider the vectors u=(6,2,4) and v=(1,2,0) from...Ch. 5.3 - Orthogonal and Orthonormal SetsIn Exercises 1-12,...Ch. 5.3 - Orthogonal and Orthonormal Sets In Exercises 1-12,...Ch. 5.3 - Prob. 3ECh. 5.3 - Orthogonal and Orthonormal SetsIn Exercises 1-12,...Ch. 5.3 - Orthogonal and Orthonormal Sets In Exercises 1-12,...Ch. 5.3 - Prob. 6ECh. 5.3 - Orthogonal and Orthonormal SetsIn Exercises 1-12,...Ch. 5.3 - Orthogonal and Orthonormal SetsIn Exercises 1-12,...Ch. 5.3 - Orthogonal and Orthonormal SetsIn Exercises 1-12,...Ch. 5.3 - Prob. 10ECh. 5.3 - Orthogonal and Orthonormal SetsIn Exercises 1-12,...Ch. 5.3 - Prob. 12ECh. 5.3 - Normalizing an Orthogonal Set In Exercises 13-16,...Ch. 5.3 - Prob. 14ECh. 5.3 - Normalizing an Orthogonal Set In Exercises 13-16,...Ch. 5.3 - Prob. 16ECh. 5.3 - Complete Example 2 by verifying that {1,x,x2,x3}...Ch. 5.3 - Prob. 18ECh. 5.3 - Finding a Coordinate Matrix In Exercises 19-24,...Ch. 5.3 - Prob. 20ECh. 5.3 - Finding a Coordinate Matrix In Exercises 19-24,...Ch. 5.3 - Finding a Coordinate Matrix In Exercises 19-24,...Ch. 5.3 - Prob. 23ECh. 5.3 - Finding a Coordinate Matrix In Exercises 19-24,...Ch. 5.3 - Applying the Gram-Schmidt Process In Exercises...Ch. 5.3 - Prob. 26ECh. 5.3 - Prob. 27ECh. 5.3 - Prob. 28ECh. 5.3 - Prob. 29ECh. 5.3 - Prob. 30ECh. 5.3 - Prob. 31ECh. 5.3 - Prob. 32ECh. 5.3 - Prob. 33ECh. 5.3 - Prob. 34ECh. 5.3 - Prob. 35ECh. 5.3 - Prob. 36ECh. 5.3 - Prob. 37ECh. 5.3 - Prob. 38ECh. 5.3 - Applying the Gram-Schmidt Process In Exercises...Ch. 5.3 - Prob. 40ECh. 5.3 - Use the inner product u,v=2u1v1+u2v2 in R2 and...Ch. 5.3 - WritingExplain why the result of Exercise 41 is...Ch. 5.3 - Calculus In Exercises 43-48, let B={1,x,x2} be a...Ch. 5.3 - Prob. 44ECh. 5.3 - Prob. 45ECh. 5.3 - Prob. 46ECh. 5.3 - Prob. 47ECh. 5.3 - Calculus In Exercises 43-48, let B={1,x,x2} be a...Ch. 5.3 - Prob. 49ECh. 5.3 - Prob. 50ECh. 5.3 - Applying the Alternative Form of the Gram-Schmidt...Ch. 5.3 - Prob. 52ECh. 5.3 - Applying the Alternative Form of the Gram-Schmidt...Ch. 5.3 - Prob. 54ECh. 5.3 - Prob. 55ECh. 5.3 - True or False? In Exercises 55 and 56, determine...Ch. 5.3 - Orthonormal Sets in P2In Exercises 57-62, let...Ch. 5.3 - Prob. 58ECh. 5.3 - Prob. 59ECh. 5.3 - Prob. 60ECh. 5.3 - Orthonormal Sets in P2In Exercises 57-62, let...Ch. 5.3 - Orthonormal Sets in P2In Exercises 57-62, let...Ch. 5.3 - Prob. 63ECh. 5.3 - Guided Proof Prove that if w is orthogonal to each...Ch. 5.3 - Prob. 65ECh. 5.3 - Prob. 66ECh. 5.3 - Prob. 67ECh. 5.3 - Prob. 68ECh. 5.3 - Prob. 69ECh. 5.3 - Prob. 70ECh. 5.3 - Prob. 71ECh. 5.4 - Least Squares Regression LineIn Exercises 1-4,...Ch. 5.4 - Prob. 2ECh. 5.4 - Prob. 3ECh. 5.4 - Prob. 4ECh. 5.4 - Prob. 5ECh. 5.4 - Prob. 6ECh. 5.4 - Prob. 7ECh. 5.4 - Prob. 8ECh. 5.4 - Prob. 9ECh. 5.4 - Prob. 10ECh. 5.4 - Prob. 11ECh. 5.4 - Prob. 12ECh. 5.4 - Prob. 13ECh. 5.4 - Prob. 14ECh. 5.4 - Prob. 15ECh. 5.4 - Prob. 16ECh. 5.4 - Prob. 17ECh. 5.4 - Prob. 18ECh. 5.4 - Prob. 19ECh. 5.4 - Projection Onto a Subspace In Exercises 17-20,...Ch. 5.4 - Fundamental Subspaces In Exercises 21-24, find...Ch. 5.4 - Prob. 22ECh. 5.4 - Prob. 23ECh. 5.4 - Prob. 24ECh. 5.4 - Prob. 25ECh. 5.4 - Prob. 26ECh. 5.4 - Finding the Least Squares Solutions In Exercises...Ch. 5.4 - Finding the Least Squares Solution In Exercises...Ch. 5.4 - Prob. 29ECh. 5.4 - Prob. 30ECh. 5.4 - Prob. 31ECh. 5.4 - Prob. 32ECh. 5.4 - Prob. 33ECh. 5.4 - Prob. 34ECh. 5.4 - Prob. 35ECh. 5.4 - Prob. 36ECh. 5.4 - Prob. 37ECh. 5.4 - Prob. 38ECh. 5.4 - Prob. 39ECh. 5.4 - Prob. 40ECh. 5.4 - Prob. 41ECh. 5.4 - Prob. 42ECh. 5.4 - True or false? In Exercises 43and 44, determine...Ch. 5.4 - True or false? In Exercises 43 and 44, determine...Ch. 5.4 - Proof Prove that if S1 and S2 are orthogonal...Ch. 5.4 - Prob. 46ECh. 5.4 - Prob. 47ECh. 5.4 - Prob. 48ECh. 5.5 - Finding the Cross Product In Exercises 1-6, find...Ch. 5.5 - Prob. 2ECh. 5.5 - Prob. 3ECh. 5.5 - Prob. 4ECh. 5.5 - Prob. 5ECh. 5.5 - Prob. 6ECh. 5.5 - Prob. 7ECh. 5.5 - Prob. 8ECh. 5.5 - Prob. 9ECh. 5.5 - Prob. 10ECh. 5.5 - Prob. 11ECh. 5.5 - Prob. 12ECh. 5.5 - Prob. 13ECh. 5.5 - Prob. 14ECh. 5.5 - Prob. 15ECh. 5.5 - Prob. 16ECh. 5.5 - Prob. 17ECh. 5.5 - Prob. 18ECh. 5.5 - Prob. 19ECh. 5.5 - Prob. 20ECh. 5.5 - Prob. 21ECh. 5.5 - Prob. 22ECh. 5.5 - Prob. 23ECh. 5.5 - Prob. 24ECh. 5.5 - Prob. 25ECh. 5.5 - Prob. 26ECh. 5.5 - Prob. 27ECh. 5.5 - Prob. 28ECh. 5.5 - Prob. 29ECh. 5.5 - Prob. 30ECh. 5.5 - Prob. 31ECh. 5.5 - Prob. 32ECh. 5.5 - Prob. 33ECh. 5.5 - Prob. 34ECh. 5.5 - Prob. 35ECh. 5.5 - Prob. 36ECh. 5.5 - Prob. 37ECh. 5.5 - Prob. 38ECh. 5.5 - Prob. 39ECh. 5.5 - Prob. 40ECh. 5.5 - Prob. 41ECh. 5.5 - Prob. 42ECh. 5.5 - Prob. 43ECh. 5.5 - Prob. 44ECh. 5.5 - Prob. 45ECh. 5.5 - Finding the Area of a Parallelogram In Exercises...Ch. 5.5 - Prob. 47ECh. 5.5 - Prob. 48ECh. 5.5 - Finding the Area of a Triangle In Exercises 49 and...Ch. 5.5 - Prob. 50ECh. 5.5 - Prob. 51ECh. 5.5 - Prob. 52ECh. 5.5 - Prob. 53ECh. 5.5 - Prob. 54ECh. 5.5 - Prob. 55ECh. 5.5 - Prob. 56ECh. 5.5 - Prob. 57ECh. 5.5 - Prob. 58ECh. 5.5 - Prob. 59ECh. 5.5 - Prob. 60ECh. 5.5 - Prob. 61ECh. 5.5 - Prob. 62ECh. 5.5 - Prob. 63ECh. 5.5 - Prob. 64ECh. 5.5 - Prob. 65ECh. 5.5 - Prob. 66ECh. 5.5 - Prob. 67ECh. 5.5 - Prob. 68ECh. 5.5 - Prob. 69ECh. 5.5 - Prob. 70ECh. 5.5 - Prob. 71ECh. 5.5 - Prob. 72ECh. 5.5 - Prob. 73ECh. 5.5 - Prob. 74ECh. 5.5 - Finding a Least Squares Approximation In Exercises...Ch. 5.5 - Prob. 76ECh. 5.5 - Prob. 77ECh. 5.5 - Prob. 78ECh. 5.5 - Prob. 79ECh. 5.5 - Prob. 80ECh. 5.5 - Prob. 81ECh. 5.5 - Prob. 82ECh. 5.5 - Prob. 83ECh. 5.5 - Prob. 84ECh. 5.5 - Prob. 85ECh. 5.5 - Prob. 86ECh. 5.5 - Prob. 87ECh. 5.5 - Prob. 88ECh. 5.5 - Prob. 89ECh. 5.5 - Prob. 90ECh. 5.5 - Prob. 91ECh. 5.5 - Prob. 92ECh. 5.5 - Use your schools library, the Internet, or some...Ch. 5.CR - Finding Lengths, Dot Product, and Distance In...Ch. 5.CR - Finding Lengths, Dot Product, and Distance In...Ch. 5.CR - Prob. 3CRCh. 5.CR - Prob. 4CRCh. 5.CR - Finding Lengths, Dot Product, and Distance In...Ch. 5.CR - Finding Lengths, Dot Product, and Distance In...Ch. 5.CR - Finding Lengths, Dot Product, and Distance In...Ch. 5.CR - Finding Lengths, Dot Product, and Distance In...Ch. 5.CR - Prob. 9CRCh. 5.CR - Prob. 10CRCh. 5.CR - Prob. 11CRCh. 5.CR - Prob. 12CRCh. 5.CR - Prob. 13CRCh. 5.CR - Prob. 14CRCh. 5.CR - Prob. 15CRCh. 5.CR - Prob. 16CRCh. 5.CR - Prob. 17CRCh. 5.CR - Prob. 18CRCh. 5.CR - Finding the Angle Between Two VectorsIn Exercises...Ch. 5.CR - Finding the Angle Between Two Vectors In Exercises...Ch. 5.CR - Prob. 21CRCh. 5.CR - Prob. 22CRCh. 5.CR - Prob. 23CRCh. 5.CR - Prob. 24CRCh. 5.CR - For u=(4,32,1) and v=(12,3,1), a find the inner...Ch. 5.CR - For u=(0,3,13) and v=(43,1,3), a find the inner...Ch. 5.CR - Verify the triangle inequality and the...Ch. 5.CR - Prob. 28CRCh. 5.CR - CalculusIn Exercises 29 and 30, a find the inner...Ch. 5.CR - CalculusIn Exercises 29 and 30, a find the inner...Ch. 5.CR - Prob. 31CRCh. 5.CR - Prob. 32CRCh. 5.CR - Finding an Orthogonal ProjectionIn Exercises...Ch. 5.CR - Finding an Orthogonal ProjectionIn Exercises...Ch. 5.CR - Finding an Orthogonal ProjectionIn Exercises...Ch. 5.CR - Finding an Orthogonal ProjectionIn Exercises...Ch. 5.CR - Applying the Gram-Schmidt ProcessIn Exercises...Ch. 5.CR - Prob. 38CRCh. 5.CR - Prob. 39CRCh. 5.CR - Prob. 40CRCh. 5.CR - Let B={(0,2,2),(1,0,2)} be a basis for a subspace...Ch. 5.CR - Repeat Exercise 41 for B={(1,2,2),(1,0,0)} and...Ch. 5.CR - Prob. 43CRCh. 5.CR - Prob. 44CRCh. 5.CR - Calculus In Exercises 43-46, let f and g be...Ch. 5.CR - Calculus In Exercises 43-46, let f and g be...Ch. 5.CR - Find an orthonormal basis for the subspace of...Ch. 5.CR - Find an orthonormal basis for the solution space...Ch. 5.CR - Prob. 49CRCh. 5.CR - Prob. 50CRCh. 5.CR - Prob. 51CRCh. 5.CR - Prob. 52CRCh. 5.CR - Prob. 53CRCh. 5.CR - Let V be an two dimensional subspace of R4 spanned...Ch. 5.CR - Prob. 55CRCh. 5.CR - Prob. 56CRCh. 5.CR - Prob. 57CRCh. 5.CR - Prob. 58CRCh. 5.CR - Prob. 59CRCh. 5.CR - Find the projection of the vector v=[102]T onto...Ch. 5.CR - Find the bases for the four fundamental subspaces...Ch. 5.CR - Prob. 62CRCh. 5.CR - Prob. 63CRCh. 5.CR - Prob. 64CRCh. 5.CR - Finding the Cross Product In Exercises 65-68, find...Ch. 5.CR - Finding the Cross Product In Exercises 65-68, find...Ch. 5.CR - Prob. 67CRCh. 5.CR - Finding the Cross Product In Exercises 65-68, find...Ch. 5.CR - Prob. 69CRCh. 5.CR - Prob. 70CRCh. 5.CR - Finding the Volume of a ParallelepipedIn Exercises...Ch. 5.CR - Prob. 72CRCh. 5.CR - Prob. 73CRCh. 5.CR - Prob. 74CRCh. 5.CR - Finding a Least Approximation In Exercises 75-78,...Ch. 5.CR - Finding a Least Approximation In Exercises 75-78,...Ch. 5.CR - Prob. 77CRCh. 5.CR - Finding a Least Approximation In Exercises 75-78,...Ch. 5.CR - Finding a Least Squares Approximation In Exercises...Ch. 5.CR - Finding a Least Squares Approximation In Exercises...Ch. 5.CR - Prob. 81CRCh. 5.CR - Prob. 82CRCh. 5.CR - Prob. 83CRCh. 5.CR - Prob. 84CRCh. 5.CM - Prob. 1CMCh. 5.CM - Take this test to review the material in Chapters...Ch. 5.CM - Take this test to review the material in Chapters...Ch. 5.CM - Use a software program or a graphing utility to...Ch. 5.CM - Take this test to review the material in Chapters...Ch. 5.CM - Prob. 6CMCh. 5.CM - Prob. 7CMCh. 5.CM - Take this test to review the material in Chapters...Ch. 5.CM - Take this test to review the material in Chapters...Ch. 5.CM - Prob. 10CMCh. 5.CM - Prob. 11CMCh. 5.CM - Prob. 12CMCh. 5.CM - Prob. 13CMCh. 5.CM - Prob. 14CMCh. 5.CM - Prob. 15CMCh. 5.CM - Prob. 16CMCh. 5.CM - Prob. 17CMCh. 5.CM - Prob. 18CMCh. 5.CM - Prob. 19CMCh. 5.CM - Prob. 20CMCh. 5.CM - Prob. 21CMCh. 5.CM - The two matrices A and B are row-equivalent....Ch. 5.CM - Prob. 23CMCh. 5.CM - Prob. 24CM
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, algebra and related others by exploring similar questions and additional content below.Similar questions
- 3. Let M = (a) - (b) 2 −1 1 -1 2 7 4 -22 Find a basis for Col(M). Find a basis for Null(M).arrow_forwardSchoology X 1. IXL-Write a system of X Project Check #5 | Schx Thomas Edison essay, x Untitled presentation ixl.com/math/algebra-1/write-a-system-of-equations-given-a-graph d.net bookmarks Play Gimkit! - Enter... Imported Imported (1) Thomas Edison Inv... ◄›) What system of equations does the graph show? -8 -6 -4 -2 y 8 LO 6 4 2 -2 -4 -6 -8. 2 4 6 8 Write the equations in slope-intercept form. Simplify any fractions. y = y = = 00 S olo 20arrow_forwardEXERCICE 2: 6.5 points Le plan complexe est rapporté à un repère orthonormé (O, u, v ).Soit [0,[. 1/a. Résoudre dans l'équation (E₁): z2-2z+2 = 0. Ecrire les solutions sous forme exponentielle. I b. En déduire les solutions de l'équation (E2): z6-2 z³ + 2 = 0. 1-2 2/ Résoudre dans C l'équation (E): z² - 2z+1+e2i0 = 0. Ecrire les solutions sous forme exponentielle. 3/ On considère les points A, B et C d'affixes respectives: ZA = 1 + ie 10, zB = 1-ie 10 et zc = 2. a. Déterminer l'ensemble EA décrit par le point A lorsque e varie sur [0, 1. b. Calculer l'affixe du milieu K du segment [AB]. C. Déduire l'ensemble EB décrit par le point B lorsque varie sur [0,¹ [. d. Montrer que OACB est un parallelogramme. e. Donner une mesure de l'angle orienté (OA, OB) puis déterminer pour que OACB soit un carré.arrow_forward
- 2 Use grouping to factor: 10x + 13x + 3 = 0 Identify A B and C in the chart below feach responce inarrow_forward2 Use grouping to factor: 10x² + 13x + 3 = 0 Identify A, B, and C in the chart below. (each rearrow_forward2 Use grouping to factor: 10x + 13x + 3 = 0 Identify A B and C in the chart below feach responce inarrow_forward
- Use grouping to fully factor: x³ + 3x² - 16x - 48 = 0 3 2arrow_forwardName: Tay Jones Level Two Date: Algebra 3 Unit 3: Functions and Equations Practice Assessment Class: #7-OneNote 1. The function f(x) = x² is transformed in the following functions. List the vertex for each function, circle whether the function opens up or down, and why. All three parts must be correct to receive Level 2 points. You can receive points for a, b, and c. a) g(x) = -2(x+5)² Vertex: Opens Up Opens Down Why? ais negative -2 Vertex: b) g(x) = (x + 2)² - 3 c) g(x) = -4(x + 2)² + 2 Opens Up Opens Down Vertex: Opens Up Opens Down Why? 4 Ca is negative) Why? his positive 2. The graph of the function f(x) is shown below. Find the domain, range, and end behavior. Then list the values of x for which the function values are increasing and decreasing. f(x) Domain: End Behavior: As x → ∞o, f(x) -> -6 As x, f(x) -> Range: Where is it Increasing? (002] Where is it Decreasing? (1,00)arrow_forwardShow what to do on the graph visually please!arrow_forward
- The county's new asphalt paving machine can surface 1 km of highway in 10 h. A much older machine can surface 1 km in 18 h. How long will it take them to surface 21 km of highway if they start at opposite ends and work day and night?arrow_forward3. Write a system of linear equations in slope intercept form that has exactly one solution at the point (3, 4), such that one line has positive slope (but not 1) and the other line has negative slope (but not "1). Also write your system of equations with both equations written in standard form with out any fractions 8- 7 8 5 4 3 -2- + -8-7-6-5-4-3-2-1 1 2 3 -1 2 - ° 4 -5 - -8arrow_forward2. Write a system of linear equations in slope-intercept form has exactly one solution at the point (3, 4), such that both lines have negative slope (but neither one has slope of 1). Also write your system of equations with both equations written in standard form without any fractions. B 0 5 4 3 -2 1 -8-7-6-5-4-3-2 -1 12 3 -1 2 -3 -5 6 -7 -8arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Elementary Linear Algebra (MindTap Course List)AlgebraISBN:9781305658004Author:Ron LarsonPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Elementary Linear Algebra (MindTap Course List)
Algebra
ISBN:9781305658004
Author:Ron Larson
Publisher:Cengage Learning
Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Algebra and Trigonometry (MindTap Course List)
Algebra
ISBN:9781305071742
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning
Inner Product Spaces; Author: Jeff Suzuki: The Random Professor;https://www.youtube.com/watch?v=JzCZUx9ZTe8;License: Standard YouTube License, CC-BY