
Concept explainers
Imagine a situation in which eight people, numbered consecutively 1—8, are arranged in a circle. Starting from person #1, every second person in the circle is eliminated. The elimination process continues until only one person remains. In the first round the people numbered 2, 4, 6, and 3 are eliminated, in the second round the people numbered 3 and 7 are eliminated, and in the third round person #5 is eliminated, so after the third round only person #1 remains, as shown on the next page.
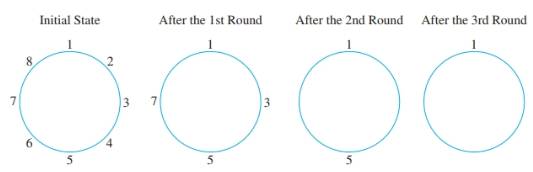
a. Given a set of sixteen people arranged in a circle and numbered, consecutively 1—16, list the numbers of the people who are eliminated in each round if every second person is eliminated and the elimination process continues until only one person remains. Assume that the starting point is person #1.
b. Use ordinary mathematical induction to prove that for every integer
c. Use the result of part (b) to prove that for any nonnegative integers n and m with
Trending nowThis is a popular solution!

Chapter 5 Solutions
DISCRETE MATHEMATICS WITH APPLICATION (
- या it 11 if the mechanism is given, then using Newton's posterior formula for the derivative Lind P(0.9) × 0 0.2 0.4 0.6 0.8 1 f 0 0.12 0.48 1.1 2 3.2arrow_forwardConsider an MA(6) model with θ1 = 0.5, θ2 = −25, θ3 = 0.125, θ4 = −0.0625, θ5 = 0.03125, and θ6 = −0.015625. Find a much simpler model that has nearly the same ψ-weights.arrow_forwardLet {Yt} be an AR(2) process of the special form Yt = φ2Yt − 2 + et. Use first principles to find the range of values of φ2 for which the process is stationary.arrow_forward
- Describe the important characteristics of the autocorrelation function for the following models: (a) MA(1), (b) MA(2), (c) AR(1), (d) AR(2), and (e) ARMA(1,1).arrow_forwarda) prove that if (x) is increasing then (x~) is bounded below and prove if (is decrasing then (xn) is bounded above- 6) If Xn is bounded and monotone then (Xa) is Convergent. In particular. i) if (xn) is bounded above and incrasing then lim xn = sups xn: ne№3 n700 ii) if (X) is bounded below and decrasing then I'm Xn = inf\x₂,neN} 4500 143arrow_forward5. Consider the following vectors 0.1 3.2 -0-0-0 = 5.4 6.0 = z= 3 0.1 For each of exercises a-e, either compute the desired quantity by hand with work shown or explain why the desired quantity is not defined. (a) 10x (b) 10-27 (c) J+Z (d) (x, y) (e) (x, z)arrow_forward
- 1) let X: N R be a sequence and let Y: N+R be the squence obtained from x by di scarding the first meN terms of x in other words Y(n) = x(m+h) then X converges to L If and only is y converges to L- 11) let Xn = cos(n) where nyo prove D2-1 that lim xn = 0 by def. h→00 ii) prove that for any irrational numbers ther exsist asquence of rational numbers (xn) converg to S.arrow_forwardConsider the graph/network plotted below. 1 6 5 3 Explicitly give (i.e., write down all of the entries) the adjacency matrix A of the graph.arrow_forward. Given the function f: XY (with X and Y as above) defined as f(2) = 2, f(4) = 1, ƒ(6)=3, ƒ(8) = 2, answer the following questions. Justify your answers. (a) [4 points] Is f injective? (b) [4 points] Is f surjective? (c) [2 points] Is f bijective?arrow_forward
- 1. Let 15 -14 A = -10 9 13-12 -8 7 11 15 -14 13 -12 -6 and B = -10 9 -8 7 -6 5 -4 3 -2 E 5 -4 3 -2 1 Explicitly give the values of A2,3, A1,5, and B1,4- Is A a 5 x 3 matrix? Explain your answer. Are A and B (mathematically) equal? Explain your answer.arrow_forwardGiven the following set X = {2, 4, 6, 8} and Y = {1, 2, 3}, explicitly give (e.g., write down the sets with numerical entries) of the outputs of the following requested set operations: (a) [2 points] XUY (Union) (b) [2 points] XY (Intersection) (c) [3 points] X\Y (Difference) (d) [3 points] XAY (Symmetric Difference)arrow_forward4.2 Product and Quotient Rules 1. 9(x)=125+1 y14+2 Use the product and/or quotient rule to find the derivative of each function. a. g(x)= b. y (2x-3)(x-1) c. y== 3x-4 √xarrow_forward
 College AlgebraAlgebraISBN:9781305115545Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
College AlgebraAlgebraISBN:9781305115545Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
 Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL





