HEART OF MATHEMATICS
4th Edition
ISBN: 9781119760061
Author: Burger
Publisher: WILEY
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 5.3, Problem 40MS
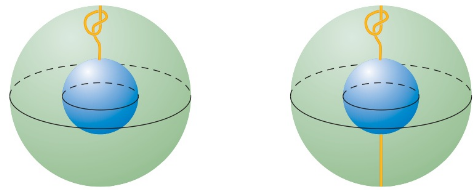
Fixed spheres again. We are given two spheres that are made of glass and thus are not distortable. They are rigidly fixed in space, one inside the other as illustrated, and they cannot be moved! The middle sphere floats miraculously in midair without any means of support. There is a rubber rope that hangs from the inside ceiling of the big sphere to the roof of the smaller sphere, as shown. The rope has a knot in it. Show that it is possible to unknot the rope without moving or distorting the two spheres. (This quest ion appeared in Section 5.1, Mindscape 38.) Suppose now we add another straight rope as shown. Can you unknot the top rope without introducing a knot in the bottom rope?
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
y =
log
5
– x2
-
4
00
For what value of A and B the function f(x) will be continuous everywhere for the given definition?..
Please fill in the rest of the steps of the proof of Thm 2.5. Show how "Repeating this step with n-1,n-2,...,2 in place of n" gives us the desired result.
Chapter 5 Solutions
HEART OF MATHEMATICS
Ch. 5.1 - Describing distortion. What does it mean to say...Ch. 5.1 - Your last sheet. Youre in your bathroom reading...Ch. 5.1 - Rubber polygons. Find a large rubber band and...Ch. 5.1 - Out, out red spot. Remove the red spot from the...Ch. 5.1 - That theta (S). Does there exist a pair of points...Ch. 5.1 - Your ABCs (H). Consider the following letters made...Ch. 5.1 - Half dollar and a straw. Suppose we drill a hole...Ch. 5.1 - Drop them. Is it possible to take off your...Ch. 5.1 - Coffee and doughnuts (H). Is a standard coffee mug...Ch. 5.1 - Lasting ties. Tie a thin rope around a friends...
Ch. 5.1 - Will you spill? (S). Suppose you rest a glass of...Ch. 5.1 - Grabbing the brass ring. Suppose a string attached...Ch. 5.1 - Hair care. Is a regular comb equivalent by...Ch. 5.1 - Three two-folds. Take three pieces of paper and...Ch. 5.1 - Equivalent objects. Group the objects in this...Ch. 5.1 - Clips. Is a paper clip equivalent to a circle? If...Ch. 5.1 - Pennies plus. Consider the two objects pictured...Ch. 5.1 - Starry-eyed. Consider the two stars below. Are...Ch. 5.1 - Learning the ropes. Pictured below are two ropes,...Ch. 5.1 - HoIy spheres. Consider the two spheres shown. Each...Ch. 5.1 - From sphere to torus. The following sequence of...Ch. 5.1 - Half full, half empty. One glass is half filled...Ch. 5.1 - Male versus female. Consider the male and female...Ch. 5.1 - Holey tori. Are these two objects equivalent by...Ch. 5.1 - More holey tori (H). Are these two objects...Ch. 5.1 - Last holey tori. Are these two objects equivalent...Ch. 5.1 - Beyond the holey inner tube. Suppose you are given...Ch. 5.1 - Heavy metal. Carefully examine this picture of a...Ch. 5.1 - The disk and the inner tube (ExH). Suppose you...Ch. 5.1 - Building a torus (S). Suppose you are given a...Ch. 5.1 - Lasso that hole. Consider the first two tori on...Ch. 5.1 - Knots in dougtnuts. We are given two solid...Ch. 5.1 - From knots to glasses (ExH). Take the thickened...Ch. 5.1 - More Jell-O. Suppose we take a cube of Jell-O,...Ch. 5.1 - Fixed spheres (H). We are given two spheres made...Ch. 5.1 - Holes. Is a torus equivalent to a two-holed torus?...Ch. 5.1 - More holes. Is a two-holed torus equivalent to a...Ch. 5.1 - Here we celebrate the power of algebra as a...Ch. 5.1 - Here we celebrate the power of algebra as a...Ch. 5.1 - Here we celebrate the power of algebra as a...Ch. 5.1 - Here we celebrate the power of algebra as a...Ch. 5.1 - Here we celebrate the power of algebra as a...Ch. 5.2 - One side to every story. What is a Mobius band?Ch. 5.2 - Maybe Mobius. How can you look at a loop of paper...Ch. 5.2 - Singin the blues. Take an ordinary strip of white...Ch. 5.2 - Whos blue now? Take an ordinary strip of white...Ch. 5.2 - Twisted sister. Your sister holds a strip of...Ch. 5.2 - Two twists. Take a strip of paper, put two half...Ch. 5.2 - Two twists again. Take a strip of paper, put two...Ch. 5.2 - Three twists (H). Take a strip of paper, put three...Ch. 5.2 - Prob. 11MSCh. 5.2 - Möbius lengths. Use the edge identification...Ch. 5.2 - Squash and cut. Take a Möbius band and squash it...Ch. 5.2 - Two at once. Take two strips of paper and put them...Ch. 5.2 - Parallel Möbius. Is it possible to have two...Ch. 5.2 - Puzzling. Suppose you have a collection of jigsaw...Ch. 5.2 - Möbius triangle. Make a 1-inch-wide Möbius band,...Ch. 5.2 - Thickened Möbius. Imagine a Möbius band...Ch. 5.2 - Thickened faces. How many faces (sides) does a...Ch. 5.2 - Thick then thin. Suppose we take a Môbius band,...Ch. 5.2 - Drawing the band (ExH). Imagine you have a Möbius...Ch. 5.2 - Tubing (H). Suppose we take two Möbius bands and...Ch. 5.2 - Bug out (ExH). Suppose you are a ladybug on the...Ch. 5.2 - Open cider. Consider the Klein bottle half filled...Ch. 5.2 - Rubber Klein (S). Suppose you have a rectangular...Ch. 5.2 - One edge. Using the method on page 347 for...Ch. 5.2 - Twist of fate (S). Using the edge-identification...Ch. 5.2 - Linked together. Using the edge-identification...Ch. 5.2 - Count twists. Using the edge-identification...Ch. 5.2 - Dont cross. Can you draw a curve that does not...Ch. 5.2 - Twisted up (H). Suppose you are given a band of...Ch. 5.2 - Prob. 32MSCh. 5.2 - Find a band. Find a Möbius band on the surface of...Ch. 5.2 - Holy Klein. Show that the figure on the left is...Ch. 5.2 - Möbius Möbius. Show that the Klein bottle is two...Ch. 5.2 - Attaching tubes. Consider a Möbius band with two...Ch. 5.2 - Möbius map (H). Using felt-tip color pens that...Ch. 5.2 - Thick slices. Thicken a Môbius band and then...Ch. 5.2 - Bagel slices. If we take a bagel and slice it in...Ch. 5.2 - Gluing and cutting. Consider a rectangular sheet...Ch. 5.2 - Here we celebrate the power of algebra as a...Ch. 5.2 - Here we celebrate the power of algebra as a...Ch. 5.2 - Here we celebrate the power of algebra as a...Ch. 5.2 - Here we celebrate the power of algebra as a...Ch. 5.2 - Here we celebrate the power of algebra as a...Ch. 5.3 - Knotty start. Which of the followign knots are...Ch. 5.3 - The not knot. What is the unknot?Ch. 5.3 - Crossing count. Count the crossings in each knot...Ch. 5.3 - Tangled up. Is the figure below a knot or a link?Ch. 5.3 - Ringing endorsement. What are the Borromean rings?Ch. 5.3 - Human trefoil. What is the minimum number of...Ch. 5.3 - Human figure eight. What is the minimum number of...Ch. 5.3 - Stick number (ExH). What is the smallest number...Ch. 5.3 - More Möbius. Make a Möbius band with three half...Ch. 5.3 - Slinky (H). Take a Slinky, lengthen one of its...Ch. 5.3 - More slink. Take a Slinky, and this time weave an...Ch. 5.3 - Make it. Use a piece of string or an extenstion...Ch. 5.3 - Knotted (S). Take an unknotted loop. Tie a knot in...Ch. 5.3 - Slip. Take an unknotted loop and put a slip knot...Ch. 5.3 - Dollar link. Take two paper clips and a dollar and...Ch. 5.3 - Prob. 18MSCh. 5.3 - Unknotting knots (H). In each of the two knots at...Ch. 5.3 - Alternating. A picture of a knot is alternating...Ch. 5.3 - Making it alternating. Consider the knot on the...Ch. 5.3 - Prob. 22MSCh. 5.3 - One cross (H). Prove that any loop with exactly...Ch. 5.3 - Two loops (S). Is there a picture of two linked...Ch. 5.3 - Hold the phone. Disconnect the wire from the phone...Ch. 5.3 - More unknotting knots. In these two knots, find...Ch. 5.3 - Unknotting pictures (S). Suppose you are given a...Ch. 5.3 - Twisted. Suppose we are given a figure consisting...Ch. 5.3 - More alternating. First reread Mindscape 20. For...Ch. 5.3 - Crossing numbers. Suppose you are given pictures...Ch. 5.3 - Lots of crossings. Suppose you arc given a picture...Ch. 5.3 - Torus knots (H). Can you draw a trefoil knot on a...Ch. 5.3 - Two crosses. Prove that any loop with exactly two...Ch. 5.3 - Hoop it up. Show that every knot can be positioned...Ch. 5.3 - The switcheroo. Pictured below is a way of...Ch. 5.3 - 4D washout. Why is the study of knots and links...Ch. 5.3 - Brunnian links (H). Link four loops together in...Ch. 5.3 - Fire drill (ExH). A fire starts in your...Ch. 5.3 - Fixed spheres again. We are given two spheres that...Ch. 5.3 - Here we celebrate the power of algebra as a...Ch. 5.3 - Here we celebrate the power of algebra as a...Ch. 5.3 - Here we celebrate the power of algebra as a...Ch. 5.3 - Here we celebrate the power of algebra as a...Ch. 5.3 - Here we celebrate the power of algebra as a...Ch. 5.4 - Fixed things first. What does the Brouwer Fixed...Ch. 5.4 - Say cheese. Youre making an open-faced cheese...Ch. 5.4 - Fixed flapjacks. Youre making pancakes and...Ch. 5.4 - Prob. 4MSCh. 5.4 - Loop around. What does the Hot Loop Theorem...Ch. 5.4 - Fixed on a square. Does the Brouwer Fixed Point...Ch. 5.4 - Fixed on a circle. Does the Brouwer Fixed Point...Ch. 5.4 - Winding arrows. In each drawing below we have a...Ch. 5.4 - Prob. 10MSCh. 5.4 - Prob. 11MSCh. 5.4 - Home heating (H). Prove that there are two points...Ch. 5.4 - Prob. 13MSCh. 5.4 - Prob. 14MSCh. 5.4 - Prob. 15MSCh. 5.4 - Lining up (H). Suppose we have two line segments...Ch. 5.4 - A nice temp. Must there be two antipodal points on...Ch. 5.4 - Prob. 18MSCh. 5.4 - Diet drill. Suppose someone weighs 160 lbs. and...Ch. 5.4 - Speedy (S). You enter a tollway and are given a...Ch. 5.4 - The cut core. Suppose we have the red and blue...Ch. 5.4 - Fixed without boundary. Do you think that the...Ch. 5.4 - Take a hike (ExH). A hiker decides to climb up...Ch. 5.4 - Here we celebrate the power of algebra as a...Ch. 5.4 - Here we celebrate the power of algebra as a...Ch. 5.4 - Here we celebrate the power of algebra as a...Ch. 5.4 - Here we celebrate the power of algebra as a...Ch. 5.4 - Here we celebrate the power of algebra as a...
Additional Math Textbook Solutions
Find more solutions based on key concepts
Voting A random sample of likely voters showed that 49 planned to support Measure X. The margin of error is 3 p...
Introductory Statistics
Explain the meaning of the term “statistically significant difference” in statistics terminology.
Intro Stats, Books a la Carte Edition (5th Edition)
Standard Normal Distribution. In Exercises 9–12, find the area of the shaded region. The graph depicts the stan...
Elementary Statistics (13th Edition)
Testing Hypotheses. In Exercises 13-24, assume that a simple random sample has been selected and test the given...
Elementary Statistics Using The Ti-83/84 Plus Calculator, Books A La Carte Edition (5th Edition)
Mathematical Connections Explain why a number and a numeral are considered different.
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.Similar questions
- 2. [-/1 Points] DETAILS MY NOTES SESSCALCET2 6.4.006.MI. Use the Table of Integrals to evaluate the integral. (Remember to use absolute values where appropriate. Use C for the constant of integration.) 7y2 y² 11 dy Need Help? Read It Master It SUBMIT ANSWER 3. [-/1 Points] DETAILS MY NOTES SESSCALCET2 6.4.009. Use the Table of Integrals to evaluate the integral. (Remember to use absolute values where appropriate. Use C for the constant of integration.) tan³(12/z) dz Need Help? Read It Watch It SUBMIT ANSWER 4. [-/1 Points] DETAILS MY NOTES SESSCALCET2 6.4.014. Use the Table of Integrals to evaluate the integral. (Use C for the constant of integration.) 5 sinб12x dx Need Help? Read Itarrow_forwardPlease refer belowarrow_forwardy"-9y+20y= 80t-156 y(0) = −6, y'(0) = 5 y(t) =arrow_forward
- Please refer belowarrow_forwardHi, can you guys help me with this? Thank you! Can you guys help me calculate again the Term GPA, Combined GPA, Cumulative GPA, Transfer GPA & Combined Cumulative GPA section? It's just not right right now. Here's the transfer totals point that I want to provide just in case you guys may ask where I get these from:arrow_forwardUse undetermined coefficients to find the particular solution to y"-3y+2y=4e3 Y(t) =arrow_forward
- Please refer belowarrow_forwardUse undetermined coefficients to find the particular solution to y"-y+5y = 7t² +61 +3 Yp(t) =arrow_forwardConsider the initial value problem y"+y'-12y= 0, y(0) = a, y'(0) = 4 Find the value of a so that the solution to the initial value problem approaches zero as too a =arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Holt Mcdougal Larson Pre-algebra: Student Edition...
Algebra
ISBN:9780547587776
Author:HOLT MCDOUGAL
Publisher:HOLT MCDOUGAL
Surface Area Of A Sphere | Geometry | Math | Letstute; Author: Let'stute;https://www.youtube.com/watch?v=T_DBkFnr4NM;License: Standard YouTube License, CC-BY