Mathematical Excursions (MindTap Course List)
4th Edition
ISBN: 9781305965584
Author: Richard N. Aufmann, Joanne Lockwood, Richard D. Nation, Daniel K. Clegg
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 5.2, Problem 9ES
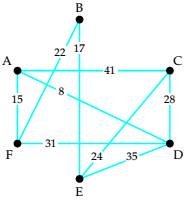
Use trial and error to find two Hamiltonian circuits of different total weights, starting at vertex A in the weighted graph. Compute the total weight of each circuit.
Expert Solution & Answer
Trending nowThis is a popular solution!
Students have asked these similar questions
please solve handwritten without use of AI
You’re scrolling through Instagram and you notice that a lot of people are posting selfies. This piques yourcuriosity and you want to estimate the percentage of photos on Instagram that are selfies.(a) (5 points) Is there a “ground truth” for the percentage of selfies on Instagram? Why or why not?(b) (5 points) Is it possible to estimate the ground truth percentage of selfies on Instagram?Irrespective of your answer to the previous question, you decide to pull up n = 250 randomly chosenphotos from your friends’ Instagram accounts and find that 32% of these photos are selfies.(c) (15 points) Determine which of the following is an observation, a variable, a sample statistic (valuecalculated based on the observed sample), or a population parameter.• A photo on Instagram.• Whether or not a photo is a selfie.• Percentage of all photos on Instagram that are selfies.• 32%.(d) (5 points) Based on the sample you collected, do you think 32% is a reliable ballpark estimate for theground truth…
Part 1 and 2
Chapter 5 Solutions
Mathematical Excursions (MindTap Course List)
Ch. 5.1 - A pen-tracing puzzle is given. See if you can find...Ch. 5.1 - A pen-tracing puzzle is given. See if you can find...Ch. 5.1 - A pen-tracing puzzle is given. See if you can find...Ch. 5.1 - A pen-tracing puzzle is given. See if you can find...Ch. 5.1 - Explain why the following pen-tracing puzzle is...Ch. 5.1 - Transportation An X in the table below indicates a...Ch. 5.1 - Transportation The table below shows the nonstop...Ch. 5.1 - Social Network A group of friends is represented...Ch. 5.1 - Prob. 4ESCh. 5.1 - Determine (a) the number of edges in the graph,...
Ch. 5.1 - Determine (a) the number of edges in the graph,...Ch. 5.1 - Determine (a) the number of edges in the graph,...Ch. 5.1 - Determine (a) the number of edges in the graph,...Ch. 5.1 - Determine whether the two graphs are equivalent.Ch. 5.1 - Determine whether the two graphs are equivalent.Ch. 5.1 - Determine whether the two graphs are equivalent.Ch. 5.1 - Determine whether the two graphs are equivalent.Ch. 5.1 - Explain why the following two graphs cannot be...Ch. 5.1 - Label the vertices of the second graph so that it...Ch. 5.1 - (a) determine whether the graph is Eulerian. If it...Ch. 5.1 - (a) determine whether the graph is Eulerian. If it...Ch. 5.1 - (a) determine whether the graph is Eulerian. If it...Ch. 5.1 - (a) determine whether the graph is Eulerian. If it...Ch. 5.1 - (a) determine whether the graph is Eulerian. If it...Ch. 5.1 - (a) determine whether the graph is Eulerian. If it...Ch. 5.1 - (a) determine whether the graph is Eulerian. If it...Ch. 5.1 - (a) determine whether the graph is Eulerian. If it...Ch. 5.1 - Parks in Exercises 23 and 24, a map of a park is...Ch. 5.1 - Parks in Exercises 23 and 24, a map of a park is...Ch. 5.1 - Transportation For the train routes given in...Ch. 5.1 - Transportation For the direct air flights given in...Ch. 5.1 - Pets The diagram below shows the arrangement of a...Ch. 5.1 - Transportation A subway map is shown below. Is it...Ch. 5.1 - Prob. 29ESCh. 5.1 - Prob. 30ESCh. 5.1 - Degrees of Separation In the graph below, an edge...Ch. 5.1 - Social Network In the graph below, an edge...Ch. 5.1 - Prob. 33ESCh. 5.1 - Travel A map of South America is shown at the...Ch. 5.2 - Continue investigating Hamiltonian circuits in...Ch. 5.2 - Use the greedy algorithm and the weighted graph...Ch. 5.2 - Use the edge-picking algorithm to find a...Ch. 5.2 - Use Dirac's theorem to verify that the graph is...Ch. 5.2 - Use Dirac's theorem to verify that the graph is...Ch. 5.2 - Use Dirac's theorem to verify that the graph is...Ch. 5.2 - Use Dirac's theorem to verify that the graph is...Ch. 5.2 - Transportation For the train routes given in...Ch. 5.2 - Transportation For the direct air flights given in...Ch. 5.2 - Use trial and error to find two Hamiltonian...Ch. 5.2 - Use trial and error to find two Hamiltonian...Ch. 5.2 - Use trial and error to find two Hamiltonian...Ch. 5.2 - Use trial and error to find two Hamiltonian...Ch. 5.2 - Use the greedy algorithm to find a Hamiltonian...Ch. 5.2 - Use the greedy algorithm to find a Hamiltonian...Ch. 5.2 - Use the greedy algorithm to find a Hamiltonian...Ch. 5.2 - Use the greedy algorithm to find a Hamiltonian...Ch. 5.2 - Use the edge-picking algorithm to find a...Ch. 5.2 - Use the edge-picking algorithm to find a...Ch. 5.2 - Use the edge-picking algorithm to find a...Ch. 5.2 - Use the edge-picking algorithm to find a...Ch. 5.2 - Travel A company representative lives in...Ch. 5.2 - Travel A tourist is staying in Toronto, Canada,...Ch. 5.2 - Travel Use the edge-picking algorithm to design a...Ch. 5.2 - Travel Use the edge-picking algorithm to design a...Ch. 5.2 - Travel Nicole wants to tour Asia. She will start...Ch. 5.2 - Travel The prices for traveling between five...Ch. 5.2 - Travel Use the edge-picking algorithm to find a...Ch. 5.2 - Travel Use the edge-picking algorithm to find a...Ch. 5.2 - Route Planning Brian needs to visit the pet store,...Ch. 5.2 - Route Planning A bike messenger needs to deliver...Ch. 5.2 - Scheduling A research company has a large...Ch. 5.2 - Computer Networks A small office wishes to network...Ch. 5.2 - Route Planning A security officer patrolling a...Ch. 5.2 - Route Planning A city engineer needs to inspect...Ch. 5.2 - Draw a connected graph with six vertices that has...Ch. 5.2 - Assign weights to the edges of the following...Ch. 5.3 - The tetrahedron in figure 5.20 consists of four...Ch. 5.3 - The following graph is the projection of one ofthe...Ch. 5.3 - Prob. 3EECh. 5.3 - Give a reason why the graph below Cannot be the...Ch. 5.3 - Prob. 1ESCh. 5.3 - Prob. 2ESCh. 5.3 - Prob. 3ESCh. 5.3 - Prob. 4ESCh. 5.3 - Prob. 5ESCh. 5.3 - Prob. 6ESCh. 5.3 - Prob. 7ESCh. 5.3 - Prob. 8ESCh. 5.3 - Prob. 9ESCh. 5.3 - Prob. 10ESCh. 5.3 - Prob. 11ESCh. 5.3 - Prob. 12ESCh. 5.3 - Show that the following graph contracts to K5.Ch. 5.3 - Show that the following graph contracts to the...Ch. 5.3 - Prob. 15ESCh. 5.3 - Prob. 16ESCh. 5.3 - Prob. 17ESCh. 5.3 - Count the number of vertices, edges, and faces,...Ch. 5.3 - Count the number of vertices, edges, and faces,...Ch. 5.3 - Count the number of vertices, edges, and faces,...Ch. 5.3 - Count the number of vertices, edges, and faces,...Ch. 5.3 - Count the number of vertices, edges, and faces,...Ch. 5.3 - Prob. 23ESCh. 5.3 - Prob. 24ESCh. 5.3 - Prob. 25ESCh. 5.3 - Prob. 26ESCh. 5.3 - Prob. 27ESCh. 5.3 - Prob. 28ESCh. 5.3 - Prob. 29ESCh. 5.3 - Prob. 30ESCh. 5.4 - A one-way road ends at a two-way street. The...Ch. 5.4 - A one-way road intersects a two-way road in a...Ch. 5.4 - A two-way road intersects another two-way road in...Ch. 5.4 - Prob. 1ESCh. 5.4 - Prob. 2ESCh. 5.4 - Prob. 3ESCh. 5.4 - Prob. 4ESCh. 5.4 - Prob. 5ESCh. 5.4 - Prob. 6ESCh. 5.4 - Prob. 7ESCh. 5.4 - Prob. 8ESCh. 5.4 - Prob. 9ESCh. 5.4 - Prob. 10ESCh. 5.4 - Prob. 11ESCh. 5.4 - Prob. 12ESCh. 5.4 - Prob. 13ESCh. 5.4 - Prob. 14ESCh. 5.4 - Prob. 15ESCh. 5.4 - Prob. 16ESCh. 5.4 - Prob. 17ESCh. 5.4 - Prob. 18ESCh. 5.4 - Prob. 19ESCh. 5.4 - Prob. 20ESCh. 5.4 - Prob. 21ESCh. 5.4 - Prob. 22ESCh. 5.4 - Scheduling Six different groups of children would...Ch. 5.4 - Scheduling Five different charity organizations...Ch. 5.4 - Scheduling Students in a film class have...Ch. 5.4 - Animal Housing A researcher has discovered six new...Ch. 5.4 - Prob. 27ESCh. 5.4 - Prob. 28ESCh. 5.4 - Prob. 29ESCh. 5.4 - Prob. 30ESCh. 5.4 - Scheduling Edge colorings, as explained in...Ch. 5 - (a) determine the number of edges in the graph,...Ch. 5 - (a) determine the number of edges in the graph,...Ch. 5 - Soccer In the table below, an X indicates teams...Ch. 5 - Each vertex in the graph at the left represents a...Ch. 5 - Determine whether the two graphs are equivalent.Ch. 5 - Determine whether the two graphs are equivalent.Ch. 5 - Find an Euler path if possible, and (b) find an...Ch. 5 - Find an Euler path if possible, and (b) find an...Ch. 5 - Find an Euler path if possible, and (b) find an...Ch. 5 - Prob. 10RECh. 5 - Prob. 11RECh. 5 - Architecture The floor plan of a sculpture gallery...Ch. 5 - Use Dirac's theorem to verify that the graph is...Ch. 5 - Use Dirac's theorem to verify that the graph is...Ch. 5 - Prob. 15RECh. 5 - Prob. 16RECh. 5 - Use the greedy algorithm to find a Hamiltonian...Ch. 5 - Use the greedy algorithm to find a Hamiltonian...Ch. 5 - Use the edge-picking algorithm to find a...Ch. 5 - Use the edge-picking algorithm to find a...Ch. 5 - Efficient Route The distances, in miles, between...Ch. 5 - Computer Networking A small office needs to...Ch. 5 - Prob. 23RECh. 5 - Prob. 24RECh. 5 - Prob. 25RECh. 5 - Prob. 26RECh. 5 - Count the number of vertices, edges, and faces in...Ch. 5 - Count the number of vertices, edges, and faces in...Ch. 5 - Prob. 29RECh. 5 - Prob. 30RECh. 5 - Prob. 31RECh. 5 - Prob. 32RECh. 5 - Prob. 33RECh. 5 - Prob. 34RECh. 5 - Scheduling A company has scheduled a retreat at a...Ch. 5 - Social Network Each vertex in the graph at the...Ch. 5 - Determine whether the following two graphs are...Ch. 5 - Answer the following questions for the graph shown...Ch. 5 - Recreation The illustration below depicts bridges...Ch. 5 - a. What does Dirac's theorem state? Explain how it...Ch. 5 - Low-Cost Route The table below shows the cost of...Ch. 5 - Use the greedy algorithm to find a Hamiltonian...Ch. 5 - Prob. 8TCh. 5 - Answer the following questions for the graph shown...Ch. 5 - Prob. 10TCh. 5 - Prob. 11TCh. 5 - A group of eight friends is planning a vacation in...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.Similar questions
- Part 1 and 2arrow_forwardAdvanced Mathematics Mastery Quiz Instructions: . No partial credit will be awarded; any mistake will result in a score of 0. . Submit your solution before the deadline. • Ensure your solution is detailed, and all steps are well-documented. . No Al tools (such as ChatGPT or others) may be used to assist in solving the problems. All work must be your own. Solutions will be checked for Al usage and plagiarism. Any detected violation will result in a score of 0. Problem Let the function f(x, y, z) = r³y-2xy + 3yz² +e+y+ and consider the following tasks: 1. [Critical Points and Classification] a. Find all critical points of f(x, y, z). b. Use the second partial derivative test to classify each critical point as a local minimum, local maximum, or saddle point. 2. [Gradient and Divergence] a. Compute the gradient vector Vf. b. Calculate the divergence of the gradient field and explain its significance. 3. [Line Integral Evaluation] Consider the vector field F(x, y, z) = (e² + yz, x²y ar). a.…arrow_forwardAdvanced Functional Analysis Mastery Quiz Instructions: . No partial credit will be awarded; any mistake will result in a score of 0. ⚫ Submit your solution before the deadline. . Ensure your solution is detailed, and all steps are well-documented. • No Al tools (such as ChatGPT or others) may be used to assist in solving the problems. All work must be your own. Solutions will be checked for Al usage and plagiarism. Any detected violation will result in a score of 0. Problem Let X te a Banach space, and let T: XX be a linear operetor satisfying ||T|| - 1. Corsider the following tasks: 1. [Bounded Linear Operators] a. Prove that I is a bounded linear operator if and only if there exists a constant C such that ||T()||C|||| for all 2 € X. b. Show that if I' is a linear operator on a Banach space X and ||T||-1, then ||T(x)||||||| for all EX. 2. [Spectral Theorem] Let A be a self-adjoint operator on a Hibert space H. Assume that A has a non-empty spectrum. a. State and prove the Spectral…arrow_forward
- Advanced Mathematics Mastery Quiz Instructions: . No partial credit will be awarded; any mistake will result in a score of 0. Submit your solution before the deadline. . Ensure your solution is detailed, and all steps are well-documented. . . No Al tools (such as ChatGPT or others) may be used to assist in solving the problems. All work must be your own. Solutions will be checked for Al usage and plagiarism. Any detected violation will result in a score of 0. Problem Let the function f(x, y, z)=-42y+2ay" +22 tasks: and consider the following 1. [Critical Points and Classification] a. Find all critical points of f(x, y, z). b. Use the second partial derivative test to classify each critical point as a local minimum, local maximum, or saddle point. 2. [Directional Derivatives and Gradients] a. Compute the gradient vector Vf of f(x, y, z). b. Find the directional derivative of f at the point (1, 1, 1) in the direction of the vector v = (1,-2,3). 3. [Line Integral Evaluation] Consider the…arrow_forwardQ11. A president and a treasurer are to be chosen from a student club consisting of 50 people. How many different choices of officers are possible if (a) there are no restrictions (b) A will serve only if he is president (c) B and C will serve together or not at allarrow_forwardAdvanced Functional Analysis Mastery Quiz Instructions: . . No partial credit will be awarded; any mistake will result in a score of 0. Submit your solution before the deadline. . Ensure your solution is detailed, and all steps are well-documented. . . No Al tools (such as ChatGPT or others) may be used to assist in solving the problems. All work must be your own. Solutions will be checked for Al usage and plagiarism. Any detected violation will result in a score of 0. Problem Let X and Y be Banach spaces, and let T: XY be a bounded linear operator. Consider the following tasks: 1. [Baire's Category Theorem and Applications] a. State and prove Baire's Category Theorem for Banach spaces. Use the theorem to prove that a complete metric space cannot be the countable union of nowhere dense sets. b. Use Baire's Category Theorem to show that if T: XY is a bounded linear operator between Banach spaces, then the set of points in X where I' is continuous is a dense G8 set. 2. [Norms and…arrow_forward
- Advanced Functional Analysis Mastery Quiz Instructions: No partial credit will be awarded; any mistake will result in a score of 0. . Submit your solution before the deadline. . Ensure your solution is detailed, and all steps are well-documented. No Al tools (such as ChatGPT or others) may be used to assist in solving the problems. All work must be your own. Solutions will be checked for Al usage and plagiarism. Any detected violation will result in a score of 0. Problem Let X be a Banach space, and 7' be a bounded linear operator acting on X. Consider the following tasks: 1. [Operator Norm and Boundedness] a. Prove that the operator norm of a linear operator T': X →→ X is given by: ||T|| =sup ||T(2)|| 2-1 b. Show that if 'T' is a bounded linear operator on a Banach space, then the sequence {7"} converges to zero pointwise on any bounded subset of X if and only if ||T|| p, from X to X, where 4, (y)=(x, y), is a linear operator. b. Consider a sequence {} CX. Prove that if →→ 6(2)→→ (2)…arrow_forwardSolve this differential equation: dy 0.05y(900 - y) dt y(0) = 2 y(t) =arrow_forwardMathematics Challenge Quiz Instructions: • You must submit your solution before the deadline. • Any mistake will result in a score of 0 for this quiz. • Partial credit is not allowed; ensure your answer is complete and accurate. Problem Consider the parametric equations: x(t) = e cos(3t), y(t) = e sin(3t) fort Є R. 1. [Parametric Curve Analysis] a. Prove that the parametric curve represents a spiral by eliminating t and deriving the general equation in Cartesian form. b. Find the curvature (t) of the curve at any point 1. 2. [Integral Evaluation] For the region enclosed by the spiral between t = 0 and t =π, compute the area using the formula: where t₁ = 0 and t₂ = . A == √ √ ²x²(1)y (t) − y(t) x' (t)] dt 3. [Differential Equation Application] The curve satisfies a differential equation of the form: d'y da2 dy + P(x)+q(x)y = 0 a. Derive the explicit forms of p(x) and q(2). b. Verify your solution by substituting (t) and y(t) into the differential equation. 4. [Optimization and Limits]…arrow_forward
- Advanced Functional Analysis Mastery Quiz Instructions: No partial credit will be awarded: any mistake will result in a score of 0. Submit your solution before the deadline. Ensure your solution is detailed, and all stops are well-documented. No Al tools (such as ChatGPT or others) may be used to assist in solving the problems. All work must be your own. Solutions will be checked for Al usage and plagiarism. Any detected violation will result in a score of 0. Problem Let X and Y be Banach spaces, and let T: X →Y be a bounded linear operator. Consider the following tasks: 1. [Banach Fixed-Point Theorem] a State and prove the Banach Fixed-Point Theorem (Contraction Mapping Theorem). Provide a detailed explanation of how the theorem guarantees the existence of a unique fixed point for a contraction mapping on a complete metric space. b. Let T: X → X be a contraction mapping on X = R² with T(r. u) = (3.). Find the unique fixed point of T. 2. [Duality and the Hahn-Banach Theorem] a. State…arrow_forwardSuppose that you are holding your toy submarine under the water. You release it and it begins to ascend. The graph models the depth of the submarine as a function of time. What is the domain and range of the function in the graph? 1- t (time) 1 2 4/5 6 7 8 -2 -3 456700 -4 -5 -6 -7 d (depth) -8 D: 00 t≤ R:arrow_forward0 5 -1 2 1 N = 1 to x = 3 Based on the graph above, estimate to one decimal place the average rate of change from x =arrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_ios
Recommended textbooks for you
Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
College AlgebraAlgebraISBN:9781305115545Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Elementary Linear Algebra (MindTap Course List)AlgebraISBN:9781305658004Author:Ron LarsonPublisher:Cengage Learning
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
Algebra and Trigonometry (MindTap Course List)
Algebra
ISBN:9781305071742
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning
College Algebra
Algebra
ISBN:9781305115545
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning
Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Elementary Linear Algebra (MindTap Course List)
Algebra
ISBN:9781305658004
Author:Ron Larson
Publisher:Cengage Learning
College Algebra (MindTap Course List)
Algebra
ISBN:9781305652231
Author:R. David Gustafson, Jeff Hughes
Publisher:Cengage Learning
Graph Theory: Euler Paths and Euler Circuits; Author: Mathispower4u;https://www.youtube.com/watch?v=5M-m62qTR-s;License: Standard YouTube License, CC-BY
WALK,TRIAL,CIRCUIT,PATH,CYCLE IN GRAPH THEORY; Author: DIVVELA SRINIVASA RAO;https://www.youtube.com/watch?v=iYVltZtnAik;License: Standard YouTube License, CC-BY