Chapter 5.2, Problem 28E

a.

To determine

Find the equation of the least-squares line, if y is expressed in kilograms instead of pounds.

Answer to Problem 28E

The equation of the least-squares line, if y is expressed in kilograms instead of pounds is $\underset{\_}{\widehat{y}=-427+3.90x}$.

Explanation of Solution

Calculation:

The least-squares regression equation relating the shear strength (pounds or lb), y of steel to weld diameter, x is given as $\widehat{y}=-936.22+8.577x$. It is also mentioned that $1\text{ lb}=0.4536\text{ kg}$, so that, when y is expressed in kilograms instead of pounds, $\text{new }y=0.4536\left(\text{old }y\right)$.

Data transformation:

Software procedure:

Step-by-step procedure to transform the data using the MINITAB software:

• Choose Calc > Calculator.
• Enter the column of y1 under Store result in variable.
• Enter the 0.4536*‘y’ in Expression.
• Click OK in all dialogue boxes.

The transformed data, where each $\text{new }y=0.4536\left(\text{old }y\right)$, is stored in the column y1.

The least-squares equation can be obtained using software.

Least-squares equation:

Software procedure:

Step-by-step procedure to obtain the least-squares equation using the MINITAB software:

• Choose Stat > Regression > Regression > Fit Regression Model.
• Enter the column of y1 under Responses.
• Enter the column of x under Continuous predictors.
• Choose Results and select Coefficients, Regression Equation.
• Click OK in all dialogue boxes.

Output obtained using MINITAB is given below:

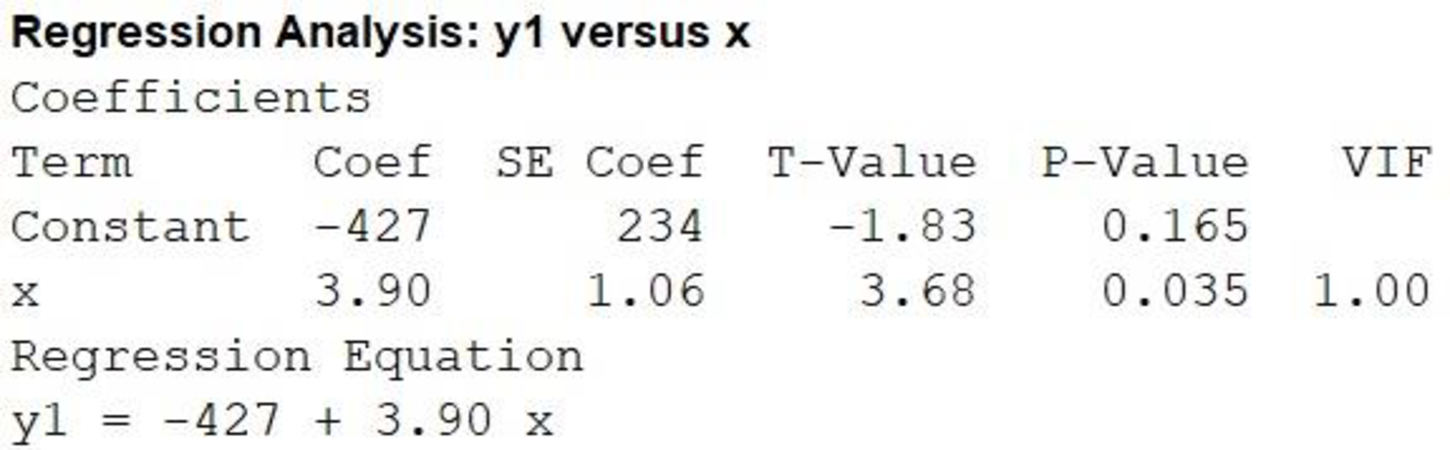
Thus, the equation of the least-squares line, if y is expressed in kilograms instead of pounds is $\underset{\_}{\widehat{y}=-427+3.90x}$.

b.

To determine

Find the new slope, intercept, and equation of the least-squares line, if a constant, c is multiplied with every observation on y.

Answer to Problem 28E

The new slope is –936.22 c.

The new intercept is 8.577 c.

The new equation of the least-squares line is $\underset{\_}{\widehat{y}=-936.22c+8.577cx}$.

Explanation of Solution

Calculation:

Changing the unit of y from pounds to kilograms causes a change in the scale of y. Now, if the scale of the response variable in a regression, y, is changed by multiplying with a constant c, each least-squares coefficient is also multiplied by c.

Thus, each coefficient in the regression equation, that is, the intercept –936.22 and the slope corresponding to x, 8.577, will be multiplied by c, in order to get the regression equation in this situation. The regression equation is $\text{new}\left(\widehat{y}\right)=-936.22c+8.577cx$.

Thus, the new slope is –936.22 c; the new intercept is 8.577 c; the new equation of the least-squares line is $\underset{\_}{\widehat{y}=-936.22c+8.577cx}$.

These can be verified by using the formulae for a and b. The general formulae for a and b, for a simple least-squares regression equation, $\widehat{y}=a+bx$, are as follows:

$\begin{array}{l}a=\overline{y}-b\overline{x};\\ b=\frac{{\displaystyle \sum xy}-\frac{\left({\displaystyle \sum x}\right)\left({\displaystyle \sum y}\right)}{n}}{{\displaystyle \sum x^2}-\frac{{\left({\displaystyle \sum x}\right)}^2}{n}}.\end{array}$

Now, $\text{new }y=c\left(\text{old }y\right)$, replace each value of y by cy in the above formulae. Note that, if each observation on y is multiplied by c, then the mean of y is also multiplied by c, that is, $\text{new }\overline{y}=c\overline{y}$.

Therefore,

$\begin{array}{c}\text{new}b=\frac{{\displaystyle \sum x\left(cy\right)}-\frac{\left({\displaystyle \sum x}\right)\left({\displaystyle \sum cy}\right)}{n}}{{\displaystyle \sum x^2}-\frac{{\left({\displaystyle \sum x}\right)}^2}{n}}\\ =\frac{c{\displaystyle \sum xy}-\frac{c\left({\displaystyle \sum x}\right)\left({\displaystyle \sum y}\right)}{n}}{{\displaystyle \sum x^2}-\frac{{\left({\displaystyle \sum x}\right)}^2}{n}}\\ =c\left[\frac{{\displaystyle \sum xy}-\frac{\left({\displaystyle \sum x}\right)\left({\displaystyle \sum y}\right)}{n}}{{\displaystyle \sum x^2}-\frac{{\left({\displaystyle \sum x}\right)}^2}{n}}\right]\\ =cb.\end{array}$

$\begin{array}{c}\text{new}a=c\overline{y}-\left(\text{new}b\right)\overline{x}\\ =c\overline{y}-cb\overline{x}\\ =c\left(\overline{y}-b\overline{x}\right)\\ =ca.\end{array}$

Substitute the values of new a and newb in the general form of the regression equation.

$\begin{array}{c}\text{new}\widehat{y}=\text{new}a+\text{new}bx\\ =ca+cbx.\end{array}$

In this problem, a = –936.22 and b = 8.577. As a result, the new slope is –936.22 c; the new intercept is 8.577 c.

Substituting these values in the equation for $\text{new}\widehat{y}$, the new equation of the least-squares line is $\underset{\_}{\widehat{y}=-936.22c+8.577cx}$.