
Concept explainers
This exercise comes to you courtesy of Euler himself. Here is the question in Euler's own words,
accompanied by the diagram shown in Fig. 5-63.
Let us take an example of two islands with four rivers forming the surrounding water. There are fifteen bridges marked a, b, c, d, etc., across the water around the islands and the adjoining rivers. The question is whether a journey can be arranged that will pass over all the bridges but not over any of them more than once.
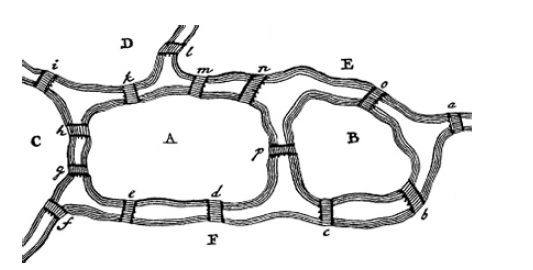
Figure 5-63
What is the answer to Euler's question? If the “journey” is possible, describe it. If it isn't, explain why not.
Want to see the full answer?
Check out a sample textbook solution
Chapter 5 Solutions
Excursions in Modern Mathematics (9th Edition)
Additional Math Textbook Solutions
Pathways To Math Literacy (looseleaf)
College Algebra (7th Edition)
Introductory Statistics
Elementary Statistics: Picturing the World (7th Edition)
Finite Mathematics for Business, Economics, Life Sciences and Social Sciences
Elementary Statistics (13th Edition)
- Example: If ƒ (x + 2π) = ƒ (x), find the Fourier expansion f(x) = eax in the interval [−π,π]arrow_forwardThis box plot represents the score out of 90 received by students on a driver's education exam. 75% of the students passed the exam. What is the minimum score needed to pass the exam? Submitting x and Whickers Graph Low 62, C 62 66 70 74 78 82 86 90 Driver's education exam score (out of 90)arrow_forwardExample: If ƒ (x + 2π) = ƒ (x), find the Fourier expansion f(x) = eax in the interval [−π,π]arrow_forward
- Please can you give detailed steps on how the solutions change from complex form to real form. Thanks.arrow_forwardExamples: Solve the following differential equation using Laplace transform (e) ty"-ty+y=0 with y(0) = 0, and y'(0) = 1arrow_forwardExamples: Solve the following differential equation using Laplace transform (a) y" +2y+y=t with y(0) = 0, and y'(0) = 1arrow_forward
- Temperature for Sudbury (degrees Celsius) 3. The following table gives the mean monthly temperatures for Sudbury, Ontario and Windsor, Ontario. Each month is represented by the day of the year in the middle of the month. Month Day of Year Temperature for Windsor (degrees Celsius) January 15 -13.7 -4.7 February 45 -11.9 -3.8 March 75 -5.9 2.3 April 106 3.0 8.7 May 136 10.6 14.6 June 167 15.8 20.2 July 197 18.9 22.6 August 228 17.4 22.0 September 259 12.2 17.9 October 289 6.2 11.5 November 320 -1.2 4.8 December 350 -10.1 -1.2 a) Create a scatter plot of temperature vs. day of the year for each city. b) Draw the curve of best fit for each graph. c) Use your graphs to estimate when the temperature increases fastest, for each set of temperature data. Explain how you determined these values. d) Use your graphs to estimate the rate at which the temperature is increasing at the two times from question 3. e) Determine an equation of a sinusoidal function to model the data for each cityarrow_forwardNot use ai pleasearrow_forwardπ 25. If lies in the interval <0arrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_ios
 Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage, Trigonometry (MindTap Course List)TrigonometryISBN:9781305652224Author:Charles P. McKeague, Mark D. TurnerPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781305652224Author:Charles P. McKeague, Mark D. TurnerPublisher:Cengage Learning Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning
Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning, College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning





