College Physics: Explore And Apply, Volume 2 (2nd Edition)

2nd Edition

ISBN: 9780134862910

Author: Eugenia Etkina, Gorazd Planinsic, Alan Van Heuvelen, Gorzad Planinsic

Publisher: PEARSON

See similar textbooks

Concept explainers

Newton’s Third Law of Motion

The horse pulls the cart and the cart pulls the horse in response as per Newton third law. So the big question is, “Don’t the two forces cancel each other? How does the cart accelerate?”

Videos

Textbook Question

Chapter 5, Problem 19CQ

Two identical cars are moving with equal speeds on a wet road toward a turn, as shown in Figure Q5.19. Which car is more likely to slide off the road during the turn and why, assuming their speeds remain unchanged?

a. The two cars are equally likely to slide off the road because their masses are equal.

b. The two cars are equally likely to slide off the road because their speeds are equal.

c. Car B because it is closer to the outer side of the turn

d. Car B because the distance it travels around the turn is larger

e. Car A because it moves through the turn with a smaller radius

f. Car A because the distance it travels around the turn is smaller

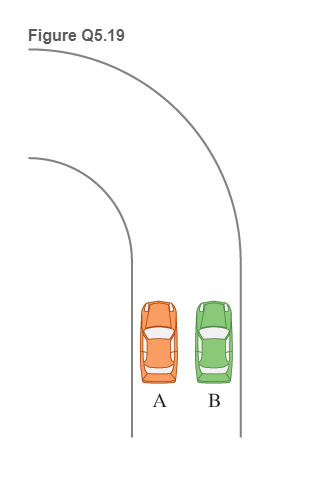
Chapter 5, Problem 19CQ, 19. Two identical cars are moving with equal speeds on a wet road toward a turn, as shown in Figure

Expert Solution & Answer

Want to see the full answer?

Check out a sample textbook solution

See solution

Chapter 5, Problem 18CQ

Chapter 5, Problem 20CQ

Students have asked these similar questions

I do not understand the process to answer the second part of question b. Please help me understand how to get there!

Rank the six combinations of electric charges on the basis of the electric force acting on 91. Define forces pointing to the right as positive and forces pointing to the left as negative. Rank in increasing order by placing the most negative on the left and the most positive on the right. To rank items as equivalent, overlap them. ▸ View Available Hint(s) [most negative 91 = +1nC 92 = +1nC 91 = -1nC 93 = +1nC 92- +1nC 93 = +1nC -1nC 92- -1nC 93- -1nC 91= +1nC 92 = +1nC 93=-1nC 91 +1nC 92=-1nC 93=-1nC 91 = +1nC 2 = −1nC 93 = +1nC The correct ranking cannot be determined. Reset Help most positive

Part A Find the x-component of the electric field at the origin, point O. Express your answer in newtons per coulomb to three significant figures, keeping in mind that an x component that points to the right is positive. ▸ View Available Hint(s) Eoz = Η ΑΣΦ ? N/C Submit Part B Now, assume that charge q2 is negative; q2 = -6 nC, as shown in (Figure 2). What is the x-component of the net electric field at the origin, point O? Express your answer in newtons per coulomb to three significant figures, keeping in mind that an x component that points to the right is positive. ▸ View Available Hint(s) Eoz= Η ΑΣΦ ? N/C

Chapter 5 Solutions

College Physics: Explore And Apply, Volume 2 (2nd Edition)

Ch. 5 - Review Question 5.1 How do we know that the sum of...Ch. 5 - Review Question 5.2 Why is it true that when an...Ch. 5 - Review Question 5.3 Show that the two expressions...Ch. 5 - Review Question 5.4 Think back to Example 5.5 ...Ch. 5 - Review Question 5.5 A friend says he has heard...Ch. 5 - Which of the objects below is accelerating? Object...Ch. 5 - The circle in Figure Q5.2 represents the path...Ch. 5 - One of your classmates drew a force diagram for a...Ch. 5 - Why is it difficult for a high-speed car to...Ch. 5 - How does a person standing on the ground explain...

Ch. 5 - 6. A pilot performs a vertical loop-the-loop at...Ch. 5 - 7. Why is the following an inaccurate statement...Ch. 5 - 8 Two point-like objects P and Q are undergoing...Ch. 5 - Compare the magnitude of the normal force of a car...Ch. 5 - If you put a penny on the center of a rotating...Ch. 5 - Where on Earths surface would you expect to...Ch. 5 - 12. What observational data might Newton have used...Ch. 5 - What observations combined with his second and...Ch. 5 - What would happen to the force exerted by the Sun...Ch. 5 - James fixes a camera on a tripod and takes several...Ch. 5 - Your friend says that an object weighs less on...Ch. 5 - Your friend says that when an object is moving in...Ch. 5 - Describe three everyday phenomena that are...Ch. 5 - 19. Two identical cars are moving with equal...Ch. 5 - 20. Astronauts on the space station orbiting Earth...Ch. 5 - 21. In the movies you often see space stations...Ch. 5 - 22. Give one example of a situation in which an...Ch. 5 - Name a planet on which you would weigh less than...Ch. 5 - A motorized cart is moving at a constant speed...Ch. 5 - 1. Mountain biker While mountain biking, you first...Ch. 5 - * You swing a rock tied to a string in a vertical...Ch. 5 - * Loop-the-loop You ride a roller coaster with a...Ch. 5 - 4. You start an old record player and notice a bug...Ch. 5 - 5. Determine the acceleration of Earth due to its...Ch. 5 - The Moon is an average distance of 3.8108 m from...Ch. 5 - Aborted plane landing You are on an airplane that...Ch. 5 - BIO Ultracentrifuge You are working in a biology...Ch. 5 - 9. * EST A tire-pressure monitoring system warns...Ch. 5 - Imagine that you are standing on a horizontal...Ch. 5 - 11. * Rolling is a combination of linear and...Ch. 5 - 14. * Consider the scenario described in Problem...Ch. 5 - 15. * You want to determine the radial...Ch. 5 - 16. Ferris wheel You are sitting on a rotating...Ch. 5 - 17. * EST Estimate the radial acceleration of the...Ch. 5 - * EST Estimate the radial acceleration of the toe...Ch. 5 - 19. * Is it safe to drive your 1600-kg car at...Ch. 5 - 20. * You are fixing a broken rotary lawn mower....Ch. 5 - * Your car speeds around the 80-m-radius curved...Ch. 5 - How fast do you need to swing a 200-g ball at the...Ch. 5 - 23. ** A small ball is attached by a string to a...Ch. 5 - A coin rests on a record 0.15 m from its center....Ch. 5 - 25. * Roller coaster ride A roller coaster car...Ch. 5 - * A person sitting in a chair (combined mass 80...Ch. 5 - 27. * A car moves around a 50-m-radius highway...Ch. 5 - 28. * A 20.0-g ball is attached to a 120-cm-long...Ch. 5 - 29. A 50-kg ice skater goes around a circle of...Ch. 5 - * A car traveling at 10 m/s passes over a hill on...Ch. 5 - 31. A 1000-kg car is moving at 30 m/s around a...Ch. 5 - * Equation Jeopardy 1 Describe using words, a...Ch. 5 - ** Banked curve raceway design You need to design...Ch. 5 - * A circular track is in a horizontal plane, has a...Ch. 5 - 36. ** Design a quantitative test for Newton’s...Ch. 5 - 37. * Your friend says that the force that the Sun...Ch. 5 - Determine the gravitational force that (a) the Sun...Ch. 5 - 39. * (a) What is the ratio of the gravitational...Ch. 5 - 40. ** EST Estimate (a) the average distance...Ch. 5 - 41. * EST The average radius of Earth s orbit...Ch. 5 - * The Moon travels in a 3.8105-km-radius orbit...Ch. 5 - 43. * Determine the ratio of Earth’s gravitational...Ch. 5 - 44. * Determine the magnitude of the gravitational...Ch. 5 - 45. * When you stand on a bathroom scale here on...Ch. 5 - 46. The free-fall acceleration on the surface of...Ch. 5 - 47.* A satellite moves in a circular orbit a...Ch. 5 - 48. * Mars has a mass of kg and a radius of m....Ch. 5 - 49. * Determine the speed a projectile must reach...Ch. 5 - 50. ** Determine the distance above Earth’s...Ch. 5 - 51. *Determine the period of an Earth satellite...Ch. 5 - 52. * A spaceship in outer space has a doughnut...Ch. 5 - 53. * Using the velocity change method from...Ch. 5 - 54. * Loop-the-loop You have to design a...Ch. 5 - ** A Tarzan swing Tarzan (mass 80 kg) swings at...Ch. 5 - 56. * (a) If the masses of Earth and the Moon were...Ch. 5 - 57. * EST Estimate the radial acceleration of the...Ch. 5 - 58. ** EST Estimate the force exerted by the tire...Ch. 5 - 59. ** EST Estimate the maximum radial force that...Ch. 5 - 60. * EST Estimate the force exerted by the wheel...Ch. 5 - Lucia's bathroom scale on the equator reads 110 lb...Ch. 5 - ** Demolition An old building is being demolished...Ch. 5 - 65. Designing a banked roadway You need to design...Ch. 5 - * Evaluation question You find the following in a...Ch. 5 - 67. * Suppose that Earth rotated much faster on...Ch. 5 - 68. * On Earth, an average person’s vertical jump...Ch. 5 - 69. * You read in a science magazine that on the...Ch. 5 - 70. * Determining the forces between powders and...Ch. 5 - ** Isabel notices that if she places a small...Ch. 5 - Texas Motor Speedway On October 28, 2000 Gil de...Ch. 5 - Texas Motor Speedway On October 28, 2000 Gil de...Ch. 5 - Texas Motor Speedway On October 28, 2000 Gil de...Ch. 5 - Texas Motor Speedway On October 28, 2000 Gil de...Ch. 5 - Texas Motor Speedway On October 28, 2000 Gil de...Ch. 5 - Halley's Comet Edmond Halley was the first to...Ch. 5 - Halley's Comet Edmond Halley was the first to...Ch. 5 - Halley's Comet Edmond Halley was the first to...Ch. 5 - Halley's Comet Edmond Halley was the first to...Ch. 5 - Halley's Comet Edmond Halley was the first to...Ch. 5 - Halley's Comet Edmond Halley was the first to...

Additional Science Textbook Solutions

Find more solutions based on key concepts

7. Both Tim and Jan (problem 6) have a widow’s peak (see Module 9.8), but Mike has a straight hairline. What ar...

Campbell Biology: Concepts & Connections (9th Edition)

12. FIGURE Q7.12 shows two masses at rest. The string is massless and the pullies are frictionless. The spring ...

Physics for Scientists and Engineers: A Strategic Approach, Vol. 1 (Chs 1-21) (4th Edition)

What are four functions of connective tissue?

Anatomy & Physiology (6th Edition)

Using the South Atlantic as an example, label the beginning of the normal polarity period C that began 2 millio...

Applications and Investigations in Earth Science (9th Edition)

Explain the role of gene flow in the biological species concept.

Campbell Biology (11th Edition)

1. What are the main organs of the skeletal system?

Human Anatomy & Physiology (2nd Edition)

Knowledge Booster

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.