The Heart of Mathematics: An Invitation to Effective Thinking
4th Edition
ISBN: 9781118156599
Author: Edward B. Burger, Michael Starbird
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 4.6, Problem 9MS
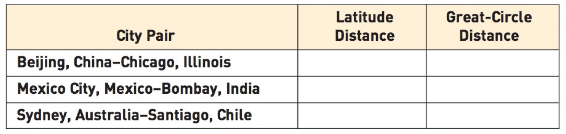
Latitude losers (H). In each of the following three Mindscapes, you are given a pair of cities that are on the same latitude. Fill in the table by measuring the distance from city to city, first staying along the latitude and then measuring the distance taking the great-circle route.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Can you Identify the picture
Darnell and Jake are playing a game they made up which includes throwing darts at this target.
The point values for each region of the target are shown. The radius of the 50-point region is 3 inches. The width of each of the other regions is 4 inches.
5 concentric circles where each circle contains values from 10, 20, 30, 40 and 50.
Darnell and Jake are currently tied in their game, and Jake has to choose one of these options:
Option 1: He can accept the tie, and the game is over.
Option 2: He can make one more throw. Jake wins if he earns at least 30 points on the throw, but he loses if he earns less than 30 points.
Complete each part of this task to determine which option Jake should choose.
Part A
Describe the process you could use to find the probability that Jake will earn at least 30 points on a throw, given that he hits the target? Note: Assume that it is equally likely that he will hit any region in the target.
You organized a charity stream to help out those in need. Two generous people
donated money through the stream. The total money donated over a certain duration
of streaming is shown below. Circle) the correct equation for each donation.
2
Time
Donation ($)
Time
Donation ($)
HELPING HAND
BY
(in min)
(in min)
GAMERS
10
120
10
25
30
300
30
225
50
480
50
625
DONATE NOW
y = 9x + 30
у 3 5x - 25
y = %
x2
y = | 2
Chapter 4 Solutions
The Heart of Mathematics: An Invitation to Effective Thinking
Ch. 4.1 - The main event. State the Pythagorean Theorem.Ch. 4.1 - Two out of three. If a right triangle has legs of...Ch. 4.1 - Hypotenuse hype. If a right triangle has legs of...Ch. 4.1 - Assesing area. Suppose you know the base of a...Ch. 4.1 - Squares all around. How does the figure below...Ch. 4.1 - Operating on the triangle. Using a straightedge,...Ch. 4.1 - Excite your friends about right triangles....Ch. 4.1 - Easy as 1,2,3? Can there be a right triangle with...Ch. 4.1 - Sky high (S). On a sunny, warm day, a student...Ch. 4.1 - Sand masting (H). The sailboat named Sand Bug has...
Ch. 4.1 - Getting a pole on a bus. For his 13th birthday,...Ch. 4.1 - The Scarecrow (ExH). In the 1939 movie The Wizard...Ch. 4.1 - Rooting through a spiral. Start with a right...Ch. 4.1 - Is it right? (H) Suppose someone tells you that...Ch. 4.1 - Tfrain trouble (H). Train tracks are made of...Ch. 4.1 - Does everyone have what it takes to be a triangle?...Ch. 4.1 - Getting squared away. In our proof of the...Ch. 4.1 - The practical side of Pythagoras. Suppose you are...Ch. 4.1 - Pythagorean pizzas (H). You have a choice at the...Ch. 4.1 - Natural right (S). Suppose r and s are any two...Ch. 4.1 - Well-rounded shapes. Suppose we have two circles...Ch. 4.1 - A Pythagorean Theorem for triangles other than...Ch. 4.1 - With a group of folks. In a small group, discuss...Ch. 4.1 - Double trouble. Suppose you know a right triangle...Ch. 4.1 - K-ple trouble. Suppose you have a right triangle...Ch. 4.1 - Padding around. You have a rectangular patio with...Ch. 4.1 - Pythagoras goes the distance. Plot the points (5,...Ch. 4.1 - Ahoy there! (H) Your exotic sailboat, which you...Ch. 4.2 - Standing guard. Draw the floor plan of a gallery...Ch. 4.2 - Art appreciation. State the Art Gallery Theorem.Ch. 4.2 - Upping the ante. How many guards do you need for a...Ch. 4.2 - Keep it safe. At what vertices would you place...Ch. 4.2 - Puttoing guards in their place. For each floor...Ch. 4.2 - Guarding the Guggenheim. The Art Gallery Theorem...Ch. 4.2 - TriangulatIng the Louvre (H). Triangulate the...Ch. 4.2 - Triangulating the Clark. Triangulate the floor...Ch. 4.2 - Tricolor me (ExH). For each triangulation, color...Ch. 4.2 - Tricolor hue. For each triangulation, color the...Ch. 4.2 - One-third. Write the number 6 as a sum of three...Ch. 4.2 - Easy watch. Draw a floor plan of a museum with six...Ch. 4.2 - Two watches (S). Draw the floor plan of a museum...Ch. 4.2 - Mirror, mirror on the wall. Consider the floor...Ch. 4.2 - Nine needs three (H). Draw a floor plan for a...Ch. 4.2 - One-third again (ExH). If a natural number is...Ch. 4.2 - Square museum (S). If a museum has only...Ch. 4.2 - Worst squares (H). Draw examples of museums with...Ch. 4.2 - Pie are squared. The circumference of a circle of...Ch. 4.2 - I can see the light. Suppose you are in a...Ch. 4.2 - Less than. Youve tnangulated your polygon and...Ch. 4.2 - Greater than. Youve triangulated your polygon and...Ch. 4.2 - Counting the colors. Your polygon has 40 vertices....Ch. 4.2 - Only red. Twelve of your polygons vertices have...Ch. 4.2 - Totaling triangles. If a polygon has n sides, it...Ch. 4.3 - Defining gold. Explain what makes a rectangle a...Ch. 4.3 - Approximating gold. Which of these numbers is...Ch. 4.3 - Approximating again. Which of the following...Ch. 4.3 - Same solution. Why does the equation l1=1l have...Ch. 4.3 - X marks the unkonw (ExH). Solve eachh equation for...Ch. 4.3 - A cold tall one? Can a Golden Rectangle have a...Ch. 4.3 - Fold the gold (H). Suppose you have a Golden...Ch. 4.3 - Sheets of gold. Suppose you have two sheets of...Ch. 4.3 - Circular logic? (H). Take a Golden Rectangle and...Ch. 4.3 - Growing gold (H). Take a Golden Rectangle and...Ch. 4.3 - Counterfeit gold? Draw a rectangle with its longer...Ch. 4.3 - In the grid (S). Consider the 1010 grid at left....Ch. 4.3 - A nest of gold. Consider the figure of infinitely...Ch. 4.3 - Comparing areas (ExH). Let G be a Golden Rectangle...Ch. 4.3 - Do we get gold? Lets make a rectangle somewhat...Ch. 4.3 - Do we get gold this time? (S) We now describe...Ch. 4.3 - A silver lining? (H) Consider the diagonal in the...Ch. 4.3 - Prob. 20MSCh. 4.3 - Going platinum. Determine the dimensions of a...Ch. 4.3 - Golden triangles. Draw a right triangle with one...Ch. 4.3 - Prob. 23MSCh. 4.3 - Prob. 24MSCh. 4.3 - Prob. 25MSCh. 4.3 - Power beyond the mathematics. Provide several...Ch. 4.3 - Special K. As a student at the University of...Ch. 4.3 - Special x. Find all values of x satisfying the...Ch. 4.3 - In search of x. Solve each equation for x:...Ch. 4.3 - Adding a square. Your school Healthy Eating garden...Ch. 4.3 - Golden Pythagoras (H). If you have a Golden...Ch. 4.4 - To tile or not to tile. Which of the following...Ch. 4.4 - Shifting Into symmetry. Shown below are small...Ch. 4.4 - Prob. 3MSCh. 4.4 - Prob. 4MSCh. 4.4 - Symmetric scaling (ExH). Each of the two patterns...Ch. 4.4 - Build a super. Draw a 1,2,5 right triangle in the...Ch. 4.4 - Another angle. Look at the 5-unit super-tile you...Ch. 4.4 - Super-super. Surround your 5-unit super-tile with...Ch. 4.4 - Expand forever (H). If you continue the process of...Ch. 4.4 - Prob. 10MSCh. 4.4 - Expand again. Take your 4.unit equilateral...Ch. 4.4 - One-answer supers. Here is a Pinwheel Pattern. For...Ch. 4.4 - Prob. 14MSCh. 4.4 - Many answer supers (H). Shown here are pictures of...Ch. 4.4 - Fill er up? (ExH) For each tile below, could...Ch. 4.4 - Prob. 18MSCh. 4.4 - Prob. 19MSCh. 4.4 - Prob. 20MSCh. 4.4 - Penrose tiles. Roger Penrose constructed two tiles...Ch. 4.4 - Expand forever. Why does any shape that can be...Ch. 4.4 - Super total. Recall that the Pinwheel Triangle has...Ch. 4.4 - Prob. 26MSCh. 4.4 - XY-tiles. The trapezoidal tile on the left has one...Ch. 4.4 - School spirit. Your dorm bathroom is tiled using...Ch. 4.4 - T-total (H). Suppose you start with one small...Ch. 4.5 - Its nice to be regular. What makes a polygon a...Ch. 4.5 - Keeping it Platonic. What makes a solid a regular...Ch. 4.5 - Countem up. How many faces, edges, and vertices...Ch. 4.5 - Defending duality. Explain why the cube and the...Ch. 4.5 - The eye of the beholder. Suppose you have models...Ch. 4.5 - Drawing solids. Draw each solid by completing the...Ch. 4.5 - Count. For each of the regular solids, take the...Ch. 4.5 - Soccer counts (ExH). Look at a soccer ball. Take...Ch. 4.5 - A solid slice (S). For each regular solid, imagine...Ch. 4.5 - Siding on the cube. Suppose we start with the...Ch. 4.5 - Cube slices (H). Consider slicing the cube with a...Ch. 4.5 - Dual quads (S). Suppose you have a cube with edges...Ch. 4.5 - Super dual. Suppose you take a cube with edges of...Ch. 4.5 - Self-duals. Suppose you have a tetrahedron having...Ch. 4.5 - Not quite regular (ExH). Suppose you allow...Ch. 4.5 - Truncated solids. Slice off all the vertices of...Ch. 4.5 - Stellated solids. Take each regular solid and...Ch. 4.5 - Prob. 24MSCh. 4.5 - Here we celeb rate the power of algebra as a...Ch. 4.5 - Here we celeb rate the power of algebra as a...Ch. 4.5 - Here we celeb rate the power of algebra as a...Ch. 4.5 - Here we celeb rate the power of algebra as a...Ch. 4.5 - Here we celeb rate the power of algebra as a...Ch. 4.6 - Walkind the walk. Here are three walks from corner...Ch. 4.6 - Missing angle in action. The triangles below are...Ch. 4.6 - Slippery X. A triangle is drawn on a sphere. Can...Ch. 4.6 - A triangular trio. The sphere below has three...Ch. 4.6 - Saddle sores. The triangle at right is drawn on a...Ch. 4.6 - Travel agent. In each of the following three...Ch. 4.6 - Travel agent. In each of the following three...Ch. 4.6 - Travel agent. In each of the following three...Ch. 4.6 - Latitude losers (H). In each of the following...Ch. 4.6 - Latitude losers (H). In each of the following...Ch. 4.6 - Latitude losers (H). In each of the following...Ch. 4.6 - Spider and bug. For each pair of points on the...Ch. 4.6 - Spider and bug. For each pair of points on the...Ch. 4.6 - Spider and bug. For each pair of points on the...Ch. 4.6 - Spider and bug. For each pair of points on the...Ch. 4.6 - Spider and bug. For each pair of points on the...Ch. 4.6 - Big angles (H). What is the largest value we can...Ch. 4.6 - Many angles (S). Draw three different great...Ch. 4.6 - Quads in a plane. Measure the sum of the angles of...Ch. 4.6 - Quads on the sphere. Below are quadrilaterals on...Ch. 4.6 - Parallel lines (ExH). On a plane, if you draw a...Ch. 4.6 - Cubical spheres (ExH). Take a cube. Put a point in...Ch. 4.6 - Tetrahedral spheres. Lets do a similar calculation...Ch. 4.6 - Dodecahedral spheres. This Mindscape is the same...Ch. 4.6 - Total excess. Using the observations from the...Ch. 4.6 - What is the sum of the three angles? Why? Consider...Ch. 4.6 - What is the sum of the angles of your triangle? Is...Ch. 4.6 - Removing a slice of the pie. Complete the...Ch. 4.6 - Conjuring up a conjecture. Make a conjecture about...Ch. 4.6 - Tetrahedral angles. What is the sum of the angles...Ch. 4.6 - Here we celebrate the power of algebra as a...Ch. 4.6 - Here we celebrate the power of algebra as a...Ch. 4.6 - Here we celebrate the power of algebra as a...Ch. 4.6 - Here we celebrate the power of algebra as a...Ch. 4.6 - Here we celebrate the power of algebra as a...Ch. 4.7 - At one with the univers. Below is a sketch of a...Ch. 4.7 - Are we there yet? Why does the information x=4 not...Ch. 4.7 - Plain places. Plot the following points in the...Ch. 4.7 - Big stack. If you take a huge number of sheets of...Ch. 4.7 - A bigger stack. If you take a huge number of...Ch. 4.7 - On the level in two dimensions. Pictured in the...Ch. 4.7 - On the level in two dimensions (S). Pictured in...Ch. 4.7 - On the level in four dimensions. Pictured in the...Ch. 4.7 - Tearible 2s. In the pictures below, describe how...Ch. 4.7 - Dare not to tear? For the figures in the Tearible...Ch. 4.7 - Unlinking (H). Using the fourth dimension,...Ch. 4.7 - Unknotting. Describe how you would unknot the...Ch. 4.7 - Prob. 13MSCh. 4.7 - Edgy hypercubes (H). Produce drawings of the...Ch. 4.7 - Prob. 15MSCh. 4.7 - Prob. 16MSCh. 4.7 - Doughnuts in dimensions. Suppose we have a...Ch. 4.7 - Assembly required (S). As promised in the...Ch. 4.7 - Slicing the cube. Take a 3-dimensional cube...Ch. 4.7 - Here we celebrate the power of algebra as a...Ch. 4.7 - Here we celebrate the power of algebra as a...Ch. 4.7 - Here we celebrate the power of algebra as a...Ch. 4.7 - Here we celebrate the power of algebra as a...Ch. 4.7 - Here we celebrate the power of algebra as a...
Additional Math Textbook Solutions
Find more solutions based on key concepts
In Problems 1 and 2, verify that the pair x(t), y(t) is a solution to the given system. Sketch the trajectory o...
Fundamentals of Differential Equations and Boundary Value Problems
a. The number of cube in the stack and number of face that are glued together.
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
In Exercises 1–4, fill in the blank with an appropriate word, phrase, or symbol(s).
1. The number of regions c...
A Survey of Mathematics with Applications (10th Edition) - Standalone book
Checkpoint1
Use the substitution method to solve this system:
Answers to Checkpoint exercises are found at the...
Mathematics with Applications In the Management, Natural, and Social Sciences (12th Edition)
Percentiles. The pth percentile of a sorted data set is a number xp such that p of the data fall at or below xp...
Excursions in Modern Mathematics (9th Edition)
In Exercise 1-10, assume that p is true, q is false, and r is true. Compute the truth value for each expression...
Mathematics All Around (6th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.Similar questions
- The figure shows the intersections of three one-way streets. To keep traffic moving, the number of cars per minute entering an intersection must equal the number exiting that intersection. Write an equation for intersection 1₂ that keeps traffic moving. ... (Type an equation.) 7 Cars/min 14 Cars/min 4 6 Cars/min CINE 10 Cars/min 9 Cars/min 8 Cars/min Write an equation for intersection 1₂ that keeps traffic moving. Make sure to use the same variables as in the figure.arrow_forwardRunning Shoes. A shoe designer tests a new material for the soles of their most popular line of running shoes. She has 6 athletes run 100 miles with one of last years shoes and one of these new shoes, randomly assigning the new shoe to the left or right foot. Afterward, she measures the amount of wear on each of the shoes in millimeters. Test the claim that the amount of wear with the new material is different from the amount of wear with the old material. Runner 4 1 2 3 1.14 1.04 1.08 1.09 New Material Wear Old Material Wear 1.03 0.87 0.96 1.01 (b) Write the hypotheses in symbols. Ho: Hdiff-new-old = 0 Ha: Hdiff-new-old > 0 O Ho: Pdiff-new-old (a) Why are these paired data? O The top and bottom values in each column are from the same runner. Runners typically run in groups of two. There are an equal number of shoes of each type. This table does not have paired data; there is no clear relationship between the top and bottom values in any column. Each runner has a pair of shoes. -0 Ha:…arrow_forwardGraph each set of points. Which model is most appropriate for each set? (-2, -3), (-1, 0), (0, 1), (1, 0), (2, -3)arrow_forward
- a and b are two points in the third dimension. Write an equation that describes the set of points that are equidistant to a and b. Draw a picture of this set in the case where a = i and b = j.arrow_forwardDirections: For each question, be sure to answer each questions in as much detail as possible in the spaces provided. 1. The city of Petersburg has 12,500 registered voters. There are two candidates for city council in an upcoming election: Brown and Stephens. The day before the election, a telephone poll of 500 randomly selected registered voters was conducted. 207 said they vote for Brown, 249 said they’d vote for Stephens, and 45 was undecided. Describe the population the surveyors are really interested in. Give the sample statistic for the proportion of voters surveyed who said they would vote for Brown. The proportion should be a fraction or decimal, not a percent.arrow_forwardPage of 11 ZOOM + 5. The table shows how the cost of a carne asada taco at my favorite taco stand has increased as they have become more popular since their opening in 2013. Use the data to answer the questions below. Year, x 2013, 0 2014, 1 2015, 2 2016, 3 2017, 4 2018, 5 2019,6 Cost ($) 0.50 0.55 0.65 0.75 0.90 1.00 1.10 (a) What is the regression line given by your TI-84 for this data? Round values to 3 decimal places. (b) Using the regression equation above, predict the cost of a carne asada taco at my favorite taco stand in 2020. Show the work.arrow_forward
- Write an ordered pair for each point described. Point C is four units to the right of the yaxis and seven units below the xaxis.arrow_forwardTwo scatterplots are shown below. Scatterplot 1 A scatterplot has 14 points. The horizontal axis is labeled "x" and has values from 30 to 110. The vertical axis is labeled "y" and has values from 30 to 110. The points are plotted from approximately (55, 60) up and right to approximately (95, 85). The points are somewhat scattered. Scatterplot 2 A scatterplot has 10 points. The horizontal axis is labeled "x" and has values from 30 to 110. The vertical axis is labeled "y" and has values from 30 to 110. The points are plotted from approximately (55, 55) steeply up and right to approximately (70, 90), and then steeply down and right to approximately (85, 60). The points are somewhat scattered. Explain why it makes sense to use the least-squares line to summarize the relationship between x and y for one of these data sets but not the other. Scatterplot 1 seems to show a relationship between x and y, while Scatterplot 2 shows a relationship between the two variables. So it makes sense to use…arrow_forwardDirection: Determine the diagram which would best present the data in the situation below. Then draw the diagram in the space provided to present the data. Make sure to add a title and a legend in the diagram. Situation: According to literacy reports in the city of Gotham, youth literacy went up to 7% since 2012. Here is the data: In 2010, only 43% of the youth population was literate. In the next census at 2015, it went up by 2%. After five years, the literacy rate was at 47%. During the most recent survey on 2020, the rate is already at 50%.arrow_forward
- Question 7 II Pause Q Zoom Q Review I Finish Test TASK 2: Awards Dinner Tran is in charge of the school's Awards Dinner. She set up the multi-purpose room with a stage in front and round tables for parents, students, and family members to sit around for dinner Below is the lloor plan that she drew for the event. 0000 Question 7: Use t to stand for the number of tables and s to stand for the total number of seats. In the box below, write an equation to show the relationship between the total number of seats and the number of tables. ©2021 Illuminate Education TM, Inc. acerarrow_forwardsomeone please help!!arrow_forwardCATEGORY Question 2 The City Planner overlayed a map of the city on a coordinate plane and found the equation that models seven of the streets as follows: Main Street: y=x-5 North St: y=-x-5 Carbon St: y=x+20 School St: y=x+3 Reo Ave: y = -x +9 Church St: y=-x-26 5 Mercer Rd: y=x+1 Determine how each street listed relates to Main Street. Press on a road name in the answer bank, then press on the category that describes how it relates to Main Street. To remove an item, you can press on the box and the trash can icon to the right of the item you want to remove.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Holt Mcdougal Larson Pre-algebra: Student Edition...
Algebra
ISBN:9780547587776
Author:HOLT MCDOUGAL
Publisher:HOLT MCDOUGAL
Algebra: Structure And Method, Book 1
Algebra
ISBN:9780395977224
Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:McDougal Littell
Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill
2.1 Introduction to inequalities; Author: Oli Notes;https://www.youtube.com/watch?v=D6erN5YTlXE;License: Standard YouTube License, CC-BY
GCSE Maths - What are Inequalities? (Inequalities Part 1) #56; Author: Cognito;https://www.youtube.com/watch?v=e_tY6X5PwWw;License: Standard YouTube License, CC-BY
Introduction to Inequalities | Inequality Symbols | Testing Solutions for Inequalities; Author: Scam Squad Math;https://www.youtube.com/watch?v=paZSN7sV1R8;License: Standard YouTube License, CC-BY