High School Math 2015 Common Core Algebra 1 Student Edition Grade 8/9
15th Edition
ISBN: 9780133281149
Author: Prentice Hall
Publisher: Prentice Hall
expand_more
expand_more
format_list_bulleted
Videos
Question
Chapter 4.5, Problem 29PPE
a.
To determine
the maximum image width that can be projected.
a.
Expert Solution
Answer to Problem 29PPE
y = 1. 8x
Explanation of Solution
Given Information
A projector and a screen, of which the distance between is ’12 feet’ and the width of the image described as a condition that for obtaining the required distance we have to multiply the image width by 1.8.
Calculation
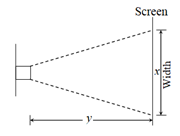
Let, the distance of projector to the screen be ‘ y’ and, the desired width of image be ‘ x’ .
Now, we will establish a relation between distance and width of image as a function of width.
Since, the manual clearly states that for requiring distance, we have to multiply the desired width by 1.8
So,
b.
To determine
that can the projector able to project the image
b.
Expert Solution
Answer to Problem 29PPE
not possible
Explanation of Solution
Given Information
A projector and a screen, of which the distance between is ’12 feet’ and the width of the image described as a condition that for obtaining the required distance we have to multiply the image width by 1.8.
Calculation:
For an image of 7 feet, we have to put the value of x = 7 feet in our established equation,
⇒
So, the distance comes out to be 12.6 feet.
But the maximum Length of hall is 12 feet.
Conclusion
So, it is not possible to project an image of 7 feet.
c.
To determine
that can the projector able to project the image
c.
Expert Solution
Answer to Problem 29PPE
6.6 feet
Explanation of Solution
Given Information
A projector and a screen, of which the distance between is ’12 feet’ and the width of the image described as a condition that for obtaining the required distance we have to multiply the image width by 1.8.
Calculation:
The maximum image width, which can be projected in room, shall be calculated as
⇒
We are given,
So,
Conclusion So, the maximum image width that can be obtained is 6.6 feet.
Chapter 4 Solutions
High School Math 2015 Common Core Algebra 1 Student Edition Grade 8/9
Ch. 4.1 - Prob. 1PCh. 4.1 - Prob. 2PCh. 4.1 - Prob. 3PCh. 4.1 - Prob. 1LCCh. 4.1 - Prob. 2LCCh. 4.1 - Prob. 3LCCh. 4.1 - Prob. 4LCCh. 4.1 - Prob. 5PPECh. 4.1 - Prob. 6PPECh. 4.1 - Prob. 7PPE
Ch. 4.1 - Prob. 8PPECh. 4.1 - Prob. 9PPECh. 4.1 - Prob. 10PPECh. 4.1 - Prob. 11PPECh. 4.1 - Prob. 12PPECh. 4.1 - Prob. 13PPECh. 4.1 - Prob. 14PPECh. 4.1 - Prob. 15PPECh. 4.1 - Prob. 16PPECh. 4.1 - Prob. 17PPECh. 4.1 - Prob. 18PPECh. 4.1 - Prob. 19PPECh. 4.1 - Prob. 20PPECh. 4.1 - Prob. 21STPCh. 4.1 - Prob. 22STPCh. 4.1 - Prob. 23STPCh. 4.1 - Prob. 24MRCh. 4.1 - Prob. 25MRCh. 4.1 - Prob. 26MRCh. 4.1 - Prob. 27MRCh. 4.1 - Prob. 28MRCh. 4.1 - Prob. 29MRCh. 4.2 - Prob. 1PCh. 4.2 - Prob. 2PCh. 4.2 - Prob. 1LCCh. 4.2 - Prob. 2LCCh. 4.2 - Prob. 3LCCh. 4.2 - Prob. 4LCCh. 4.2 - Prob. 5LCCh. 4.2 - Prob. 6PPECh. 4.2 - Prob. 7PPECh. 4.2 - Prob. 8PPECh. 4.2 - Prob. 9PPECh. 4.2 - Prob. 10PPECh. 4.2 - Prob. 11PPECh. 4.2 - Prob. 12PPECh. 4.2 - Prob. 13PPECh. 4.2 - Prob. 14PPECh. 4.2 - Prob. 15PPECh. 4.2 - Prob. 16PPECh. 4.2 - Prob. 17PPECh. 4.2 - Prob. 18PPECh. 4.2 - Prob. 19PPECh. 4.2 - Prob. 20PPECh. 4.2 - Prob. 1MPCh. 4.3 - Prob. 1PCh. 4.3 - Prob. 2PCh. 4.3 - Prob. 3PCh. 4.3 - Prob. 1LCCh. 4.3 - Prob. 2LCCh. 4.3 - Prob. 3LCCh. 4.3 - Prob. 4LCCh. 4.3 - Prob. 5LCCh. 4.3 - Prob. 6PPECh. 4.3 - Prob. 7PPECh. 4.3 - Prob. 8PPECh. 4.3 - Prob. 9PPECh. 4.3 - Prob. 10PPECh. 4.3 - Prob. 11PPECh. 4.3 - Prob. 12PPECh. 4.3 - Prob. 13PPECh. 4.3 - Prob. 14PPECh. 4.3 - Prob. 15PPECh. 4.3 - Prob. 16PPECh. 4.3 - Prob. 17PPECh. 4.3 - Prob. 18PPECh. 4.3 - Prob. 19PPECh. 4.3 - Prob. 20PPECh. 4.3 - Prob. 21PPECh. 4.3 - Prob. 22PPECh. 4.3 - Prob. 23STPCh. 4.3 - Prob. 24STPCh. 4.3 - Prob. 25STPCh. 4.3 - Prob. 26MRCh. 4.3 - Prob. 27MRCh. 4.3 - Prob. 28MRCh. 4.3 - Prob. 29MRCh. 4.3 - Prob. 1MCQCh. 4.3 - Prob. 2MCQCh. 4.3 - Prob. 3MCQCh. 4.3 - Prob. 4MCQCh. 4.3 - Prob. 5MCQCh. 4.3 - Prob. 6MCQCh. 4.3 - Prob. 7MCQCh. 4.3 - Prob. 8MCQCh. 4.3 - Prob. 9MCQCh. 4.3 - Prob. 10MCQCh. 4.3 - Prob. 11MCQCh. 4.4 - Prob. 1PCh. 4.4 - Prob. 2PCh. 4.4 - Prob. 3PCh. 4.4 - Prob. 4PCh. 4.4 - Prob. 1LCCh. 4.4 - Prob. 2LCCh. 4.4 - Prob. 3LCCh. 4.4 - Prob. 4LCCh. 4.4 - Prob. 5LCCh. 4.4 - Prob. 6LCCh. 4.4 - Prob. 7LCCh. 4.4 - Prob. 8LCCh. 4.4 - Prob. 9PPECh. 4.4 - Prob. 10PPECh. 4.4 - Prob. 11PPECh. 4.4 - Prob. 12PPECh. 4.4 - Prob. 13PPECh. 4.4 - Prob. 14PPECh. 4.4 - Prob. 15PPECh. 4.4 - Prob. 16PPECh. 4.4 - Prob. 17PPECh. 4.4 - Prob. 18PPECh. 4.4 - Prob. 19PPECh. 4.4 - Prob. 20PPECh. 4.4 - Prob. 21PPECh. 4.4 - Prob. 22PPECh. 4.4 - Prob. 23PPECh. 4.4 - Prob. 24PPECh. 4.4 - Prob. 25PPECh. 4.4 - Prob. 26PPECh. 4.4 - Prob. 27PPECh. 4.4 - Prob. 28PPECh. 4.4 - Prob. 29PPECh. 4.4 - Prob. 30PPECh. 4.4 - Prob. 31PPECh. 4.4 - Prob. 32PPECh. 4.4 - Prob. 33PPECh. 4.4 - Prob. 34PPECh. 4.4 - Prob. 35PPECh. 4.4 - Prob. 36PPECh. 4.4 - Prob. 37PPECh. 4.4 - Prob. 38PPECh. 4.4 - Prob. 39PPECh. 4.4 - Prob. 40PPECh. 4.4 - Prob. 41PPECh. 4.4 - Prob. 1MPCh. 4.4 - Prob. 1CBCh. 4.4 - Prob. 2CBCh. 4.4 - Prob. 3CBCh. 4.4 - Prob. 4CBCh. 4.4 - Prob. 5CBCh. 4.4 - Prob. 6CBCh. 4.4 - Prob. 7CBCh. 4.4 - Prob. 8CBCh. 4.4 - Prob. 9CBCh. 4.4 - Prob. 10CBCh. 4.4 - Prob. 11CBCh. 4.4 - Prob. 12CBCh. 4.4 - Prob. 13CBCh. 4.4 - Prob. 14CBCh. 4.5 - Prob. 1PCh. 4.5 - Prob. 2PCh. 4.5 - Prob. 3PCh. 4.5 - Prob. 1LCCh. 4.5 - Prob. 2LCCh. 4.5 - Prob. 3LCCh. 4.5 - Prob. 4LCCh. 4.5 - Prob. 5LCCh. 4.5 - Prob. 6LCCh. 4.5 - Prob. 7LCCh. 4.5 - Prob. 8PPECh. 4.5 - Prob. 9PPECh. 4.5 - Prob. 10PPECh. 4.5 - Prob. 11PPECh. 4.5 - Prob. 12PPECh. 4.5 - Prob. 13PPECh. 4.5 - Prob. 14PPECh. 4.5 - Prob. 15PPECh. 4.5 - Prob. 16PPECh. 4.5 - Prob. 17PPECh. 4.5 - Prob. 18PPECh. 4.5 - Prob. 19PPECh. 4.5 - Prob. 20PPECh. 4.5 - Prob. 21PPECh. 4.5 - Prob. 22PPECh. 4.5 - Prob. 23PPECh. 4.5 - Prob. 24PPECh. 4.5 - Prob. 25PPECh. 4.5 - Prob. 26PPECh. 4.5 - Prob. 27PPECh. 4.5 - Prob. 28PPECh. 4.5 - Prob. 29PPECh. 4.5 - Prob. 30PPECh. 4.5 - Prob. 31PPECh. 4.5 - Prob. 32PPECh. 4.5 - Prob. 33STPCh. 4.5 - Prob. 34STPCh. 4.5 - Prob. 35STPCh. 4.5 - Prob. 36STPCh. 4.5 - Prob. 37MRCh. 4.5 - Prob. 38MRCh. 4.5 - Prob. 39MRCh. 4.5 - Prob. 40MRCh. 4.5 - Prob. 41MRCh. 4.5 - Prob. 42MRCh. 4.5 - Prob. 43MRCh. 4.5 - Prob. 44MRCh. 4.5 - Prob. 45MRCh. 4.5 - Prob. 46MRCh. 4.5 - Prob. 47MRCh. 4.5 - Prob. 48MRCh. 4.5 - Prob. 49MRCh. 4.5 - Prob. 50MRCh. 4.5 - Prob. 51MRCh. 4.5 - Prob. 52MRCh. 4.5 - Prob. 53MRCh. 4.5 - Prob. 54MRCh. 4.5 - Prob. 55MRCh. 4.5 - Prob. 56MRCh. 4.6 - Prob. 1PCh. 4.6 - Prob. 2PCh. 4.6 - Prob. 3PCh. 4.6 - Prob. 4PCh. 4.6 - Prob. 5PCh. 4.6 - Prob. 1LCCh. 4.6 - Prob. 2LCCh. 4.6 - Prob. 3LCCh. 4.6 - Prob. 4LCCh. 4.6 - Prob. 5LCCh. 4.6 - Prob. 6LCCh. 4.6 - Prob. 7LCCh. 4.6 - Prob. 8PPECh. 4.6 - Prob. 9PPECh. 4.6 - Prob. 10PPECh. 4.6 - Prob. 11PPECh. 4.6 - Prob. 12PPECh. 4.6 - Prob. 13PPECh. 4.6 - Prob. 14PPECh. 4.6 - Prob. 15PPECh. 4.6 - Prob. 16PPECh. 4.6 - Prob. 17PPECh. 4.6 - Prob. 18PPECh. 4.6 - Prob. 19PPECh. 4.6 - Prob. 20PPECh. 4.6 - Prob. 21PPECh. 4.6 - Prob. 22PPECh. 4.6 - Prob. 23PPECh. 4.6 - Prob. 24PPECh. 4.6 - Prob. 25PPECh. 4.6 - Prob. 26PPECh. 4.6 - Prob. 27PPECh. 4.6 - Prob. 28PPECh. 4.6 - Prob. 29PPECh. 4.6 - Prob. 30PPECh. 4.6 - Prob. 31PPECh. 4.6 - Prob. 32PPECh. 4.6 - Prob. 33PPECh. 4.6 - Prob. 34PPECh. 4.6 - Prob. 35PPECh. 4.6 - Prob. 36PPECh. 4.6 - Prob. 37PPECh. 4.6 - Prob. 38PPECh. 4.6 - Prob. 39PPECh. 4.6 - Prob. 40PPECh. 4.6 - Prob. 41STPCh. 4.6 - Prob. 42STPCh. 4.6 - Prob. 43STPCh. 4.6 - Prob. 44STPCh. 4.6 - Prob. 45MRCh. 4.6 - Prob. 46MRCh. 4.6 - Prob. 47MRCh. 4.6 - Prob. 48MRCh. 4.6 - Prob. 49MRCh. 4.6 - Prob. 50MRCh. 4.7 - Prob. 1PCh. 4.7 - Prob. 2PCh. 4.7 - Prob. 3PCh. 4.7 - Prob. 4PCh. 4.7 - Prob. 5PCh. 4.7 - Prob. 6PCh. 4.7 - Prob. 1LCCh. 4.7 - Prob. 2LCCh. 4.7 - Prob. 3LCCh. 4.7 - Prob. 4LCCh. 4.7 - Prob. 5LCCh. 4.7 - Prob. 6LCCh. 4.7 - Prob. 7LCCh. 4.7 - Prob. 8LCCh. 4.7 - Prob. 9PPECh. 4.7 - Prob. 10PPECh. 4.7 - Prob. 11PPECh. 4.7 - Prob. 12PPECh. 4.7 - Prob. 13PPECh. 4.7 - Prob. 14PPECh. 4.7 - Prob. 15PPECh. 4.7 - Prob. 16PPECh. 4.7 - Prob. 17PPECh. 4.7 - Prob. 18PPECh. 4.7 - Prob. 19PPECh. 4.7 - Prob. 20PPECh. 4.7 - Prob. 21PPECh. 4.7 - Prob. 22PPECh. 4.7 - Prob. 23PPECh. 4.7 - Prob. 24PPECh. 4.7 - Prob. 25PPECh. 4.7 - Prob. 26PPECh. 4.7 - Prob. 27PPECh. 4.7 - Prob. 28PPECh. 4.7 - Prob. 29PPECh. 4.7 - Prob. 30PPECh. 4.7 - Prob. 31PPECh. 4.7 - Prob. 32PPECh. 4.7 - Prob. 33PPECh. 4.7 - Prob. 34PPECh. 4.7 - Prob. 35PPECh. 4.7 - Prob. 36PPECh. 4.7 - Prob. 37PPECh. 4.7 - Prob. 38PPECh. 4.7 - Prob. 39PPECh. 4.7 - Prob. 40PPECh. 4.7 - Prob. 41PPECh. 4.7 - Prob. 42PPECh. 4.7 - Prob. 43PPECh. 4.7 - Prob. 44PPECh. 4.7 - Prob. 45PPECh. 4.7 - Prob. 46PPECh. 4.7 - Prob. 47PPECh. 4.7 - Prob. 48PPECh. 4.7 - Prob. 49PPECh. 4.7 - Prob. 50PPECh. 4.7 - Prob. 51PPECh. 4.7 - Prob. 52PPECh. 4.7 - Prob. 53PPECh. 4.7 - Prob. 54PPECh. 4.7 - Prob. 55PPECh. 4.7 - Prob. 56PPECh. 4.7 - Prob. 57PPECh. 4.7 - Prob. 58PPECh. 4.7 - Prob. 59PPECh. 4.7 - Prob. 60PPECh. 4.7 - Prob. 61PPECh. 4.7 - Prob. 62PPECh. 4.7 - Prob. 63PPECh. 4.7 - Prob. 64PPECh. 4.7 - Prob. 65PPECh. 4.7 - Prob. 66PPECh. 4.7 - Prob. 67PPECh. 4.7 - Prob. 68PPECh. 4.7 - Prob. 69PPECh. 4.7 - Prob. 70PPECh. 4.7 - Prob. 71PPECh. 4.7 - Prob. 72PPECh. 4.7 - Prob. 73PPECh. 4.7 - Prob. 74PPECh. 4.7 - Prob. 75PPECh. 4.7 - Prob. 1MPCh. 4 - Prob. 1GRCh. 4 - Prob. 2GRCh. 4 - Prob. 3GRCh. 4 - Prob. 4GRCh. 4 - Prob. 5GRCh. 4 - Prob. 6GRCh. 4 - Prob. 7GRCh. 4 - Prob. 8GRCh. 4 - Prob. 9GRCh. 4 - Prob. 10GRCh. 4 - Prob. 11GRCh. 4 - Prob. 12GRCh. 4 - Prob. 13GRCh. 4 - Prob. 14GRCh. 4 - Prob. 15GRCh. 4 - Prob. 16GRCh. 4 - Prob. 17GRCh. 4 - Prob. 18GRCh. 4 - Prob. 19GRCh. 4 - Prob. 20GRCh. 4 - Prob. 21GRCh. 4 - Prob. 22GRCh. 4 - Prob. 1CRCh. 4 - Prob. 2CRCh. 4 - Prob. 3CRCh. 4 - Prob. 4CRCh. 4 - Prob. 5CRCh. 4 - Prob. 6CRCh. 4 - Prob. 7CRCh. 4 - Prob. 8CRCh. 4 - Prob. 9CRCh. 4 - Prob. 10CRCh. 4 - Prob. 11CRCh. 4 - Prob. 12CRCh. 4 - Prob. 13CRCh. 4 - Prob. 14CRCh. 4 - Prob. 15CRCh. 4 - Prob. 16CRCh. 4 - Prob. 17CRCh. 4 - Prob. 18CRCh. 4 - Prob. 19CRCh. 4 - Prob. 20CRCh. 4 - Prob. 21CRCh. 4 - Prob. 22CRCh. 4 - Prob. 23CRCh. 4 - Prob. 24CRCh. 4 - Prob. 25CRCh. 4 - Prob. 26CRCh. 4 - Prob. 27CRCh. 4 - Prob. 28CRCh. 4 - Prob. 29CRCh. 4 - Prob. 30CRCh. 4 - Prob. 1CTCh. 4 - Prob. 2CTCh. 4 - Prob. 3CTCh. 4 - Prob. 4CTCh. 4 - Prob. 5CTCh. 4 - Prob. 6CTCh. 4 - Prob. 7CTCh. 4 - Prob. 8CTCh. 4 - Prob. 9CTCh. 4 - Prob. 10CTCh. 4 - Prob. 11CTCh. 4 - Prob. 12CTCh. 4 - Prob. 13CTCh. 4 - Prob. 14CTCh. 4 - Prob. 15CTCh. 4 - Prob. 16CTCh. 4 - Prob. 17CTCh. 4 - Prob. 18CTCh. 4 - Prob. 19CTCh. 4 - Prob. 20CTCh. 4 - Prob. 1CCSRCh. 4 - Prob. 2CCSRCh. 4 - Prob. 3CCSRCh. 4 - Prob. 4CCSRCh. 4 - Prob. 5CCSRCh. 4 - Prob. 6CCSRCh. 4 - Prob. 7CCSRCh. 4 - Prob. 8CCSRCh. 4 - Prob. 9CCSRCh. 4 - Prob. 10CCSRCh. 4 - Prob. 11CCSRCh. 4 - Prob. 12CCSRCh. 4 - Prob. 13CCSRCh. 4 - Prob. 14CCSRCh. 4 - Prob. 15CCSRCh. 4 - Prob. 16CCSRCh. 4 - Prob. 17CCSRCh. 4 - Prob. 18CCSRCh. 4 - Prob. 19CCSRCh. 4 - Prob. 20CCSRCh. 4 - Prob. 21CCSRCh. 4 - Prob. 22CCSRCh. 4 - Prob. 23CCSRCh. 4 - Prob. 24CCSRCh. 4 - Prob. 25CCSRCh. 4 - Prob. 26CCSRCh. 4 - Prob. 27CCSR
Additional Math Textbook Solutions
Find more solutions based on key concepts
Explain the meaning of the term “statistically significant difference” in statistics terminology.
Intro Stats, Books a la Carte Edition (5th Edition)
1. combination of numbers, variables, and operation symbols is called an algebraic______.
Algebra and Trigonometry (6th Edition)
Whether the ‘Physicians Committee for Responsible Medicine’ has the potential to create a bias in a statistical...
Elementary Statistics
The following set of data is from sample of n=5: a. Compute the mean, median, and mode. b. Compute the range, v...
Basic Business Statistics, Student Value Edition
Fill in each blank so that the resulting statement is true. If n is a counting number, bn, read ______, indicat...
College Algebra (7th Edition)
Implicit differentiation Carry out the following steps. a. Use implicit differentiation to find dydx. b. Find t...
Calculus: Early Transcendentals (2nd Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, algebra and related others by exploring similar questions and additional content below.Similar questions
- 2) If Mand N be two water hyper Plane ofx Show that MUN and MN is hy Per Plane ofx with prove and Examplame. or 3) IS AUB is convex set and affine set or blensed set or symmetre setorsubsie.... Show that A and B is convex or affine or Hensedsed or symmetivce or subspace. 4) 18 MUN is independence show that Prove or ExPlane Mand Nave independend. or not. 5) Jet X be Vector Pace over I show that is xty tnx st Xty 3 fix→ F s-t f(x) (9) Jet Mand N be two blanced set of Xbe Vector space show tha MUNIS ansed setarrow_forwardFind a polynomial with integer coefficients that satisfies the given conditions. T(x) has degree 4, zeros i and 1 + i, and constant term 12.arrow_forwardHow to solve 2542000/64132 without a calculator?arrow_forward
- How much is the circumference of a circle whose diameter is 7 feet?C =π darrow_forwardHow to solve 2542/64.132arrow_forwardAssume that you fancy polynomial splines, while you actually need ƒ(t) = e²/3 – 1 for t€ [−1, 1]. See the figure for a plot of f(t). Your goal is to approximate f(t) with an inter- polating polynomial spline of degree d that is given as sa(t) = • Σk=0 Pd,k bd,k(t) so that sd(tk) = = Pd,k for tk = −1 + 2 (given d > 0) with basis functions bd,k(t) = Σi±0 Cd,k,i = • The special case of d 0 is trivial: the only basis function b0,0 (t) is constant 1 and so(t) is thus constant po,0 for all t = [−1, 1]. ...9 The d+1 basis functions bd,k (t) form a ba- sis Bd {ba,o(t), ba,1(t), bd,d(t)} of the function space of all possible sα (t) functions. Clearly, you wish to find out, which of them given a particular maximal degree d is the best-possible approximation of f(t) in the least- squares sense. _ 1 0.9 0.8 0.7 0.6 0.5 0.4 0.3 0.2 0.1 0 -0.1 -0.2 -0.3 -0.4 -0.5 -0.6 -0.7 -0.8 -0.9 -1 function f(t) = exp((2t)/3) - 1 to project -1 -0.9 -0.8 -0.7 -0.6 -0.5 -0.4 -0.3 -0.2 -0.1 0 0.1 0.2 0.3 0.4 0.5…arrow_forward
- An image processor considered a 750×750 pixels large subset of an image and converted it into gray-scale, resulting in matrix gIn - a false-color visualization of gIn is shown in the top-left below. He prepared a two-dim. box filter f1 as a 25×25 matrix with only the 5×5 values in the middle being non-zero – this filter is shown in the top-middle position below. He then convolved £1 with itself to get £2, before convolving £2 with itself to get f3. In both of the steps, he maintained the 25×25 size. Next, he convolved gIn with £3 to get gl. Which of the six panels below shows g1? Argue by explaining all the steps, so far: What did the image processor do when preparing ₤3? What image processing operation (from gin to g1) did he prepare and what's the effect that can be seen? Next, he convolved the rows of f3 with filter 1/2 (-1, 8, 0, -8, 1) to get f4 - you find a visualization of filter f 4 below. He then convolved gIn with f4 to get g2 and you can find the result shown below. What…arrow_forward3ur Colors are enchanting and elusive. A multitude of color systems has been proposed over a three-digits number of years - maybe more than the number of purposes that they serve... - Everyone knows the additive RGB color system – we usually serve light-emitting IT components like monitors with colors in that system. Here, we use c = (r, g, b) RGB with r, g, bЄ [0,1] to describe a color c. = T For printing, however, we usually use the subtractive CMY color system. The same color c becomes c = (c, m, y) CMY (1-c, 1-m, 1-y) RGB Note how we use subscripts to indicate with coordinate system the coordinates correspond to. Explain, why it is not possible to find a linear transformation between RGB and CMY coordinates. Farbenlehr c von Goethe Erster Band. Roſt einen Defte mit fergen up Tübingen, is et 3. Cotta'fden Babarblung. ISIO Homogeneous coordinates give us a work-around: If we specify colors in 4D, instead, with the 4th coordinate being the homogeneous coordinate h so that every actual…arrow_forwardCan someone provide an answer & detailed explanation please? Thank you kindly!arrow_forward
- Given the cubic function f(x) = x^3-6x^2 + 11x- 6, do the following: Plot the graph of the function. Find the critical points and determine whether each is a local minimum, local maximum, or a saddle point. Find the inflection point(s) (if any).Identify the intervals where the function is increasing and decreasing. Determine the end behavior of the graph.arrow_forwardGiven the quadratic function f(x) = x^2-4x+3, plot the graph of the function and find the following: The vertex of the parabola .The x-intercepts (if any). The y-intercept. Create graph also before solve.arrow_forwardwhat model best fits this dataarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSON
Contemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Algebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSON
Introduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge Press
College Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education
Algebra and Trigonometry (6th Edition)
Algebra
ISBN:9780134463216
Author:Robert F. Blitzer
Publisher:PEARSON
Contemporary Abstract Algebra
Algebra
ISBN:9781305657960
Author:Joseph Gallian
Publisher:Cengage Learning
Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning
Algebra And Trigonometry (11th Edition)
Algebra
ISBN:9780135163078
Author:Michael Sullivan
Publisher:PEARSON
Introduction to Linear Algebra, Fifth Edition
Algebra
ISBN:9780980232776
Author:Gilbert Strang
Publisher:Wellesley-Cambridge Press
College Algebra (Collegiate Math)
Algebra
ISBN:9780077836344
Author:Julie Miller, Donna Gerken
Publisher:McGraw-Hill Education
Derivatives of Trigonometric Functions - Product Rule Quotient & Chain Rule - Calculus Tutorial; Author: The Organic Chemistry Tutor;https://www.youtube.com/watch?v=_niP0JaOgHY;License: Standard YouTube License, CC-BY