Chapter 4.3, Problem 2E

Interpretation Introduction

Interpretation:

To solve the given equation for $\text{θ}$, where the given integral is $\text{T = }{\displaystyle \underset{\text{-π}}{\overset{\text{π}}{\int }}\frac{\text{dθ}}{\text{ω-asinθ}}}$. Show that $\text{sinθ = }\frac{\text{2u}}{{\text{1+u}}^{\text{2}}}$ by using right angle triangle. Express T as an integral with respect to u.

Concept Introduction:

Non-uniform oscillator is moving with time period T and angular frequency $\text{ω}$.

The period of oscillation can be found analytically, which is shown below.

Answer to Problem 2E

Solution:

The solution for equation $\text{T = }{\displaystyle \underset{\text{-π}}{\overset{\text{π}}{\int }}\frac{\text{dθ}}{\text{ω-asinθ}}}$ is $\text{dθ = }\frac{\text{2du}}{{\text{1+u}}^{\text{2}}}$.

$\text{sinθ = }\frac{\text{2u}}{{\text{1+u}}^{\text{2}}}$ is shown below.

It is proved that $\text{u}\to \pm \infty$ when $\text{as θ}\to \text{± π}$.

The expression for T in the form of u is shown below.

The period of oscillation for non-uniform oscillator is $\text{T = }\frac{\text{2π}}{\sqrt{{\text{ω}}^{\text{2}}{\text{-a}}^{\text{2}}}}$.

Explanation of Solution

The period of oscillation for the non-uniform oscillator is

$\text{T = }{\displaystyle \underset{\text{-π}}{\overset{\text{π}}{\int }}\frac{\text{dθ}}{\text{ω-asinθ}}}$

Here, $\omega$ is the angular frequency, and $\text{a}$ is the amplitude $\text{(ω > a > 0)}$.

The differentiation of ${\text{tan}}^{\text{-1}}\text{x}$ is

$\frac{\text{d}}{\text{dx}}{\text{(tan}}^{\text{-x}}\text{) = }\frac{\text{1}}{{\text{1+x}}^{\text{2}}}$

(a)

Solve the equation for $\text{θ}$, and express $\text{dθ}$ in terms of $\text{u}$ and $\text{du}$.

Consider the equation.

Rearrange the equation as

$\frac{\text{θ}}{\text{2}}{\text{ = tan}}^{\text{-1}}\text{ u}$

${\text{θ = 2tan}}^{\text{-1}}\text{u}$

Differentiate the above equation.

$\text{T = }{\displaystyle \underset{\text{-π}}{\overset{\text{π}}{\int }}\frac{\text{dθ}}{\text{ω-asinθ}}}\text{, sinθ = }\frac{\text{2u}}{{\text{1+u}}^{\text{2}}}\text{ and dθ = }\frac{\text{2du}}{{\text{1+u}}^{\text{2}}}$

Hence, the expression for $\text{dθ}$ in terms of $\text{u}$ and $\text{du}$ is $\text{dθ = }\frac{\text{2du}}{{\text{1+u}}^{\text{2}}}$.

(b)

Show that $\text{sinθ = }\frac{\text{2u}}{{\text{1+u}}^{\text{2}}}$

The figure below shows the right-angled triangle with base $\text{1}$ and height or perpendicular $\text{u}$:

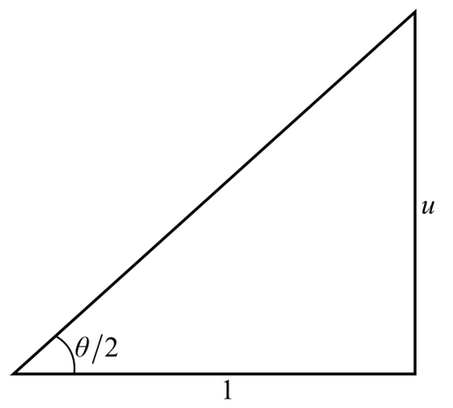
From the above triangle,

$\begin{array}{l}\text{cos}\frac{\text{θ}}{\text{2}}\text{ = }\frac{\text{base}}{\text{hypotenuse}}\\ \text{ = }\frac{\text{1}}{\sqrt{{\text{1+u}}^{\text{2}}}}\end{array}$

From the half-angle formula,

$\begin{array}{l}\text{sinθ = 2sin}\frac{\text{θ}}{\text{2}}\text{cos}\frac{\text{θ}}{\text{2}}\\ \text{ = 2}\left(\frac{\text{u}}{\sqrt{{\text{1+u}}^{\text{2}}}}\right)\left(\frac{\text{1}}{\sqrt{{\text{1+u}}^{\text{2}}}}\right)\\ \text{ = }\frac{\text{2u}}{{\text{1+u}}^{\text{2}}}\end{array}$

Hence, it is proved that $\text{sinθ = }\frac{\text{2u}}{{\text{1+u}}^{\text{2}}}$

(c)

Show that $\text{u}\to \pm \infty$ $\text{as θ}\to \text{± π}$.

Substitute $\text{π}$ for $\text{θ}$ in the equation $\text{u = tan}\frac{\text{θ}}{\text{2}}$.

$\begin{array}{l}\text{u = tan}\frac{\text{π}}{\text{2}}\\ \text{ = +}\infty \end{array}$

Substitute $\text{-π}$ for $\text{θ}$ in the equation $\text{u = tan}\frac{\text{θ}}{\text{2}}$.

$\text{u = tan}\frac{\text{-π}}{\text{2}}$

$\text{ = -tan}\frac{\text{π}}{\text{2}}$

$\text{= -}\infty$

Hence, it is proved that $\text{u}\to \pm \infty$ $\text{as θ}\to \text{± π}$.

(d)

Express T as an integral with respect to u.

Consider $\text{T = }{\displaystyle \underset{\text{-π}}{\overset{\text{π}}{\int }}\frac{\text{dθ}}{\text{ω-asinθ}}}\text{, sinθ = }\frac{\text{2u}}{{\text{1+u}}^{\text{2}}}\text{ and dθ = }\frac{\text{2du}}{{\text{1+u}}^{\text{2}}}$

$\text{θ}$	$\text{u = tan}\frac{\text{θ}}{\text{2}}$
$\text{-π}$	$\text{tan}\frac{\text{-π}}{\text{2}}=-\infty$
$\text{π}$	$\text{tan}\frac{\text{π}}{\text{2}}=\infty$

Substitute $\frac{\text{2u}}{{\text{1+u}}^{\text{2}}}$ for $\text{sinθ and }\frac{\text{2du}}{{\text{1+u}}^{\text{2}}}\text{ for dθ in T = }{\displaystyle \underset{\text{-π}}{\overset{\text{π}}{\int }}\frac{\text{dθ}}{\text{ω-asinθ}}}$.

$\text{T = 2}{\displaystyle \underset{\text{-π}}{\overset{\text{π}}{\int }}\frac{\text{du}}{{\text{ωu}}^{\text{2}}\text{ - 2au + ω}}}$

$\text{T = }{\displaystyle \underset{\text{-π}}{\overset{\text{π}}{\int }}\frac{\left(\frac{\text{2du}}{{\text{1+u}}^{\text{2}}}\right)}{\text{ω - a}\left(\frac{\text{2u}}{{\text{1+u}}^{\text{2}}}\right)}}$

$\text{ = 2}{\displaystyle \underset{\text{-}\infty }{\overset{\infty }{\int }}\frac{\text{du}}{\text{ω}\left({\text{1+u}}^{\text{2}}\right)\text{ - (a)(2u)}}}$

$\text{ = 2}{\displaystyle \underset{\text{-}\infty }{\overset{\infty }{\int }}\frac{\text{du}}{{\text{ωu}}^{\text{2}}\text{ - 2au + ω}}}$

$\text{T = 2}{\displaystyle \underset{\text{-π}}{\overset{\text{π}}{\int }}\frac{\text{du}}{{\text{ωu}}^{\text{2}}\text{ - 2au + ω}}}$

Hence, the expression for T as an integral with respect to u is

$\text{T = 2}{\displaystyle \underset{\text{-π}}{\overset{\text{π}}{\int }}\frac{\text{du}}{{\text{ωu}}^{\text{2}}\text{ - 2au + ω}}}$

(e)

Complete the square in the denominator of the integrand of part (d).

From part (d),

$\text{T = 2}{\displaystyle \underset{\text{-}\infty }{\overset{\infty }{\int }}\frac{\text{du}}{{\text{ωu}}^{\text{2}}\text{ - 2au + ω}}}$

Simplify as,

$\text{T = 2}{\displaystyle \underset{\text{-}\infty }{\overset{\infty }{\int }}\frac{\text{du}}{\text{ω}\left({\text{u}}^{\text{2}}\text{ - 2}\frac{\text{a}}{\text{ω}}\text{ + 1}\right)}}$

$\text{= }\frac{\text{2}}{\text{ω}}{\displaystyle \underset{\text{-}\infty }{\overset{\infty }{\int }}\frac{\text{du}}{{\text{u}}^{\text{2}}\text{ - 2u}\frac{\text{a}}{\text{ω}}\text{+}\frac{{\text{a}}^{\text{2}}}{{\text{ω}}^{\text{2}}}\text{ + }\left(\text{1-}\frac{{\text{a}}^{\text{2}}}{{\text{ω}}^{\text{2}}}\right)}}$

$\text{= }\frac{\text{2}}{\text{ω}}{\displaystyle \underset{\text{-}\infty }{\overset{\infty }{\int }}\frac{\text{du}}{\left(\text{u - }\frac{\text{a}}{\text{ω}}\right)\text{ + }\left(\text{1-}\frac{{\text{a}}^{\text{2}}}{{\text{ω}}^{\text{2}}}\right)}}$

Let $\text{x = u-}\frac{\text{a}}{\text{ω}}\text{, r = 1-}\frac{{\text{a}}^{\text{2}}}{{\text{ω}}^{\text{2}}}$

Then,

$\begin{array}{l}\text{u = x + }\frac{\text{a}}{\text{ω}}\\ \text{du = dx+0}\\ \text{du = dx}\end{array}$

So, replace $\text{du = dx}$, $\text{x = u - }\frac{\text{a}}{\text{ω}}\text{, r = 1-}\frac{{\text{a}}^{\text{2}}}{{\text{ω}}^{\text{2}}}$.

$\begin{array}{l}\text{T = }\frac{\text{2}}{\text{ω}}{\displaystyle \underset{\text{-}\infty }{\overset{\infty }{\int }}\frac{\text{dx}}{{\text{x}}^{\text{2}}\text{ + r}}}\\ \text{ = }\frac{\text{2}}{\text{ω}}{\displaystyle \underset{\text{-}\infty }{\overset{\infty }{\int }}\frac{\text{dx}}{{\text{x}}^{\text{2}}\text{ + }{\left(\sqrt{\text{r}}\right)}^2}}\end{array}$

Put the limits.

$\begin{array}{l}\text{T = }\frac{\text{2}}{\text{ω}}\frac{\text{1}}{\sqrt{\text{r}}}\left\{\left[{\text{tan}}^{\text{-1}}\text{(}\infty \text{)}\right]\text{-}\left[{\text{tan}}^{\text{-1}}\text{(-}\infty \text{)}\right]\right\}\\ \text{ = }\frac{\text{2}}{\text{ω}\sqrt{\text{r}}}\left[\left(\frac{\text{π}}{\text{2}}\right)\text{-}\left(\frac{\text{π}}{\text{2}}\right)\right]\\ \text{ = }\frac{\text{2π}}{\text{ω}\sqrt{\text{r}}}\end{array}$

Replace $\text{r = 1-}\frac{{\text{a}}^{\text{2}}}{{\text{ω}}^{\text{2}}}$.

$\text{T = }\frac{\text{2π}}{\text{ω}\sqrt{\text{1-}\frac{{\text{a}}^{\text{2}}}{{\text{ω}}^{\text{2}}}}}$

$\text{ = }\frac{\text{2π}}{\text{ω}\sqrt{\frac{{\text{ω}}^{\text{2}}{\text{-a}}^{\text{2}}}{{\text{ω}}^{\text{2}}}}}$.

$\text{= }\frac{\text{2π}}{\sqrt{{\text{ω}}^{\text{2}}{\text{-a}}^{\text{2}}}}$

Hence, the period of oscillation for the non-uniform oscillator is $\text{T = }\frac{\text{2π}}{\sqrt{{\text{ω}}^{\text{2}}{\text{-a}}^{\text{2}}}}$.