
Concept explainers
Logistic Growth Animal populations are not capable of unrestricted growth because of limited habitat and food supplies. Under such conditions the population follows a logistic growth model:
where c, d, and k are positive constants. For a certain fish population in a small pond d = 1200, k = 11, c = 0.2, and t is measured in years. The fish were introduced into the pond at time t = 0.
- (a) How many fish were originally put in the pond?
- (b) Find the population after 10, 20, and 30 years.
- (c) Evaluate P(t) for large values of t. What value does the population approach as t → ∞? Does the graph shown confirm your calculations?
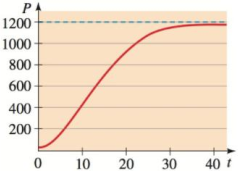
(a)
The numbers of fish were originally put in the pond.
Answer to Problem 25E
The numbers of fish were originally put in the pond is
Explanation of Solution
Given:
The fish population follows the logistic growth model
Where,
- c, d and k are positive constants.
Calculations:
The fish population follows the logistic growth model
Substitute 0 for t, 1200 for d, 0.2 for c 11 for k in the above formula to calculate the numbers of fish were originally put in the pond.
Hence, the numbers of fish were originally put in the pond is
(b)
The population of fish in the pond after 10, 20 and 30 years.
Answer to Problem 25E
The population fish after 30 years in the pond. is
Explanation of Solution
Given:
The fish population follows the logistic growth model
Where,
- c, d and k are positive constants.
Calculations:
The fish population follows the logistic growth model
Substitute 10 for t, 1200 for d, 0.2 for c 11 for k in the above formula to calculate the population of fish after 10 years in the pond.
Hence, the population fish after 10 years in the pond. is
Now, Substitute 20 for t, 1200 for d, 0.2 for c 11 for k in the above formula to calculate the population of fish after 20 years in the pond.
Hence, the population fish after 20 years in the pond. is
Now, Substitute 30 for t, 1200 for d, 0.2 for c 11 for k in the above formula to calculate the population of fish after 30 years in the pond.
Hence, the population fish after 30 years in the pond. is
(c)
To Evaluate: The population of fish for larger value of t.
Answer to Problem 25E
The population of fish for larger value of t is
Explanation of Solution
Given:
The fish population follows the logistic growth model shown below,
Calculation:
The fish population follows the logistic growth model shown below,
The graph of the function
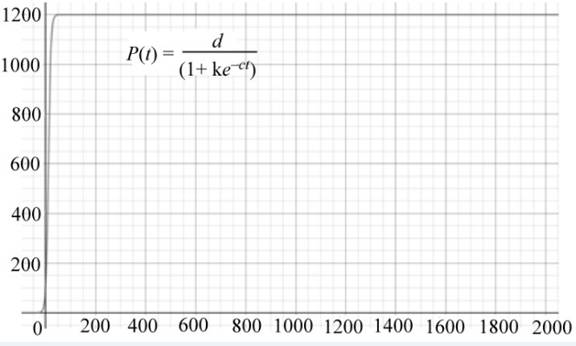
Figure (1)
From the Figure (1) 1200 is the highest population at
Confirm the highest population 1200 By calculation,
Substitute
Hence, the population of fish for larger value of t is
Chapter 4 Solutions
Precalculus: Mathematics for Calculus - 6th Edition
- Cancel Done RESET Suppose that R(x) is a polynomial of degree 7 whose coefficients are real numbers. Also, suppose that R(x) has the following zeros. -1-4i, -3i, 5+i Answer the following. (a) Find another zero of R(x). ☐ | | | | |│ | | | -1 བ ¢ Live Adjust Filters Croparrow_forwardSuppose that R (x) is a polynomial of degree 7 whose coefficients are real numbers. Also, suppose that R (x) has the following zeros. -1-4i, -3i, 5+i Answer the following. (c) What is the maximum number of nonreal zeros that R (x) can have? ☐arrow_forwardSuppose that R (x) is a polynomial of degree 7 whose coefficients are real numbers. Also, suppose that R (x) has the following zeros. -1-4i, -3i, 5+i Answer the following. (b) What is the maximum number of real zeros that R (x) can have? ☐arrow_forward
- i need help please dont use chat gptarrow_forward3.1 Limits 1. If lim f(x)=-6 and lim f(x)=5, then lim f(x). Explain your choice. x+3° x+3* x+3 (a) Is 5 (c) Does not exist (b) is 6 (d) is infinitearrow_forward1 pts Let F and G be vector fields such that ▼ × F(0, 0, 0) = (0.76, -9.78, 3.29), G(0, 0, 0) = (−3.99, 6.15, 2.94), and G is irrotational. Then sin(5V (F × G)) at (0, 0, 0) is Question 1 -0.246 0.072 -0.934 0.478 -0.914 -0.855 0.710 0.262 .arrow_forward
- 2. Answer the following questions. (A) [50%] Given the vector field F(x, y, z) = (x²y, e", yz²), verify the differential identity Vx (VF) V(V •F) - V²F (B) [50%] Remark. You are confined to use the differential identities. Let u and v be scalar fields, and F be a vector field given by F = (Vu) x (Vv) (i) Show that F is solenoidal (or incompressible). (ii) Show that G = (uvv – vVu) is a vector potential for F.arrow_forwardA driver is traveling along a straight road when a buffalo runs into the street. This driver has a reaction time of 0.75 seconds. When the driver sees the buffalo he is traveling at 44 ft/s, his car can decelerate at 2 ft/s^2 when the brakes are applied. What is the stopping distance between when the driver first saw the buffalo, to when the car stops.arrow_forwardTopic 2 Evaluate S x dx, using u-substitution. Then find the integral using 1-x2 trigonometric substitution. Discuss the results! Topic 3 Explain what an elementary anti-derivative is. Then consider the following ex integrals: fed dx x 1 Sdx In x Joseph Liouville proved that the first integral does not have an elementary anti- derivative Use this fact to prove that the second integral does not have an elementary anti-derivative. (hint: use an appropriate u-substitution!)arrow_forward
 Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
 Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning





